Short Answers
Q.1. Can an object be in pure translation as well as in pure rotation?
Yes, such motion is possible if the translation takes place along the axis of rotation. This type of motion is called screw motion.
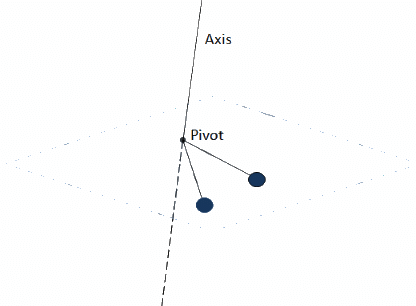
Q.2. A simple pendulum is a point mass suspended by a light thread from a fixed point. The particle is displaced towards one side and then released. It makes small oscillations. Is the motion of such a simple pendulum a pure rotation? If yes, where is the axis of rotation?
Yes, it is an example of pure rotation.
The axis of rotation passes through the pivot of the pendulum, perpendicular to the plane containing the pendulum.
Q.3. In a rotating body, 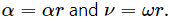 Thus
Thus 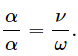 Can you use the theorems of ration and proportion studied in algebra so as to write
Can you use the theorems of ration and proportion studied in algebra so as to write 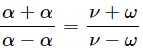
No, we cannot use componendo-dividendo theorem of proportion here. This is because α and a, and v and ω are dimensionally different. Therefore, v + ω and/or α + a are not possible.
Q.4. A ball is whirled in a circle by attaching it to a fixed point with a string. Is there an angular rotation of the ball about its centre? If yes, is this angular velocity equal to the angular velocity of the ball about the fixed point?
Yes, there is an angular rotation of the ball about its centre.
Yes, angular velocity of the ball about its centre is same as the angular velocity of the ball about the fixed point.
Explanation:-
Let the time period of angular rotation of the ball be T.
Therefore, we get
Angular velocity of the ball about the fixed point = 2π/T
After one revolution about the fixed centre is completed, the ball has come back to its original position. In this case, the point at which the ball meets with the string is again visible after one revolution. This means that it has undertaken one complete rotation about its centre.
The ball has taken one complete rotation about its centre. Therefore, we have
Angular displacement of the ball = 2π
Time period = T
So, angular velocity is again 2π/T.
Thus, in both the cases, angular velocities are the same.
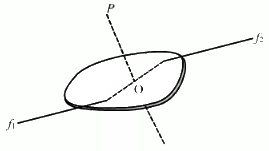
Q.5. The moon rotates about the earth in such a way that only one hemisphere of the moon faces the earth (see the following figure). Can we ever see the "other face" of the moon from the earth? Can a person on the moon ever see all the faces of the earth?
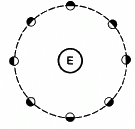
No, we cannot see the other face of the Moon from the Earth.
Yes, a person on the Moon can see all the faces of the Earth.
Explanation: Angular velocity of the Moon about its own axis of rotation is same as its angular velocity of revolution about the Earth. This means that its rotation time period equals its revolution time period. So, we can see only one face of the Moon from the Earth.
However, angular velocity of the Earth about its axis is not same as the angular velocity of Moon about the Earth. So, all the faces of the Earth is visible from the Moon.
Q.6. The torque of the weight of any body about any vertical axis is zero. If it always correct?
No, its not always correct.
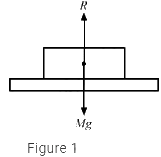
Explanation: If the centre of mass of the body is not on the same vertical line as the normal reaction R of the body, a net torque acts on the body about its vertical axis. In fig. 1, R and CM lies in the same vertical line. Thus, there is no torque about any vertical axis
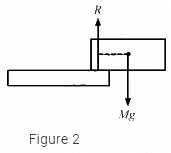
But in fig. 2, as R and CM do not lie along the same vertical line, there exists a torque about the vertical axis.
Q.7. The torque of a force 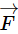 about a point is defined as
about a point is defined as 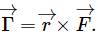 Suppose
Suppose  are all nonzero. Is
are all nonzero. Is 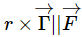 always true? Is it ever true?
always true? Is it ever true?
No, 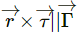 is not true.
is not true.
In fact, it is never true.This is because:
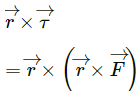
Applying vector triple product, we get:
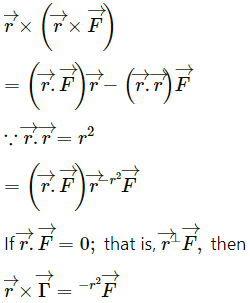
We know that r2 is never negative and 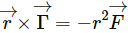
This implies that both vectors may be antiparallel to each other but not parallel.
Q.8. A heavy particle of mass m falls freely near the earth's surface. What is the torque acting on this particle about a point 50 cm east to the line of motion? Does this torque produce any angular acceleration in the particle?
We know that
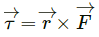
Given:-
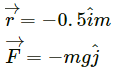
The torque becomes
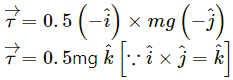
No, there will be no angular acceleration on the particle due to the torque.
Angular acceleration is given by 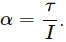 As the particle here moves in a straight line, the centre of rotation lies at a distance infinity
As the particle here moves in a straight line, the centre of rotation lies at a distance infinity  so, moment of inertia
so, moment of inertia  of the particle is infinity.
of the particle is infinity.
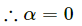
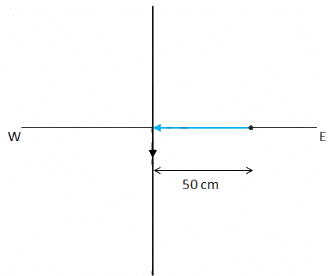
Q.9. If several forces act on a particle, the total torque on the particle may be obtained by first finding the resultant force and then taking torque of this resultant. Prove this. Is this result valid for the forces acting on different particles of a body in such a way that their lines of action intersect at a common point?
Let  be the forces acting on a point P.
be the forces acting on a point P.
Let O be the point along which torques \(moments) will be taken.
Let:-
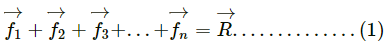
Moments of force (torque) 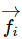 about O will be
about O will be
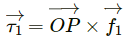
The sum of the torques about O will be
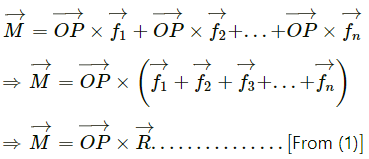
Thus, we see that the torque of the resultant force 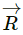 of the forces
of the forces 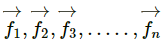 gives the sum of the moments of the torques.
gives the sum of the moments of the torques.
Q.10. If the sum of all the forces acting on a body is zero, is it necessarily in equilibrium? If the sum of all the forces on a particle is zero, is it necessarily in equilibrium?
No, if the sum of all the forces acting on a body is zero, the body is not necessarily in equilibrium . To be in equilibrium, the sum of torque acting on the body must be zero too (see the figure). In the above case, although the sum of the forces acting on the body is zero 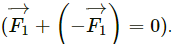 Still, the body will rotate along
Still, the body will rotate along  So, it won't remain in equilibrium.
So, it won't remain in equilibrium.
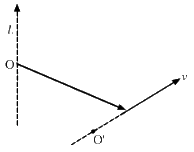
Q.11. If the angular momentum of a body is found to be zero about a point, is it necessary that it will also be zero about a different point?
No, angular momentum is dependent on the position vector of the particle, angle between the radius vector and the linear velocity of the particle. So, there may be finite angular momentum along any different point even if it is zero at a particular point. If angular momentum is zero along O' but finite along O.
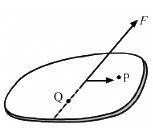
Q.12. If the resultant torque of all the forces acting on a body is zero about a point, is it necessary that it will be zero about any other point?
No, it is not necessary that the torque about any other point be zero if it is zero about one point.
Let 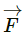 be the resultant force due to all the forces acting on the plane of the body. Therefore, torque due to force \vec{F} at any point will be the resultant torque . Now, we see that the torque due to
be the resultant force due to all the forces acting on the plane of the body. Therefore, torque due to force \vec{F} at any point will be the resultant torque . Now, we see that the torque due to 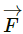 at point Q will be zero because Q lies on the line of support of the force F but the torque due to force
at point Q will be zero because Q lies on the line of support of the force F but the torque due to force 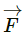 will not be zero along the point P.
will not be zero along the point P.
Q.13. A body is in translational equilibrium under the action of coplanar forces. If the torque of these forces is zero about a point, is it necessary that it will also be zero about any other point?
Yes , if the torque due to forces in translation equillibrium is zero about a point, it will be zero about other point in the plane.
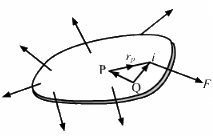
Let us consider a planner lamina of some mass, acted upon by forces  etc.
etc.
Let a force 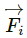 act on a ith particle and torque due to
act on a ith particle and torque due to 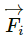 be zero at a point Q.
be zero at a point Q.
Since the body is in translation equillibrium, we have
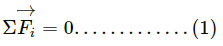
Again, torque about P is zero . Therefore, we have
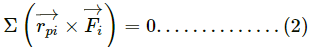
Now, torque about point Q will be
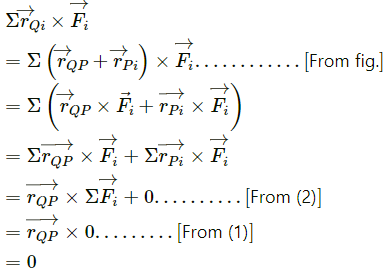
Thus, 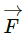 is zero about any other point Q.
is zero about any other point Q.
Q.14. A rectangular brick is kept on a table with a part of its length projecting out. It remains at rest if the length projected is slightly less than half the total length but it falls down if the length projected is slightly more than half the total length. Give reason.
The centre of mass (CM) of a rectangular block lies in the middle of the block . When the block is projected less than half of its length (CM being over the table), no net force acts on it . Thus, no net torque acts upon the body . But if the block is projected more than half of its length outside the table (CM being outside the table), gravitational force acts along the CM of the block . This force produces a moment along the edge of the table . This rotates the block, and as a result, it falls down.
Q.15. When a fat person tries to touch his toes, keeping the legs straight, he generally falls. Explain with reference to the following figure.
When the man tries to touch his toe, he exerts force along the hand downwards . This force produces a moment along the Centre of Mass (CM) of the man as shown in the figure. This moment makes him rotate and, thus, he falls down after losing the balance.

Q.16. A ladder is resting with one end on a vertical wall and the other end on a horizontal floor. If it more likely to slip when a man stands near the bottom or near the top?
The ladder is more likely to slide when the man stands near the top. This is because when the man stands near the top, it creates more torque compared to the torque caused by the weight of man near the bottom.
When the man stands near the bottom, the Centre of Gravity of the ladder is shifted to C' from C. Now, the couple due to forces (m + M)g and N makes the ladder fall . We see that due to its shift from C to C' the moment arm of the couple decreases from r to r'; hence, the couple decreases.
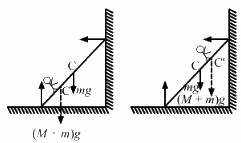
When the man stands near the top of the ladder, the Centre of Mass shifts from C to C'. This increases the moment arm of the couple and from r to r'.
Increase in moment arm increases the couple and thus, the ladder easily falls.
Q.17. When a body is weighed on an ordinary balance we demand that the arum should be horizontal if the weights on the two pans are equal. Suppose equal weights are put on the two pans, the arm is kept at an angle with the horizontal and released. Is the torque of the two weights about the middle point (point of support) zero? Is the total torque zero? If so, why does the arm rotate and finally become horizontal?
When the balance is kept at an angle, there is a net extra torque given to one of its arm. When the extra torque is removed, the balance becomes torque free and sum of all the torque acting on it is zero.
But balance kept at an angle has got a greater potential energy compared to the balance kept horizontal. The potential energy acquired is due to the initial torque applied on it. This displaces the balance by an angle. As soon as the body is set free to rotate, the body tends to have the lowest potential energy. Thus, potential energy starts converting in to kinetic energy, but on the other side, kinetic energy converts into potential energy when the other arm of the balance is raised. This energy transformation oscillates the balance. But in this process, friction with the air and fulcrum dissipates energy converting into heat. Finally, the balance loses the energy and becomes horizontal, or attains equilibrium.
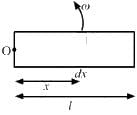
Q.18. The density of a rod AB continuously increases from A to B. Is it easier to set it in rotation by clamping it at A and applying a perpendicular force at B or by clamping it at B and applying the force at A?
It will require more force to set the bar into rotation by clamping at A and then clamping at B.

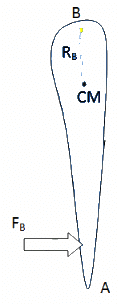
Explanation:- Since the rod has mass density increasing towards B, the Center of Mass (CM) of the rod is near B. If the rod is clamped along A, the distance of CM of the rod from the pivot will be greater when the rod is clamped along B. Greater distance of CM from the Center of rotation increases the moment of inertia of the rod and hence more torque will be necessary to rotate the bar about A. Greater torque implies greater force will be necessary to rotate it.
FA = Force required to rotated the rod clamped at A
RA= Distance of CM from pivot A
M = Mass of the rod
FB = Force required to rotate the rod clamped at B
RB= Distance of CM from pivot B
We have RA>RB.
We have to find the torque required to rotate rod clamped at A to produce angular acceleration a.
TA = MRA2a = RAFA
⇒ FA = MRAa
We have to find torque required to rotate rod clamped at B to produce angular acceleration a.
TB = MRB2a = RBFB
⇒ FB = MRBa
On comparing, since RA>RB, we get
FA>FB
Q.19. When tall building are constructed on earth, the duration of day-night slightly increases. Is it true?
Yes, because tall buildings have their CM much above the ground. It increases moment of inertia of the Earth. As the Earth’s rotation does not involve torque, its angular momentum is constant. Thus, an increase in MI leads to lower angular velocity of the Earth about its axis of rotation. This means length of night and day will increase. However, the increase is very small.
Q.20. If the ice at the poles melts and flows towards the equator, how will it affect the duration of day-night?
Ice caps near the poles concentrate the mass of water at the poles through which axis of rotation of the Earth passes. If the ice melts, water will spread across the globe due to hydrostatic equilibrium and tend to move to the equatorial areas of the Earth due to centrifugal force of rotation. Mass, now being distributed more along the equator, will increase MI of the Earth and this in turn will decrease the angular velocity of the Earth. Decrease in angular velocity will increase the duration of day-night.
Q.21. A hollow sphere, a solid sphere, a disc and a ring all having same mass and radius are rolled down on an inclined plane. If no slipping takes place, which one will take the smallest time to cover a given length?
The body with the smallest moment of inertia will roll down taking the smallest time. Here, the solid sphere has the lowest moment of inertia among all the other bodies. So, it will roll down taking the least time.
Q.22. A sphere rolls on a horizontal surface. If there any point of the sphere which has a vertical velocity?
Some points on the equator of the sphere has got vertical velocity with respect to the direction of motion of the sphere.
Multiple Choice Questions
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
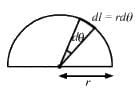
Try yourself:The moment of inertia of a uniform semicircular wire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is ___________ .
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Let 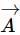 be a unit vector along the axis of rotation of a purely rotating body and
be a unit vector along the axis of rotation of a purely rotating body and 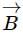 be a unit vector along the velocity of a particle P of the body away from the axis. The value of
be a unit vector along the velocity of a particle P of the body away from the axis. The value of 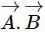 is ____________ .
is ____________ .
Explanation
For a purely rotating body, the axis of rotation is always perpendicular to the velocity of the particle.
Therefore, we have 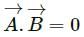
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A body is uniformly rotating about an axis fixed in an inertial frame of reference. Let 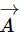 be a unit vector along the axis of rotation and
be a unit vector along the axis of rotation and 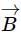 be the unit vector along the resultant force on a particle P of the body away from the axis. The value of
be the unit vector along the resultant force on a particle P of the body away from the axis. The value of 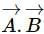 is _________.
is _________.
Explanation
The unit vector along the axis of rotation and the unit vector along the resultant force on the particle are perpendicular to each other in a uniform rotation.
Therefore, we have
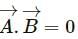
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A particle moves with a constant velocity parallel to the X-axis. Its angular momentum with respect to the origin ____________.
Explanation
For angular momentum, we have
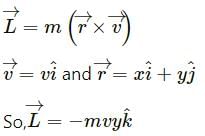
m, v and y are constant; therefore, angular momentum remains constant.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A body is in pure rotation. The linear speed v of a particle, the distance r of the particle from the axis and the angular velocity ω of the body are related as ω = v/r. Thus
Explanation
In a pure rotation, angular velocity of all the particles remains same and does not depend on the position of the particle from the axis of rotation.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:The following figure shows a small wheel fixed coaxially on a bigger one of double the radius. The system rotates about the common axis. The strings supporting A and B do not slip on the wheels. If x and y be the distance travelled by A and B in the same time interval, then _________ .
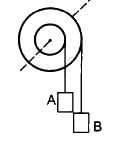
Explanation
It is given that angular velocity is same for both the wheels.
Therefore, we have
vA = ωR
vB = ω2R
x = vAt = ωRT ...(1)
y = vBt = ω(2R)t ...(2)
From equations (1) and (2), we get
y = 2x
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A body is rotating uniformly about a vertical axis fixed in an inertial frame. The resultant force on a particle of the body not on the axis is _________ .
Explanation
Because resultant force on a particle of the body rotating uniformly is always perpendicular to the rotation axis and pass through it.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A body is rotating nonuniformity about a vertical axis fixed in an inertial frame. The resultant force on a particle of the body not on the axis is _________ .
Explanation
The resultant force on a particle of the body rotating non-uniformly is always horizontal and skew with the rotation axis because net torque on the body is non-zero.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Let 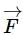 be a force acting on a particle having position vector
be a force acting on a particle having position vector 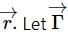 be the torque of this force about the origin, then __________ .
be the torque of this force about the origin, then __________ .
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:One end of a uniform rod of mass m and length l is clamped. The rod lies on a smooth horizontal surface and rotates on it about the clamped end at a uniform angular velocity ω. The force exerted by the clamp on the rod has a horizontal component
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A uniform rod is kept vertically on a horizontal smooth surface at a point O. If it is rotated slightly and released, it falls down on the horizontal surface. The lower end will remain _________ .
Explanation
It is given that there is no force along x-axis.
COM of rod will remain and will not shift along x-axis (horizontal direction).
Force gravity is acting along y-axis (vertical direction). So, COM will shift along the y-axis by l/2distance and COM of horizontal rod is at a distance l/2 from one end. Therefore, lower end of the rod will remain at a distance l/2 from O.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A circular disc A of radius r is made from an iron plate of thickness t and another circular disc B of radius 4r is made from an iron plate of thickness t/4. The relation between the moments of inertia IA and IB is __________ .
Explanation
Moment of inertia of circular disc of radius r:
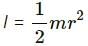
Mass = Volume × Density
Volume of disc = πr2t
Here, t is the thickness of the disc.
As density is same for both the rods, we have
Moment of inertia,
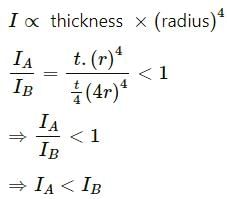
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Equal torques act on the disc A and B of the previous problem, initially both being at rest. At a later instant, the linear speeds of a point on the rim of A and another point on the rim of B are vA and vB respectively. We have
Explanation
τ = Iα (magnitude)
For equal torque, we have
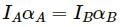
IA < IB
⇒ αA > αB ...(1)
Now,
ω = αt
Or,
v/r = αt
vA > vB ...(Using (1))
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A closed cylindrical tube containing some water (not filling the entire tube) lies in a horizontal plane. If the tube is rotated about a perpendicular bisector, the moment of inertia of water about the axis __________ .
Explanation
Moment of inertia of a mass is directly proportional to the square of the distance of mass from the axis of rotation.
Therefore, we have
I α r2
As the tube is rotated, water is collected at the end of tube because of centrifugal force and distance from the rotation axis increases. Hence, moment of inertia increases.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Let I1 an I2 be the moments of inertia of two bodies of identical geometrical shape, the first made of aluminium and the second of iron.
Explanation
In the given case, we have
MOI
∝ Density
The density of iron is more; therefore, I2 will be greater.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A body having its centre of mass at the origin has three of its particles at (a,0,0), (0,a,0), (0,0,a). The moments of inertia of the body about the X and Y axes are 0⋅20 kg-m2 each. The moment of inertia about the Z-axis
Explanation
Ix = m2a2 + m3a2 = 0.20 ......(1)
Iy = m1a2 + m3a2 = 0.20 ......(2)
Iz = m1a2 + m2a2 ......(3)
We have three equations and four variables. So, Iz cannot be deduced with the given information.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
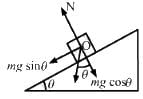
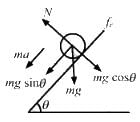
Try yourself:A cubical block of mass M and edge a slides down a rough inclined plane of inclination θ with a uniform velocity. The torque of the normal force on the block about its centre has a magnitude
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A thin circular ring of mass M and radius r is rotating about its axis with an angular speed ω. Two particles having mass m each are now attached at diametrically opposite points. The angular speed of the ring will become
Explanation
No external torque is applied on the ring; therefore, the angular momentum will be conserved.
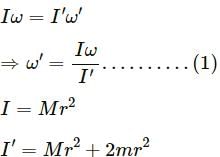
On putting these values in equation (1), we get
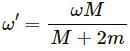
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A person sitting firmly over a rotating stool has his arms stretched. If he folds his arms, his angular momentum about the axis of rotation ___________ .
Explanation
Rate of change of angular momentum of the body is directly proportional to the net external torque acting on the body.
No external torque is applied on the person or on the table; therefore, the angular momentum will be conserved.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
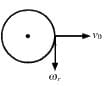
Try yourself:The centre of a wheel rolling on a plane surface moves with a speed v0 A particle on the rim of the wheel at the same level as the centre will be moving at speed ___________ .
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A wheel of radius 20 cm is pushed to move it on a rough horizontal surface. If is found to move through a distance of 60 cm on the road during the time it completes one revolution about the centre. Assume that the linear and the angular accelerations are uniform. The frictional force acting on the wheel by the surface is ___________ .
Explanation
As the distance covered in one revolution about the centre is less than the perimeter of the wheel, it means that the direction of torque due to frictional force opposes the motion of wheel, i.e., the frictional force acting on the wheel by the surface is along the velocity of the wheel.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:The angular velocity of the engine (and hence of the wheel) of a scooter is proportional to the petrol input per second. The scooter is moving on a frictionless road with uniform velocity. If the petrol input is increased by 10%, the linear velocity of the scooter is increased by ___________ .
Explanation
On a frictionless road, we have
Angular velocity of the engine = 0
Therefore, increase in petrol input will not affect the angular velocity and hence the linear velocity of the scooter will remain the same.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A solid sphere, a hollow sphere and a disc, all having same mass and radius, are placed at the top of a smooth incline and released. Least time will be taken in reaching the bottom by _________ .
Explanation
The incline is smooth; therefore, all bodies will slip on the incline. Also, as the mass of bodies is same, they will reach the bottom in equal time.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A solid sphere, a hollow sphere and a disc, all having same mass and radius, are placed at the top on an incline and released. The friction coefficients between the objects and the incline are same and not sufficient to allow pure rolling. Least time will be taken in reaching the bottom by ___________ .
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:In the previous question, the smallest kinetic energy at the bottom of the incline will be achieved by ___________ .
Explanation
Torque is same for all the bodies; therefore, the angular momentum will be conserved.
Now, total kinetic energy = 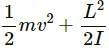
So, the body with greater value of moment of inertia will have smallest kinetic energy at the bottom of the incline.
Order of the moment of inertia of the bodies:-
hollow sphere > disc > solid sphere
Hence, the hollow sphere will have the smallest kinetic energy at the bottom.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A string of negligible thickness is wrapped several times around a cylinder kept on a rough horizontal surface. A man standing at a distance l from the cylinder holds one end of the string and pulls the cylinder towards him (see the following figure). There is no slipping anywhere. The length of the string passed through the hand of the man while the cylinder reaches his hands is _________ .
Explanation
Report a problem
*Multiple options can be correct
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:The axis of rotation of a purely rotating body
Explanation
It is not necessary that the axis of rotation of a purely rotating body should pass through the centre of mass or through a particle of the body. It can also lie outside the body.
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Consider the following two equations
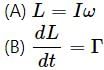
In noninertial frames _____.
Explanation
In non-inertial frames,
Here,
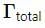 is the total torque on the system due to all the external forces acting on the system. So, equation (B) is not true as in non-inertial frames, pseudo force must be applied to study the motion of the object.
is the total torque on the system due to all the external forces acting on the system. So, equation (B) is not true as in non-inertial frames, pseudo force must be applied to study the motion of the object.
Report a problem
*Multiple options can be correct
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A particle moves on a straight line with a uniform velocity. Its angular momentum __________ .
*Multiple options can be correct
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:If there is no external force acting on a nonrigid body, which of the following quantities must remain constant?
Explanation
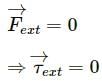
That is, the change in linear momentum and angular momentum is zero. This is because:-
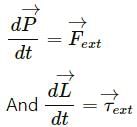
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Let IA and IB be moments of inertia of a body about two axes A and B respectively. The axis A passes through the centre of mass of the body but B does not.
Explanation
If axes A and B are parallel, we get
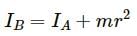
Here, r is the distance between two axes and m is the mass of the body.
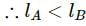
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A sphere is rotating about a diameter.
Explanation
Linear acceleration of a rotating particle is given as
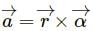
The sphere is rotating about a diameter; therefore, the position vector of the particles on the diameter is zero. Thus, linear acceleration of the particle is zero.
Explanation of other options:- All the particles of the body have the same angular velocity. All the particle on the surface have different linear speeds that depend on the position of the particle from the axis of rotation.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:The density of a rod gradually decreases from one end to the other. It is pivoted at an end so that it can move about a vertical axis though the pivot. A horizontal force F is applied on the free end in a direction perpendicular to the rod. The quantities, that do not depend on which end of the rod is pivoted, are ______ .
Explanation
The torque of the applied force does not depend on the density of a rod. It depend on the distance between the pivot and the point where F is applied. So, it does not depend on which end of the rod is pivoted.
Report a problem
*Multiple options can be correct
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
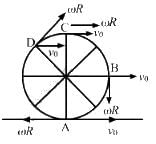
Try yourself:Consider a wheel of a bicycle rolling on a level road at a linear speed v0 (see the following figure)
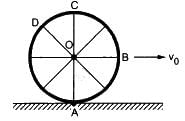
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:Two uniform solid spheres having unequal masses and unequal radii are released from rest from the same height on a rough incline. If the spheres roll without slipping, ____ .
Explanation
Acceleration of a sphere on the incline plane is given by
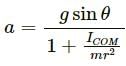
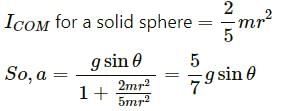
a is independent of mass and radii; therefore, the two spheres reach the bottom together.
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A hollow sphere and a solid sphere having same mss and same radii are rolled down a rough inclined plane.
Explanation
Report a problem
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
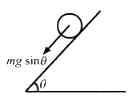
Try yourself:A sphere cannot roll on
Explanation
A sphere cannot roll on a smooth inclined surface and on a smooth horizontal surface because there is no backward force (force of friction) to prevent its slipping.
Report a problem
*Multiple options can be correct
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:In rear-wheel drive cars, the engine rotates the rear wheels and the front wheels rotate only because the car moves. If such a car accelerates on a horizontal road the friction
Explanation
(a) On the rear wheels, friction force is in the forward direction because it favours the motion and accelerates the car in forward direction.
(b) Because of the movement of the car in forward direction, front wheels push the road in forward direction and in reaction, the road applies friction force in the backward direction.
(c) As the car is moving in forward direction, the rear wheels have larger magnitude of friction force (in forward direction) than on the front wheels.
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A sphere can roll on a surface inclined at an angle θ if the friction coefficient is more than 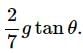 Suppose the friction coefficient is
Suppose the friction coefficient is 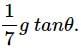 If a sphere is released from rest on the incline, ______.
If a sphere is released from rest on the incline, ______.
Explanation
The given coefficient of friction 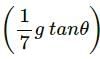 is less than the coefficient friction
is less than the coefficient friction 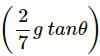 required for perfect rolling of the sphere on the inclined plane.
required for perfect rolling of the sphere on the inclined plane.
Therefore, sphere may slip while rolling and it will translate and rotate about the centre.
Report a problem
*Multiple options can be correct
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:A sphere is rolled on a rough horizontal surface. If gradually slows down and stops. The force of friction tries to
Explanation
If a sphere is rolled on a rough horizontal surface, the force of friction tries to oppose the linear motion and favours the angular motion.
Question for HC Verma Questions and Solutions: Chapter 10: Rotational Mechanics- 1
Try yourself:The following figure shows a smooth inclined plane fixed in a car accelerating on a horizontal road. The angle of incline θ is related to the acceleration a of the car as a = g tanθ. If the sphere is set in pure rolling on the incline, _____.
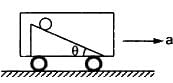
Explanation
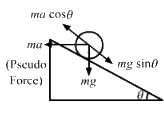
From the free body diagram of sphere, we have
Net force on the sphere along the incline,
Fnet = mgsinθ − macosθ ...(i)
On putting a = gtanθ in equation (i), we get
Fnet = 0
Therefore, if the sphere is set in pure rolling on the incline, it will continue pure rolling.
Report a problem

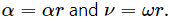 Thus
Thus 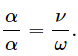 Can you use the theorems of ration and proportion studied in algebra so as to write
Can you use the theorems of ration and proportion studied in algebra so as to write 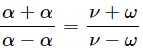

 about a point is defined as
about a point is defined as 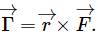 Suppose
Suppose  are all nonzero. Is
are all nonzero. Is 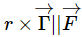 always true? Is it ever true?
always true? Is it ever true?is not true.
As the particle here moves in a straight line, the centre of rotation lies at a distance infinity
so, moment of inertia
of the particle is infinity.
be the forces acting on a point P.
about O will be
of the forces
gives the sum of the moments of the torques.
Still, the body will rotate along
So, it won't remain in equilibrium.

be the resultant force due to all the forces acting on the plane of the body. Therefore, torque due to force \vec{F} at any point will be the resultant torque . Now, we see that the torque due to
at point Q will be zero because Q lies on the line of support of the force F but the torque due to force
will not be zero along the point P.
etc.
act on a ith particle and torque due to
be zero at a point Q.
is zero about any other point Q.



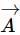 be a unit vector along the axis of rotation of a purely rotating body and
be a unit vector along the axis of rotation of a purely rotating body and 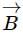 be a unit vector along the velocity of a particle P of the body away from the axis. The value of
be a unit vector along the velocity of a particle P of the body away from the axis. The value of 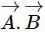 is ____________ .
is ____________ .
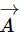 be a unit vector along the axis of rotation and
be a unit vector along the axis of rotation and 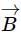 be the unit vector along the resultant force on a particle P of the body away from the axis. The value of
be the unit vector along the resultant force on a particle P of the body away from the axis. The value of 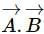 is _________.
is _________.

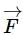 be a force acting on a particle having position vector
be a force acting on a particle having position vector 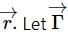 be the torque of this force about the origin, then __________ .
be the torque of this force about the origin, then __________ .
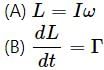

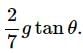 Suppose the friction coefficient is
Suppose the friction coefficient is 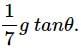 If a sphere is released from rest on the incline, ______.
If a sphere is released from rest on the incline, ______.









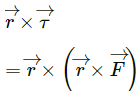
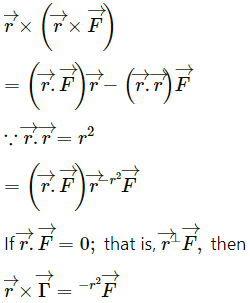
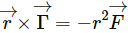
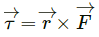
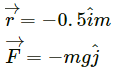
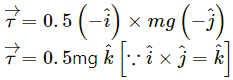
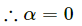


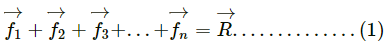
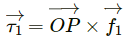
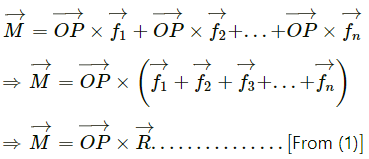



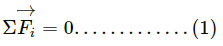
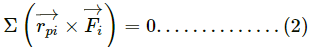
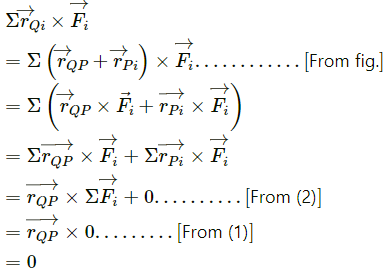




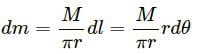

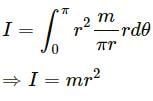
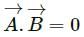
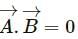
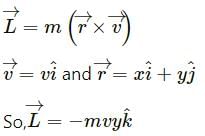
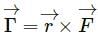
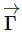 is perpendicular to
is perpendicular to 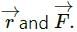

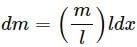
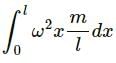
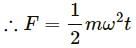
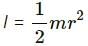
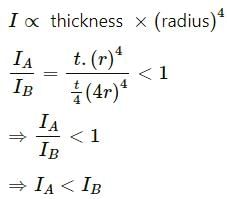
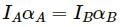

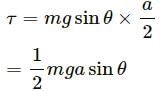
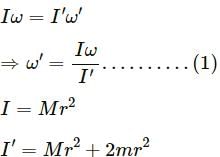
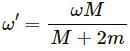
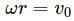
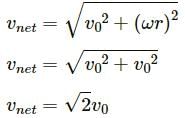
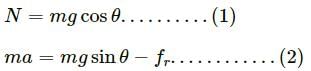
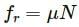 in (2) we get,
in (2) we get,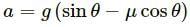
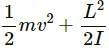
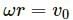
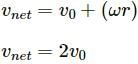
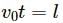
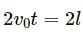
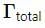 is the total torque on the system due to all the external forces acting on the system. So, equation (B) is not true as in non-inertial frames, pseudo force must be applied to study the motion of the object.
is the total torque on the system due to all the external forces acting on the system. So, equation (B) is not true as in non-inertial frames, pseudo force must be applied to study the motion of the object.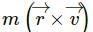
 will have the same direction and their cross product will be zero. Hence, angular momentum is zero.
will have the same direction and their cross product will be zero. Hence, angular momentum is zero. will not have the same direction and their cross product will not be zero. Hence, angular momentum is non-zero.
will not have the same direction and their cross product will not be zero. Hence, angular momentum is non-zero.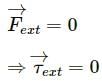
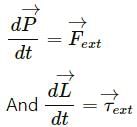
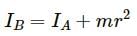
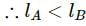
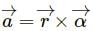
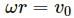
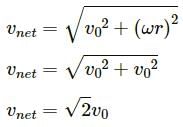
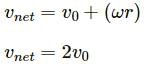
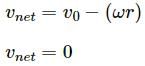
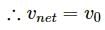
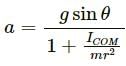
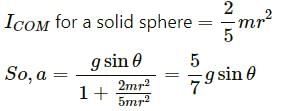
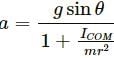
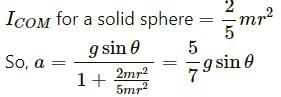
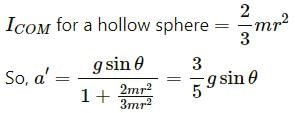

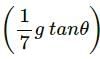 is less than the coefficient friction
is less than the coefficient friction 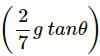 required for perfect rolling of the sphere on the inclined plane.
required for perfect rolling of the sphere on the inclined plane.




















