HC Verma Questions and Solutions: Chapter 41: Electric Current through Gases- 2 | HC Verma Solutions - JEE PDF Download
Exercises
Q.1. A discharge tube contains helium at low pressure. A large potential difference is applied across the tube. Consider a helium atom that has just been ionised due to the detachment of an atomic electron. Find the ratio of the distance travelled by the free electron to that by the positive ion in a short time dt after ionisation.
Charge on the electron,
- q = −1.6 × 10−19 C
Charge on the helium ion = +q = +1.6 × 10−19 C
Let the electric field in the tube be E.
Mass of electron, me = 9.1 × 10−31 kg
Mass of proton, mp = 1.67 × 10−27 kg
Mass of helium ion, = 4mp = 4×(1.67 × 10−27 ) kg
Magnitude of the force experienced by the electron,
F = qE
Magnitude of acceleration of the electron,
Distance travelled by the electron in time t,
Acceleration of the positive ion
Distance travelled by the helium ion,
The ratio of distance travelled by the given particles is given by
Q.2. A molecule of a gas, filled in a discharge tube, gets ionised when an electron is detached from it. An electric field of 5.0 kV m−1 exists in the vicinity of the event. (a) Find the distance travelled by the free electron in 1 µs, assuming there's no collision. (b) If the mean free path of the electron is 1.0 mm, estimate the time of transit of the free electron between successive collisions.
Given:
Electric field inside discharge tube, E = 5.0 kV/m
(a) Let S be distance travelled by the free electron in 1 µs.
(b) Let the time of transit of the free electron between successive collisions be t.
Q.3. The mean free path of electrons in a gas in a discharge tube is inversely proportional to the pressure inside it. The Crookes dark space occupies half the length of the discharge tube when the pressure is 0.02 mm of mercury. Estimate the pressure at which the dark space will fill the whole tube.
Let the mean free path of the electrons be L and pressure inside the tube be P.
It is given that the mean free path of electrons and the pressure inside the tube are related as:
L ∝ (1/P)
Here, L = half of tube's length
Pressure inside the tube, P = 0.02 mm of Hg
As pressure 'P' becomes half, mean free path of electrons, L, doubles. So, the whole tube is filled with Crookes dark space.
Thus, the pressure required for filling the whole tube with Crookes dark space,
P' = 0.02/2 = 0.01 mm of Hg
Q.4. Two discharge tubes have identical material structures and the same gas is filled in them. The length of one tube is 10 cm and that of the other tube is 20 cm. Sparking starts in both the tubes when the potential difference between the cathode and the anode is 100 V. If the pressure in the shorter tube is 1.0 mm of mercury, what is the pressure in the longer tube?
According to Paschen's equation, sparking potential (V) is a function of the product of pressure (P) inside the tube and the length (d) of the tube.
V = f(Pd)
As the sparking potential is same in both the tubes, the product of P and d must be equal for both the tubes.
Length of the smaller tube, ds = 10 cm
Pressure inside the smaller tube, Ps = 1.0 mm of mercury
Let the pressure inside the longer tube, Pl,, be x mm of mercury.
Length of the longer tube, dl = 20 cm
Hence, the pressure in the longer tube is 0.5 mm of Hg.
Q.5. Calculate n(T)/n(1000 K) for tungsten emitter at T = 300 K, 2000 K and 3000 K, where n(T) represents the number of thermions emitted per second by the surface at temperature T. Work function of tungsten is 4.52 eV.
According to Richardson-Dushman equation, the number of thermions (n) emitted by a surface, in a given time (t), is given by
Here,
Q.6. The saturation current from a thoriated-tungsten cathode at 2000 K is 100 mA. What will be the saturation current for a pure-tungsten cathode of the same surface area operating at the same temperature? The constant A in the Richardson-Dushman equation is 60 × 104 Am −2 K−2 for pure tungsten and 3.0 × 104 Am −2 k−2 for thoriated tungsten. The work function of pure tungsten is 4.5 eV and that of thoriated tungsten is 2.6 eV.
According to Richardson-Dushman equation, the current of thermions is given by
For the thoriated tungsten cathode:
Saturation current, i = 100 mA
Temperature, T = 2000 K
A = 3.0 × 104 Am−2K−2
ϕ = 2.6 eV.
For the pure tungsten cathode:
Let the saturation current be i.
Temperature, T = 2000 K
A = 60 × 104 Am −2 K−2
ϕ = 2.6 eV
k = 1.38× 10−23 J/K
Substituting the values of the quantities in the Richard-Dushman equation, we get:
Dividing equation (1) by (2)
Q.7. A tungsten cathode and a thoriated-tungsten cathode have the same geometric dimensions and are operated at the same temperature. The thoriated-tungsten cathode gives 5000 times more current than the other cathode. The constant A in the Richardson−Dushman equation is 60 × 104 Am −2 K−2 for pure tungsten and 3.0 × 104 Am −2 k−2 for thoriated tungsten. The work function of pure tungsten is 4.5 eV and that of thoriated tungsten is 2.6 eV. Find the operating temperature.
For the pure tungsten cathode,
work function, ϕ = 4.5 eV
A = 60 × 104 Am −2 K−2
For the thoriated-tungsten cathode
work function, ϕ = 2.6 eV
A = 3.0 × 104 Am−2K−2
Saturation current,
For thoriated tungsten,
So,
Taking 'ln' of both sides, we get:
Q.8. If the temperature of a tungsten filament is raised from 2000 K to 2010 K, by what factor does the emission current change? Work function of tungsten is 4.5 eV.
Given:
Work function of tungsten = 4.5 eV
Initial temperature of tungsten filament, T = 2000 K
Final temperature of tungsten filament, T' = 2010 K
Let the emission current at (T = 2000 K) be i.
Let the emission current at (T' = 2010 K) be i'.
The emission currents are given by
Dividing i by i', we get:
Q.9. The constant A in the Richardson−Dushman equation for tungsten is 60 × 104 A m−2K−2. The work function of tungsten is 4.5 eV. A tungsten cathode with a surface area 2.0 × 10−5 m2 is heated by a 24 W electric heater. In steady state, the heat radiated by the heater and the cathode equals the energy input by the heater and the temperature becomes constant. Assuming that the cathode radiates like a blackbody, calculate the saturation current due to thermions. Take Stefan's Constant = 6 × 10−8 W m−2 K−1. Assume that the thermions take only a small fraction of the heat supplied.
Given:
A = 60 × 104 A m−2 K−2
Work function of tungsten, ϕ = 4.5 eV
Stefan's Constant, σ = 6 × 10−8 W m−2 K−1
Surface area of tungsten cathode, S = 2.0 × 10−5 m2
Boltzmann's Constant, k = 1.38× 10−23 J/K
Heat supplied by the heater, H = 24 W
The cathode acts as a black body; thus, its emissivity is 1.
According Stefan's Law:
The power (P) radiated by a blackbody with surface area (A) and temperature (T),
According to the Richard-Dushmann equation, emission current,
Q.10. A plate current of 10 mA is obtained when 60 volts are applied across a diode tube. Assuming the Langmuir-Child relation 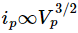 to hold, find the dynamic resistance rp in this operating condition.
to hold, find the dynamic resistance rp in this operating condition.
According to Lamgmuir-Child Law, the relation between plate current (ip) and the plate voltage (Vp) is given by
...(1)
Differentiating equation (1) with respect Vp, we get:...(2)
Dividing (2) and (1), we get:
The dynamic resistance is given by:
Q.11. The plate current in a diode is 20 mA when the plate voltage is 50 V or 60 V. What will be the current if the plate voltage is 70 V?
For 50 V or 60 V, the plate current is 20 mA. That means 20 mA is the saturation current.
At the given temperature, the plate current is 20 mA for all other values of voltages.
Thus, the current at 70 V will be 20 mA.
Q.12. The power delivered in the plate circular of a diode is 1.0 W when the plate voltage is 36 V. Find the power delivered if the plate voltage is increased to 49 V. Assume Langmuir-Child equation to hold.
Given:
When plate voltage, Vp, is 36 V, power delivered in the plate circular of a diode, P, is 1.0 W.
Let the plate current be Ip.
Let the power delivered be P' and plate current be Ip' when plate voltage, Vp, is increased to 49 V
According to Langmuir-Child equation,
Thus, power delivered when the plate voltage is increased to 49 V,
Q.13. A triode value operates at Vp = 225 V and Vg = −0.5 V.
The plate current remains unchanged if the plate voltage is increased to 250 V and the grid voltage is decreased to −2.5 V. Calculate the amplification factor.
Amplification factor for a triode valve,
At constant plate current,
This implies that the amplification factor of the triode is 12.5.
Q.14. Calculate the amplification factor of a triode valve that has plate resistance of 2 kΩ and transconductance of 2 millimho.
Given:
Plate resistance,
Transconductance of plate,
Therefore, the amplification factor is given as:
That is, amplification factor is 4.
Q.15. The dynamic plate resistance of a triode value is 10 kΩ. Find the change in the plate current if the plate voltage is changed from 200 V to 220 V.
Given:
Plate resistance,
Change in plate voltage,
Plate resistance at constant grid voltage is given as:
Q.16. Find the values of rp, µ and gm of a triode operating at plate voltage 200 V and grid voltage −6. The plate characteristics are shown in the figure.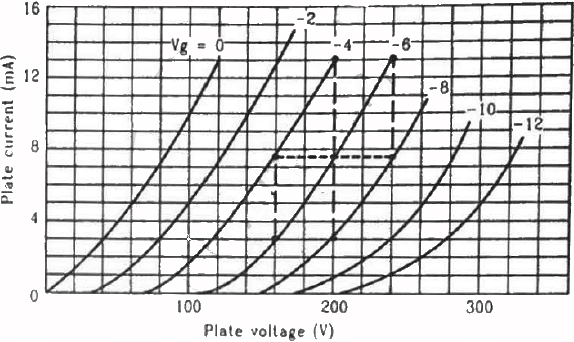
Dynamic plate resistance,
at constant grid voltage
We need to find the slope of the graph for a particular value of grid voltage, i.e. Vg = −6 V.
Consider two points for the plot of Vg = −6 V:
Consider two points on the 200 V line:
Amplification factor,
Q.17. The plate resistance of a triode is 8 kΩ and the transconductance is 2.5 millimho. (a) If the plate voltage is increased by 48 V and the grid voltage is kept constant, what will be the increase in the plate current? (b) With plate voltage kept constant at this increased value, by how much should the grid voltage be decreased in order to bring the plate current back to its initial value?
Given:
Plate resistance,
Change in plate voltage,
Formula for plate resistance:
(b) Now, Vp is kept constant.
Change in plate current,
Trasconductance,at constant plate voltage.
Q.18. The plate resistance and amplification factor of a triode are 10kΩ and 20. The tube is operated at plate voltage 250 V and grid voltage −7.5 V. The plate current is 10 mA. (a) To what value should the grid voltage be changed so as to increase the plate current to 15 mA? (b) To what value should the plate voltage be changed to take the plate current back to 10 mA?
Given:
Plate resistance,
Amplification factor,
μ = 20
Plate voltage,
VP = 240 V
Grid Voltage,
(a) Transconductance,
Q.19. The plate current, plate voltage and grid voltage of a 6F6 triode tube are related as ip = 41 (Vp + 7 Vg)1.41
Here, Vp and Vg are in volts and ip in microamperes.
The tube is operated at Vp = 250 V, Vg = −20 V. Calculate (a) the tube current, (b) the plate resistance, (c) the mutual conductance and (d) the amplification factor.
Given:
Plate voltage, VP = 250 V
Grid voltage, VG = -20V
(a) As given in the question, plate current varies as,
Differentiating this equation, we get:...(1)
Plate resistance is defined as:
From equation (1),
(c) From above:
From equation (1),
(d) Amplification factor,
Q.20. The plate current in a triode can be written as 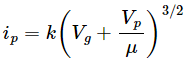 Show that the mutual conductance is proportional to the cube root of the plate current.
Show that the mutual conductance is proportional to the cube root of the plate current.
Given:- The plate current varies with plate and grid voltage as
...(1)
Differentiating the equation w.r.t VG, we get:
From (1), plate current can be written in terms of transconductance as:
Here, K' is a constant =
Q.21. A triode has mutual conductance of 2.0 millimho and plate resistance of 20 kΩ. It is desired to amplify a signal by a factor of 30. What load resistance should be added in the circuit?
Plate resistance, rp = 20 kΩ
Mutual Conductance, gm = 2.0 milli mho
gm = 2 × 10−3 mho
Amplification factor, µ = 30
Load Resistance = RL = ?
We know:
where A is the voltage amplification factor.
Also, amplification factor,
On substitution of all the given values, we get:
Q.22. The gain factor of an amplifier in increased from 10 to 12 as the load resistance is changed from 4 kΩ to 8 kΩ. Calculate (a) the amplification factor and (b) the plate resistance.
We know:
Voltage gain =...(1)
When voltage amplification factor, A = 10,
RL = 4 kΩ...(2)
Now, increased gain, A = 12
Substituting this value in (1) ,we get:...(3)
On solving equations (2) and (3), we get:
Q.23. The figure shows two identical triode tubes connected in parallel. The anodes are connected together and the cathodes are connected together. Show that the equivalent plate resistance is half the individual plate resistance, the equivalent mutual conductance is double the individual mutual conductance and the equivalent amplification factor is the same as the individual amplification factor.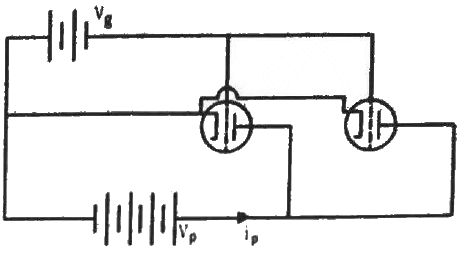
From the circuit,
Cathode of both the triodes are connected to a common point and their anodes are also connected together. Thus, the 2 triodes are connected in parallel.
As plate resistance is obtained by observing the variation of plate voltage with plate current, keeping grid voltage as constant.
Hence equivalent plate resistance
if both the triodes have equal plate resistance
To find the equivalent transconductance
We need to look at the variation of current with respect to grid voltage, keeping plate voltage as constant.
From the circuit
Now the 2 triodes are in series w.r.t grid voltage.
Hence equivalent transconductance will be equal to
Gm = 2gm
if the 2 triodes have equal trens conductance i.e gm1 = gm2 = gm
Now the equivalent amplification factor is,
|
136 docs
|

|
Explore Courses for JEE exam
|

|

























 ...(1)
...(1) ...(2)
...(2)












 at constant grid voltage
at constant grid voltage







 at constant plate voltage.
at constant plate voltage.





 ...(1)
...(1)




 ...(1)
...(1)






 ...(1)
...(1)
 ...(2)
...(2)
 ...(3)
...(3)



















