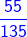Important Formulas: Fractions | Maths Olympiad Class 6 PDF Download

1. Fraction: A fraction represents a part of a whole. The whole can be a group of objects or a single object.

For example:

2. Proper Fraction: A proper fraction is a fraction where the numerator (top number) is less than the denominator (bottom number). It represents a part of a whole.

For example:

3. Improper Fraction: An improper fraction is a fraction where the numerator is greater than or equal to the denominator.

For example:

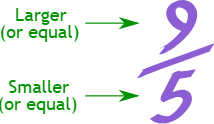
4. Mixed Fraction: A mixed fraction combines a whole number with a proper fraction.

For example:

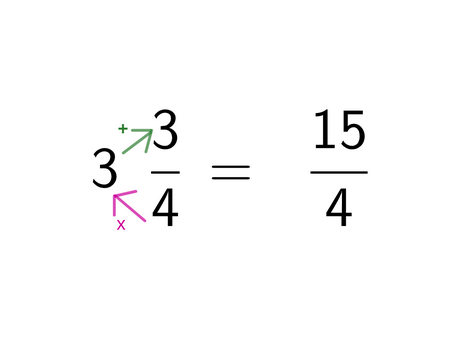
5. Equivalent Fractions: Fractions that represent the same value or part of a whole, even though they have different numerators and denominators. Equivalent fractions can be obtained by multiplying or dividing both the numerator and denominator by the same non-zero integer.

For example:

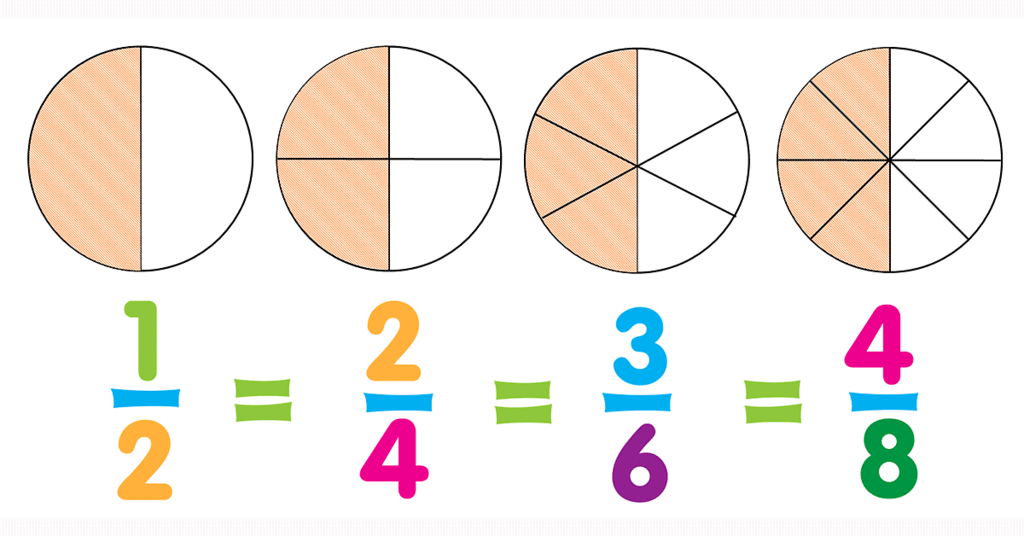
6. Simplest Form: A fraction is in its simplest or lowest form if the numerator and denominator have no common factor other than 1. This can be found by dividing both the numerator and denominator by their highest common factor (HCF).

For example:

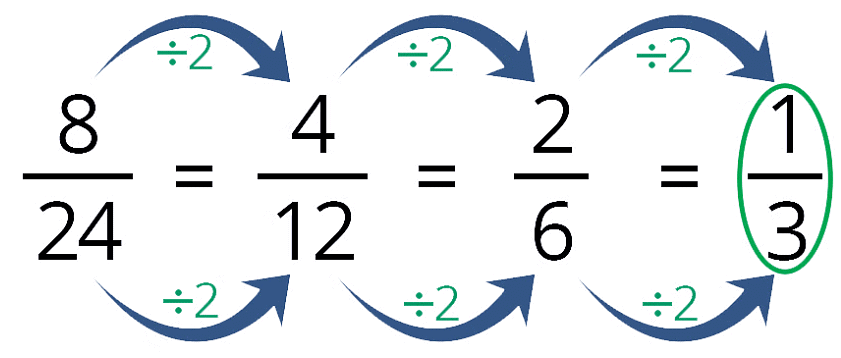
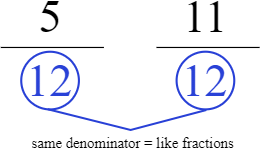
7. Like Fractions: Fractions that have the same denominator.

For example:

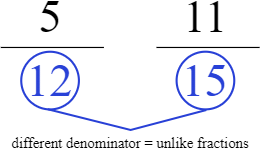
8. Unlike Fractions: Fractions that have different denominators.

For example:

9. Comparing Fractions: To compare fractions, convert them to have a common denominator or convert them to decimal form. 

For like fractions, the one with the greater numerator is larger. 

• For Example: Among fractions 5/7 and 3/7, 5/7 is greater than 3/7 as 5 is greater than 3.

For unlike fractions, find the least common multiple (LCM) of the denominators to compare.

• For Example: Compare: 1/4 and 2/3.
• Step 1: First, observe the denominators of the given fractions, i.e., 1/4 and 2/3. Since the denominators are different make them equal by finding the LCM of 4 and 3. LCM(4,3) = 12.
• Step 2: Now, let us convert the given fraction in such a way that they have the same denominators. So, multiply the first fraction with 3/3, i.e., 1/4 × 3/3 = 4/12.
• Step 3: Similarly, multiply the second fraction with 4/4, i.e., 2/3 × 4/4 = 8/12. Thus, the first fraction becomes 4/12 and the other becomes 8/12.
• Step 4: Compare the obtained new fractions, i.e., 4/12 and 8/12. As the denominators are the same, we will compare the numerators. We can observe that 4 < 8.
• Step 5: The fraction that has a large numerator is the larger fraction. So, 8/12 > 4/12. So, 1/4 > 2/3.

10. Addition and Subtraction of Fractions:

For like fractions, add or subtract the numerators and keep the denominator the same.

Examples:

1)  +

The two fractions are like fractions, so we add their numerators and keep the denominator the same.
 +  =   =

2)   −

Here, the given fractions are like fractions. So, we subtract their numerators and keep the denominator the same.
  −   = =

For unlike fractions, first find the LCM of the denominators, convert to like fractions, then add or subtract the numerators.

Examples:

1)  +

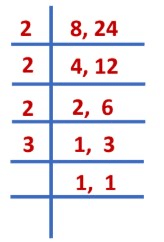
The given fractions are unlike fractions, so we first find LCM of their denominators.

LCM of 8 and 24 = 2 × 2 × 2 × 3 = 24
Now, we convert the fractions into like fractions.
(Changing the denominator of fractions to 24)

 =  and

 

 +  =  =

2)   −

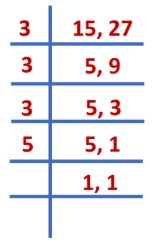
As the given fractions are unlike fractions, we find the LCM of their denominator.

LCM of 15 and 27 = 3 × 3 × 3 × 5 = 135
Next, we convert the fractions into like fractions
(Fractions with the same denominator)

 =  and  =

 

 -  =  =

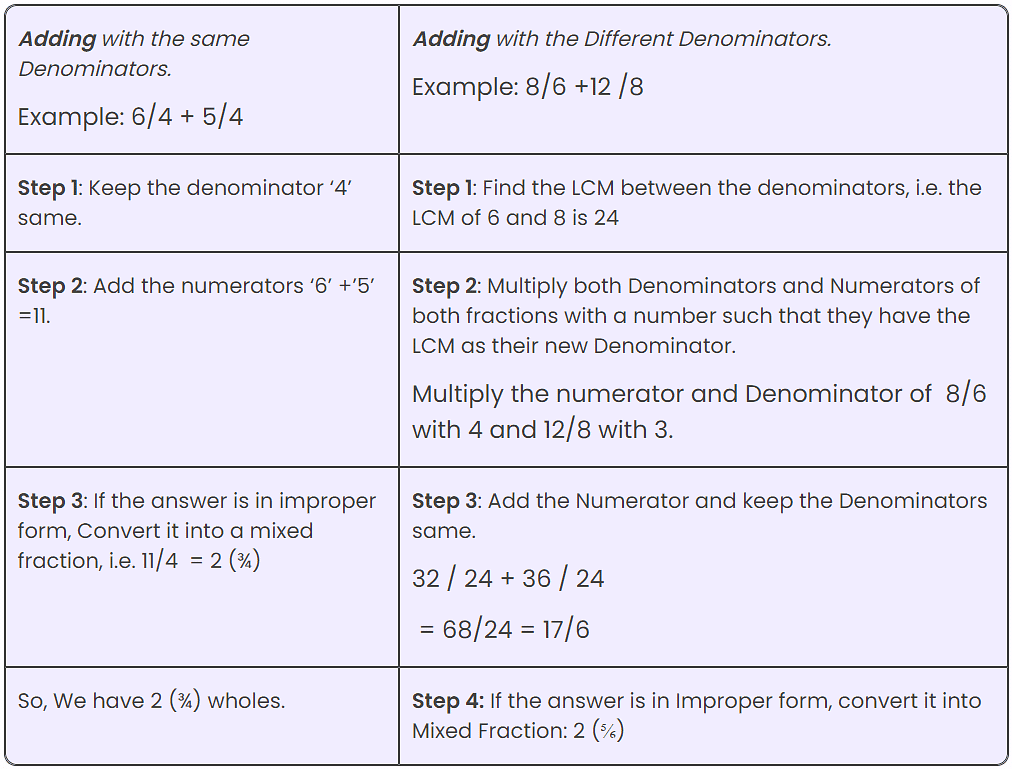
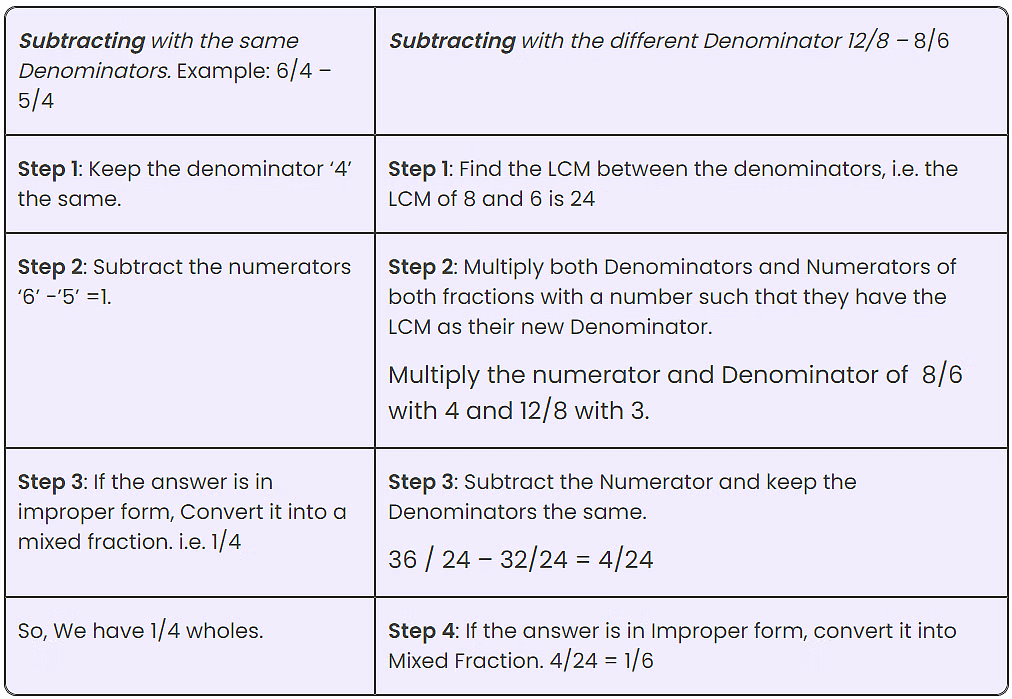
11. Mixed fractions 

• The fraction should be converted to improper fractions before performing operations.
• For Adding Mixed Fractions

• For Subtracting Mixed Fraction