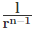Important Formulas: Geometric Progressions | Quantitative Aptitude for SSC CGL PDF Download
| Table of contents |

|
| Geometric Progression |

|
| Formulas for GP |

|
| Properties of Geometric Progression |

|
| Using Formulas of Geometric Progression in Questions |

|
Geometric Progression
A Geometric Progression is a sequence of numbers in which each term, except the first, is determined by multiplying the preceding one by a constant, non-zero number known as the common ratio.

Formulas for GP
- Common ratio:
Formula for finding the common ratio a2/a1 - nth term of an GP:
Formula for finding the nth term of an GP an = a1rn-1 where a1 = First Term,
r = common ratio and
n = number of Terms - Sum of first n terms in an GP:
Standard Formula for sum of first n terms in an GP {if r>1} where, r = common ratio, a1 = First Term, n = number of terms
{if r>1} where, r = common ratio, a1 = First Term, n = number of terms {if r<1} where, r = common ratio, a1 = First Term, n = number of terms
{if r<1} where, r = common ratio, a1 = First Term, n = number of terms - Sum of an infinite GP :
Formula for Sum of an infinite GP (if -1<r<1)
(if -1<r<1) - Geometric Mean (GM):
If two non-zero numbers a and b are in GP, then there GM is GM = (ab)1/2
If three non-zero numbers a,b and c are in GP, then there GM is GM = (abc)1/3
Properties of Geometric Progression
- If ‘a’ is the first term, r is the common ratio of a finite G.P. consisting of m terms, then the nth term from the end will be = a rm-n.
- The nth term from the end of the G.P. with the last term ‘l’ and common ratio r is

- Reciprocal of all the term in G.P are also considered in the form of G.P.
- When all terms is GP raised to same power, the new series of geometric progression is form.
Using Formulas of Geometric Progression in Questions
Q1: What is the 7th term of a geometric progression if the first term (a) is 3 and the common ratio (r) is -2?
(a) -192
(b) 192
(c) -96
(d) 96
Ans: (b)
The nth term of a GP is given by an = a * r(n-1). Substituting the values, a = 3, r = -2, and n = 7:
a7 = 3 * (-2)(7-1) = 3 * (-2)6 = 3 * 64 = 192
Q2: If the sum of an infinite geometric progression is 72 and the common ratio (r) is 0.5, what is the first term (a)?
(a) 2
(b) 12
(c) 24
(d) 36
Ans: (d)
The sum of an infinite GP is given by S = a / (1 – r). Substituting the values, S = 72 and r = 0.5:
72 = a / (1 – 0.5)
72 = a / 0.5
a = 72 * 0.5
a = 36
Q3: Find the common ratio (r) of a geometric progression if the sum of the first 8 terms (S8) is 4374 and the first term (a) is 3.
(a) 3
(b) 2
(c) 4
(d) 5
Ans: (b)
The sum of the first n terms of a GP is given by Sn = a * (rn – 1) / (r – 1). Substituting the values, a = 3, S8 = 4374, and n = 8:
4374 = 3 * (r8 – 1) / (r – 1)
Now, solving for r:
3 * (r8 – 1) = 4374 * (r – 1)
r8 – 1 = 1458 * (r – 1)
r8 – 1458r + 1457 = 0
Using numerical methods, we find that r ≈ 2.362
Q4: What is the sum of the first 5 terms of a geometric progression if the first term (a) is 2 and the common ratio (r) is 3/4?
(a) 6.75
(b) 8.25
(c) 7.50
(d) 5.50
Ans: (c)
The sum of the first n terms of a GP is given by Sn = a * (r^n – 1) / (r – 1). Substituting the values, a = 2, r = 3/4, and n = 5:
S5 = 2 * ((3/4)5 – 1) / (3/4 – 1)
= 2 * (243/1024 – 1) / (-1/4)
= 2 * (-781/1024) / (-1/4) = 7.50
Q5: What is the 10th term of a geometric progression if the 4th term is 54 and the common ratio (r) is 3?
(a) 1458
(b) 2187
(c) 729
(d) None of the above
Ans: (d)
The nth term of a GP is given by an = a * r(n-1).
Substituting the values, a = 54, r = 3, and n = 10:
a10 = 54 * 3(10-1) = 54 * 39 = 54 * 19683 = 1058841
|
314 videos|170 docs|185 tests
|

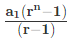 {if r>1} where, r = common ratio, a1 = First Term, n = number of terms
{if r>1} where, r = common ratio, a1 = First Term, n = number of terms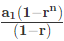 {if r<1} where, r = common ratio, a1 = First Term, n = number of terms
{if r<1} where, r = common ratio, a1 = First Term, n = number of terms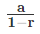 (if -1<r<1)
(if -1<r<1)