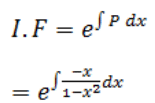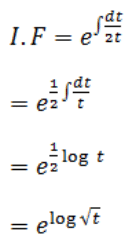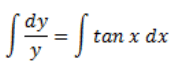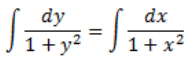Important Questions: Differential Equations | Mathematics (Maths) Class 12 - JEE PDF Download

Q1: Integrating factor of the differential equation (1 – x²)dy/dx – xy = 1 is
Ans: The given differential equation is (1 – x²)dy/dx – xy = 1
(1 – x²)dy/dx = 1 + xy
dy/dx = (1/1 – x²) + (x/1 – x²)y
dy/dx – (x/1 – x²)y = 1/1-x²
This is of the form dy/dx + Py = Q
We can get the integrating factor as below:

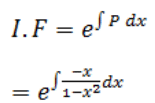
Let 1 – x² = t
Differentiating with respect to x
-2x dx = dt
-x dx = dt/2
Now,

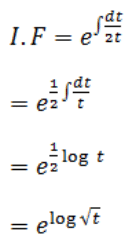
I.F = √t = √(1- x²)

Q2: For each of the given differential equations, find a particular solution satisfying the given condition:
dy/dx = y tan x ; y = 1 when x = 0
Ans: dy/dx = y tan x
dy/y = tan x dx
Integrating on both sides,

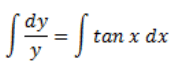
log y = log (sec x) +C
log y = log (C sec x)
⇒ y = C sec x ……..(i)
Now consider y = 1 when x = 0.
1 = C sec 0
1 = C (1)
C = 1
Substituting C = 1 in (i)
y = sec x
Hence, this is the required particular solution of the given differential equation.

Q3: Form the differential equation of the family of circles having a centre on y-axis and radius 3 units.
Ans: The general equation of the family of circles having a centre on the y-axis is x² + (y – b)2 = r²
Given the radius of the circle is 3 units.
The differential; equation of the family of circles having a centre on the y-axis and a radius 3 units is as below:
x² + (y – b)² = 3²
x² + (y – b)² = 9 ……(i)
Differentiating (i) with respect to x,
2x + 2(y – b).y′ = 0
⇒ (y – b). y′ = -x
⇒ (y – b) = -x/y′ …….(ii)
Substituting (ii) in (i),
x² + (-x/y′)² = 9
⇒ x²[1 + 1/(y′)²] = 9
⇒ x² [(y′)² + 1) = 9 (y′)²
⇒ (x² – 9) (y′)² + x² = 0
Hence, this is the required differential equation.

Q4: Form the differential equation representing the family of curves y = a sin (x + b), where a, b are arbitrary constants.
Ans: Given,
y = a sin (x + b) … (1)
Differentiating both sides of equation (1) with respect to x,
dy/dx = a cos (x + b) … (2)
Differentiating again on both sides with respect to x,
d²y/dx² = – a sin (x + b) … (3)
Eliminating a and b from equations (1), (2) and (3),
d²y/ dx² + y = 0 … (4)
The above equation is free from the arbitrary constants a and b.
This the required differential equation.

Q5: Verify that the function y = a cos x + b sin x, where, a, b ∈ R is a solution of the differential equation d²y/dx² + y = 0.
Ans: The given function is y = a cos x + b sin x … (1)
Differentiating both sides of equation (1) with respect to x,
dy/dx = – a sinx + b cos x
d²y/dx² = – a cos x – b sinx
LHS = d²y/dx² + y
= – a cos x – b sinx + a cos x + b sin x
= 0
= RHS
Hence, the given function is a solution to the given differential equation.

Q6:Find the equation of a curve passing through (1, π/4) if the slope of the tangent to the curve at any point P (x, y) is y/x – cos²(y/x).
Ans: According to the given condition,
dy/dx = y/x – cos²(y/x) ………….(i)
This is a homogeneous differential equation.
Substituting y = vx in (i),
v + (x) dv/dx = v – cos²v
⇒ (x)dv/dx = – cos²v
⇒ sec²v dv = – dx/x
By integrating on both the sides,
⇒ ∫sec²v dv = – ∫dx/x
⇒ tan v = – log x + c
⇒ tan (y/x) + log x = c ……….(ii)
Substituting x = 1 and y = π/4,
⇒ tan (π/4) + log 1 = c
⇒ 1 + 0 = c
⇒ c = 1
Substituting c = 1 in (ii),
tan (y/x) + log x = 1

Q7: Find the general solution of the differential equation dy/dx = 1 + y²/1 + x².
Ans: Given differential equation is dy/dx = 1 + y²/1 + x²
Since 1 + y² ≠ 0, therefore by separating the variables, the given differential equation can be written as:
dy/1 + y² = dx/1 + x² …….(i)
Integrating equation (i) on both sides,

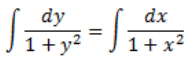
tan^-1y = tan^-1x + C
This is the general solution of the given differential equation.

Q8: Find the differential equation of the family of lines through the origin.
Ans: Let y = mx be the family of lines through the origin.
Therefore, dy/dx = m
Eliminating m, (substituting m = y/x)
y = (dy/dx) . x
or
x. dy/dx – y = 0

Q9: The number of arbitrary constants in the general solution of a differential equation of fourth order is.
Ans: We know that the number of constants in the general solution of a differential equation of order n is equal to its order.
Therefore, the number of constants in the general equation of the fourth-order differential equation is four.
Note: The number of constants in the general solution of a differential equation of order n is equal to zero.

Q10: Determine order and degree (if defined) of differential equation (y′′′)² + (y″)³ + (y′)⁴ + y⁵ = 0
Ans: Given differential equation is (y′′′)² + (y″)³ + (y′)⁴ + y⁵ = 0
The highest-order derivative present in the differential equation is y′′′.
Therefore, its order is 3.
The given differential equation is a polynomial equation in y′′′, y′′, and y′.
The highest power raised to y′′′ is 2.