Important Questions: The Triangles and its properties | Mathematics (Maths) Class 7 (Old NCERT) PDF Download
Q1: Which of the following cannot be the sides of a triangle?
(i) 4.5 cm, 3.5 cm, 6.4 cm
(ii) 2.5 cm, 3.5 cm, 6.0 cm
(iii) 2.5 cm, 4.2 cm, 8 cm
Ans: (i) Given sides are, 4.5 cm, 3.5 cm, 6.4 cm
Sum of any two sides = 4.5 cm + 3.5 cm = 8 cm
Since 8 cm > 6.4 cm (Triangle inequality)
The given sides form a triangle.
(ii) Given sides are 2.5 cm, 3.5 cm, 6.0 cm
Sum of any two sides = 2.5 cm + 3.5 cm = 6.0 cm
Since 6.0 cm = 6.0 cm
The given sides do not form a triangle.
(iii) 2.5 cm, 4.2 cm, 8 cm
Sum of any two sides = 2.5 cm + 4.2 cm = 6.7 cm
Since 6.7 cm < 8 cm
The given sides do not form a triangle.
Q2: One of the equal angles of an isosceles triangle is 50°. Find all the angles of this triangle.
Ans: Let the third angle be x°.
x + 50° + 50° = 180°
⇒ x° + 100° = 180°
⇒ x° = 180° – 100° = 80°
Thus ∠x = 80°
Q3: Two sides of a triangle are 4 cm and 7 cm. What can be the length of its third side to make the triangle possible?
Ans: Let the length of the third side be x cm.
Condition I: Sum of two sides > the third side
i.e. 4 + 7 > x ⇒ 11 > x ⇒ x < 11
Condition II: The difference of two sides less than the third side.
i.e. 7 – 4 < x ⇒ 3 < x ⇒ x > 3
Hence the possible value of x are 3 < x < 11
i.e. x < 3 < 11
Q4: Find whether the following triplets are Pythagorean or not?
(a) (5, 8, 17)
(b) (8, 15, 17)
Ans:
(a) Given triplet: (5, 8, 17)
172 = 289
82 = 64
52 = 25
82 + 52 = 64 + 25 = 89
Since 89 ≠ 289
52 + 82 ≠ 172
Hence (5, 8, 17) is not Pythagorean triplet.
(b) Given triplet: (8, 15, 17)
172 = 289
152 = 225
82 = 64
152 + 82 = 225 + 64 = 289
172 = 152 + 82
Hence (8, 15, 17) is a Pythagorean triplet.
Q5: The sides of a triangle are in the ratio 3 : 4 : 5. State whether the triangle is right-angled or not.
Ans: Let the sides of the given triangle are 3x, 4x and 5x units.
For right angled triangle, we have
Square of the longer side = Sum of the square of the other two sides
(5x)2 = (3x)2 + (4x)2
⇒ 25x2 = 9x2 + 16x2
⇒ 25x2 = 25x2
Hence, the given triangle is a right-angled.
Q6: I have three sides. One of my angle measure 15°. Another has a measure of 60°. What kind of a polygon am I? If I am a triangle, then what kind of triangle am I? [NCERT Exemplar]
Ans: Since I have three sides.
It is a triangle i.e. three-sided polygon.
Two angles are 15° and 60°.
Third angle = 180° – (15° + 60°)
= 180° – 75° (Angle sum property)
= 105°
which is greater than 90°.
Hence, it is an obtuse triangle.
Q7: In ∆ABC, write the following:
(a) Angle opposite to side BC.
(b) The side opposite to ∠ABC.
(c) Vertex opposite to side AC.
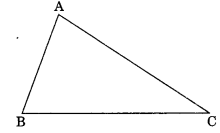
Ans: (a) In ∆ABC, Angle opposite to BC is ∠BAC
(b) Side opposite to ∠ABC is AC
(c) Vertex opposite to side AC is B
Q8: In the given figure, find x.
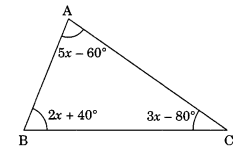
Ans:
In ∆ABC, we have
5x – 60° + 2x + 40° + 3x – 80° = 180° (Angle sum property of a triangle)
⇒ 5x + 2x + 3x – 60° + 40° – 80° = 180°
⇒ 10x – 100° = 180°
⇒ 10x = 180° + 100°
⇒ 10x = 280°
⇒ x = 28°
Thus, x = 28°
Q9: In ΔABC, AC = BC and ∠C = 110°. Find ∠A and ∠B.
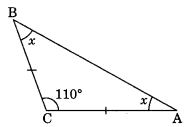
Ans:
In given ΔABC, ∠C = 110°
Let ∠A = ∠B = x° (Angle opposite to equal sides of a triangle are equal)
x + x + 110° = 180°
⇒ 2x + 110° = 180°
⇒ 2x = 180° – 110°
⇒ 2x = 70°
⇒ x = 35°
Thus, ∠A = ∠B = 35°
Q10: In the given right-angled triangle ABC, ∠B = 90°. Find the value of x.
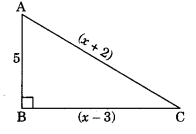 Ans:
Ans:
In ΔABC, ∠B = 90°
AB2 + BC2 = AC2 (By Pythagoras property)
(5)2 + (x – 3)2 = (x + 2)2
⇒ 25 + x2 + 9 – 6x = x2 + 4 + 4x
⇒ -6x – 4x = 4 – 9 – 25
⇒ -10x = -30
⇒ x = 3
Hence, the required value of x = 3
|
77 videos|386 docs|39 tests
|
FAQs on Important Questions: The Triangles and its properties - Mathematics (Maths) Class 7 (Old NCERT)
| 1. What are the different types of triangles and their properties? |  |
| 2. How can I find the area of a triangle? |  |
| 3. What is the Pythagorean theorem and how does it relate to triangles? |  |
| 4. What is the sum of the interior angles of a triangle? |  |
| 5. How can I determine if three given side lengths can form a triangle? |  |
















