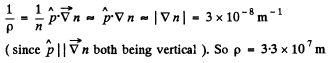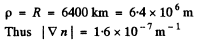Irodov Solutions: Photometry & Geometrical Optics - 3 | I. E. Irodov Solutions for Physics Class 11 & Class 12 - JEE PDF Download
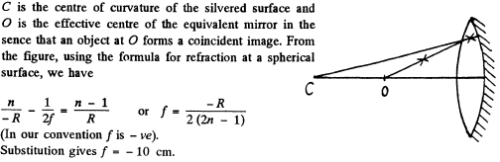
Q.41. Determine the focal length of a concave spherical mirror which is manufactured in the form of a thin symmetric biconvex glass lens one of whose surfaces is silvered. The curvature radius of the lens surface is R = 40 cm.
Ans.
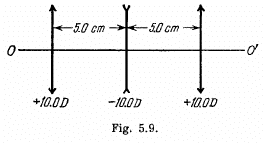
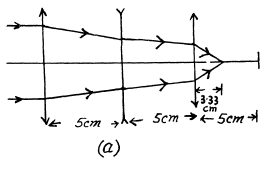
Q.42. Figure 5.9 illustrates an aligned system consisting of three thin lenses. The system is located in air. Determine:
(a) the position of the pointof convergence of a parallel ray incoming from the left after passing through the system;
(b) the distance between the first lens and a point lying on the axis to the leftof the system, at which that point and its image are located symmetrically with respect to the lens system.
Ans. ( a ) Path of a ray, as it passes th rough the lens system is as shown below.
F o c a l le n g th of all the three lenses,
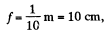 , n e g le c t in g their signs.
, n e g le c t in g their signs.
Applying lens formula for the first lens, considering a ray coming from infinity,
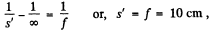
and so the position of the image is 5 cm to the right of the second lens, when only the firstone is present, but the ray again gets refracted while passing through the second, so
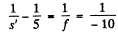
or, s' = 10 cm, which is now 5 cm left to the third lens sofor this lens,
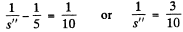
or,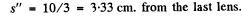
(b) This means that if the object is x cm to be left of the first lens on the axis OO' then the image is x on to the right of the 3rd (la s t ) lens. Call the lenses 1,2,3 from the left and leto be the object, O1 its image by the first lens, O2 the image of O1 by the 2nd lens and O3, the image of O2 by the third lens.
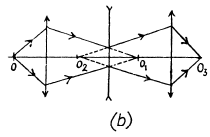
O1 and O2 must be symmetrically located with respect to the lens L2 and since this lens is concave, O1 must be at a distance 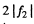 to be the right of L2 and O2 must be
to be the right of L2 and O2 must be 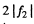 to be the left of L2, One can check that this satisfies lens equation for the third lens Ls
to be the left of L2, One can check that this satisfies lens equation for the third lens Ls
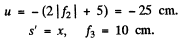
Hence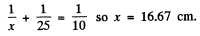
Q.43. A Galilean telescope of 10-fold magnification has the length of 45 cm when adjusted to infinity. Determine:
(a) the focal lengths of the telescope's objective and ocular;
(b) by what distance the ocular should be displaced to adjust the telescope to the distance of 50 m.
Ans. (a) Angular magnification for Galilean telescope in normal adjustment is given as.
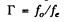
or, 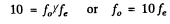 (1)
(1)
The length of the telescope in this case.
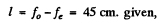
So, using (1), we get,
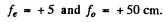
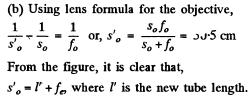
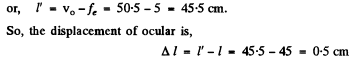
Q.44. Find the magnification of a Keplerian telescope adjusted to infinity if the mounting of the objective has a diameter D and the image of that mounting formed by the telescope's ocular has a diameter d.
Ans. In the Keplerian telescope, in normal adjustment, the distance between the objective and eyepiece is f0 + fe . The image of the mounting produced by the eyepiece is formed at a distance v to the right where
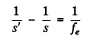
But 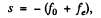
so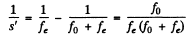
The linear magnification produced by the eyepiece of the mounting is, in magnitude,
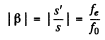
This equals 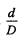 according to the problem so
according to the problem so
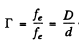
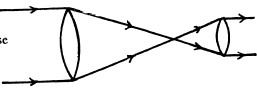
Q.45. On passing through a telescope a flux of light increases its intensity η = 4.0.104 times. Find the angular dimension of a distantobject if its image formed by that telescope has an angular dimension ψ' = 2.0°.
Ans. It is clear from the figure that a parallel beam of light, originally of intensity l0 has, on emerging from the telescope, an intensity.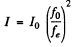
because it is concentrated over a section whose diameter is fe/fo of the diameter of the cross section of the incident beam.
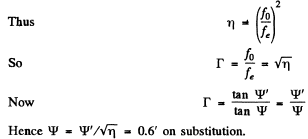
Q.46. A Keplerian telescope with magnification 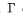 = 15 was submerged into water which filled up the inside of the telescope. To make the system work as a telescope again within the former dimensions, the objective was replaced.What has the magnification of the telescope become equal to? The refractive index of the glass of which the ocular is made is equal to n == 1.50.
= 15 was submerged into water which filled up the inside of the telescope. To make the system work as a telescope again within the former dimensions, the objective was replaced.What has the magnification of the telescope become equal to? The refractive index of the glass of which the ocular is made is equal to n == 1.50.
Ans. W hen a glass lens is immersed in w ater its focal length increases approximately four times. We check this as follows as :
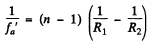
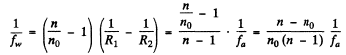
Now back to the problem. Originally in air
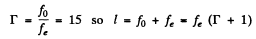
In water, 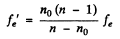
and the focal length of the replaced objective is given by the condition
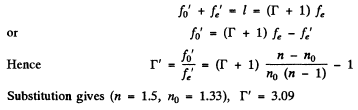
Q.47. At what magnification 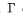 of a telescope with a diameter of the objective D = 6.0 cm is the illuminance of the image of an objecton the retina not less than without the telescope? The pupil diameter is assumed to be equal to d0 = 3.0 mm. The losses of light in the telescope are negligible.
of a telescope with a diameter of the objective D = 6.0 cm is the illuminance of the image of an objecton the retina not less than without the telescope? The pupil diameter is assumed to be equal to d0 = 3.0 mm. The losses of light in the telescope are negligible.
Ans. If L is the luminance of the object, A is its area, s = distance of the object then light falling on the objective is
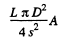
The area of the image formed by the telescope (assuming that the image coincides with the object) is 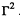 A and the area of the final image on the retina is
A and the area of the final image on the retina is
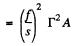
Where f = focal length of the eye lens. Thus the illuminance of the image on the retin (when the object is observed through the telescope) is
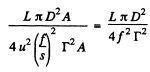
When the object is viewed directly, the illuminance is, similarly, 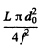
We want 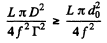
So,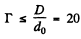 on substitution of the values.
on substitution of the values.
Q.48. The optical powers of the objective and the ocular of a microscope are equal to 100 and 20 D respectively. The microscope magnification is equal to 50. What will the magnification of the microscope be when the distance between the objective and the ocular is increased by 2.0 cm?
Ans. Obviously, 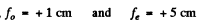
Now, we know that, magnification of a microscope,
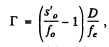 for distinct vision
for distinct vision
or, 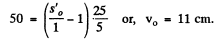
Since distance between objective and ocular has increased by 2 cm, hence it will cause the increase of tube length by 2cm.
so, 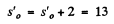
and hence, :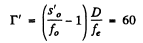
Q.49. A microscope has a numerical aperture sin α = 0.12, where a is the aperture angle subtended by the entrance pupil of the microscope. Assuming the diameter of an eye's pupil to be equal to do = = 4.0 mm, determine the microscope magnification at which
(a) the diameter of the beam of light coming from the microscope is equal to the diameter of the eye's pupil;
(b) the illuminance of the image on the retina is independentof magnification (consider the case when the beam of light passing through the system "microscope-eye" is bounded by the mounting of the objective).
Ans. It is implied in the problem that final image of the object is at infinity (otherwise light coming outof the eyepiece will not have a definite diameter).
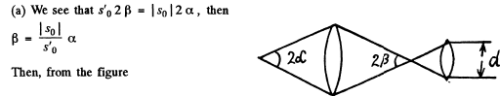
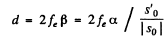
But when the final image is at infinity, the magnification 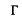 in a microscope is given by
in a microscope is given by
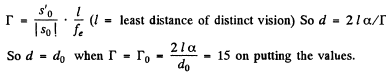
(b) If 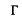 is the magnification produced by the microscope, then the area of the image produced on the retina (when we observe an object through a microscope) is:
is the magnification produced by the microscope, then the area of the image produced on the retina (when we observe an object through a microscope) is:
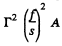
Where u = distance of the image produced by the microscope from the eye lens, f = focal length of the eye lens and A = area of the object If φ = luminous flux reaching the objective from the object and d = d0 so that the entire flux is admitted into the eye), then the illuminance of the final image on the retina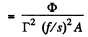
But if 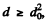 then only a fraction (d0 | d)2 of light is admitted into the eye and the illuminance becomes
then only a fraction (d0 | d)2 of light is admitted into the eye and the illuminance becomes
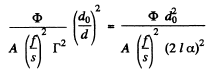
independentof  The condition for this is then
The condition for this is then
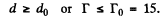
Q.50. Find the positions of the principal planes, the focal and nodal points of a thin biconvex symmetric glass lens with curvature radius of its surfaces equal to R = 7.50 cm. There is air on one side of the lens and water on the other.
Ans. The primary and secondary focal length of a thick lens are given as,
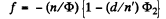
and 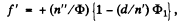
where φ is the lens power n , n' and n" are the refractive indices of first medium, lens material and the second medium beyond the lens.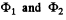 are the powers of first and second spherical surface of the lens.
are the powers of first and second spherical surface of the lens.
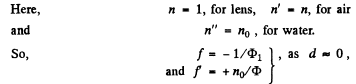 (1)
(1)
Now, power of a thin lens,
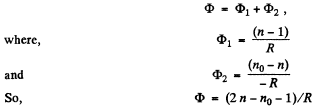 (2)
(2)
From equations (1) and (2), we get
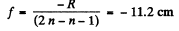
and 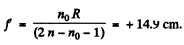
Since the distance between the primary principal point and primary nodal point is given as,
So, in this case, 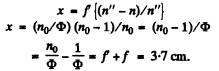
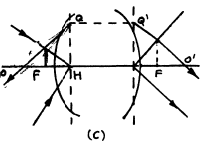
Q.51. By means of plotting find the positions of focal points and principal planes of aligned optical systems illustrated in Fig. 5.10:
(a) a telephoto lens, that is a combination of a converging and a diverging thin lenses (f1 = 1.5 a, f2 = —1.5 a);

(b) a system of two thin converging lenses 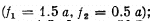
(c) a thick convex-concave lens 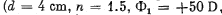
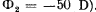
Ans.
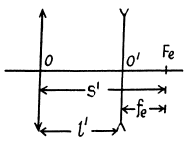
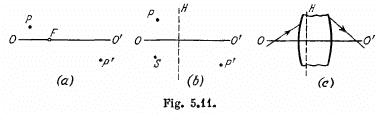
Q.52. An optical system is located in air. Let 00' be its optical axis, F and F' are the front and rear focal points, H and H' are the front and rear principal planes, P and P' are the conjugate points. By means of plotting find:
(a) the positions F' and H' (Fig. 5.11a);
(b) the position of the point S' conjugate to the point S (Fig. 5.11b);
(c) the positions F, F', and H' (Fig. 5.11c, where the path of the ray of light is shown before and after passing through the system).
Ans. (a) Draw P' X parallel to the axis OO' and let PF interest it at X. That determines the principal point H. As the' medium on both sides of the system is the same, the principal point coincides with the nodal point Draw a ray parallel to PH through P' . That determines H' Draw a ray P X' parallel to the axis and join P'X".
That gives F' .
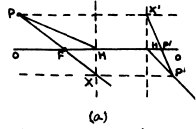
(b) We let H stand for the principal point (on the axis). Determ ine H" by drawing a ray P'H" passing through P' and parallel to PH. One ray (conjugate to SH) can be obtained from this. To get the other ray one needs to know F or F" . This is easy because P and F are known. Finally we get S'.
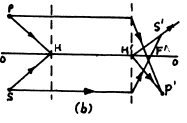
(c) From the incident ray we determine Q. A line parallel to OO' through Q determines Q and hence H'.
H and H ' are then also the nodal points. A ray p arallel to the incident ray through H will emeige parallel to itse lf through H '. That determines F . Sim ilatiy a ray parallel to the emergent ray through H determines F.
Q.53. Suppose F and F' are the front and rear focal points of an optical system, and H and H' are its front and rear principal points. By means of plotting find the position of the image S' of the point S for the following relative positions of the points S, F, F', H, and H':
(a) FSHH'F'; (b) HSF'FH'; (c) H'SF'FH; (d) F'H'SHF.
Ans. Here we do not assume that the media on the two sides of the system are the same.
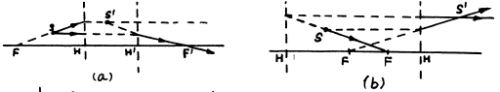
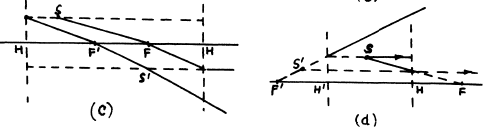
Q.54. A telephoto lens consists of two thin lenses, the front converging lens and the rear diverging lens with optical powers 
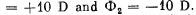 Find:
Find:
(a) the focal length and the positions of principal axes of that system if the lenses are separated by a distance d = 4.0 cm;
(b) the distance d between the lenses at which the ratio of a focal length f of the system to a distance l between the converging lens and the rear principal focal point is the highest. What is this ratio equal to?
Ans. (a) Optical power of the system of combination of two lenses,
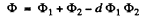
on putting the values,
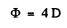
or, 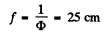
Now, the position of primary principal plane with respect to the vertex of conveiging lens,
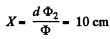
Similarly, the distance of secondary principal plane with respect to the vertex of diverging lens.
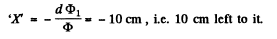
(b) The distance between the rear principal focal point F' and the vertex of conveiging lens,
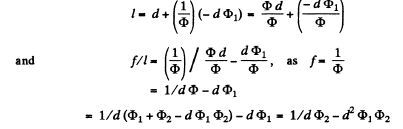
Now , if f/l is maximum for certain value of d then f/l will be minimum for the same value of d. And for minimum f/l,
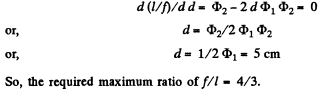
Q.55. Calculate the positions of the principal planes and focal points of a thick convex-concave glass lens if the curvature radius of the convex surface is equal to R1 = 10.0 cm and of the concave surface to R2 = 5.0 cm and the lens thickness is d = 3.0 cm.
Ans. The optical power of first convex surface is,
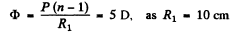
and the optical power of second concave surface is,
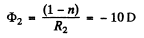
So, the optical power of the system,
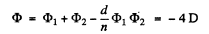
Now , the distance of the primary principal plane from the vertex of convex surface is given as,

and the distance of secondary principal plane from the vertex of second concave surface,
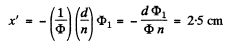
Q.56. An aligned optical system consists of two thin lenses with focal lengths f1 and f2 the distance between the lenses being equal to d. The given system has to be replaced by one thin lens which, at any position of an object, would provide the same transverse magnification as the system. What must the focal length of this lens be equal to and in what position must it be placed with respect to the two-lens system?
Ans. The optical power of the system of two thin lenses placed in air is given as,
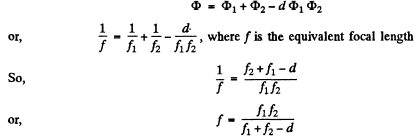 (1)
(1)
This equivalent fo cal length of the'system of two lenses is measured from the primary principal plane.
A s clear from the figure, the distance of the primary principal plane from the optical centre of the first is
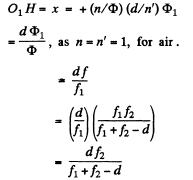
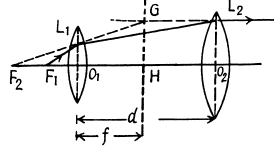
Now, if we place the equivalent lens at the primary principal plane of the lens system, it will provid e the same transverse magnification as the system. So, the distance of equivalent lens from the Vertex of the first lens is,
Q.57. A system consists of a thin symmetrical converging glass lens with the curvature radius of its surfaces R = 38 cm and a plane mirror oriented at right angles to the optical axis of the lens. The distance between the lens and the mirror is l = 12 cm. What is the optical power of this system when the space between the lens and the mirror is filled up with water?
Ans. The plane mirror forms the image of the lens, and water, filled in the space between the two, behind the mirror, as shown in the figure.

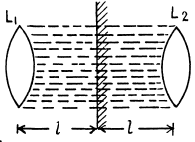
= optical power of individual lens and n0 = R.I. of water.
Now,  optical power of first convex surface + optical power of second concave surface.
optical power of first convex surface + optical power of second concave surface.
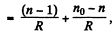 n is the refractive index of glass.
n is the refractive index of glass.
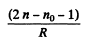 (1)
(1)
and so, the optical power of whole system,
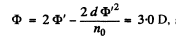 substituting the values.
substituting the values.
Q.58. At what thickness will a thick convex-concave glass lens in air
(a) serve as a telescope provided the curvature radius of its convex surface is ΔR = 1.5 cm greater than thatof its concave surface?
(b) have the optical power equal to —1.0 D if the curvature radii of its convex and concave surfaces are equal to 10.0 and 7.5 cm respectively?
Ans. (a) A telescope in normal adjustment is a zero power conbination of lenses. Thus we require

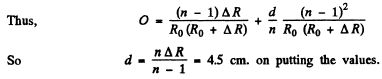
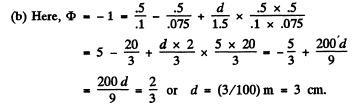
Q.59. Find the positions of the principal planes, the focal length and the sign of the optical power of a thick convex-concave glass lens
(a) whose thickness is equal to d and curvature radii of the surfaces are the same and equal to R;
(b) whose refractive surfaces are concentric and have the curvature radii R1 and R2 (R2> R1).
Ans. (a) The power of the lens is (as in the previous problem)

The principal planes are located on the side of the convex surface at a distance d from each other, with the front principal plane being removed from the con vex surface of the lens by a distance R /(n - 1) .
(b)
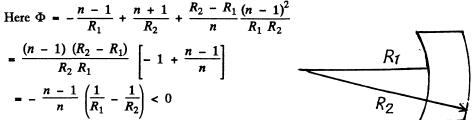
Q.60. A telescope system consists of two glass balls with radii R1 = 5.0 cm and R2 = 1.0 cm. What are the distance between the centres of the balls and the magnification of the system if the bigger ball serves as an objective?
Ans. Let the optical powers of the first and second surfaces of the ball of radius R1 be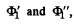 then
then
This ball may be treated as a thick spherical lens of thickness 2R1. So the optical power of this sphere is,
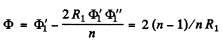 (1)
(1)
Similarly, the optical power of second ball,
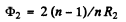
If the distance between the centres of these balls bed. Then the optical power of whole system,
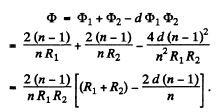
Now, since this system serves as telescope, the optical power of the system must be equal to zero.
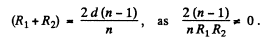
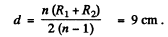
Since the diam eter D of the ob jective is 2R1 and thatof the eye-piece is d = 2R2 So, the magnification,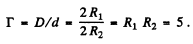
Q.61. Two identical thick symmetrical biconvex lenses are put close together. The thickness of each lens equals the curvature radius of its surfaces, i.e. d = R = 3.0 cm. Find the optical power of this system in air.
Ans. Optical powers of the two surfaces of the lens are
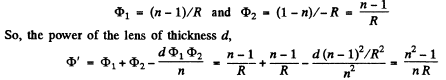
and optical power of the combination of these two thick lenses,
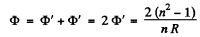
So, power of this system in air is, 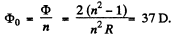
Q.62. A ray of light propagating in an isotropic medium with refractive index n varying gradually from point to point has a curvature radius ρ determined by the formula
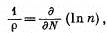
where the derivative is taken with respect to the principal normal to the ray. Derive this formula, assuming that in such a medium the law of refraction n sin θ = const holds. Here θ is the angle between the ray and the direction of the vector 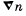 at a given point.
at a given point.
Ans.

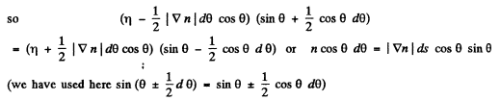

Q.63. Find the curvature radius of a ray of light propagating in a horizontal direction close to the Earth's surface where the gradientof the refractive index in air is equal to approximately 3.10-8 m-1. At what value of that gradient would the ray of light propagate all the way round the Earth?
Ans.