JEE Advanced (Fill in the Blanks): Properties of Triangle | Chapter-wise Tests for JEE Main & Advanced PDF Download
Fill in the Blanks
Q. 1. In a ΔABC, ÐA = 90° and AD is an altitude. Complete the relation
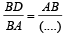
Ans. BC
Solution. We know that altitude from right vertex to hypotenuse in right angled triangle divides it into two triangles each being similar to the original triangle.
ΔBDA - ΔBAC
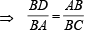
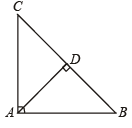
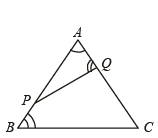
Q. 2. ABC is a triangle, P is a point on AB, and Q is point on AC such that ∠AQP = ∠ABC. Complete the relation
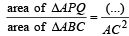
Ans. AP2
Solution. In ΔAPQ and ΔACB
∠A = ∠A (common)
∠AQP = ∠ABC (given)
∴ ΔAPQ - ΔACB fig is with (iii) (by AA similarity)
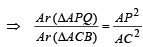
Q. 3. ABC is a triangle with ∠B greater than ∠C. D and E are points on BC such that AD is perpendicular to BC and AE is the bisector of angle A . Complete the relation
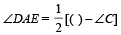
Ans. ∠B
Solution. We have ∠BAE = ∠CAE (given) and
∠ADB = ∠ADC = 90° (given)
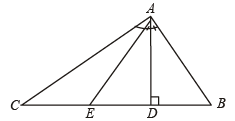
Now ∠DAE = ∠BAE – ∠BAD
= ∠CAE – (90° – ÐB)
= (∠CAD – ∠DAE) – 90° + ∠B
= (90° – ∠C) – ∠DAE – 90° + ∠B
⇒ 2 ∠DAE = ∠B – ∠C 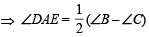
Q. 4. The set of all real number s a such that a2 + 2a, 2a + 3 and a2 + 3a + 8 are the sides of a triangle is ..................
Ans. (5, ∞)
Solution. If a2 + 2a, 2a + 3, a2 + 3a + 8 are sides of a Δ then sum of any two sides is greater than the third side.
Let x = a2 + 2a ; y = 2a + 3 ; z = a2 + 3a + 8
Then x + y > z ⇒ a2 + 4a + 3 > a2 + 3a + 8
⇒ a > 5 .... (1)
y + z > x
⇒ a2 + 5a + 11 > a2 + 2a ⇒ 3a > – 11
⇒ a > – 11/3 .... (2)
z + x > y
⇒ 2a2 + 5a + 8 > 2a + 3 ⇒ 2a2 + 3a + 5 > 0
Here coeff. of a2 > 0 and D = 9 – 40 = –ve
∴ it is true for all values of a. Therefore, identity.
Combining (1) and (2), we get a > 5
∴ a ∈ (5,∞)
Q. 5. In a triangle ABC, if cot A, cot B, cot C ar e in A.P., then a2, b2, c2, are in .................. progression.
Ans. arithmetic
Solution. cot A, cot B, cot C are in A.P.
⇒ cot B – cot A = cot C – cot B
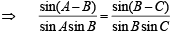
⇒ sin (A – B) sin (A + B) = sin (B + C) sin (B – C)
⇒ sin2A – sin2 B = sin2 B – sin2 C
⇒ a2 – b2 = b2 – c2 ⇒ a2, b2, c2 are in A.P..
Q. 6. A polygon of nine sides, each of length 2, is inscribed in a circle. The radius of the circle is ..................
Ans. 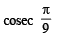
Solution. Let AB = 2 units be one of the sides of the polygon.
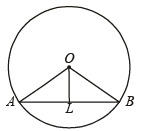
Then ∠AOB = 2p/9 where O is the centre of circle.
If OL ⊥ AB, then AL = 1 and ∠AOL = π/9
∴ Radius of the circle
= OA = AL cosec π/9 = cosec π/9.
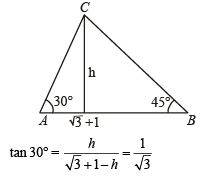
Q. 7. If the angles of a triangle are 30° and 45° and the included side is (√3 + 1) cms, then the area of the triangle is ..................
Ans. 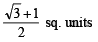
Solution.
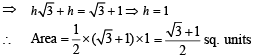
Q. 8. If in a triangle ABC, 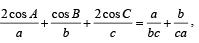 then the value of the angle A is .................. degrees.
then the value of the angle A is .................. degrees.
Ans. 90°
Solution. In ΔABC, we have
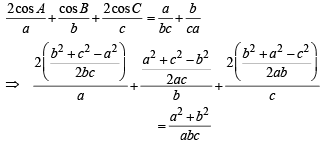
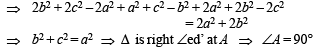
Q. 9. In a triangle ABC, AD is the altitude from A. Given b > c, ∠C = 23º and 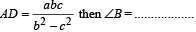
Ans. 113°
Solution.
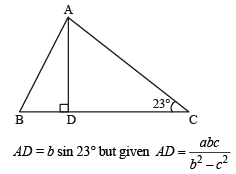
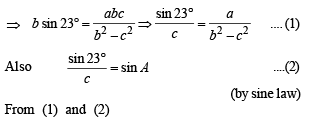
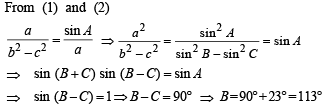
Q. 10. A circle is inscribed in an equilateral triangle of side a. The area of any square inscribed in this circle is ..................
Ans. 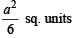
Solution. Given that ABC is an equilateral Δ, of side a, r is the radius of circle inscribed in it

If PQRS is the square inscribed in circle of radius r, then
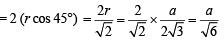
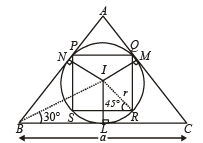
∴ area of square = a2/6 sq. units.
Q. 11. In a triangle ABC, a : b : c = 4 : 5 : 6. The ratio of the radius of the circumcircle to that of the incircle is ..................
Ans. 16 : 7
Solution.
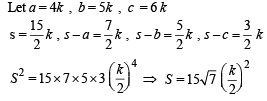
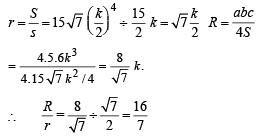
|
446 docs|929 tests
|
















