JEE Advanced (Fill in the Blanks): Straight Lines & Pair of Straight Lines | Chapter-wise Tests for JEE Main & Advanced PDF Download
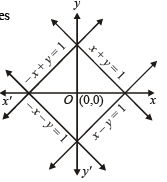
Q.1. The area enclosed within the curve | x | + | y | = 1 is ................... (1981 - 2 Marks)
Ans. 2 sq. units.
Sol. | x | + | y | = 1
The curve represents four lines
x + y = 1, x – y = 1, – x + y = 1, – x – y = 1
which enclose a square of side = distance between opp. sides x + y = 1 and x + y = – 1
Side 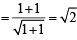
∴ Req. area = (side)2 = 2 sq. units.
Q.2. y = 10x is the reflection of y = log10 x in the line whose equation is ................ (1982 - 2 Marks)
Ans. y = x
Sol. As y = log10 x can be obtained by replacing x by y and y by x in y = 10x
∴ The line of reflection is y = x.
Q.3. The set of lines ax+by+c = 0, where 3a + 2b + 4c = 0 is concurrent at the point ................... (1982 - 2 Marks)
Ans. (3/4, 1/2)
Sol. Given that 3a + 2b + 4c = 0 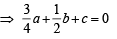
⇒ The set of lines ax + by + c = 0 passes through the point (3/4, 1/2).
Q.4. Given the points A (0, 4) and B (0, –4), the equation of the locus of the point P(x, y) such that | AP – BP | = 6 is ................... (1983 - 1 Mark)
Ans.
Sol. AP – BP | = 6
We know that locus of a point, difference of whose distances from two fixed points is constant, is hyperbola with the fixed points as focii and the difference of distances as length of transverse axis.
Thus, ae = 4 and 2a = 6 ⇒ a = 3, e = 4 /3
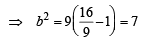 ∴ Equation is
∴ Equation is 
(foci being on y-axis, it is vertical hyperbola)
Q.5. If a, b and c are in A.P., then the straight line ax + by + c = 0 will always pass through a fixed point whose coordinates are ................... (1984 - 2 Marks)
Ans. (1, – 2)
Sol. If a, b, c are in A.P. then a + c = 2b ⇒ a – 2b + c = 0
⇒ ax + by + c = 0 passes through (1,– 2).
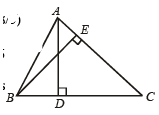
Q.6. The orthocentre of the triangle for med by the lines x + y = 1, 2x + 3y = 6 and 4x – y + 4 = 0 lies in quadrant number ................... . (1985 - 2 Marks)
Ans. first quadrant
Sol.
The equations of sides of triangle ABC are
AB : x + y = 1
BC : 2x + 3y = 6
CA : 4x – y = – 4
Solving these pairwise we get the vertices of D as follows A (– 3/5, 8/5) B (– 3, 4) C (– 3/7, 16/7)
Now AD is line ⊥ lar to BC and passes through A. Any line perpendicular to BC is 3x – 2y + l = 0 As it passes through A(– 3/5, 8/5)
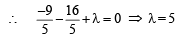
∴ Equation of altitude AD is 3x – 2y + 5 = 0 ...(1)
Any line perpendicular to side AC is x + 4y + µ = 0
As it passes through point B (– 3, 4)
∴ – 3 + 16 + µ = 0 ⇒ µ = – 13
∴ Equation of altitude BE is x + 4y –13 = 0 ...(2)
Now orthocentre is the point of intersection of equations (1) and (2) (AD and BE)
Solving (1) and (2), we get x = 3/7, y = 22/7
As both the co-ordinates are positive, orthocentre lies in first quadrant.
Q.7. Let the algebraic sum of the perpendicular distances from the points (2, 0), (0, 2) and (1, 1) to a variable straight line be zero; then the line passes through a fixed point whose cordinates are ................... . (1991 - 2 Marks)
Ans. (1, 1)
Sol. Let the variable line be ax + by + c = 0 .....… (1) 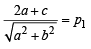
Then ⊥lar distance of line from (0, 2) = 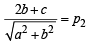
⊥lar distance of line from (1, 1) = 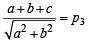
ATQ p1 + p2 + p3 = 0
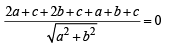
⇒ 3a + 3b + 3c = 0
⇒ a + b + c = 0 .....… (2)
From (1) and (2), we can say variable line (1) passes through the fixed point (1, 1).
Q.8. The vertices of a triangle are A (–1, –7), B (5, 1) and C (1, 4).
The equation of th e bisector of the an gle ∠ABC is ................... . (1993 - 2 Marks)
Ans. x - 7y + 2 = 0
Sol. Let BD be the bisector of ∠ABC.
NOTE THIS STEP :
Then AD : DC = AB : BC
And
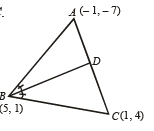
AB = 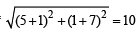
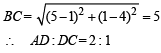
∴ By section formula 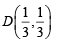
Therefore equation of BD is
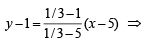
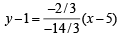
⇒ 7y – 7 = x – 5 ⇒ x – 7y + 2 = 0
|
446 docs|929 tests
|
















