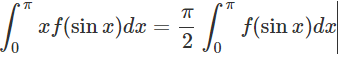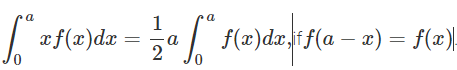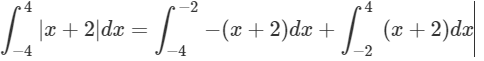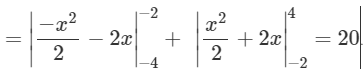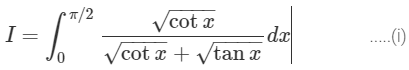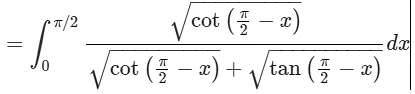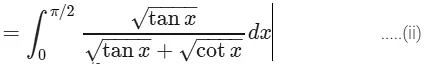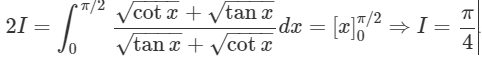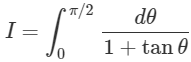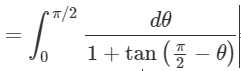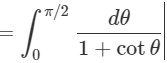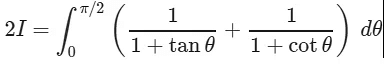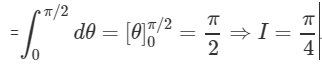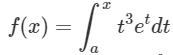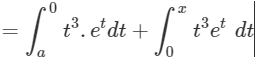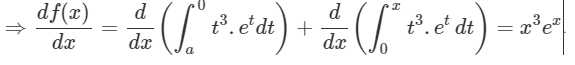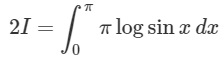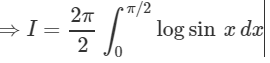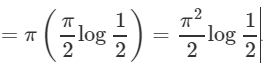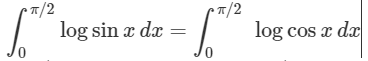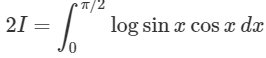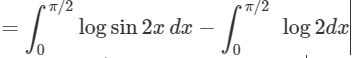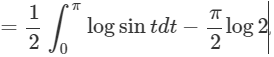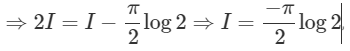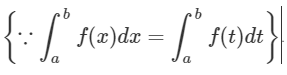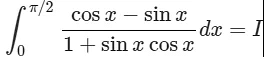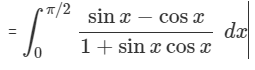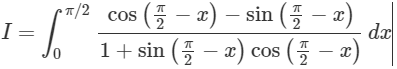JEE Advanced (Single Correct Type): Definite Integrals & Application of Integrals | Chapter-wise Tests for JEE Main & Advanced PDF Download
Q.1. 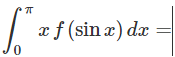
(a) 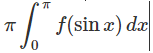
(b) 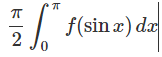
(c) 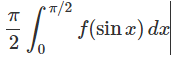
(d) None of these
Correct Answer is option (b)
Since
Q.2. The area bounded by the curves y2 = 4x and y = x is equal to
(a) 1/3
(b) 8/3
(c) 35/6
(d) None of these
Correct Answer is option (b)
For the given curves, y2 = 4x and y = x, the intersection points are (0, 0) and (4, 4).
Therefore, the area bounded by the curves,
A =0∫4 [√(4x)-x] dx [since y2 = 4x, y = √(4x) ]
A = 2. 0∫4√x dx – 0∫4 x dx
Integrate the function and apply the limits, we get
A = (4/3)(8) – 8
A = (32-24)/3 = 8/3.
Hence, option (b) is the correct answer.
Q.3. 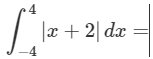
(a) 50
(b) 24
(c) 20
(d) None of these
Correct Answer is option (c)
Q.4. The area of the figure bounded by the curve y = logex, the x-axis and the straight line x = e is
(a) 5 - e
(b) 3 + e
(c) 1
(d) None of these
Correct Answer is option (c)
At, x= 1, y = loge (1) = 0
At, x = e, y = loge (e) = 1
Therefore, A = 1∫e logex dx
Using integration by parts,
A = [x loge x – x]1e
Now, apply the limits, we get
A = [e-e-0+1]
A = 1
Q.5. 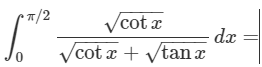
(a) π|
(b) π/2|
(c) π/4|
(d) π/3|
Correct Answer is option (c)
Now adding (i) and (ii), we get
Q.6. The area of the region bounded by the curve x² = 4y and the straight line x = 4y – 2 is
(a) ⅜ sq. units
(b) ⅝ sq. units
(c) ⅞ sq. units
(d) 9/8 sq. units
Correct Answer is option (d)
For the curves x2 = y and x = 4y-2, the points of intersection are x = -1 and x = 2.
Hence, the required area, A = -1∫2 {[(x+2)/4]- [x2/4] } dx
Now, integrate the function and apply the limits, we get
A = (¼)[(10/3)-(-7/6)]
A = (¼)(9/2) = 9/8 sq. units
Hence, the correct answer is option (d) 9/8 sq. units
Q.7. 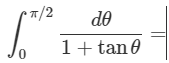
(a) π|
(b) π/2|
(c) π/3|
(d) π/4|
Correct Answer is option (d)
On adding,
Q.8. The area enclosed between the graph of y = x3 and the lines x = 0, y = 1, y = 8 is
(a) 7
(b) 14
(c) 45/6
(d) None of these
Correct Answer is option (c)
Given curve, y=x3 or x = y1/3.
Hence, the required area, A = 1∫8 y1/3 dy
A = [(y4/3)/(4/3)]18
Now, apply the limits, we get
A = (¾)(16-1)
A = (¾)(15) = 45/4.
Hence, option (c) 45/4 is the correct answer.
Q.9. if 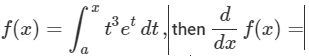
(a) ex(x3+3x2)
(b) x3ex
(c) a3ea
(d) None of these
Correct Answer is option (b)
Q.10. The area of the region bounded by the curve y² = x, the y-axis and between y = 2 and y = 4 is
(a) 52/3 sq. units
(b) 54/3 sq. units
(c) 56/3 sq. units
(d) None of these
Correct Answer is option (c)
Given: y2 = x
Hence, the required area, A = 2∫4 y2 dy
A = [y3/3]24
A = (43/3) – (23/3)
A = (64/3) – (8/3)
A = 56/3 sq. units.
Q.11. 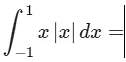
(a) 1
(b) 0
(c) 2
(d) -2
Correct Answer is option (b)
Let f(x)=x|x|. Then f(−x)=−x|−x|=−x|x|=−f(x)
Therefore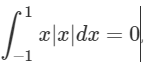
(By the property of definite integral).
Q.12. Area of the region bounded by the curve y = cos x between x = 0 and x = π is
(a) 1 sq. units
(b) 2 sq. units
(c) 3 sq. units
(d) 4 sq. units
Correct Answer is option (b)
Given: y= cos x and also provided that x= 0 and x = π
Hence, the required area, A =0∫π |cos x| dx
It can also be written as,
A = 20∫π/2 cos x dx
Now, integrate the function, we get
A = 2[sin x]0π/2
Now, apply the limits we get
A = 2 sq. units
Hence, the correct answer is option (b) 2 sq. units
Q.13. 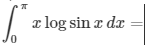
(a) 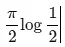
(b) 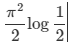
(c) 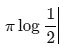
(d) 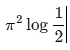
Correct Answer is option (b)
...(i)
....(ii)
By adding (i) and (ii), we get
Q.14. Area of the region bounded by the curve x = 2y + 3, the y-axis and between y = -1 and y = 1 is
(a) 6 sq. units
(b) 4 sq. units
(c) 8 sq. units
(d) 3/2 sq. units
Correct Answer is option (a)
Required Area =-1∫1(2y+3)dyA=[(2y2/2)+3y]-11
Now, apply the limits, we get
A = 1+3-1+3
A = 6 sq. units.
Q.15. 
(a) 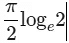
(b) 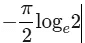
(c) πloge2
(d) 0
Correct Answer is option (d)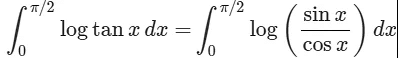
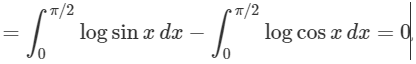
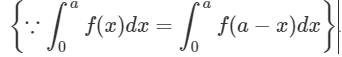
Q.16. The area bounded by the curve y = x3, the x-axis and two ordinates x = 1 and x = 2 is
(a) 15/2 sq. units
(b) 15/4 sq. units
(c) 17/2 sq. units
(d) 17/4 sq. units
Correct Answer is option (b)
Required Area = 1∫2 x3 dx
A = [x4/4]12
Now, apply the limits, we get
A = [(24/4) – (¼)]
A = (16/4) – (¼)
A = 15/4
Hence, the required area is 15/4 is the correct answer.
Q.17. 
(a) 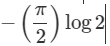
(b) 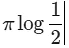
(c) 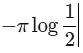
(d) 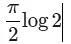
Correct Answer is option (a)
(Putting 2x=t)
Q.18. The area of the region bounded by the circle x² + y² = 1 is
(a) 2π sq. units
(b) 3π sq. units
(c) 4π sq. units
(d) 1π sq. units
Correct Answer is option (d)
Given:
Given circle equation is x2+y2 =1, whose centre is (0, 0) and radius is 1.
Therefore, y2 = 1-x2
y=√(1-x2)
Hence, the required area, A = 40∫1√(1-x2) dxNow, integrate the function and apply limits, we get
A = 4(½)(π/2)
A = π sq. units
Hence, option (d) 1π sq. unit is the correct answer.
Q.19. 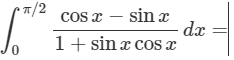
(a) 2
(b) -2
(c) 0
(d) None of these
Correct Answer is option (c)
Now,
On adding, 2I = 0 ⇒ I = 0.
Q.20. Area bounded by the curve y = sin x and the x-axis between x = 0 and x = 2π is
(a) 2 sq. units
(b) 3 sq. units
(c) 4 sq. units
(d) None of these
Correct Answer is option (c)
Required Area, A = 0∫2π |sin x| dx
A = 0∫π sin x dx + π∫2π(-sin x) dxNow, substitute the limits, we get
A= 4 sq. units.
|
481 docs|964 tests
|