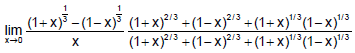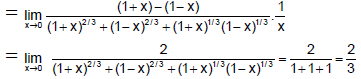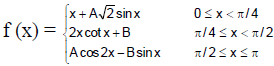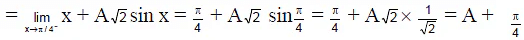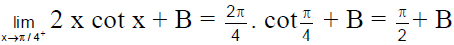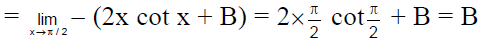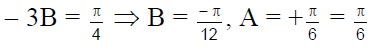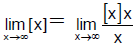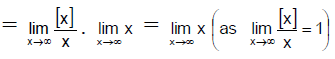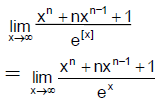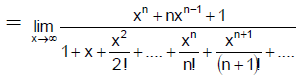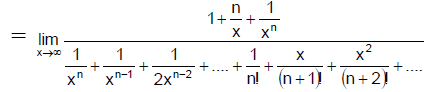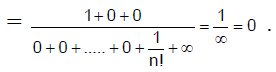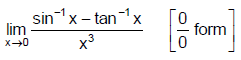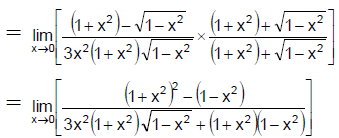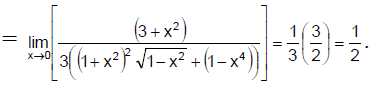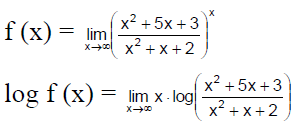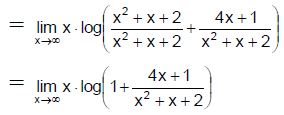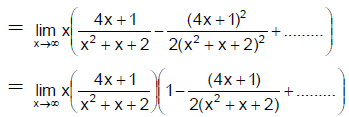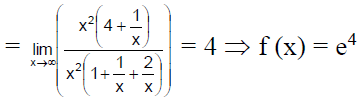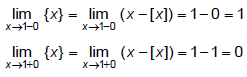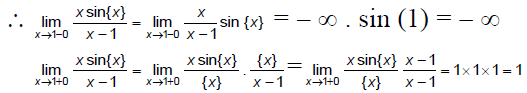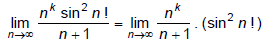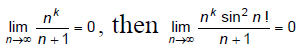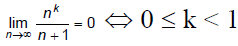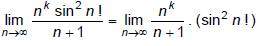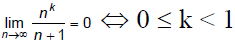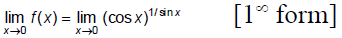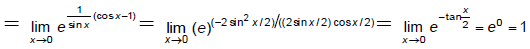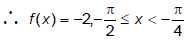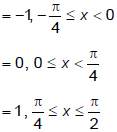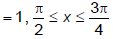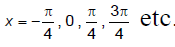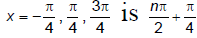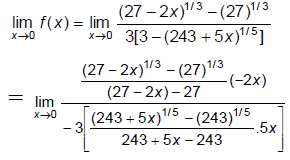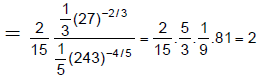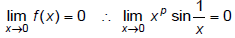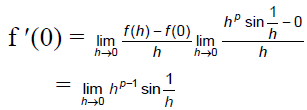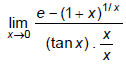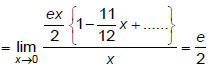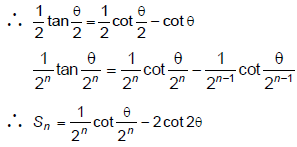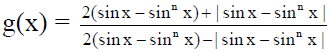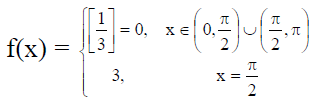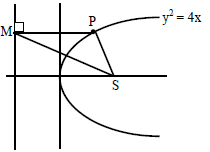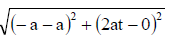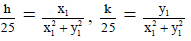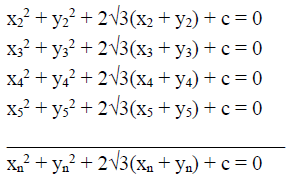JEE Advanced (Single Correct Type): Limits, Continuity & Differentiability | Chapter-wise Tests for JEE Main & Advanced PDF Download
Q.1. The value of 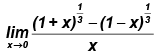 is:
is:
(a) 2/3
(b) 1/3
(c) 1
(d) 5/3
Correct Answer is option (a)
Hence, (a) is the correct answer.
Q.2. The values of A and B so that function f (x) defined by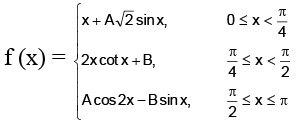
become continuous, respectively are
(a) -π/12, π/6
(b) π/6, -π/12
(c) P/12, π/6
(d) π/6, P/12
Correct Answer is option (b)
L.H. limit at x < (π/4)
R.H. limit =...(1)
L.H. limit at x < (π/2)
RH limit = x+ A cos 2x - B sin x = A cos π - B sin(π/2)
= - A - B
- A - B = B ⇒ a = - 2B … (2)
Hence, (b) is the correct answer.
Q.3. The value of 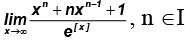 is:
is:
(a) 1
(b) 0
(c) n
(d) n(n –1)
Correct Answer is option (b)
Hence, (b) is the correct answer.
Q.4. 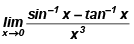 is equal to
is equal to
(a) 2
(b) 1
(c) –1
(d) 1/2
Correct Answer is option (d)
(L’ Hospital rule)
Hence, (d) is the correct answer.
Q.5. If f (x) =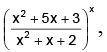 then
then 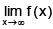 is
is
(a) e4
(b) e3
(c) e2
(d) 24
Correct Answer is option (a)
Hence, (a) is the correct answer.
Q.6. 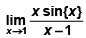 , where {x} denotes the fractional part of x, is
, where {x} denotes the fractional part of x, is
(a)-1
(b) 0
(c)1
(d) does not exist
Correct Answer is option (d)
Since, L.H. limit ≠ R.H. limit
∴ limit does not exist.
Hence, (d) is the correct answer.
Q.7. If 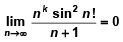 for
for
(a) all k
(b) 0 ≤ k < 1
(c) k = 1
(d) for k > 1
Correct Answer is option (b)
does not exist but if
But
Hence, (b) is the correct answer.
Q.8. If 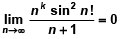 for
for
(a) all k
(b) 0 ≤ k < 1
(c) k = 1
(d) for k > 1
Correct Answer is option (b)
does not exist but if
then
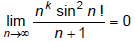
But,
Hence, (b) is the correct answer.
Q.9. If 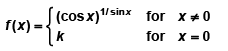
The value of k, so that f is continuous at x = 0 is
(a) 0
(b) 1
(c) 1/2
(d) none of these
Correct Answer is option (b)
Given, f(0) = k
For f(x) to be continuous at x = 0, k = 1.
Hence, (b) is the correct answer.
Q.10. If f(x) = [√2 sin x ] , where [x] denotes the greatest integer function, then
(a) f(x) is continuous at x = 0
(b) maximum value of f(x) is 1 in interval [-2π, 2π]
(c) f(x) is discontinuous at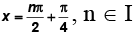
(d) f(x) is differentiable at x = nπ, n ∈ I
Correct Answer is option (b)
f (x) = [√2 sin x]clearly f(x) is discontinuous at
General value corresponding to
maximum value of f(x) in [-2π, 2π] is 1 at x = π/2.
f(x) is discontinuous and non-differentiable at x = 0 therefore choice (d) is not correct.
Hence, (b) is the correct answer.
Q.11. The value of f(0), so that the function f(x) =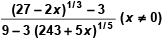 is continuous is
is continuous is
(a) 2/3
(b) 6
(c) 2
(d) 4
Correct Answer is option (c)
Hence, (c) is the correct answer.
Q.12. Let 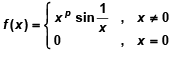
Then f(x) is continuous but not differentiable at x = 0 if
(A) p < 0
(B) p = 0
(C) 0 < p ≤ 1
(D) p ≥ 1
Correct Answer is option (c)
f(0) = 0
For f(x) to be continuous at x = 0
This is possible only when p > 0 …(i)
f'(0) = will exist only when p > 1
∴ f(x) will not be differentiable if p ≤ 1 …(ii)
From (i) and (ii), for f(x) to be not differentiable but continuous at x = 0, possible values of p are given by 0 < p ≤ 1.
Hence, (c) is the correct answer.
Q.13. 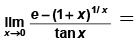
(a) e
(b) e/2
(c) e2
(d) none of these
Correct Answer is option (b)
[using expansion]
Hence, (b) is correct answer.
Q.14. 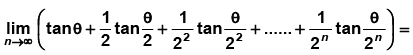
(a) 1/θ
(b) (1/θ) - 2 cot2θ
(c) 2cot 2θ
(d) none of these
Correct Answer is option (b)
tanθ = cotθ - 2 cot 2θ
∴ Required limit =
= (1/θ) - 2cot2θ
Hence, (b) is correct answer.
Q.15. If g(x) = 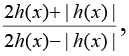 where h(x) = sinx - sinnx, n ∈ R+, the set of positive real numbers, and f(x) =
where h(x) = sinx - sinnx, n ∈ R+, the set of positive real numbers, and f(x) = 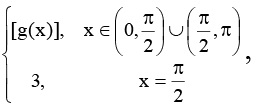 where, [x] denotes the greatest integer function, then
where, [x] denotes the greatest integer function, then
(a) f(x) is continuous and differentiable at x = (π/2), when 0 < n < 1
(b) f(x) is continuous and differentiable at x =(π/2), when n > 1
(c) f(x) is continuous but not differentiable at x =(π/2), when 0 < n < 1
(d) f(x) is continuous but not differentiable at x = π/2, when n > 1
Correct Answer is option (b)
for 0 < n < 1, sinx < sinnx, g(x) = (1/3) & for n > 1, sinx > sinnx, g(x) = 3
∴ for n > 1, f(x) = 3, x ∈ (0, π)
∴ f(x) is continuous and differentiable at x = π/2 & for 0 < n < 1
∴ f(x) is not continuous at x = π/2.
Hence, f(x) is also not differentiable at x = π/2.
Q.16. If f (x) = x + 2 (1 ≤ x ≤ 2) and g(x) = x (2 ≤ x ≤ 3). Then f(g(x)) is
(a) f(g(x)) = x + 2, (1 ≤ x ≤ 2)
(b) f(g(x)) = 4, x = 2
(c) f(g(x)) = x + 2, (2 ≤ x ≤ 3)
(d) f(g(x)) = 2, x = 2
Correct Answer is option (b)
Equation of the required circle may be given as x2 -y2 + 2(cxy - 2x + 1 + λ(y2 - 4(c + 1)x)since coefficient of xy = 0
⇒ c = 0
coefficient of x2 = coefficient of y2
⇒ 1 = -1 + λ ⇒ λ = 2
By putting the values of λ and c we get the equation of the required circle as
x2 + y2 -10x + 1 = 0 ⇒ center of the circle is (5, 0).
Q.17. 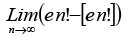 is
is
(a) 0
(b) 1
(c) 1/2
(d) does not exist
Correct Answer is option (a)
Let the coordinate of P is (at2, 2at)
Coordinate of M will be (-a, 2at), the coordinate of S(focus) will be (a, 0)
Since the triangle PMS is equilateral triangle
⇒ PM = MS = PS
⇒ at2 + a =
⇒ t = ±3
⇒ (at2, 2at) ≡ (3, 2√3) or (3, -2√3)
Q.18. 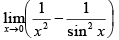 is
is
(a) 1
(b) 1/3
(c) -1/3
(d) does not exist
Correct Answer is option (a)
Centre of C3 will lie on the radical axis of C1 and C2 which is 10x + 6y + 26 = 0.
Let center of C3 is (h, k).
Equation of chord of contact through (h, k) to the C1 may be given as hx + ky = 25 ...(I)
Let the mid point of the chord is (x1, y1) the equation of the chord with the help of mid point may be given as xx1 + yy1 = x12 + y12 ...(II)
Since, (I) and (II) represents same straight lines
⇒
Since, (h, k) lie on the radical axis 10x + 6y + 26 = 0
⇒⇒ the locus of (x1, y1) is 5x + 3y + (13/25)(x2 + y2) = 0
Q.19. 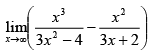
(a) does not exist
(b) 1/3
(c) 0
(d) 2/9
Correct Answer is option (a)
In the given equation of parabola the focus is (1, 1) and equation of directrix is
x + y + 2 = 0
⇒ axis of parabola is y = x.
Vertex of the parabola C is (0, 0). Let a is the distance between vertex and focus = √2 the distance of the point on the axis from which three normals may be drawn will be minimum 2a from the vertex which is equal to 2√2 coordinate the point nearest to the vertex from which three normals may be drawn can be given as (2, 2).
⇒ h ≥ 2.
Q.20. If f (x) = 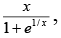 x ≠ 0, f(0) = 0 then
x ≠ 0, f(0) = 0 then
(a) f (x) is continuous at x = 0 and f'(x) = 1
(b) f (x) is discontinuous at x = 0
(c) f(x) is continuous at x = 0 and f'(x) does not exist
(d) f (x) is continuous at x = 0 and f'(x) = 0
Correct Answer is option (b)
Equation of the circle with center (√3, √3) may be given as x2 + y2 + 2√3(x + y) + c = 0
Let (x1, y1), (x2, y2), (x3, y3) … (x4, y4) are the rational points satisfying the equation of circles
⇒ The following relations holds good x12 + y12 + 2√3(x1 +y1) + c = 0
All the above relations may holds only if
x1 + y1 = 0, x2 + y2 = 0…..xn + yn = 0
⇒ If any rational point (xr, yr) lie on the circle it should also satisfy the straight line x + y = 0. Since there may be at the most two points of intersection between a circle and a straight line.
⇒ There are at the most two rational points lying in the circle.
|
446 docs|929 tests
|