JEE Advanced (Subjective Type Questions): Equilibrium- 2 | Chapter-wise Tests for JEE Main & Advanced PDF Download

Q.21. A 40.0 ml solution of weak base, BOH is titrated with 0.1N HCl solution. The pH of the solution is found to be 10.04 and 9.14 after adding 5.0 ml and 20.0 ml of the acid respectively. Find out the dissociation constant of the base.

Ans. 1.828 × 10^–5

Solution. Case I. Write the concerned chemical reaction

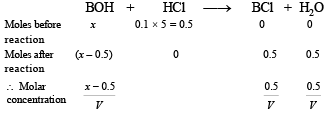
Since the solution represents a basic buffer, following Hendersen equation can be applied.

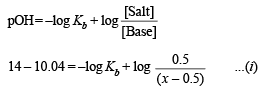
Case II.

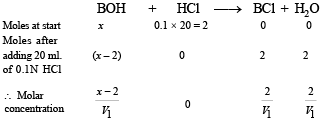
Again the solution is acting as basic buffer

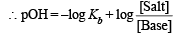
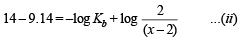
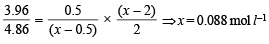
Substituting x in (i) and solving for K_b

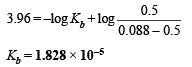
Q.22. The solubility product (K_sp) of Ca(OH)₂ at 25ºC is 4.42 × 10^–5. A 500 ml. of saturated solution of Ca(OH)² is mixed with equal volume of 0.4 M NaOH. How much Ca(OH)₂ in milligrams is precipitated?

Ans. 743.3 mg

Solution. Let the solubility of Ca(OH)2 in pure water = S moles/litre

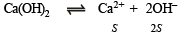
Then  K_sp = [Ca²⁺] [OH^–]²

4.42 × 10^–5 = S × (2S)²; 4.42 × 10^–5 = 4S³
S = 2.224 × 10^–2 = 0.0223 moles litre^–1

∴ No. of moles of Ca²⁺ ions in 500 ml. of solution = λ

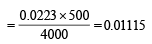
NOTE THIS STEP : Now when 500 ml. of saturated solution is mixed with 500 ml of 0.4M NaOH, the resultant volume is 1000 ml. The molarity of OH– ions in the resultant solution would therefore be 0.2 M.

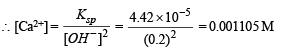
Thus, No. of moles of Ca²⁺ or Ca(OH)₂ precipitated = 0.01115 – 0.001105 = 0.010045

Mass of Ca(OH)₂ precipitated

= 0.010045 × 74 = 0.7433 g = 743.3 mg

[mole wt. of Ca(OH)₂ = 74]

Q.23. 0.15 mole of CO taken in a 2.5 l flask is maintained at 750 K along with a catalyst so that the following reaction can take place :

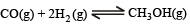
Hydrogen is introduced until the total pressure of the system is 8.5 atmosphere at equilibrium and 0.08 mole of methanol is formed. Calculate (i) K_p and K_c and (ii) the final pressure if the same amount of CO and H₂ as before are used, but with no catalyst so that the reaction does not take place.

Ans. (i) 0.05 atm^–2, 187.85 mol^–2 l², (ii) 12.438 atm

Solution. (i)

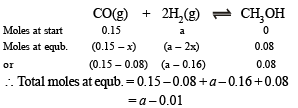
Total moles at equilibrium can also be calculated from the following relation

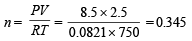
∴ 0.345 = a – 0.01 [Comparing (i) and (ii)]

or  a   =  0.355

Thus, Moles of CO at equilibrium = 0.15 – 0.08 = 0.07

Moles of H₂ at equilibrium = 0.355 – 0.16 = 0.195

Moles of CH₃OH at equilibrium = 0.08

Substituting the values in the relation,

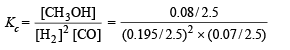
   =  187.85 mole^–2 litre2       [∵ V = 2.5 L]

Calculation of K_p

K_p = K_c (RT)^Δn = 187.85 × (0.0821 × 750)^–2 = 0.05 atm^–2

[∵ Δn = –2]

(ii) Calculation of final pressure when there is no reaction

Moles of CO = 0.15; Moles of H₂ = 0.355

∴ Total moles = 0.15 + 0.355 = 0.505

PV = nRT

P × 2.5 = 0.505 × 0.0821 × 750 ⇒ P = 12.438 atm.

Q.24. The pH of blood stream is maintained by a proper balance of H₂CO₃ and NaHCO₃ concentrations. What volume of 5M NaHCO₃ solution should be mixed with a 10 ml sample of blood which is 2M in H₂CO₃ in order to maintain a pH of 7.4 ? K_a for H₂CO₃ in blood is 7.8 × 10^–7. 

Ans. 78.36 ml

Solution. Volume of blood = 10 ml. (given)
[H₂CO₃] in blood = 2 M (given)
[NaHCO₃] to be added = 5 M (given)
Let volume of NaHCO₃ added in 10 ml blood = V ml

∴ [H₂CO₃] in blood mixture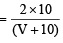 

[NaHCO₃] in blood mixture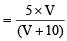

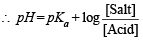
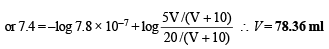
Q.25. An aqueous solution of a metal bromide MBr₂ (0.05M) is saturated with H₂S. What is the minimum pH at which MS will precipitate?

K_sp for MS = 6.0 x 10 ^-21; concentration of saturated H₂S = 0.1 M
 K₁ = 10^–7 and K₂ = 1.3 x 10^–13 , for H₂S.

Ans. 0.983

Solution. 

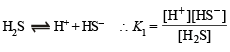
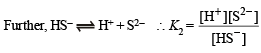
Dissociation constant of H₂S, K = K₁ × K₂

i.e.K = 1 × 10^–7 × 1.3 × 10^–13 = 1.3 × 10^–20

Now we know that

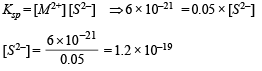
Substituting the various values in the following relation

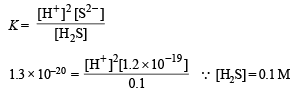
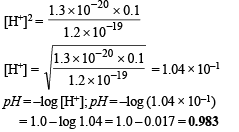
Q.26. At temperature T, a compound AB₂ (g) dissociates according to the reaction

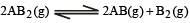
with a degree of dissociation x which is small compared with unity. Deduce the expression for x in terms of the equilibrium constant K_p and the total pressure, P.

Ans.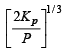 

Solution. 

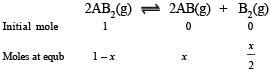
Total moles at equb.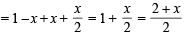

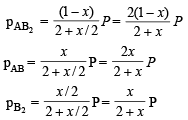
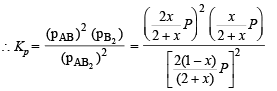
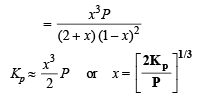
Q.27. For the reaction

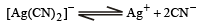
the equilibrium constant, at 25°C , is 4.0 x 10 ^-19 . Calculate the silver ion concentration in a solution which was originally 0.10 molar in KCN and 0.03 molar in AgNO₃.

Ans. 7.5 × 10^–18 M

Solution. TIPS/Formulae : Consider common ion effect

Conc. of Ag+ ions =  Conc. of AgNO3 = 0.03 M

Most of these Ag+ ions will be present in the form of [Ag(CN)₂]^–.

0.03 M AgNO₃ requires 2 × 0.03 M

= 0.06 M CN^– to form [Ag(CN)₂]^–

∴ Conc. of free CN^– at equilibrium will be 0.1 – 0.06 = 0.04 M

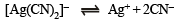
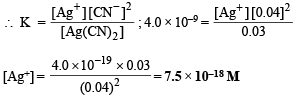
Q.28. Calculate the pH of an aqueous solution of 1.0 M ammonium formate assuming complete dissociation. 

(pK_a of formic acid = 3.8 and pK_b of ammonia = 4.8.)

Ans. 6.5

Solution. For ammonium formate which is a salt of weak acid with weak base, we know that

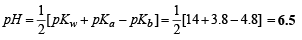
Q.29. What is the pH of a 0.50 M aqueous NaCN solution? pKb of CN^– is 4.70.

Ans. 11.5

Solution. 

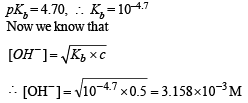
Now we know that
pOH  = –log[OH–]
pOH = –log 3.158 × 10^–3 = 2.5
or,  pH  = 14 – 2.5 = 11.5

Q.30. A sample of AgCl was tr eated with 5.0 0 mL of 1.5 M Na₂CO₃ solution to give Ag₂CO₃. The remaining solution contained 0.0026 g of Cl^– per litre. Calculate the solubility product of AgCl (Ksp(Ag₂CO₃) = 8.2 × 10^–12).

Ans. 1.71 × 10^–10

Solution. The concerned chemical reaction is

2AgCl + Na₂CO₃ → Ag₂CO₃ + 2 NaCl

Calculation of [Ag⁺] left in the solution :

 
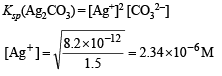
Concentration of Cl– left = 0.0026 g/l

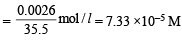
∴ Ksp(AgCl) = [Ag⁺] [Cl^–] = (2.34 × 10^–6) (7.33 × 10^–5) 

= 1.71 × 10^–10

Q.31. An acid type in dicator, HIn differ s in colour fr om its conjugate base (In^–). The human eye is sensitive to colour differences only when the ratio[In^–]/[HIn] is greater than 10 or smaller than. 0.1. What should be the minimum change in the pH of the solution to observe a complete colour change (Ka=1.0×10^–5)?

Ans. 2

Solution. Given K_a = 1 × 10^–5

∴ pK_a = 5

The two conditions when colour indicator will be visible are derived by

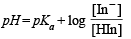
(i) pH = 5 + log 10 = 6

(ii) pH = 5 + log 0.1 = 4

Thus minimum change in pH = 2

Q.32. Given : 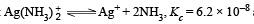and K_sp of AgCl = 1.8 × 10^–10  at 298 K. If ammonia is added to a water solution containing excess of AgCl(s) only, calculate the concentration of the complex in 1.0 M aqueous ammonia.

Ans. 0.0538 M

Solution. 

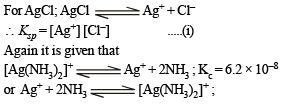
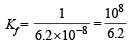
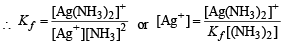
NOTE THIS STEP : Since the formation constant of the complex is very high, most of the [Ag⁺] which dissolves must be converted into complex and each Ag⁺ dissolved also requires dissolution of Cl^–.

∴ [Cl^–] = [Ag (NH₃)₂]⁺ and let it be c M

Equation (i) becomes

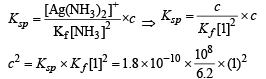
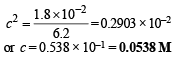
Q.33. What will be the resultant pH when 200mL of an aqueous solution of HCl (pH = 2.0) is mixed with 300  mL of an aqueous solution of NaOH (pH = 12.0) ?

Ans. 11.3010

Solution. 

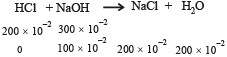
pH of HCl = 2, pH of  NaOH = 12

∴ [HCl] = 10^–2 M, ∴ [NaOH] = 10^–2 M

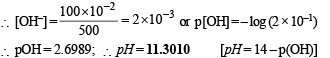
Q.34. When 3.06 g of solid NH₄HS is introduced into a two litre evacuated flask at 27° C, 30% of the solid decomposes into gaseous ammonia and hydrogen sulphide. (i) Calculate Kc and Kp for the reaction at 27°C. (ii) What would happen to the equilibrium when more solid NH₄HS is introduced into the flask ?

Ans. 8.1 × 10^–5 mol² l^–2, 4.90 × 10^–2 atm²

Solution. 

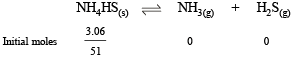
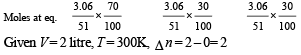
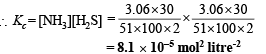
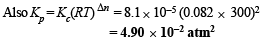
NOTE : Addition of more NH₄HS on this equilibrium will cause no effect because concentration of NH₄HS is not involved in formula of K_p or K_c.

Q.35. The solubility of Pb(OH)₂ in water is 6.7×10^–6 M. Calculate the solubility of Pb(OH)₂ in a buffer solution of  pH = 8.

Ans. 1.203 × 10^–3 mol litre^–1

Solution. 

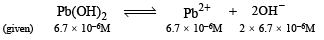
∴ K_sp = [Pb²⁺][OH^-]² = (6.7 × 10^{– 6}) (2 × 6.7 × 10^{– 6})²

= 1.203 ×10^–15

The buffer solution pH = 8 (given)

∴ pOH = 6 or [OH^–] = 10^–6

Thus in this buffer we have,   [Pb²⁺][OH^–]² = 1.203 x 10^–15

or  [Pb²⁺]  ×  [10^–6]² = 1.203 x 10^–15

∴ [Pb²⁺]   =  1.203 x 10^–3 mol litre^–1

Q.36. The average concentration of SO₂ in the atmosphere over a city on a certain day is 10 ppm, when the average temperature is 298 K. Given that the solubility of SO₂ in water at 298 K is 1.3653 moles litre^–1 and the pKa of H₂SO₃ is 1.92, estimate the pH of rain on that day.

Ans. 4.865

Solution. Amount of SO₂ in atmosphere =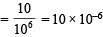 

Molar concentration of SO₂ present in water = Amount of SO₂ × Solubility of SO₂ in water = 10 × 10^–6 × 1.3653 mole L^–1 = 1.3653 × 10^–5 M

Writing the concerned chemical equation

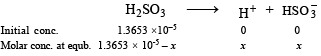
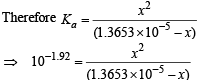
(pK_a = 1.92, ∴ K_a = 10^–1.92)

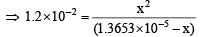
x² = 1.2 × 10^–2 (1.3653 × 10^-5 – x) On solving, x = 1.364 × 10^–5

Therefore, pH = – log (1.364 × 10^–5) = 4.865

Q.37. 500 mL of 0.2 M aqueous solution of acetic acid is mixed with 500 mL of 0.2 M HCl at 25°C. 

(i) Calculate the degree of dissociation of acetic acid in the resulting solution and pH of the solution.
 (ii) If 6 g of N aOH is a dded to th e above soluti on , determine the final pH. [Assume there is no change in volume on mixing; K_a of acetic acid is 1.75 × 10^-5 mol L^-1].

Ans. 1.75 × 10^–4, 1, 4.75

Solution. (i) The volume being doubled by mixing the two solutions, the molarity of each component will be halved i.e.

[CH₃COOH] = 0.1 M, [HCl] = 0.1 M.

NOTE :

HCl being a strong acid will remain completely ionised and hence H⁺ ion concentration furnished by it will be 0.1 M. This would exert common ion effect on the dissociation of acetic acid, (a weak acid.)

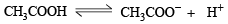
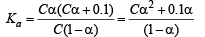
Since α is very very small, Ca² can be neglected and 1 – α can be taken as unity

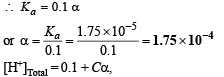
Cα is negligible as compared to 0.1.

(ii)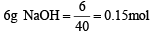 

0.1 mole of NaOH will be consumed by 0.1 mole of HCl.
Thus, 0.05 mole of NaOH will react with acetic acid according to the equation.

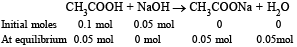
Thus, solution of acetic acid and sodium acetate will become acidic buffer. So pH of the buffer will be

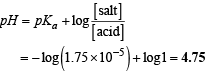
Q.38. Match the following if the molecular weights of X, Y and Z are same.

Ans. x = 0.63, y = 0.53, z = 0.98

Solution. TIPS/Formulae :

Higher the value of dipole-dipole interaction higher is b.p.
Higher value of K_b of a solvent suggests larger polarity of solvent molecules which in turn leads to higher dipole – dipole interaction implies higher boiling point due to dipole – dipole interaction. Therefore, the correct order of K_b values of the three given solvents is

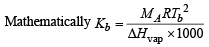
or  K_b ∝T_b (b.pt.)