JEE Advanced Previous Year Questions (2018 - 2023): Sets, Relations and Functions | Mathematics (Maths) for JEE Main & Advanced PDF Download
2023
Q1: Let S = (0, 1) ∪ (1, 2) ∪ (3, 4) and T = {0, 1, 2, 3}. Then which of the following statements is(are) true?
(a) There are infinitely many functions from S to T
(b) There are infinitely many strictly increasing functions from S to T
(c) The number of continuous functions from S to T is at most 120
(d) Every continuous function from S to T is differentiable [JEE Advanced 2023 Paper 1]
Ans: (a), (c) & (d)
S = (0, 1) ∪ (1, 2) ∪ (3, 4) and T = {0, 1, 2, 3}
Let domain and co-domain of a function y = f(x) are S and T respectively.
(A) There are infinitely many elements in domain and four elements in co-domain.
⇒ There are infinitely many functions from S to T.
⇒ Option (A) is correct
(B) If number of elements in domain is greater than number of elements in co-domain, then number of strictly increasing function is zero.
⇒ Option (B) is incorrect
(C) Maximum number of continuous functions = 4 × 4 × 4 = 64
(Every subset (0, 1),(1, 2),(3, 4) has four choices)
∵ 64<120⇒ option (C) is correct.
(D) For every point at which f(x) is continuous, f(x) = 0
⇒ Every continuous function from S to T is differentiable.
Option (D) is correct.
Q2: Let f : [0, 1] → [0, 1] be the function defined by 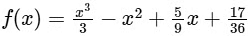 . Consider the square region S = [0, 1] × [0, 1]. Let
. Consider the square region S = [0, 1] × [0, 1]. Let 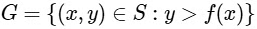 be called the green region and
be called the green region and 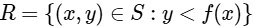 be called the red region. Let
be called the red region. Let 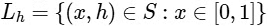 be the horizontal line drawn at a height ℎ ∈ [0, 1]. Then which of the following statements is(are) true?
be the horizontal line drawn at a height ℎ ∈ [0, 1]. Then which of the following statements is(are) true?
(a) There exists an ℎ ∈ [1/4, 2/3] such that the area of the green region above the line Lℎ equals the area of the green region below the line Lℎ
(b) There exists an ℎ ∈ [1/4, 2/3] such that the area of the red region above the line Lℎ equals the area of the red region below the line Lℎ
(c) There exists an ℎ ∈ [1/4, 2/3] such that the area of the green region above the line Lℎ equals the area of the red region below the line Lℎ
(d) There exists an ℎ ∈ [1/4, 2/3] such that the area of the red region above the line Lℎ equals the area of the green region below the line [JEE Advanced 2023 Paper 1]
Ans: (b), (c) & (d)
Given, 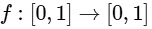
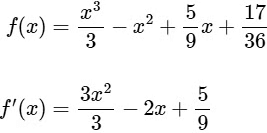
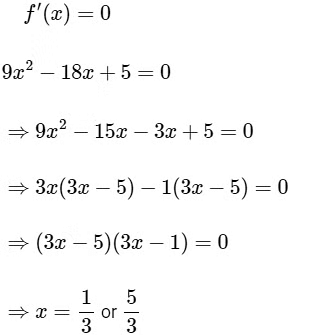
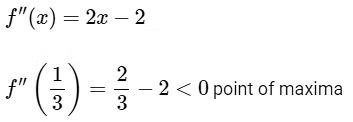
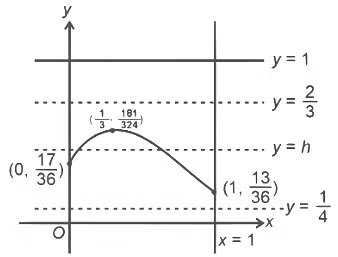
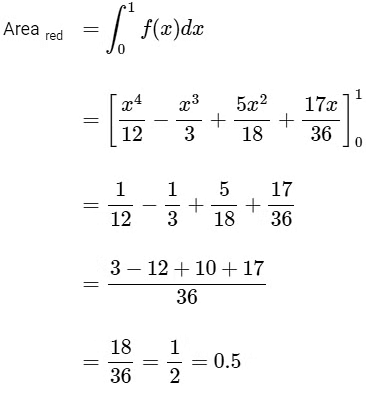
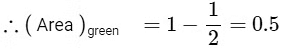
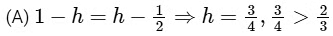 option (A) is incorrect
option (A) is incorrect
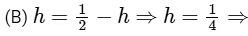 option (B) is correct.
option (B) is correct.
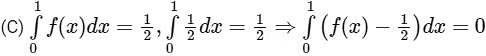
⇒ h= 1/2 ⇒ option (C) is correct.
(D) ∵ Option (C) is correct ⇒ option (D) is also correct.
2022
Q1: Let |M| denote the determinant of a square matrix M. Let 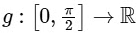 be the function defined by
be the function defined by

where
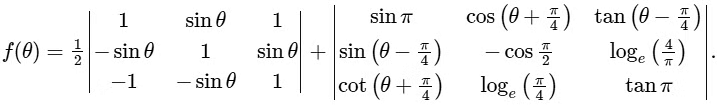
Let p(x) be a quadratic polynomial whose roots are the maximum and minimum values of the function g(θ), and p(2) =2 − √2. Then, which of the following is/are TRUE ?
(a) 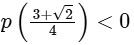
(b) 
(c) 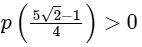
(d) 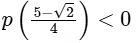 [JEE Advanced 2022 Paper 1]Ans: (a) & (c)
[JEE Advanced 2022 Paper 1]Ans: (a) & (c)
Given,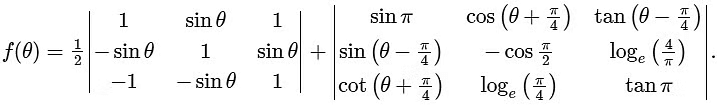
Here, 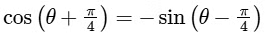
and 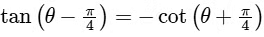
and 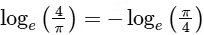
Also, 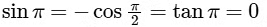
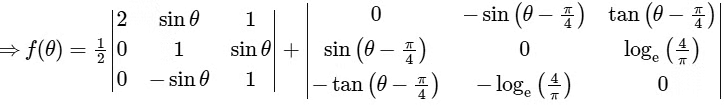
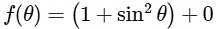 (skew symmetric)
(skew symmetric)
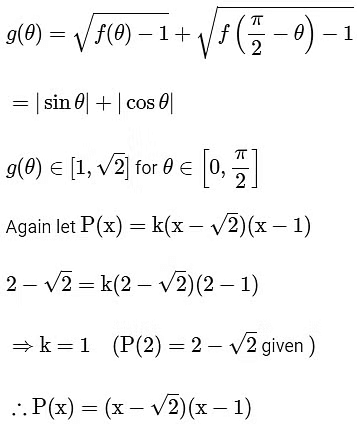
For option (A) 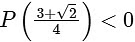 Correct.
Correct.
For option (B) 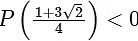 Incorrect.
Incorrect.
For option (C) 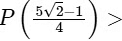 Correct.
Correct.
For option (D) 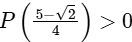 Incorrect.
Incorrect.
2020
Q1: Let the function f : [0, 1] → R be defined by [JEE Advanced 2020 Paper 2]
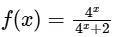 Then the value of
Then the value of 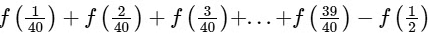 is ..........
is ..........
Ans: 19
The given function f : [0, 1] → R be define by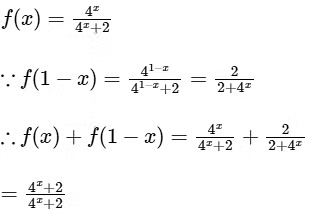
So, f(x) + f(1 − x) = 1 .....(i)
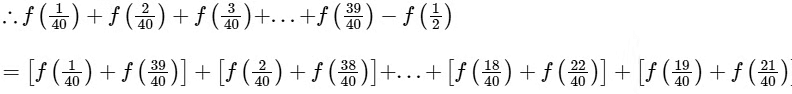
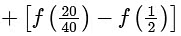
= 
=  (19 times) {from Eq. (i)}
(19 times) {from Eq. (i)}
= 19.
Q2: Let the function 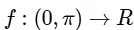 be defined by
be defined by 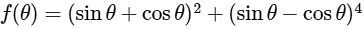 Suppose the function f has a local minimum at θ precisely when
Suppose the function f has a local minimum at θ precisely when 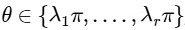 , where
, where 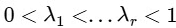 . Then the value of
. Then the value of  is ............. [JEE Advanced 2020 Paper 2]
is ............. [JEE Advanced 2020 Paper 2]
Ans: 0.5
The given function f : R → R be defined by
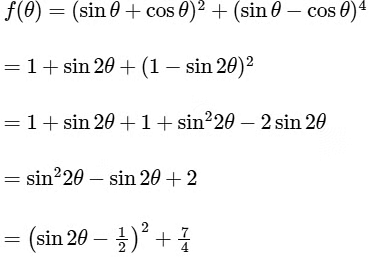
The local minimum of function 'f' occurs when
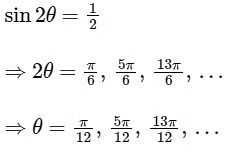
but 
Where, 
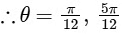
So, 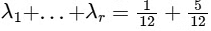 = 0.50
= 0.50
Q3: Let f : [0, 2] → R be the function defined by
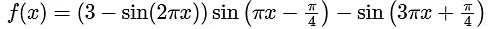
If 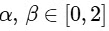 are such that
are such that 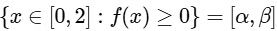 , then the value of β - α is .......... [JEE Advanced 2020 Paper 1]
, then the value of β - α is .......... [JEE Advanced 2020 Paper 1]
Ans: 1
The given function f : [0, 2] → R defined by
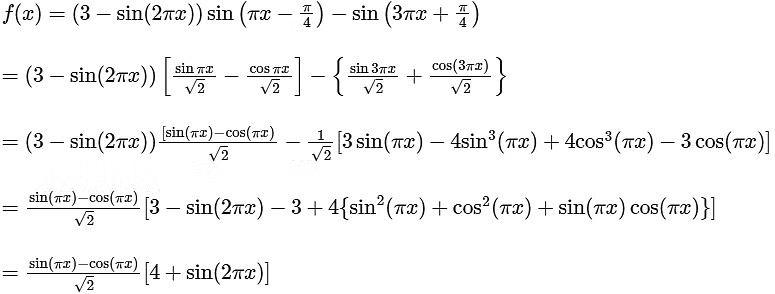
As, 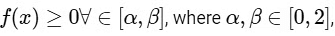 so,
so,
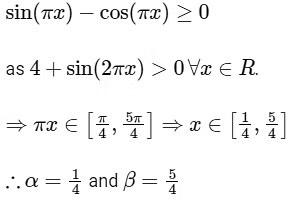
Therefore the value of (β - α) = 1
Q3: For a polynomial g(x) with real coefficients, let mg denote the number of distinct real roots of g(x). Suppose S is the set of polynomials with real coefficients defined by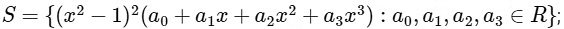 For a polynomial f, let f' and f'' denote its first and second order derivatives, respectively. Then the minimum possible value of (mf' + mf''), where f ∈ S, is .............. [JEE Advanced 2020 Paper 1]
For a polynomial f, let f' and f'' denote its first and second order derivatives, respectively. Then the minimum possible value of (mf' + mf''), where f ∈ S, is .............. [JEE Advanced 2020 Paper 1]
Ans: 5
Given set S of polynomials with real coefficients
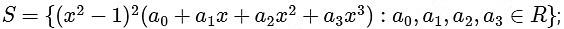
and for a polynomial f ∈ S, Let
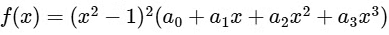 it have −1 and 1 as repeated roots twice, so graph of f(x) touches the X-axis at x = −1 and x = 1, so f'(x) having at least three roots x = −1, 1 and α. Where α ∈ (−1, 1) and f''(x) having at least two roots in interval (−1, 1)
it have −1 and 1 as repeated roots twice, so graph of f(x) touches the X-axis at x = −1 and x = 1, so f'(x) having at least three roots x = −1, 1 and α. Where α ∈ (−1, 1) and f''(x) having at least two roots in interval (−1, 1)
So, mf' = 3 and mf'' = 2
∴ Minimum possible value of (mf' + mf'') = 5
Q4: If the function f : R → R is defined by f(x) = |x| (x − sin x), then which of the following statements is TRUE?
(a) f is one-one, but NOT onto
(b) f is onto, but NOT one-one
(c) f is BOTH one-one and onto
(d) f is NEITHER one-one NOR onto [JEE Advanced 2020 Paper 1]
Ans: (c)
The given function f : R → R is
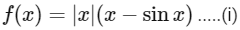
The function 'f' is a odd and continuous function and as 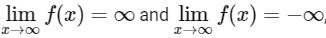 , so range is R, therefore, 'f' is a onto function.
, so range is R, therefore, 'f' is a onto function.
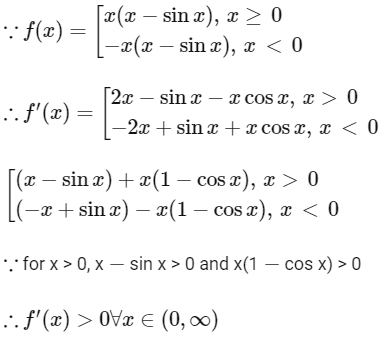
⇒ f is strictly increasing function. ∀x ∈(0, ∞).
Similarly, for x < 0, −x + sin x > 0 and (− x) (1 − cos x) > 0, therefore, f′(x)> 0∀ x ∈(−∞, 0)
⇒ f is strictly increasing function, ∀x ∈ (0, ∞)
Therefore 'f' is a strictly increasing function for x ∈ R and it implies that f is one-one function.
2018
Q1: Let X be a set with exactly 5 elements and Y be a set with exactly 7 elements. If α is the number of one-one functions from X to Y and β is the number of onto functions from Y to X, then the value of 1 / 5!(β − α) is .................. [JEE Advanced 2018 Paper 2]
Ans: 119
Given, X has exactly 5 elements and Y has exactly 7 elements.
∴ n(X) = 5
and n(Y) = 7
Now, number of one-one functions from X to Y is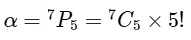 Number of onto functions from Y to X is β
Number of onto functions from Y to X is β
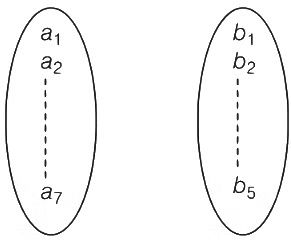
1, 1, 1, 1, 3 or 1, 1, 1, 2, 2
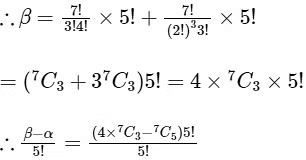 = 4 x 35 - 21= 140 - 21
= 4 x 35 - 21= 140 - 21
= 119
Q2: Let 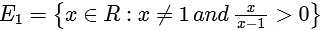 and
and 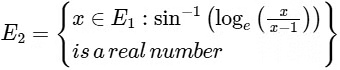 (Here, the inverse trigonometric function sin−1 x assumes values in [−π/2, π/2].).
(Here, the inverse trigonometric function sin−1 x assumes values in [−π/2, π/2].).
Let f : E1 → R be the function defined by 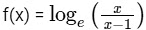 and g : E2 → R be the function defined by
and g : E2 → R be the function defined by 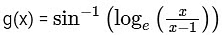 . [JEE Advanced 2018 Paper 2]
. [JEE Advanced 2018 Paper 2]
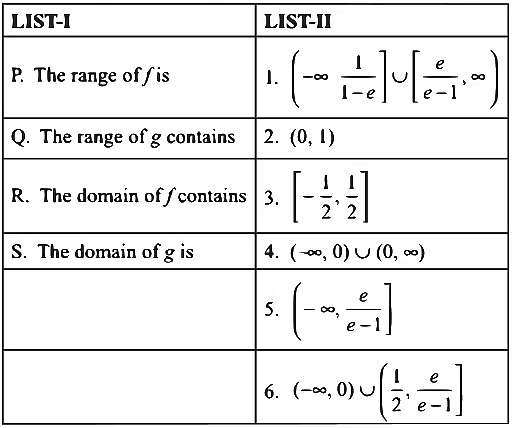
(a) P → 4; Q → 2; R → 1 ; S → 1
(b) P → 3; Q → 3; R → 6 ; S → 5
(c) P → 4; Q → 2; R → 1 ; S → 6
(d) P → 4; Q → 3; R → 6 ; S → 5 [JEE Advanced 2018 Paper 2]
Ans: (a)
We have,
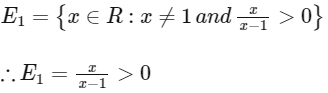

and

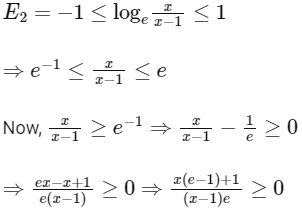
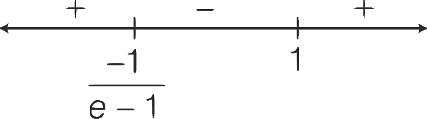
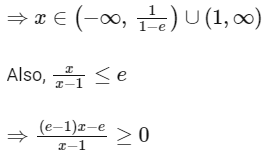
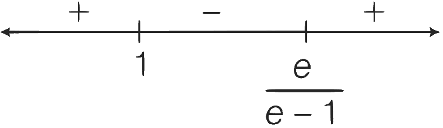
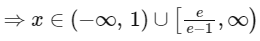
So, 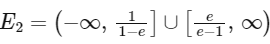
The domain of f and g are 
and Range of 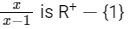
Range of f is R − {0} or (−∞, 0) ∪ (0, ∞)
Range of g is 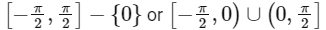
Now, P → 4, Q → 2, R → 1, S → 1
Hence, option (a) is correct answer.
|
209 videos|443 docs|143 tests
|
|
209 videos|443 docs|143 tests
|

|
Explore Courses for JEE exam
|

|

















