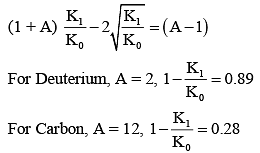Q.1. Consider the following radioactive decay process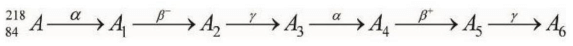
The mass number and the atomic number of A6 are given by: (JEE Main 2023)
(1) 210 and 84
(2) 210 and 82
(3) 211 and 80
(4) 210 and 80
Ans. (4)
Q.2. From the photoelectric effect experiment, following observations are made. Identify which of these are correct.
A. The stopping potential depends only on the work function of the metal.
B. The saturation current increases as the intensity of incident light increases.
C. The maximum kinetic energy of a photo electron depends on the intensity of the incident light.
D. Photoelectric effect can be explained using wave theory of light.
Choose the correct answer from the options given below: (JEE Main 2023)
(1) A, C, D only
(2) B, C only
(3) B only
(4) A, B, D only
Ans. (3)
Photoelectric effect is not explained by wave theory
Q.3. Assume that protons and neutrons have equal masses. Mass of a nucleon is 1.6 × 10−27 kg and radius of nucleus is 1.5 × 10−15 A1/3 m. The approximate ratio of the nuclear density and water density is n × 1013. The value of n is (JEE Main 2023)
Ans. 11
Q.4. A photon is emitted in transition from n = 4 to n = 1 level in hydrogen atom. The corresponding wavelength for this transition is (given, h = 4 × 10−15eVs ) : (JEE Main 2023)
(1) 99.3 nm
(2) 941 nm
(3) 974 nm
(4) 94.1 nm
Ans. (4)
Q.5. An a-particle, a proton and an electron have the same kinetic energy. Which one of the following is correct in case of their de-Broglie wavelength: (JEE Main 2023)
(1) λα < λp < λe
(2) λα = λp = λe
(3) λα > λp > λe
(4) λα > λp < λe
Ans. (1)
Q.6. The energy released per fission of nucleus of 240 X is 200MeV. The energy released if all the atoms in 120 g of pure 240X undergo fission is ______ × 1025MeV (Given NA = 6 × 1023) (JEE Main 2023)
Ans. 6
Q.7. A beam of electromagnetic radiation of intensity 6.4 x 10-5 W/cm2 is comprised of wavelength, λ = 310 nm. It falls normally on a metal (work function ϕ = 2eV) of surface area 1 cm2 . If one in 103 protons ejects an electron, total number of electrons ejected in 1s is 10x,(hc = 1240 eV nm, 1eV 1.6 10-19 J) then x is ________. (2020)
Ans. (11)
Given that λ = 310 nm, hc = 1240 eV nm We know that photons will eject electrons if the energy of incident beam is greater than work function, that is, E > ϕ
⇒ hf > ϕ ⇒ hc/λ > ϕ
⇒
⇒ 4 eV > 2 eV
So, electrons will be ejected.
Now number of electrons ejected in 1s is
ne =
Number of photon incident is given by
So,
Therefore, x = 11
Q.8. An electron (mass m) moving with initial velocity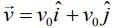 is in an electric field
is in an electric field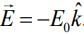 If λ0 is initial de-Broglie wavelength of electron, its de-Broglie wave length at time t is given by (2020)
If λ0 is initial de-Broglie wavelength of electron, its de-Broglie wave length at time t is given by (2020)
(1)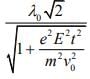
(2)
(3)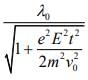
(4)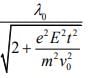
Ans. (3)
Given that
de-Broglie wavelength before entering in electric filed is given by
Velocity of electron after entering electric filed at time t is given by
Since, electric filed is at z direction so it effects only z direction velocity.
So, de-Broglie wavelength after entering in electric filed at time t is given by
=
Q.9. Radiation with wavelength 6561 Å falls on a metal surface to produce photoelectrons. The electrons are made to enter a uniform magnetic field of 3 × 10−4 T. If the radius of the largest circular path followed by the electrons is 10 mm, the work function of the metal is close to (2020)
(1) 1.1 eV
(2) 0.8 eV
(3) 1.6 eV
(4) 1.8 eV
Ans. (1)
Given that
Einstein’s Photoelectric Equation is given as............ (1)
Radius of circular path of charged particle is magnetic field is given by
For maximum radius K should be maximum. So,............. (2)
From Eqs. (1) and (2), we get
=
= 1.1eV
Q.10. A particle moving with kinetic energy E has de Broglie wavelength λ. If energy ΔE is added to its energy, the wavelength become λ/2. Value of ΔE, is (2020)
(1) E
(2) 4E
(3) 3E
(4) 2E
Ans. (3)
de Broglie wavelength is given by
So,
Q.11. A electron of mass m and magnitude of charge |e| initially at rest gets accelerated by a constant electric field E. The rate of change of de-Broglie wavelength of this electron at time t ignoring relativistic effects is (2020)
(a)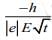
(b)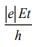
(c)
(d)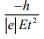
Ans. (4)
Electrostatic Force on a charge particle in a electric filed is given by
F = |e| E
Initially particle is at rest, that is, u = 0. So, velocity of particle after time t in electric filed is
De-Broglie wavelength is given by
So, the rate of change of de-Broglie wavelength of this electron at time t is given by
Q.12. The activity of a radioactive sample falls from 700/s to 500/s in 30 mins. Its half-life is close to (2020)
(1) 72 min
(2) 62 min
(3) 66 min
(4) 52 min
Ans. (2)
Given that, A0 = 700 /s, A = 500 /s and t = 30 min.
We know that, radioactivity at instant t is given by
We know that, half-life is given by
Q.13. The time period of revolution of electron in its ground state orbit in a hydrogen atom is 1.6 × 10−16s. The frequency of revolution of the electron in its first excited state (in/s) is (2020)
(1) 1.6 × 1014
(2) 7.8 × 1014
(3) 6.2 × 1015
(4) 5.6 × 1012
Ans. (2)
Given that T =
Time period of revolution of nth orbit of radius r is given by
here
So,
For hydrogen atom Z = 1, T ∝ n3
We have n = 1, T = 1.6 × 10-16s
For n = 2, we have
Therefore, frequency is
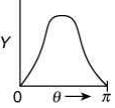
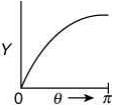
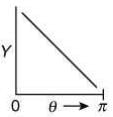
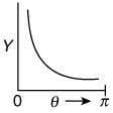
Q.14. The graph which depicts the results of Rutherford's gold foil experiment with α-particles is:
[θ: Scattering angle
Y: Number of scattered α-particles detected]
(Plots are schematic and not to scale) (2020)
(1)
(2)
(3)
(4)
Ans. (4)
Relation between scattering angle (θ) and number of scattered α-particle (Y) detected is given by
OR
So, plot of option (4) depicts the correct relation between scattering angle and number of scattered α particle detected.
Q.15. The first member of the Balmer series of hydrogen atom has a wavelength of 6561 Å. The wavelength of the second member of the Balmer series (in nm) is __________. (2020)
Ans. (486)
Given that
For Balmer series, wavelength is given by
For first member, we have................. (i)
For second member, we have................... (ii)
From Eqs. (1) and (2), we have= 486nm
Q.16. The energy required to ionise a hydrogen like ion in its ground state is 9 Rydbergs. What is the wavelength of the radiation emitted when the electron in this ion jumps from the second excited state to the ground state? (2020)
(1) 24.2 nm
(2) 11.4 nm
(3) 35.8 nm
(4) 8.6 nm
Ans. (2)
Given that EI = 9Rydbergs
We know that 1Rydberg = 13.6eV
Ionization energy is given by EI = 13.6 eV
So, 13.6Z2 = 9 x 13.6
Z2 = 9
Z = 3
The wavelength of the radiation emitted when the electron in this ion jumps from the
second excited state to the ground state is given by
Q.17. Surface of certain metal is first illuminated with light of wavelength λ1 = 350 nm and then, by light of wavelength λ2 = 540 nm. It is found that the maximum speed of the photoelectrons in the two cases differ by a factor of 2. The work function of the metal (in eV) is close to (2019)
(2019)
(1) 1.8
(2) 2.5
(3) 5.6
(4) 1.4
Ans. (1)
Now, dividing Eq. (1) by Eq. (2), we get
Q.18. The magnetic field associated with a light wave is given, at the origin, by B = B0[sin (3.14 × 107)ct + sin (6.28 × 107)ct]. If this light falls on a silver plate having a work function of 4.7 eV, what will be the maximum kinetic energy of the photoelectrons?
(c = 3 × 108 m/s, h = 6.6 × 10−34 J s) (2019)
(1) 6.82 eV
(2) 12.5 eV
(3) 8.52 eV
(4) 7.72 eV
Ans. (4)
Given, magnetic field associated with light wave is
B = B0 [sin(3.14 x 107 c)t + sin(6.28 x 107 c)t] ...(1)
where c is the speed of light
In above wave equation, there are two electromagnetic waves with different frequency.
To get maximum kinetic energy consider the photon with higher frequency
B1 = B0sin(π × 107 c)t
B2 = B0sin(2π × 107 c)t
v2 = 107c
v2 > v1
Kinetic energy of photoelectron will be a maximum for photon of higher energy.
EPh = hf = 6.6 × 10−34 × 107 × 3 × 106
= 6.6 × 3 × 10−19
Q.19. In an electron microscope, the resolution that can be achieved is of the order of the wavelength of electrons used. To resolve a width of 7.5 × 10−12 m, the minimum electron energy required is close to (2019)
(1) 500 keV
(2) 100 keV
(3) 1 keV
(4) 25 keV
Ans. (4)
Wavelength is given by
Therefore, required energy is
Q.20. In a photoelectric experiment, the wavelength of the light incident on a metal is changed from 300 nm to 400 nm. The decrease in the stopping potential is close to  (2019)
(2019)
(1) 0.5 V
(2) 1.5 V
(3) 1.0 V
(4) 2.0 V
Ans. (3)
The potential necessary to stop any electron from reaching the other side
λ1 = 300 nm
λ2 = 400 nm
For λ1
Subtracting Eq. (2) from Eq. (1), we get
Q.21. In a Frank–Hertz experiment, an electron of energy 5.6 eV passes through mercury vapor and emerges with an energy 0.7 eV. The minimum wavelength of photons emitted by mercury atoms is close to (2019)
(1) 1700 nm
(2) 2020 nm
(3) 220 nm
(4) 250 nm
Ans. (4)
In Frank–Hertz experiment
Q.22. When a certain photosensitive surface is illuminated with monochromatic light of frequency v, the stopping potential for the photocurrent is -V0/2 . When the surface is illuminated by monochromatic light of frequency v/2, the stopping potential is -V0. The threshold frequency for photoelectric emission is (2019)
(1) 5v/3
(2) 4v/3
(3) 2v
(4) 3v/2
Ans. (4)
Energy of incident photon is
From Eq. (1) and Eq. (2), we get
Q.23. A hydrogen atom, initially in the ground state is excited by absorbing a photon of wavelength 980 Å. The radius of the atom in the excited state, in terms of Bohr radius a0, will be (hc = 12,500 eV Å) (2019)
(1) 25 a0
(2) 9 a0
(3) 4 a0
(4) 16 a0
Ans. (4)
Energy of Photon is
Since, electron will excite to n = 4
Q.24. If the de Broglie wavelength of an electron is equal to 10−3 times the wavelength of a photon of frequency 6 × 1014 Hz, then the speed of electron is equal to (Given: speed of light = 3 × 108 m/s; Planck’s constant = 6.63 × 10−34 J s; mass of electron = 9.1 × 10−31 kg) (2019)
(1) 1.45 × 106 m/s
(2) 1.1 × 106 m/s
(3) 1.7 × 106 m/s
(4) 1.8 × 106 m/s
Ans. (1)
We have,
Q.25. In a hydrogen-like atom, when an electron jumps from the M-shell to the L-shell, the wavelength of emitted radiation is λ. If an electron jumps from N-shell to the L-shell, the wavelength of emitted radiation will be (2019)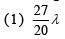
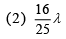
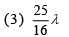
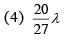
Ans. (4)
From M-shell to L-shell
From N-shell to L-shell
Dividing Eq. (1) by Eq. (2), we get
Q.26. A particle of mass m moves in a circular orbit in a central potential field 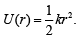 If Bohr’s quantization conditions are applied, radii of possible orbitals and energy levels vary with quantum number n as (2019)
If Bohr’s quantization conditions are applied, radii of possible orbitals and energy levels vary with quantum number n as (2019)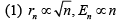
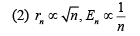
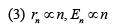
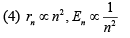
Ans. (1)
In circular motion
Bohr quantization is
From Eq. (1), we get
From Eq. (1), we get
Q.27. A particle A of mass m and charge q is accelerated by a potential difference of 50 V. Another particle B of mass 4 m and charge q is accelerated by a potential difference of 2500 V. The ratio of de Broglie wavelengths lA /lB is close to (2019)
(1) 10.00
(2) 0.07
(3) 14.14
(4) 4.47
Ans. (3)
Wave length is given by
Q.28. A sample of radioactive material A, that has an activity of 10 m Ci (1 Ci = 3.7 × 1010 decays/s), has twice the number of nuclei as another sample of a different radioactive material B which has an activity of 20 m Ci. The correct choices for half-lives of A and B would then be, respectively, (2019)
(1) 5 days and 10 days.
(2) 10 days and 40 days.
(3) 20 days and 5 days.
(4) 20 days and 10 days.
Ans. (3)
Activity is given as,
A = λN
for A λA NA = 10 (1)
for B λB NB = 20 (2)
Dividing Eq. (1) by Eq. (2), we get
(T1/2)A = 20 days
(T1/2)B = 5 days
Q.29. At a given instant, say t = 0, two radioactive substances A and B have equal activities. The ratio RB/RA of their activities after time t itself decays with time t as e−3t. If the half-life of A is ln 2, the half-life of B is (2019)
(1) 4ln2
(2) ln2/2
(3) ln2/4
(4) 2ln2
Ans. (3)
We know that,
N = N0e−λt
If T1/2 be the half-life period, then at t = T1/2 and N = N0/2
Half-life of A = ln 2 [ln = loge]
λA = 1
At t = 0 RA = RB
Q.30. Using a nuclear counter, the count rate of emitted particles from a radioactive source is measured. At t = 0 it was 1600 counts per second and t = 8 seconds it was 100 counts per second. The count rate observed, as counts per second, at t = 6 seconds is close to (2019)
(1) 200
(2) 150
(3) 400
(4) 360
Ans. (1)
At t = 0 s
Q.31. Consider the nuclear fission Ne20 → 2He4 + C12. Given that the binding energy/nucleon of Ne20, He4 and C12 are, respectively, 8.03 MeV, 7.07 MeV and 7.86 MeV, identify the correct statement: (2019)
(1) Energy of 12.4 MeV will be supplied.
(2) 8.3 MeV energy will be released.
(3) Energy of 3.6 MeV will be released.
(4) Energy of 11.9 MeV has to be supplied.
Ans. (4)
Ne2 → 2He4 + C12
Amount of energy
ΔE = 2 × (Binding energy of He4) + (Binding energy of C10) – (Binding energy of Ne20)
= 2 × (4 × 7.07) + (12 × 7.86) – (20 × 8.03)
= 56.56 + 94.32 – 160.6
= −9.72 MeV
Hence, the energy of 11.9 MeV has to be supplied.
Q.32. In a radioactive decay chain, the initial nucleus is 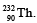 At the end there are 6 α-particles and 4 β-particles which are emitted. If the end nucleus is
At the end there are 6 α-particles and 4 β-particles which are emitted. If the end nucleus is 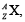 , A and Z are given by (2019)
, A and Z are given by (2019)
(1) A = 208; Z = 80
(2) A = 202; Z = 80
(3) A = 208; Z = 82
(4) A = 200; Z = 81
Ans. (3)
We have,
Hence Z = 82, A = 208 and element is Pb.
Q.33. An electron from various excited states of hydrogen atom emit radiation to come to the ground state. Let λn,λg be the de Broglie wavelength of the electron in the nth state and the ground state respectively. Let λn be the wavelength of the emitted photon in the transition from the nth state to the ground state. For large n, (A, B are constants) [2018]
(1) 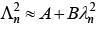
(2)
(3)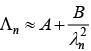
(4)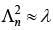
Ans: (3)
2πr = nλn
From equation (1) and (2)
Q.34. If the series limit frequency of the Lyman series is 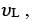 then the series limit frequency of the Pfund series is: [2018]
then the series limit frequency of the Pfund series is: [2018]
(1) 25 vL
(2) 16 vL
(3) 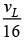
(4) 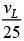
Ans: (4)
Series limit frequency of the Lyman series is given by
Series limit frequency of the Pfund series
Q.35. It is found that if a neutron suffers an elastic collinear collision with deuterium at rest, fractional loss of its energy is pd; while for its similar collision with carbon nucleus at rest, fractional loss of energy is pc. The values of pd and pc are respectively: [2018]
(1) (.89, .28)
(2) (.28, .89)
(3) (0, 0)
(4) (0, 1)
Ans: (1)
(from conservation of momentum)
and K0 = K1 + K2 (for elastic collision)
So after solving
Q.36. Two electrons are moving with non-relativistic speeds perpendicular to each other. If corresponding de Broglie wavelengths are λ1 and λ2, their de Broglie wavelength in the frame of reference attached to their centre of mass is: [2018]
(1) λCM = λ1 = λ2
(2) 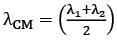
(3) 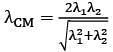
(4) 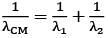
Ans: (3)
Momentum of each electron
Velocity of centre of mass
velocity of 1st particle about centre of mass

|
Explore Courses for JEE exam
|

|






















 ............ (1)
............ (1)
 ............. (2)
............. (2)


































 ................. (i)
................. (i) ................... (ii)
................... (ii)


 = 486nm
= 486nm




































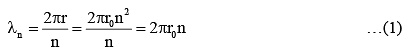

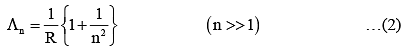
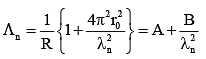

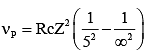
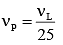

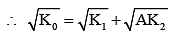 (from conservation of momentum)
(from conservation of momentum)