Class 9 Exam > Class 9 Notes > Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal
Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal - Class 9 PDF Download
Objective
To verify that the angles in the same segment of a circle are equalMaterials Required
Coloured glazed papers, Scissors, Drawing sheet, Geometry box, Sketch pens, Adhesive
Prerequisite Knowledge
(i) All basic informations related to a circle.
(ii) Knowledge about the segment of a circle and angles subtended on it.
Theory
- For basic information related to circle refer to Activity 23.
- The region between a chord and either of its arcs is called a segment of the circular region or simply a segment of the circle. This chord is called the base of the segment.
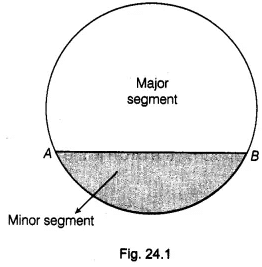The segment formed by minor arc along with chord, is called minor segment and the segment formed by major arc is called the major segment.If O is the centre of the circle, AB is an arc and P and O are two points on the remaining part of circle. Arc AB subtends ∠APB and ∠AQB in the same segment of the circle. (see Fig. 24.2)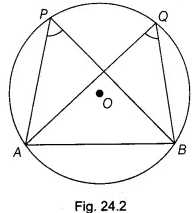
Procedure
- Take a piece of cardboard of suitable size and paste a drawing sheet on it.
- From the sheet of glazed paper, cut a circle of radius x units with centre O. (see Fig. 24.3)
- Paste the cut out circle on cardboard
- To form chord PQ, take two points P and 0 on the circle and join them, (see Fig. 24.4)
- Take a pair of points R and S on the circle in the same segment and join RP,RQ, SQ and SP. (see Fig. 24.5)
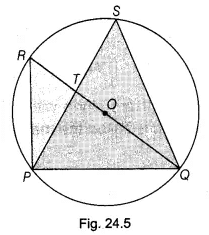
- Now, taking replicas of the ∠PRQ and ∠PSQ. (see Fig. 24.6)
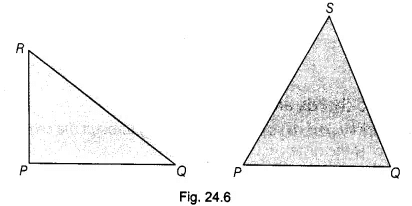
Demonstration
Put the cut outs of ∠PRQ and ∠PSQ on each other such that vertex R falls on vertex S (see Fig. 24.7). We find, ∠PRQ covers ∠PSQ completely, so ∠PRQ = ∠PSQ.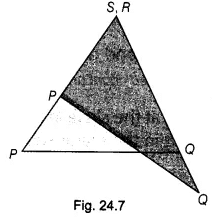
Observation
On actual measurement, we get
∠PRQ = ……… ,
∠PSQ = ……… ,
So, ∠PRQ = ∠PSQ.
Hence, the angles in the same segment are ……… .
Result
We have verified that the angles are equal in the same segment of a circle.
Application
This result can be used in proving other theorems/problems of geometry related to circles.
Related Exams
About this Document
Apr 11, 2025
Last updated
Document Description: Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal for Class 9 2025 is part of Class 9 preparation. The notes and questions for Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal have been prepared according to the Class 9 exam syllabus. Information about Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal covers topics like and Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Example, for Class 9 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal.
Introduction of Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal in English is available as part of
our Class 9 preparation & Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal in Hindi for Class 9
courses. Download more important topics, notes, lectures and mock test series for Class 9
Exam by signing up for free. Class 9: Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal - Class 9
Description
Full syllabus notes, lecture & questions for Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal - Class 9 - Class 9 | Plus excerises question with solution to help you revise complete syllabus | Best notes, free PDF download
Information about Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal
In this doc you can find the meaning of Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal defined & explained in the simplest way possible.
Besides explaining types of Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal theory,
EduRev gives you an ample number of questions to practice Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal tests, examples and also practice Class 9 tests.
Download as PDF
Top Courses for Class 9
Related Searches
ppt
,mock tests for examination
,video lectures
,Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal - Class 9
,shortcuts and tricks
,Extra Questions
,Summary
,Exam
,Semester Notes
,past year papers
,Objective type Questions
,Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal - Class 9
,MCQs
,Free
,study material
,Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal - Class 9
,Important questions
,practice quizzes
,Viva Questions
,Sample Paper
,Previous Year Questions with Solutions
;
Additional Information about Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal for Class 9 Preparation
Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Free PDF Download
The Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal is an invaluable resource that delves deep into the core of the Class 9 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal now and kickstart your journey towards success in the Class 9 exam.
Importance of Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal
The importance of Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal cannot be overstated, especially for Class 9 aspirants.
This document holds the key to success in the Class 9 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Notes
Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Notes on EduRev are your ultimate resource for success.
Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Class 9 Questions
The "Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal Class 9 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 9 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal on the App
Students of Class 9 can study Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Lab Manual: Verify that the Angles in the Same Segment of a Circle are Equal is prepared as per the latest Class 9 syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup