Method of Separation of Variables | Engineering Mathematics - Engineering Mathematics PDF Download
Introduction
Separable equations is an equation where dy/dx=f(x, y) is called separable provided algebraic operations, usually multiplication, division, and factorization, allow it to be written in a separable form dy/dx= F(x)G(y) for some functions F and G. Separable equations and associated solution methods were discovered by G. Leibniz in 1691 and formalized by J. Bernoulli in 1694.
Separable differentiable equation is one of the methods to solve the first order, first-degree differential equation. In this method separation of variables is used to find the general solution of the differential equation. And the equation of first order, first-degree differential equation can be written in this form-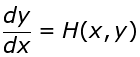 We can express H(x,y) as product of f(x) g(y). So the equation of separable differential equation is-
We can express H(x,y) as product of f(x) g(y). So the equation of separable differential equation is-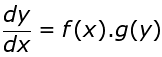 Address treating differential algebraically by just separating x’s and y’s and integrating respectively
Address treating differential algebraically by just separating x’s and y’s and integrating respectively
Identifying separable equations
To solve differential equations using the separable differential method we have to separate the variable. More concisely, a first-order differential equation is separable if and only if it can be written as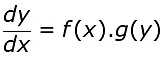
If this factoring is not possible, the equation is not separable.
where,
f(x) is a function of x that does not contain y.
g(y) is a function of y that does not contain x.
If factorization is possible then we will bring it into this form to find the general solution of the differential equation-
g(y) dy = f(x) dx
Note: In order to solve this type of differential equation we have to separate all y’s on one side and x’s on other side of the equal sign.
But this method is not applicable to all equations.
Sample Examples
Example 1: Find that the differential equation 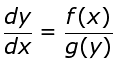 is variable separable or not?
is variable separable or not?
As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
f(x)dx = g(y)dy
Example 2: Find that the differential equation dy/dx = f(x).g(y) is variable separable or not?
As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
dy/g(y) = f(x)dx
Example 3: Find that the differential equation dy/dx = f(x) + g(y) is variable separable or not?
As you can see the following differential equation cannot be expressed in the required form, so it cannot be solved using separation of variables.
dy/dx = f(x) + g(y)
Finding a specific solution to a Separable Equation
So as we have seen how to “identify the separable equation” we can easily solve it to find the general solution of the differential equation.
By following steps:
- Try to factorize x’s and y’s in the given differential equation.
- Bring the x’s on side of equal and y’s on other side.
- Now integrate both the sides with respectively to x and y. Don’t forget “+ C” (the constant of integration).
Sample Problems
Example 1: Find the general solution of the differential equation dy/dx = (x+1)/(2-y), (y≠2).
Steps 1: As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
dy/dx = (x+1)/(2-y)
Step 2: Bring the x’s on side of equal and y’s on other side.
(2-y) dy = (x+1) dxStep 3: Integrate both the sides respectively to x and y, C is the constant of integration.
∫(2-y) dy = ∫(x+1) dx
∫2 dy- ∫y dy = ∫x dx + ∫1 dx
2y − (y2/2) = (x2/2) + x + C
2y − (y2/2) − (x2/2) − x − C = 0
The general solution of the differential equation is-
2y − (y2/2) − (x2/2) − x − C = 0
Example 2: Find the general solution of the differential equation dy/dx = (1+y2)/(1+x2).
Since 1 + y2 ≠ 0, therefore separating the variables, in the given differential.
Step 1: As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
dy/dx = (1+y2)/(1+x2)
Step 2: Bring the x’s on side of equal and y’s on other side.
dy/(1+y2) = dx/(1+x2)
Step 3: Integrate both the sides respectively to x and y, C is the constant of integration.
∫dy/(1+y2) = ∫dx/(1+x2)Tan-1y = Tan-1x + C
Tan-1x – Tan-1y + C = 0
The general solution of the differential equation is-
Tan-1x – Tan-1y + C = 0
Example 3: Find the general solution of the differential equation dy/dx=-4xy2.
Since y2 ≠ 0, therefore separating the variables, in the given differential.
Step 1: As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
dy/dx=-4xy2
Step 2: Bring the x’s on side of equal and y’s on other side.
dy/y2=-4x dx
Step 3: Integrate both the sides respectively to x and y, C is the constant of integration.
∫dy/y2=∫-4x dx
-(1/y)=-4(x2/2)+C
-(1/y)=-2x+C
-2x+(1/y)+C=0
The general solution of the differential equation is-
-2x+(1/y)+C=0
Separable equation with an implicit solution
As far as we have seen how to identify and solve separable differential equations and find a general solution of it. Now we will see how to solve the separable differential equation and find the implicit solution.
An implicit solution is when you have f(x, y) = g(x, y) which means that y and x are mixed together. y is not expressed in terms of x only. You can have x and y on both sides of the equal sign or you can have y on one side and x, y on the other side. Separating differential equations into x and y parts. But we can’t come up with y = f(x) solution. That’s because it’s an implicit solution, also known as any solution you can’t write like y = f(x). Implicit is when the dependent variable cannot be separated.
Finding the implicit solution is almost same as that of finding the general solution of the separable differential equation.
By following steps-
- Try to factorize x’s and y’s in the given differential equation.
- Bring the x’s on side of equal and y’s on other side.
- Now integrate both the sides with respectively to x and y. Don’t forget “+ C” (the constant of integration).
- Now we will put the values of x and y in the general solution given in question and find the value of C.
- Now we will put the value of C in general solution, and we will get the implicit solution.
Sample Problem
Example 1: Solve the following differential equation dy/dx=6xy2, y(1)=1/25.
Step 1: As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
dy/dx=6xy2
Step 2: Bring the x’s on side of equal and y’s on other side.
dy/y2 = 6x dx
Step 3: Integrate both the sides respectively to x and y, C is the constant of integration.
∫dy/y2 = ∫6x dx
∫dy/y2 = 6∫x dx
-(1/y) = 6(x2/2)+C
-(1/y) = 3x2+C
The general solution of the differential equation is-
-(1/y) = 3x2+C
Step 4: Put the values of x and y in the general solution given in question and find the value of C.-25 = 3+C
C = -28
The implicit solution is-
-(1/y) = 3x2-28
Example 2: Solve the following differential equation dy/dx=(3x2+4x-4)/(2y-4), y(1) = 3.
Step 1: As you can see the following differential equation can be expressed in the required form, so it can be solved using separation of variables.
dy/dx = (3x2+4x-4)/(2y-4)
Step 2: Bring the x’s on side of equal and y’s on other side.
(2y-4)dy = (3x2+4x-4)dx
Step 3: Integrate both the sides respectively to x and y, C is the constant of integration.
∫(2y-4)dy = ∫(3x2+4x-4)dx
∫2y dy-∫4 dy = ∫3x2 dx+∫4x dx-∫4 dx
y2-4y = x3+2x2-4x+C
The general solution of the differential equation is-
y2-4y =x3+2x2-4x+C
Step 4: Put the values of x and y in the general solution given i n question and find the value of C.
32-4*3 = 13+2*12-4*1+C
C = -2
The implicit solution is-y2-4y = x3+2x2- 4x - 2
|
65 videos|129 docs|94 tests
|
















