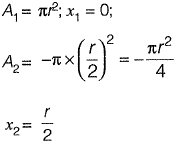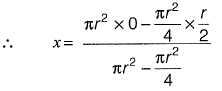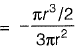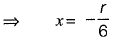Past Year Questions: Engineering Mechanics | Topic wise GATE Past Year Papers for Civil Engineering - Civil Engineering (CE) PDF Download
Q1: A linearly elalstic beam of length 2∣ with flexural rigidity El has negligible mass. A massless spring with a spring constant k and a rigid block of mass mm are attached to the beam as shown in the figure.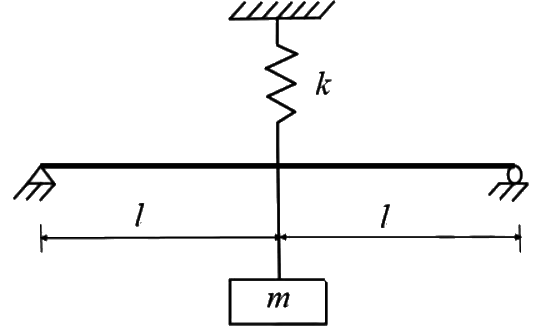
The natural frequency of this system is [GATE CE 2024 SET-2]
(a) 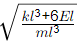
(b) 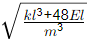
(c) 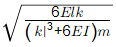
(d) 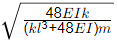
Ans: (a)
Let us consider sfittness of beam as kb. Here both the stiffness elements are in parallel.
⇒ keq = k1 + k2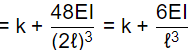
Natural frequency 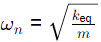
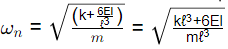
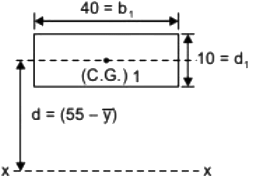
Q1: The cross-section of a girder is shown in the figure (not to scale). The section is symmetric about a vertical axis (Y−Y). The moment of inertia of the section about the horizontal axis (X−X) passing through the centroid is _____ cm4 (round off to nearest integer). [GATE CE 2023 SET-1]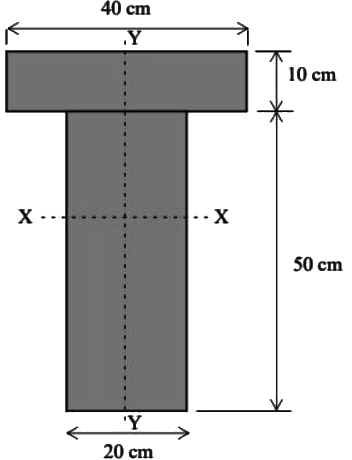 Ans: 464000 to 472000
Ans: 464000 to 472000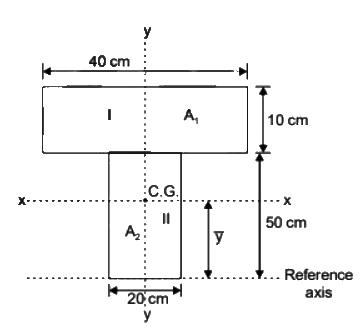 As section is symmetric about given y−y axis, hence centroid will lie on this axis.
As section is symmetric about given y−y axis, hence centroid will lie on this axis.
To calculate location of C.G. from reference axis (bottom horizontal line); 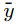
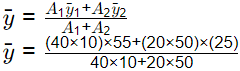
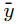 = 33.5714 cm (from bottom)
= 33.5714 cm (from bottom)
MOI of section about xx (centroidal horizontal axis)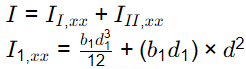
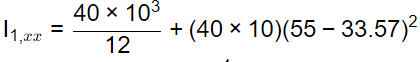
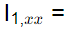 187031.29 cm4
187031.29 cm4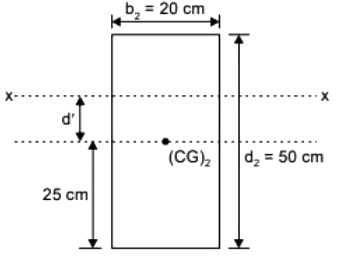
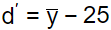
d′ = 8.5714 cm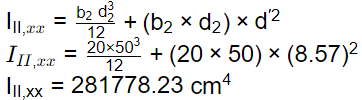
Hence, MOI of whole section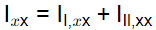
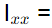 468809.52 cm4
468809.52 cm4
Q1: An undamped spring-mass system with mass m and spring stiffness k is shown in the figure. The natural frequency and natural period of this system are ω rad/s and T s, respectively. If the stiffness of the spring is doubled and the mass is halved, then the natural frequency and the natural period of the modified system, respectively, are [GATE CE 2022 SET-2]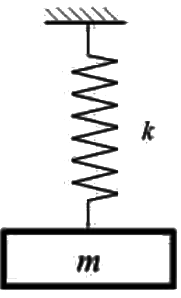 (a) 2ω rad/s and T/2 s
(a) 2ω rad/s and T/2 s
(b) ω/2 rad/s and 2T s
(c) 4ω rad/s and T/4 s
(d) ω rad/s and T s
Ans: (a)
This is question of undamped free vibration of sinle degree of freedom system. For the above figure it is given that natural frequency and natural period are w rad/s and T s, respectively. The equation for soof system is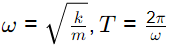
For the modified system, stiffness of the spring is doubled and the mass is halved.
Let us assume k' = 2k and m' = m/2, here k' and m' represent the stiffness and mass of the modified system respectively.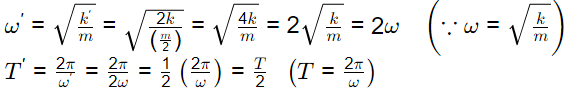
ω′ and T′ are natural frequency and natural period of the modified system.
hence, the natural frequency of the modified system is doubled and natural period is halved compared to the original given system. Hence option (A) is correct.
Q1: A wedge M and a block N are subjected to forces P and Q as shown in the figure. If force P is sufficiently large, then the block N can be raised. The weights of the wedge and the block are negligible compared to the forces P and Q. The coefficient of friction (μ) along the inclined surface between the wedge and the block is 0.2. All other surfaces are frictionless. The wedge angle is 30º.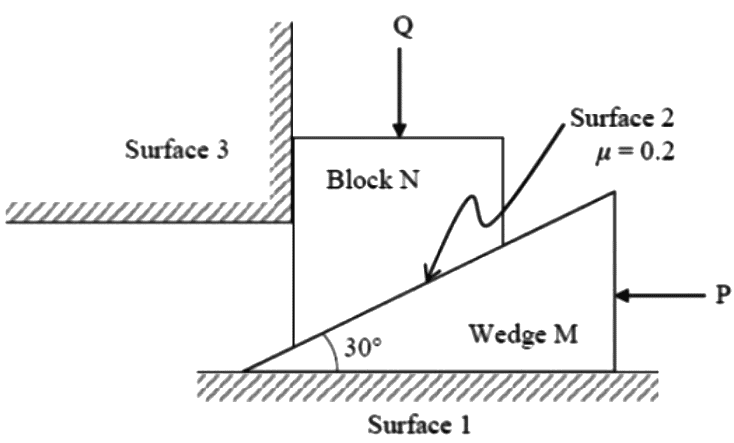
The limiting force P, in terms of Q, required for impending motion of block N to just move it in the upward direction is given as P = αQ. The value of the coefficient '?' (rounded off to one decimal place) is [GATE CE 2021 SET-1]
(a) 0.6
(b) 0.5
(c) 2.0
(d) 0.9
Ans: (d)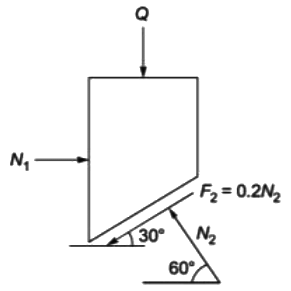 ΣY = 0 ⇒
ΣY = 0 ⇒
N2 sin 60º − 0.2N2 sin 30º − Q = 0
Q = 0.766 N2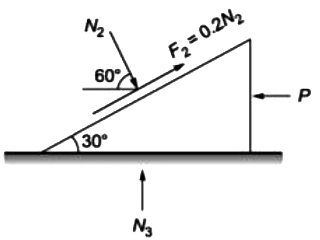 ΣX = 0
ΣX = 0
⇒ 0.2N2 cos 30º + N2 cos60º − P = 0
P = 0.67 N2
⇒ P = 0.67 x (Q/0.766)
⇒ P = 0.875Q 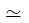 0.9Q
0.9Q
P = αQ
⇒ α = 0.9
Q1: A simple mass-spring oscillatory system consists of a mass m, suspended from a spring of stiffness k. Considering z as the displacementof the system at any time t, the equation of motion for the free vibration of the system is 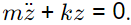 The natural frequency of the system is [GATE CE 2019 SET-1]
The natural frequency of the system is [GATE CE 2019 SET-1]
(a) k/m
(b) 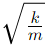
(c) m/k
(d) 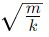
Ans: (b)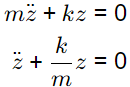
Comparing with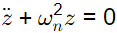
We get 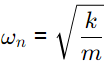
Q1: A cable PQ of length 25 m is supported at two ends at the same level as shown in the figure. The horizontal distance between the supports is 20 m. A point load of 150 kN is applied at point R which divides it into two equal parts. [2018 : 2 Marks, Sel-II]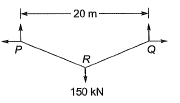
Neglecting the self-weight of the cable, the tension (in kN, integer value) in the cable due to the applied load will be ________.
Ans: Method-I
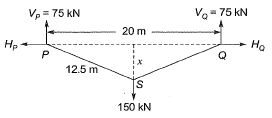
12.52 = x2 + 102
⇒ x = 7.5 m
Bending moment at S = 0 (Consider the left part)
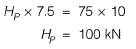
Tension in cable
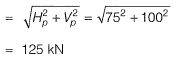
Using sine rule at joint S
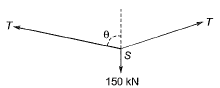
Calculating θ,
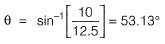
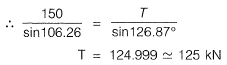
Q2: Two rigid bodies of mass 5 kg and 4 kg are at rest on a frictionless surface until acted upon by a force of 36 N as shown in the Figure. The contact force generated between the two bodies is [2018 : 2 Marks, Set-II]
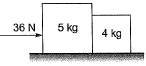
(a) 4.0 N
(b) 7.2 N
(c) 9.0 N
(d) 16.0 N
Ans: (d)
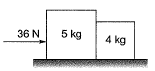
As after action of 36 N, both blocks will move with same acceleration so considering 5 kg and 4 kg together in a system and applying Newton’s 2nd law.
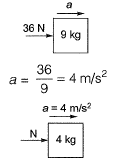
N = ma {Newton’s 2nd law}
N= 4 x 4 = 16 N
Q3: A cylinder of radius 250 mm and weight, W= 10 kN is rolled up an obstacle of height 50 mm by applying a horizontal force P at its centre as shown in the figure. [2018 : 2 Marks, Set-I]
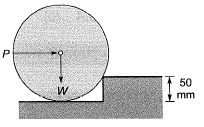
All interfaces are assumed frictionless. The minimum value of P is
(a) 4.5 kN
(b) 5.0 kN
(c) 6.0 kN
(d) 7.5 kN
Ans: (d)
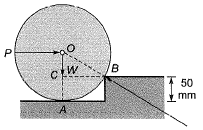
Given: r = 250 mm, W = 10 kN
Note:
1. When the cylinder will be about to move out of the cylinder, it will loose its contact at point A, only contact will be at point B.
2. Considering equation of cylinder of that instant under P, W and RB (contact force at B).
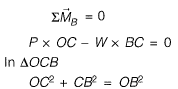
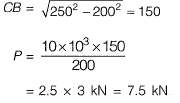
Q4: An aircraft approaches the threshold of a runway strip at a speed of 200 km/h. The pilot decelerates the aircraft at a rate of 1.697 m/s2 and takes 18 s to exit the runway strip. If the deceleration after exiting the runway is 1 m/s2, then the distance (in m, up to one decimal place) of the gate position from the location of exit on the runway is ____ [2018 : 2 Marks, Set-I]
Ans: Speed of aircraft,
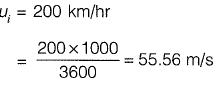
Deceleration of aircraft on the runway
= 1.697 m/s2
Aircraft takes 18 sec to exit the runway strip. Speed of the aircraft at the exit of the runway
uf = ui + at
= 55.56 - 1.697 x 18
= 25.014 m/s
After runway aircraft decelerate with 1 m/s2
Total distance travelled by the aircraft from the location of exit on the runway
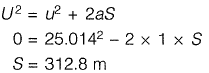
Q1: A particle of mass 2 kg is travelling at a velocity of 1.5 m/s. A force f (t) = 3t2 (in N) is applied to it in the direction of motion for a duration of 2 seconds, where f denotes time in seconds. The velocity (in m/s, up to one decimal place) of the particle immediately after the removal of the force is ___________. [2017 : 2 Marks, Set-I]
Ans: m = 2 kg, V0 = 1.5 m/sec
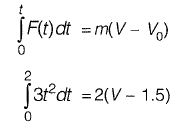
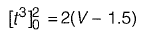
⇒ (8 - 0) = 2 (V - 1.5)
V = 5.5 m/s
Q2: The reaction rate involving reactants A and B is given by -k [A]α [B]β. Which one of the following statements is valid for the reaction to be a first- order reaction? [2017 : 1 Mark, Set-I]
(a) α = 0 and β = 0
(b) α = 1 and β = 0
(c) α = 1 and β = 1
(d) α = 1 and β = 2
Ans: (b)
The order of reaction is the exponent to which its concentration term in the rate equation is raised.
Rate, r = k [A]α [B]β
For first order reaction,
α + β = 1
Q1: The magnitudes of vectors P, Q and R are 100 kN, 250 kN and 150 kN, respectively as shown in the figure
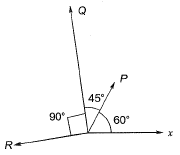
The respective values of the magnitude (in kN) and the direction (with respect to the x-axis) of the resultant vector are [2016 : 2 Marks, Set-I]
(a) 290,9 and 96.0°
(b) 368.1 and 94.7°
(c) 330.4 and 118.9°
(d) 400.1 and 113.5°
Ans: (c)
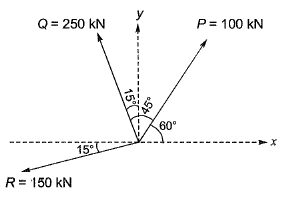
Resultant force in x-direction,
Rx = P cos60° - Q sin15° - R sin15°
= 100 cos60° - 250 sin15° - 150 cos15°
= -159.59 kN
= 159.59 kN
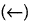
Resultant force in y-direction,
Ry = Psin60° + Qcos15° - Rsin15°
= 100 sin60° + 250 cos15° - 150 sin15°
= 289.26 kN
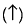
Final resultant force,
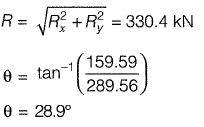
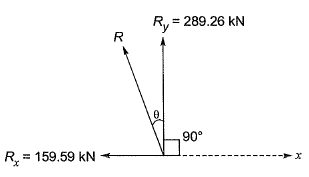
Direction with respect to x-axis
= θ + 90°
= 28.9° + 90°
= 118.9°
Q2: An assembly made of a rigid arm A-B-C hinged at end A and supported by an elastic rope C-D at end C is shown in the figure. The members may be assumed to be weightless and the lengths of the respective members are as shown in the figure
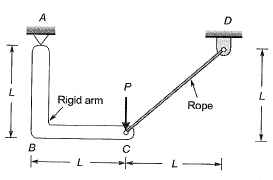
Under the action of a concentrated load P at Cas shown, the magnitude of tension developed in the rope is [2016 : 1 Mark, S e t-II]
Ans: (b)
Method-I
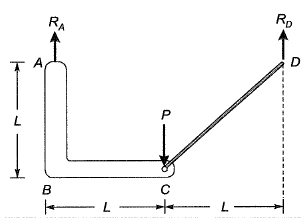
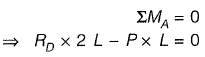
⇒ RD = P/2
At joint D:
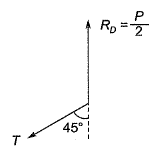
∑Fy = 0
⇒
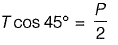
∴
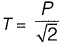
Method-ll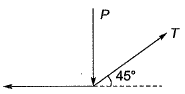
Using sine rule at joint C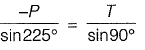
∴ 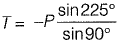
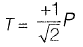
Q1: Polar moment of inertia (Ip), in cm4, of a rectangular section having width, b = 2 cm and depth, d = 6 cm is _____________. [2014 : 1 Mark, Set-II]
Ans: Polar moment of inertia is measure of an object’s ability to resist torsion under specified axis when and torque is being applied. It is also known as “second moment of area, “area moment of inertia”, “polar moment of area” or “second area moment”. Polar moment of inertia,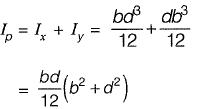
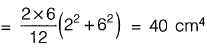
Q1: A disc of radius r has a hole of radius r/2 cut-out as shown. The centroid of the remaining disc (shaded portion) at a radial distance from the centre “O” is [2010:2 Marks]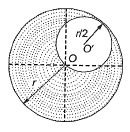
(a) r/2
(b) r/3
(c) r/6
(d) r/8
Ans: (c)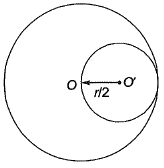
The centroid of the shaded portion of the disc is given by,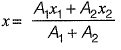
where x is the radial distance from O.