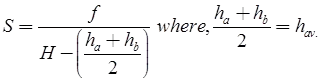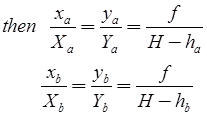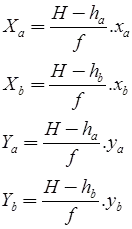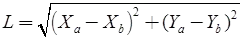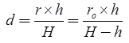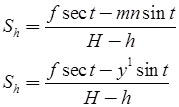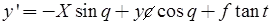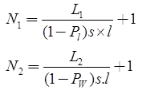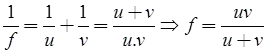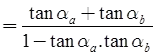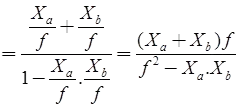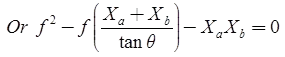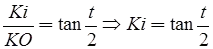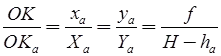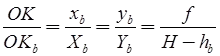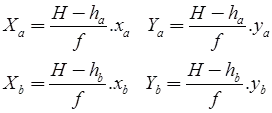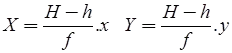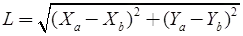Photogrammetry | Geomatics Engineering (Surveying) - Civil Engineering (CE) PDF Download
Photogrammetric Surveying
- Scale Vertical Photograph
Scale S = Map distance / Ground distance = f / (H - h)
H = height of exposure station (or the air plane) above the mean sea level.
h = Height of ground above MSL
f = Focal length of camera
(i) If A and B are two points on ground having elevations ha and hb above MSL, then Average scale of line joining A and B is given by.
(ii) Datum scale
S = f / H
(iii) Scale of a photograph
Sh = l / L
Photo scale / Map scale = Photo distance / Map distance
where, l = distance in photograph
L = distance in ground
(iv) Computation of length of the line between points of different elevations from measurement on a vertical photograph.
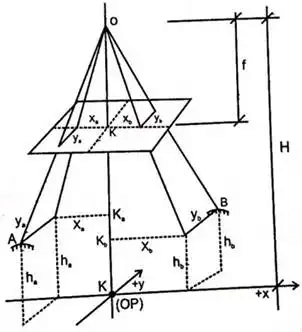
If A and B be two ground point having elevations ha and hb above MSL and coordinates (Xa, Ya) and (Xb, Yb)
Let a and b be the position of corresponding points in the photograph and (xa, ya) and (xb, yb) be the corresponding coordinates.
where,
The length between AB is given by
(v) Relief displacement on a Vertical Photograph
When the ground is not horizontal the scale of the photograph varies from point to point. The ground relief is shown in perspective on the photograph. Every point on the Photograph is therefore, displaced from true orthography position. This displacement is called relief displacement.
(vi) Relief displacement
d = Rfh / H(H - h)
(a) The relief displacement increases as the distance from the principal point increases.
(b) d α I/H - Scale of a tilted photograph

where,
θ = 180-s
s = Swing
t = tilt
f = Focal length
H = Flying height above datum
h = high of ground above datum.
It can be seen that the tilt and relief displacements tend to cancel in the upper part of the photograph while they are cumulative to the lower part. - Overlap in the Photographs
Longitudinal overlap = 55 to 65%
Lateral Overlap = 15 to 35%
for maximum rectangular area, to be covered by one photograph, the rectangle should have the dimension in the flight to be one-half the dimension normal to the direction of flight.
W = 2BW = 1.22H W = width of ground % overlap ≈ 60% in longitudinal direction. - Number of Photographs to Cover a Given Area
N = A / a
A = Total area to be photographed
a = net ground area covered by each photography
N = number of photographs required.
a = L × W
L = (1 - P1)s.l.
W = (1 - Pw)s.l.
a = l.ws2(1 - P1)(1 - Pw)
where, l = length of photograph in direction of flight
W = width of photograph.
Pl = % lap in longitudinal direction
Pw = lap in longitudinal direction
S = Scale of Photograph = H / f
If instead of total area A, the rectangular dimensions L1 × L2 (parallel and Transverse to flight) are given then, the number of photograph required are given as follows.
Let L1 = Dimension of area parallel to the direction flight
L2 = Dimension of area Transverse the direction of flight
N1 = Number of photographs in each strip
N2 = Number of strips required.
N = Total number of photographs to cover the whole area.
N = N1 x N2
(i) Interval between exposures
T = (3600 x L) / V
V = ground speed of aeroplane KMPH.
L = ground distance covered by each photograph in the direction of flight = (1 – Pl) s.l …… in Km
Photogrammetry
- Terrestrial photogrammetry: Photographs are taken from a fixed position on or near the ground.
- Aerial Photogrammetry: Photographs are taken from a camera mounted in an aircraft flying over the area.
Phototheodolite: It is a combination of “theodolite and a terrestrial camera.
Important parts are:
- Camera Box of a fixed focus type.
- Hollow rectangular frame consists of two cross hair.
- Photographic plate
- Theodolite
Important Definitions
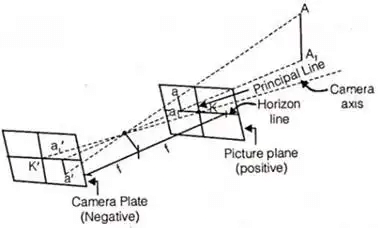
- Camera Axis: Line passing through center of camera lens perpendicular both to camera plate (Negative) and picture plane (photograph).

- Picture Plane: Positive plane, perpendicular to camera axis.
- Principal point: K or K’ point on intersection of camera axis with either picture plane or the camera plate.
- Focal length (f): Perpendicular distance from centre of camera lens to either to picture plane or camera plate. It satisfy the relation.

- Nodal point: Nodal point is either of two points on the optical axis of a lens so located that when all object distances are measured from one point, and all image distances are measured from other. They satisfy the simple lens relation.
1 / f = 1 / u + 1 / v - Principal plane: It is a plane which contain principal line and optical axis.
- Oblique photograph: Photograph taken from air with axis of camera tilted from vertical are called oblique photograph, these are of two type
(i) Low Oblique photograph: An oblique photograph that does not show the horizon is called low oblique photograph.
(ii) High Oblique photograph: If tilt is more upto such that horizon is shown in the photograph, it is called high oblique photograph. - Convergent photograph: Low oblique photographs which are taken with two cameras exposed simultaneously at successive exposure stations, with their axes tilted at a fixed inclination from vertical, so that forward exposure of first station from a stereo pair with backward exposure of next station, these photographs are called ‘Convergent Photographs’.
Horizontal and Vertical angles from terrestrial photograph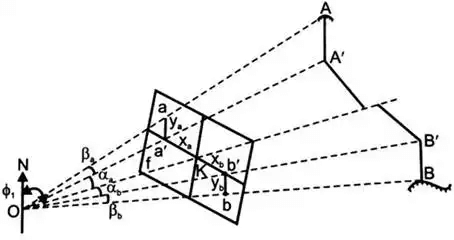
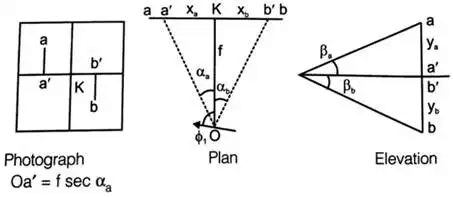
Ob' = fsecαb
Xa = tanαaya = Oa'tanβa = fsecαa = fsecαa.tanβa
Xb = tanbayb = Oa'tanβb = fsecαb = fsecαb.tanβb
tanαa = Xa/f
tanαb =Xb/f
tanβa = ya/fsecαa
tanβb = yb/fsecαb
Angle φ1 is magnetic bearing of camera axis (or principal vertical plane.)
Azimuth of line Ok = φ1
Azimuth of line OA = φ1 - αa (OA is left to OK)
Azimuth of line OB = φ1 - αb (OA is right to OK)
So, Azimuth of a line = Camera azimuth + α
Elevation of a point by photographic measurement
Consider Point A
tanαa = Xa/f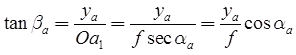
If V = Elevation of point A above Horizontal plane through camera axis.
From Similar triangle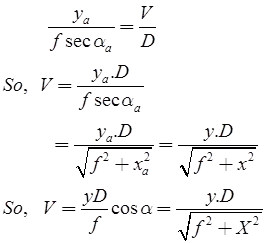
Elevation of point A.
h = HC + V + C
Where, HC = Elevation of camera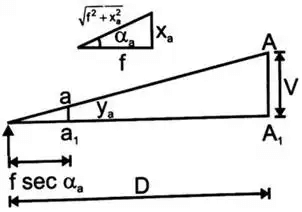
V = Elevation of point A
C = Correction for curvature and refraction.
h = HC + V + C
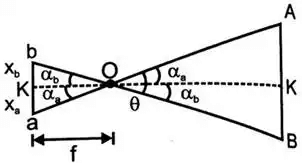
- Determination of focal length of the lens
Take two points A and B. Measure angle θ very accurately from a theodolite
∠AOB = θ
ak = xa, bk = xb
tanαa = xa/f tanαb(xb/f)
tanθ = tan(αa + αb)

f2tanθ = Xa.Xbtanθ - f(Xa-Xb) = 0
Quadratic equation in f.
Arial Photogrammetry
Arial photograph are taken from a fast speed arial camera which have very high speed and efficient shutter, using high speed emulsion for the film.
Important Definitions
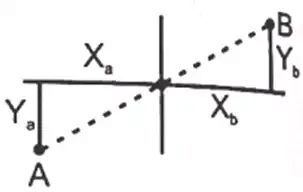
- Vertical photographs: when photograph is taken keeping camera axis vertical, coinciding with the direction of gravity, it is called a vertical photograph.
- Tilted Photograph: camera axis inclined at an angle from vertical.
- Exposure station: Point in space, occupied by camera lens at the time of exposure.
- Flying height: Elevation exposure station above sea level.
- Flight line: Line drawn on the map to represent the track of the aircraft.
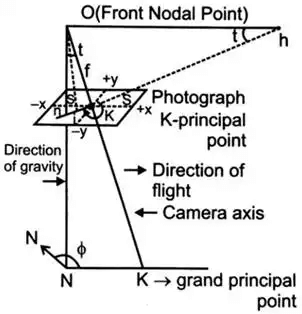
- Focal length: distance from front Nodal point of lens to plane of photograph (OK)
- Principal Point: Point where perpendicular dropped from the front nodal point strikes the photograph (K).
- Nadir Point: Nadir point is point where plumb line intersects photograph.
- Ground Nadir point: Point on the ground vertically beneath the exposure station (Point N).
- Tilt: Vertical angle defined by the intersection at the exposure station. ∠KON = t = tilt
- Principal Plane: Plane defined by lens (O) ground Nadir Point (N) Principal point produced on ground (K).
- Principal Line: Intersection of principal plane with plane of photograph (line NK)
- Isocentre: Isocentre is the point in which the bisector of angle of tilt meets the photograph. Oi is the bisector and I is isocentre – distance ki = f tan1/2.
- Swing: Angle measured in plane of photograph from + y axis clockwise to Nadir point.
- Azimuth of principal plane: Clockwise horizontal angle measured about the ground nadir point from the ground survey north meridian to the principal plane of the photograph.
- Horizon point (h): Intersection of principal line with the horizontal line through the perspective centre. Such as point h in figure is horizon point.
- Axis of Tilt: Axis of tilt is a line in photograph plane perpendicular to principal line at the isocentre such as i1, i i2 in figure. The plane of photograph is tilted about axis.

Relation between principal point, plumb point and isocentre
- NK = distance of nadir point from principal point.
NK / KO = tant ⇒ NK = KO tant = ftant - Ki = distance of the isocentre from principal point.

- Kh = distance of principal point to horizon point.
Kh = KO.cott Kh = fcott
Scale of a Vertical Photograph
Scale varies for points of different elevation, scale will be constant only when elevation of all points are same.
If all the points are on the same elevation.
Then
Scale S = Map distance/Ground distance = ka/KA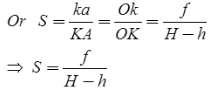
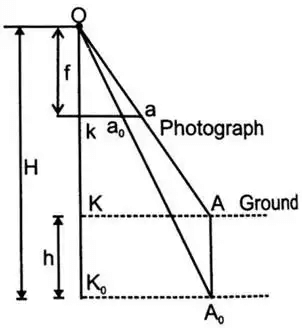
Case 2: If A and B are two points having elevation ha and hb respectively above mean sea level. Scale of photograph at elevation ha.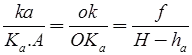
Scale of photograph at elevation hb.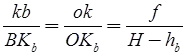
So in general scale of photograph for different elevations (h).
Sh = f/(H-h)
This can be represented by representative fraction (Rn) also.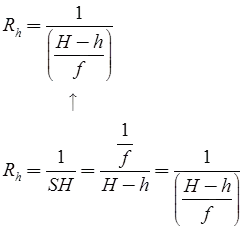
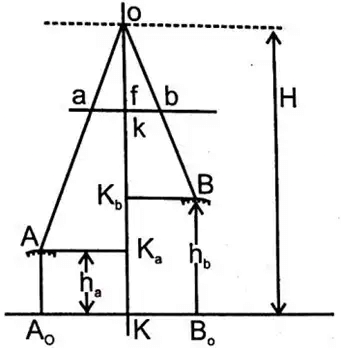
Different Scales
- Datum scale: if all points are projected at mean sea level.
Datum scale = Sd = ka / KAo = ok / OK = f / H
⇒ Sd = f / H - Average scale: If all points are projected on a plane representing the average elevation.
Sav -(f / H - hav)
Computation of length of line between points of different elevation
Coordinate of point A and B on ground in plan.
A - Xa,Ya
B - Xb,Yb
Corresponding points on photograph
A - Xa,Ya
B - Xb,Yb
For point (A) from similar triangles.
For Point B.

So,
So, In general coordinate X and Y of any point.
Length between two points A and B is given by
Xa, Xb, Xa, Xb → should be given with proper sign.
Relief Displacement
Due to different elevation of different points, every point on photograph is displaced from their original position. This displacement is called relief displacement.
r = Ka
ro = Kao
R = KoAo
aa0 is called relief displacement.
aa0 = r - ro
from similar triangle.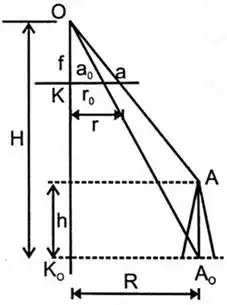
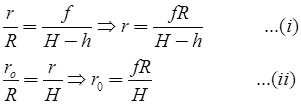
So relief displacement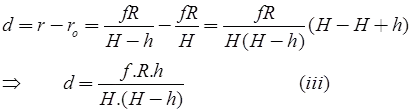
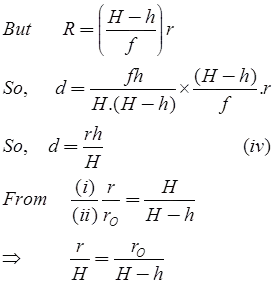
So relief displacement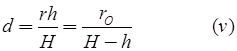
If relief displacement is known then height of an object
h = dH / R
|
19 videos|31 docs|35 tests
|
FAQs on Photogrammetry - Geomatics Engineering (Surveying) - Civil Engineering (CE)
| 1. What is photogrammetric surveying and how is it used in civil engineering? |  |
| 2. What are the advantages of using photogrammetry in civil engineering surveys? |  |
| 3. What are some common applications of photogrammetry in civil engineering? |  |
| 4. What are the key steps involved in a photogrammetric surveying process? |  |
| 5. What are some challenges and limitations of photogrammetric surveying in civil engineering? |  |

|
Explore Courses for Civil Engineering (CE) exam
|

|