Point Set Topology - II | Additional Documents & Tests for IIT JAM PDF Download
Limit Points
Neighbourhood of a Point
Definition : A set S ⊂ R is called a neighbourhood (nbd) of a point p ∈ R if there exists an open interval (p - ε, p + ε) for some ε > 0,
such that
p ∈ ( p - ε, p + ε) ⊂ S.
Examples
1. The set R of real numbers is nbd. of all its points.
For any real number x, we have
x ∈ (x - ε x + ε) ⊂ R, ε > 0.
2. The open interval (a, b) is a nbd. of all its points, since
x ∈ (a + ε, b - ε) ⊂ (a,b)
3. The half open interval (a, b] is a nbd, of all its points except b, since for any ε > 0
b ∈ [b - ε, b + ε ) <z ( a, b]. (∵ b + ε > b)
Similarly, [a, b) is a nbd. of all its points except a and [a, b] is a nbd. of all its points except a and b.
4. The set Z of integers is not a nbd. of any of its points, since for any integer p and any
ε > 0,
p ∈ (p - ε , p + ε ) ⊄ Z.
5. Each of the following open intervals.
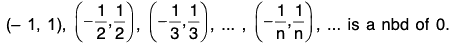
6. For any p ∈ R, N = {p} is not a a nbd. of p, since for any ε > 0, (p - ε , p + ε ) ⊂ N.
7. The set Q of rational numbers is not a nbd. of any of its points, for if (a, b) is any open interval around a point p ∈ Q, then
p ∈ (a, b) ⊂ Z.
since between any two real numbers a, b there is always an irrational number which does not belong to Z.
8. The set R ~ Q of irrational numbers is not a nbd. of any of its points.
9. A non-empty finite set S is not a nbd. of any of its points, if (a, b) is any open interval around any point x ∈ S, then
x ∈ (a, b) ⊄ S,
since (a, b) necessarily contains infinite number of points and S is a finite set.
10. The empty set Ф is a nbd. of each of its points, since there is no point in Ф which is not a nbd.
Example 16: If M is a nbd. of a point p and N ⊃ M, then N is also a nbd. of the point p.
Since M is a nbd. of the point p therefore, there exists an open interval (p - ε, p + ε),
ε > 0 such that
p ∈ (p - ε, p + ε) ∈ M
⇒ P ∈ (p - ε, P + ε) ⊂ M ⊂ N [∵ N ⊃ M ⇒ M ⊂ N]
⇒ P ∈ (p - ε, p + ε) ⊂ N.
Hence N is a nbd. of p.
Example 17: If M and N are nbds. of a point p, then M ∩ N is also a nbd. of p.
Since M and N are nbds. of p, therefore, there exist ε1 > 0 and ε2 > 0 such that
p ∈ (p - ε1, + ε2) ⊂ M,
and p ∈ (ε2 - c2, p + ε2) ⊂ N.Let ε = min {ε1, ε2} so that ε < ε1, and ε < ε2. Thus
....(1)
∴ p ∈ (p - ε, p + ε) ⊂ M ∩ N.
Hence M ∩ N is a nbd of p.
Note. Obviously, p ∈ (p - ε, p + ε) ⊂ M υ N and so M υ N is also a nbd. of p.
Example 18: Show that intersection of a finite number of neighbourhoods of a point is also a neighbourhood of the point.
Let M1, M2,Mn be a finite number of neighbourhoods of a point p. Then there exists εi > 0 such that
p ∈ ] p - εi, p + εi [ ⊂ Mi for i = 1, 2,...., n.
Let ε = min {ε1, ε2, .... εn. Then
p ∈ ] p - ε, p + ε [ ⊂ Mi for i = 1, 2, n
⇒ p ∈ ] p - ε, p + ε [ c M1, ∩ M2 ∩ ... ∩ Mn.
Hence M1 ∩ M2 ∩ ... ∩ Mn is a nbd. of p.
Example 19: Define a neighbourhood of a point. Show that the intersection of the family of all neighbourhoods of a point x is {x}.
A subset S ⊂ R is called a neighbourhood of a point p ∈ R if there exists some ε > 0 such that ( p - ε, p + ε ) ⊂ S,
Let {Nx} be a family of all neighbourhoods of a point x. We have to show that ∩ Nx = {x}.
Consider any point y ≠ x and Let ε = I x - y |
then, (x - ε, x + ε) is a nbd. of x and y ∉ (x - ε, x + ε),
since y ∈ (x - ε, x + ε) ⇒ x- ε < y < x + ε ⇒ lx-y| < ε, a contradiction.
Since y ∉ (x - ε, x + e), y ∉ n Nx. Hence n Nx = {x}.
Example 20: (i) Can a set N be a neighbourhood of any point of R ~ N ?
(ii) Can a non-empty finite set be a neighbourhood of any of its points ?
(i) We shall show that N cannot be a neighbourhood of any point of R ~ N.
Let x ∈ R ~ N then, x ∉ N.
Suppose N is a nbd. of x then there exists some c > 0 such that
x ∈ (x - ε, x + ε) ⊂ N ⇒ x ∉ N, which is a contradiction.Hence the assertion made above is not TRUE.
(ii) The answer of the second part is also No.
Let x ∈ N, where N is a non-empty finite set. If N is nbd. of x, then
x ∈ (x - ε, x + ε) ⊂ N for some ε > 0.This is impossible, since N is a finite set and (x - ε, x + ε) contains infinite number of elements.
Limit Points
Definition 1 : A real number p is said to be a limit point of a set S ⊂ R if for each ε > 0 there exists at least one point p1 ∈ S such that
P1 ∈ (p - ε, p + ε) and p1 ≠ p.
Definition 2 : The set of all limit points of a set S ⊂ R is called the derived set of S and is denoted as S’.
x ∈ S' ⇔ x is a limit point of S.
Example
1. 0 is the only limit point of the set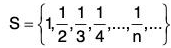
2. 1 and -1 are the only limit points of the set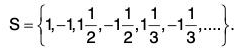
Notice that 1, -1 both belong to S and S’ = {1, -1}.
Remarks
- A set may or may not have a limit point.
For Example, R has limit points but Z has no limit point. - Limit point of a set may or may not belong to the set.
For Example, every limit point of R belongs to R and the only limit point 0 of the set does not belong to the set i.e., 0 ∉ S’.
does not belong to the set i.e., 0 ∉ S’.
Examples :
1. The set I has no limit point for a nbd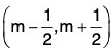 of m ∈ 1 contains no Point I other than m. Thus the derived set of I is the null set ∅.
of m ∈ 1 contains no Point I other than m. Thus the derived set of I is the null set ∅.
2. Every point of R is a limit point for every nbd of any of its points contains an infinity of members of R. Therefore, R’ = R.
Examples: Find the limit points of each of the following sets :
(a)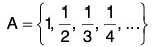
(b)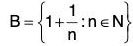
(c)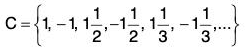
(d) D = {x : 0 < x < 1}
(e)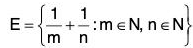
(f) F = {3-n + 5-n : n ∈ N}
(a) The only limit point of A is 0 i.e., A’ = {0}. Notice that 0 ∉ A.
(b) We have B = 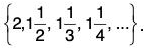
The only limit point of B is 1 i.e., B’ = {1}. Notice that 1 ∉ B.
(c) The set C = 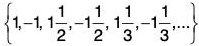 has only two limit points 1,-1. Notice that 1 e C and -1 e C. We have C' = {1, -1}.
has only two limit points 1,-1. Notice that 1 e C and -1 e C. We have C' = {1, -1}.
(d) We observe that D = [0, 1) and so D' = [0, 1].
(e) First we write all the elements of the set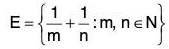
For m = 1, the elements of E are :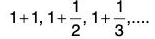 ...(1)
...(1)
For m = 2, the elements of E are :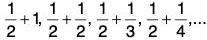 ...(2)
...(2)
For m = 3, the elements of E are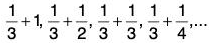 ...(3)
...(3)
and so on.
It is clear that
1 is the limit point of the elements in row (1),
1/2 s the limit point of the elements is row (2),
1/3 is the limit point of the elements in row (3),
and so on.
Thus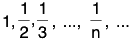 ... are all limit points of E.
... are all limit points of E.
Since E contains an infinite number of fractions lying between 0 and 1, so each nbd. of 0 surely contains a positive fraction and as such 0 is also a limit point of E.
Hence 
(f) We have 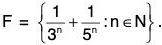
As discussed in part (e), we observe that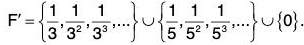
Example. Give an example of a set whose derived set is
(i) void
Ans. If Z is the set of integers, then Z' = Ф
(ii) subset of the given set
Ans. Let S = 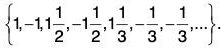
Then S’ = { 1 , - 1 } ⊂ S.
(iii) superset of the given set
Ans. Let S =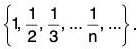 Then S’ = {0}.
Then S’ = {0}.
S’ is neither a subset nor a superset of S.
(iv) same as the given set
Ans. R' = R or [a, b]’ = [a, b].
Example : Give an example of a set which has
(i) no limit point
Ans. The set Z of integers has no limit point.
(ii) unique limit point
Ans. 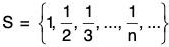 has 0 as the unique limit point.
has 0 as the unique limit point.
(iii) two limit points
Ans.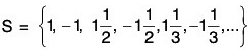
has two limit points viz 1 and -1.
(iv) three limit points
Ans.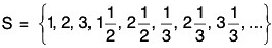 has three limit points viz. 1, 2, 3
has three limit points viz. 1, 2, 3
(v) infinite number of limit points
Ans. The set R of real numbers has infinite number of limit points. In fact every real number is a limit point of R.
(vi) a set whose every point is a limit points of the set
Ans. The set R of real numbers.
(vii) a set none of whose points is a limit point of the set
Ans. The set Z of integers.
(viii) A set with only √3 as its limit point.
Ans. S = 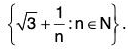
Example 21: Define limit point of a set. Show that the set N of natural numbers has no limit point.
Let m be any natural number.
Thenis a nbd. of m which does not contain any point of N different from m.
Let m ∈ R ~ N i.e., m is any real number but not a natural number. Then we can find some integer j such that j < m < j + 1. Consequently (j, j + 1) is a nbd. of m, which does not contain any point of N. Hence N has no limit point i.e., N' = Ф.
Example 22: Given an example each of :
(i) a bounded set of real numbers
(ii) an unbounded set of real numbers
Also find the set of limit points of both the sets.
(i)
is a bounded set of real numbers. Its limit points are 1 and -1.
(ii)
is an unbounded set of real numbers. 0 is the only limit point of S.
Example 23: Find the derived sets of the following :
(i) 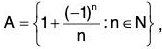
(ii)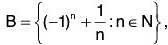
(iii)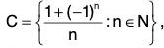
(iv)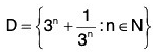
A’ = {1}, B’ = {-1, 1}, C’ = {0}, D’ = ϕ
Example 24: If S and T are sets of real numbers, then show that
(a) S ⊂ T ⇒ S’ ⊂ T’
(b) (S ∪ T)’ = S’ ∪ T’
(c) (S ∩ T)’ ⊂ S’ ∩ T’
Given an example to show that (S ∩ T)’ and S’ ∩ T’ may not be equal.
(a) Let S ⊂ T. We shall prove that S’ ⊂ T’.
Let x ∈ S’ so that x is a limit point of S. Thus for each ε > 0, (x - ε, x + ε) contains a point x, ∈ S such that x1 ≠ x. Since S ⊂ T, x1 ∈ S ⇒ x1 ∈ T.
Consequently, (x - ε, x + ε) contains a point x1 ∈ T, x1 ≠ x, and so x is a limit point of T
i.e., x ∈ T’.
∴ x ∈ S' ⇒ x ∈ T’.Hence S ’ ⊂ T
(b) We know S ⊂ S ∪ T S’ ⊂ (S ∪ T),, by part (a).Similarly, T ⊂ S ∪ T ⇒ T ’ ⊂ (S ∪ T)’.
S’ u T ' ⊂ (S ∪ T) ’ ...(1)
∴ Now we prove (S ∪ T)’ ⊂ S’ υ T’. ...(2)
Let x ∈ (S ∪ T)' ⇒ x is a limit point of S ∪ T
⇒ every nbd. of x contains a point y ∈ S ∪ T, y ≠ x
⇒ every nbd. of x contains a point (y ∈ S or y ∈ T), y ≠ x
⇒ every nbd. of x contains a point y ∈ S, y ≠ x,
or every nbd. of x contains a point y ∈ T, y ≠ x.
⇒ x is a limit point of S or x is a limit point of T
⇒ x ∈ S’ or x ∈ T’⇒ x ∈ S’ ∪ T'.
Thus (2) is proved.
From (1) and (2), (S ∪ T)' = S’ υ T.
(c) We know S ∩ T ⊂ S ⇒ (S ∩ T)' c S’, by part (a)
and S ∩ T ⊂ T ⇒ (S ∩ T)’ ⊂ T.
(S ∩ T)’ ≠ S’ ∩ T,.
To prove that the equality does not hold, we take
S = ]1, 2[ and T = ]2, 3[ so that S ∩ T = ϕ.
⇒ S’ = [1, 2], T = [2, 3] and
(S ∩ T)’ = ϕ' = ϕ).
Now S’ ∩ T' = {2} and (S ∩ T)’ = ϕ.
Thus (S ∩ T)' ≠ S’ ∩ T.
Some Theorems of Limit Points
Theorem : A point p is a limit point of a set A if and only if every neighbourhood of p contains infinitely many points of A.
Proof. The condition is necessary.
Let p be a limit point of A. We shall show that every nbd. of p contains infinitely many points of A. Suppose this is false then, there exists some ε > 0 such that (p - c, p + e) contains only a finite number of points, say pt, p2, pn ; which are different from p.
Let δ = min {Ip - p1 I , I p - p2 I ,..., I p — pn I } (δ > 0)
⇒ δ < I p - Pi I for i = 1, 2 ..., n
⇒ (p - δ, p + δ) contains no point of A other than p
⇒ p is not a limit point of A, which is a contradiction.
Hence every nbd. of p must contain infinitely many points of A.
The condition is sufficient.
Suppose every nbd. of p contains infinitely many points of A. Surely then, every nbd. of p contains a point of A different from p and so p is a limit point of A.
Corollary. Show that a finite set has no limit points.
Proof. Let S be a finite set. Let if possible p be a limit point of S.
By Theorem for every ε > 0, (p - c, p + c) must contain an infinite number of elements of S, which is impossible, as S is a finite set. Hence p cannot be a limit point of S.
Theorem : (Bolzano-Weierstrass Theorem)
Every infinite bounded set of real numbers has a limit point.
Proof. Let S be any infinite bounded set of real numbers with bounds
m = inf S and M = sup S i.e., m < x < M ∀ x∈S. ...(1)
We define a set of real numbers as follows :
T = {x : x Exceeds only a finite number of elements of S}. ...(2)
From (2), it follows that
⇒ x g S’ u T'.
Thus (2) is proved.
From (1) and (2), (S ∪ T)' = S’ ∪ T'
(c) We know S ∩ T ⊂ S ⇒ (S ∩ T)' ⊂ S’, by part (a)
and S ∩ T ⊂ T ⇒ (S ∩ T)’ ⊂ T.
(S ∩ T)’ ⊂ S’ ∩ T,.
To prove that the equality does not hold, we take
S = ]1, 2[ and T = ]2, 3[ so that S ∩ T = ϕ.
⇒ S’ = [1, 2], T = [2, 3] and
(S ∩ T)’ = ϕ, = ϕ.
Now S’ ∩ T' = {2} and (S ∩ T)’ = ϕ.
Thus (S ∩ T)' ≠ S’ ∩ T.
Some Theorems of Limit Points
Theorem : A point p is a limit point of a set A if and only if every neighbourhood of p contains infinitely many points of A.
Proof. The condition is necessary.
Let p be a limit point of A. We shall show that every nbd. of p contains infinitely many points of A. Suppose this is false then, there exists some ε > 0 such that (p - ε, p + ε) contains only a finite number of points, say p1, p2, pn ; which are different from p.
Let δ = min { I p - p1 I , I p - p2 I,... , I p - pn I } (δ > 0)
⇒ δ < I p - Pi I for i = 1, 2 ...., n
⇒ (p - δ, p + δ) contains no point of A other than p
⇒ p is not a limit point of A, which is a contradiction.
Hence every nbd. of p must contain infinitely many points of A.
The condition is sufficient.
Suppose every nbd. of p contains infinitely many points of A. Surely then, every nbd. of p contains a point of A different from p and so p is a limit point of A.
Corollary. Show that a finite set has no limit points.
Proof. Let S be a finite set. Let if possible p be a limit point of S.
By Theorem for every ε > 0, (p - ε, p + ε) must contain an infinite number of elements of S, which is impossible, as S is a finite set. Hence p cannot be a limit point of S.
Theorem : (Bolzano-Weierstrass Theorem)
Every infinite bounded set of real numbers has a limit point.
Proof. Let S be any infinite bounded set of real numbers with bounds
m = inf S and M = sup S i.e., m < x < M ∀ x∈S. ...(1)
We define a set of real numbers as follows :
T = {x : x Exceeds only a finite number of elements of S}. ...(2)
From (2), it follows that
From (1) and (4), we observe that m ∈ T and so T is non-empty.
Again from (1), M > x ∀ x ∈ S (and S is infinite).
From (3), ∀ y ∈ T
⇒ y > only a finite number of elements of S.
Thus M > y ∀ y ∈ T and so M is an upper bound of T i.e., T is bounded above. Since T is a nonempty and bounded above subset of R, so by order-completeness property of R, T must have the supremum, say p i.e.,
sup T = p.
We shall show that p is a limit point of S. Let ε > 0 be arbitrary.
Since p + ε > p and p is an upper bound to T, p + ε ∉ T
⇒ p + ε > infinite number of elements of S, using (3)
⇒ infinite number of elements of S < p + ε. ...(5)
Since p - ε < p and p is the l.u.b. of T, so p - ε cannot be an upper bound of T. Consequently, there exists some q ∈ T such that
q > p - ε, i.e., p - ε < q, where q ∈ T.
Now q e T ⇒ q < infinite number of elements of S, by (4).
Thus p - ε < q < infinite number of element s of S. ...(6)
From (5) and (6), it follows that any nbd. (p - ε, p + ε) of p contains infinite number of elements of S. Hence p must be a limit point of S.
Remark : The conditions of the Bolzano-Weierstrass Theorem cannot be relaxed.
We have seen in the corollary of Theorem that a finite set (which is of course bounded) can never have a limit point. Further there exist infinite unbounded sets having no limit point.
For Example,
(i) The set Z of integers is an infinite unbounded set, which has no limit point.
(ii) The set S = {12, 22, 32, ... n2, ...}
is an infinite unbounded set, which has no limit point.
Remark : It may, however, be noticed that
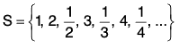
is an infinite unbounded set, which has the limit point viz. 0.
Theorem 1 : Every finite set is bounded.
Proof : Let A = {a1, a2, .... an} be a finite set and
let h = min(a1, a2, .... an) and k = max(a1, a2, ..., an)
then h < x < k, ∀ x ∈ A
Therefore A is bounded.
Theorem 2 : Every infinite bounded set of real numbers has a limit point.
Proof : Every infinite and bounded subset of R has a limit point i.e. atleast one limit point.
The Archimedean Property Of Real Numbers
If x, y are two positive real numbers, then there exists a positive integer n such that ny > x.
Proof : Suppose there does not exist any positive integer n such that ny > x. Then ny < x ∀ n ∈ N. Consequently, the set
S = {y, 2y, 3y ny, (n + 1) y, ...}
is non-empty and bounded above by x.
By order-completeness property, S has the supremum, say p.
∴ (n + 1) y < p ∀ n ∈ N
⇒ ny < p - y ∀ n ∈ N, (p - y < p, as y > 0)
⇒ p - y (< p) is an upper bound of S, which is a contradiction to the fact that p = sup S.
Corollary 1 : If x be any positive real number, then there exists a positive integer n such that n>x.
Corollary 2 : Let x by any real number and y be a positive real number, then there exists a positive integer n such that ny > x.
Corollary 3 : For any real number x, there exists a positive integer n such that n > x.
Corollary 4 : For any real number x, there exists an integer m such that m < x.
Example 25: If x be any positive real number, then there exists a positive integer n such that 1/x < x.
Applying the Archimedean property, for y = x > 0 and x = 1, there exists a positive integer n such that ny > x nx > 1 ⇒ n > 1/x. Hence 1/n < x.
Remark : The integer n satisfying n < x < n + 1 is called an integral part of x and is denoted by [x].
Example 26: If x and y are real numbers and x > 1, prove that there exists a positive n such that xn > y.
Suppose that conclusion of the problem is false.
Then xn < y ∀ n ∈ N. Consequently, the set
S = {x, x2, x3, ..., xn, ...}is non-empty subset of R, which is bounded above by y. By order-completeness property of R, S has the l.u.b. Let p = l.u.b. S. ...(1)
Since x > 1, px > p ⇒ p > p/x ⇒ p/x < p
⇒ p/x is not an upper bound of S, by (1) and so there exists some xn ∈ S such that
xn > p/x ⇒ xn +1 > p, where xn +1 ∈ S.Thus shows that p is not an upper bound of S, which is a contradiction.
Hence xn > y for some positive integer n.
Intervals
The representation of real numbers as points on a straight line helps us to define four types of intervals.
Let a and b be any two real numbers such that a < b.
1. Open Interval, denoted by ]a, b[, is defined as
(a, b) = {x : a < x < b}.
The end points a and b do not belong to (a, b).
2. Closed Interval, denoted by [a, b], is defined as
[a, b] = {x : a < x < b}.
The end points a and b belong to [a, b].
Semi-Open or Semi-Closed Intervals are defined as follow :
3. (a, b] = {x : a < x < b}.
Here a ∉ (a, b] and b ∈ (a, b].
The interval ]a, b] is said to be open from the left and closed from the right.
4. [a, b) = {x : a < x < b}.
Here a ∈ [a, b) and b ∉ [a, b).
The interval [a, b) is said to be open from the right and closed from the left. The intervals (a, b] and [a, b) are also known as half-open or half-closed intervals.
Open And Closed Sets
Open Sets
Definition : A set S ⊂ R is called an open set if it is a nbd of each of its points.
In Other Words : a set S ⊂ R is called an open set if for each p ∈ S, there exists some ε >
0 such that
p ∈ (p - ε, p + ε) ⊂ S ∀ p ∈ S.
Examples
1. The set R of real numbers is an open set.
2. Every open interval (a, b) is an open set.
3. [a, b) is not an open set, since [a, b) is a nbd. of all is points except a.
Similarly, (a, b], [a, b] are not open sets.
4. The set Z is integers is not an open set,
since Z is not a nbd. of each of its points.
5. G = (1, 2) ∪ (3, 4) is an open set, as G is a nbd. of each of its points.
6.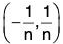 is an open set for each n e N.
is an open set for each n e N.
7. The empty set ϕ is an open set.
8. The singleton set {x} is not an open set, since for any ε > 0, (x - ε, x + ε) ⊄ {x}
9. The set Q of rational numbers is not an open set, since Q is not a nbd. of each of its points.
10. The set R - Q of all irrational numbers is not an open set.
11. The set S = is not an open set, as S is not a nbd. of each of its points.
For Example, (1 - ε, 1 + ε) is not an open set, as S is not a nbd. of each of its points.
For Example, (1 - ε, 1 + ε) contains an infinite number of irrational numbers and so (1 - e, 1 + ε) ⊂ S for each ε > 0.
Theorem : The union of an arbitrary family of open sets is an open set.
Proof. Let {GI : λ ∈ A} be an arbitrary family of open set.
Let 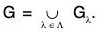 . We have to show that G is an open set.
. We have to show that G is an open set.
Let x be any element of G = ∪ Gλ
Then x ∈ Gλ for some X ∈ A.
Since Gλ is given to be an open set and x ∈ Gλ so Gλ is a nbd. of x. Thus there exist some ε > 0 such that
x ∈ (x - ε, x + ε) ⊂ Gλ
⇒ x ∈ (x - ε, x + ε) ⊂ Gλ ⊂ 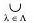 Gλ = G
Gλ = G
⇒ x ∈ (x - ε, x + ε) ⊂ G ∀ x ∈ G
⇒ G is a nbd. of x, ∀ x ∈ G
It follows that G is a nbd. of each of its points and consequently G = Gλ. is an open set.
Theorem : The intersection of a finite number of open sets is an open set.
Proof. First of all we show that the intersection of two open sets G1 and G2 is an open set. Let x be any point of Gt n G2. Then x ∈ G1 ∩ G2
⇒ x ∈ G1 and x ∈ G2
⇒ G1 and G2 are nbds. of x (∵ G1 and G2 are open sets)
⇒ G1 ∩ G2 is nbd. of x
⇒ G1 ∩ G2 is a nbd. of x for each x ∈ G1 ∈ G2.
Thus G1 ∩ G2 is an open set.
Now we consider three open sets G1,G2, G3. Then
G1 ∩ G2 n G3 = (G1 ∩ G2) ∩ G3.
R.H.S. being the intersection of two open sets viz. G1 ∩ G2 and G3 is an open set and so G1 ∩ G2 ∩....∩ G3 is an open set.
Proceeding in a similar way, if G1, G2......, Gn are a finite number of open sets, then Gt n G2 ∩ ... ∩ Gn is an open set.
Remark : The intersection of an arbitrary family of open sets may not be an open set.
Let us consider Gn = ( - 1/n, 1/n ) ∀ n ∈ N. Then
G1 = ( - 1, 1), G2 = (- 1/2, 1/2 ), G3 = (- 1/3, 1/3), ...
Since every open interval is an open set, therefore {Gn : n ∈ N} is a family of infinite number of open sets.
Clearly, G1 ∩ G2 = (- 1, 1) ∩ (- 1/2, 1/2) = (- 1/2, 1/2),
G1 ∩ G2 ∩ G3 = (- 1/2, 1/2) ∩ ( - 1/3, 1/3) = (- 1/3, 1/3)
and so on. Thus at every step, the size of the open interval is reducing and getting closer to 0.
Indeed 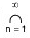 Gn = {0} which is not an open set,
Gn = {0} which is not an open set,
}since for any ε > 0, (0 - ε, 0 + ε) = (- ε, ε) ⊄ {0}.
Example : Let 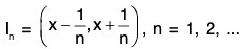
Prove that ln is an open set for each positive integer n and 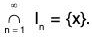
Hint. Since each open interval is an open set, (x - 1/n, x + 1/n) is an open set for n = 1, 2, 3,
... As shown above 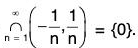
Similarly,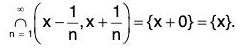
Example : Is every infinite set open? Justify your answer.
Hint. Every infinite set need not be an open set.
For Example,
(i) the set Z of integers is an infinite set, which is not an open set.
(ii) 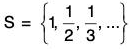 is an infinite set, which is not an open set.
is an infinite set, which is not an open set.
Definition : A set S ⊂ R is called a closed set if and only if its complement R ~ S is an open set.
S is closed ⇔ R ~ S is open.
Examples
1. The set R of real numbers is a closed set,
as R ~ R = ϕ is an open set.
2. The empty set ϕ is a closed set,
as R ~ ϕ = R is open set.
3. The set R of real numbers is both open and closed.
The empty set ϕ is both open and closed.
4. Every closed interval [a, b] is a closed set,
∵ R ~ [a, b] = (- ∞, a) ∪ (b, + ∞)
and R.H.S. being the union of two open sets is an open set.
5. [a, b) is not a closed set,
R ~ [a, b) = ( - ∞, a) ∪ [b, + ∞)
is not an open set as the R.H.S. is a nbd. of each of its points except b.
Similarly, (a, b], (a, b) are not closed sets.
6. [0, 3] ∩ [1, 2] is a closed set,
since [0, 3] ∩ [1, 2] = [1, 2], which is a closed set.
7. F = [1, 2] ∪ [2, 4] is a closed set, since F = [1, 4] is a closed set.
8. Q is not a closed set, since R ~ Q being the set of all irrational numbers is not an open
set. Thus the set Q of rational numbers is neither a closed set nor an open set.
Theorem : (a) The union of a finite number of closed set is a closed set.
(b) The intersection of an arbitrary family of closed sets is a closed set.
(c) Given an Example to show that an arbitrary union of closed sets may not be a closed set.
Proof, (a) Let F1, F2, ..., Fn be a finite number of closed sets so that R ~ F1, R ~ F2, ..., R ~ Fn are open sets.
(R ~ F1) n (R ~ F2) ∩ ... ∩ (R ~ Fn) is an open set.
By De Morgan’s law,
R~(F1u F2 ∪ ... ∪ Fn) = (R ~ F1) n (R ~ F2) ∩ ... ∩ (R ~ Fn)
⇒ R ~ (F1 ∪ F2 ∪ ... ∪ Fn) is an open set.
Hence F1 u F2 ∪ ... ∪ Fn a closed set.
(b) Let {Fλ : X ∈ ∧} be an arbitrary family of closed sets.
We have to prove that 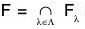 is a closed set.
is a closed set.
Equivalently, we have to prove that R ~ F is an open set.
By De Morgan's law,
R ~ F = R ~ (∩ Fλ) = ∪ (R ~ Fλ).
Since each Fλ is a closed set, so R ~ Fλ is an open set.
Now ∪ (R ~ Fλ) being an arbitrary union of open sets is an open set and so R ~ F is an open set. Hence F = ∩ Fλ is a closed set.
(c) 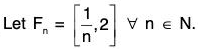
Then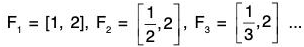
Since every closed interval is a closed set, therefore {Fn : n ∈ N} is a family of infinite number of closed sets. We observe that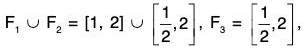
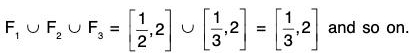
Indeed, 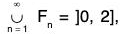 which is not a closed set.
which is not a closed set.
Example : Given an example of each of the following :
(a) an interval which is an open set.,
Ans. (a, b).
(b) an interval which is not an open set,
Ans. [a, b].
(c) an open set which is not an interval,
Ans. R or (0, 1) u (2, 3).
(d) a set which is neither an interval nor an open set.
Ans. The set Z of integers.
Example : Give an example of each of the following :
(a) an interval which is a closed set,
Ans. [a, b].
(b) an interval which is not a closed set,
Ans. [a, b).
(c) a closed set which is not an interval,
Ans. R or [0, 1] υ [2, 3].
(d) a set which is neither an interval nor a closed set.
Ans. The set Z of integers.
(e) A set which is neither an open set nor a closed set.
Ans. The set Q of all rational numbers is neither an open set nor a closed set. Example : Comment on the following statements :
(a) Can a finite set be open?
Ans. Yes. An empty set is a finite set, which is also an open set.
(b) Can a finite non-empty set be open?
Ans. No, Let S is a non-empty finite open set, then for any x ∈ S and any ε > 0
(x - ε, x + ε) ⊄ S,
since the interval (x - ε, x + ε) necessarily contains an infinite number of points and S is a finite set.
(c) Is every infinite set open?
Ans. No. The set Z of integers is an infinite set, which is not an open set.
(d) Is the intersection of any arbitrary family of open sets an open set?
Ans. No. Gn = ( - 1/n, 1/n) ∀ n ∈ N is a family of infinite number of open sets and 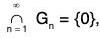 which is not an open set,
which is not an open set,
Theorem : A set S is closed if and only if it contains all its limit points.
Equivalently, S is closed o S' ⊂ S.
Proof : By definition of a closed set,
S is closed ⇔ R ~ S is an open set
⇔ R ~ S is a nbd. of each of its points
⇔ for each x ∈ R ~ S, ∃ some c > 0, such that
(x - ε, x + ε) ⊂ R ~ S or (x - ε, x + ε) ∩ S = ϕ
(∵ y ∈ (x - ε, x + ε) ⇒ y ∈ R ~ S ⇒ y ∉ S)
⇔ for each x ∈ R ~ S, ∃ a nbd. of x which contains no point of S
⇔ x is not a limit point of S ∀ x ∈ R ~ S.
⇔ x ∉ S’ ∀ x ∈ R ~ S
⇔ x ∉ S' ∀ x ∉ S
⇔ S' ⊂ S.
Remark. The above Theorem gives us a second definition of a closed set.
In order to show that a set S is closed, we must prove that if p is any limit point of S. then p ∈ S.
Corollary 1. Every finite set S is a closed set.
Proof. Since S is a finite set, S has not limit points. .\ S’ = ϕ.
Since ϕ ⊂ S. so S’ ⊂ S. Hence S is a closed set.
Corollary 2. Every singleton is a closed set.
Proof. Any singleton set {x} is a finite set and hence by Corollary 1, it is a closed set. Corollary 3. The set Z of integers is a closed set.
Proof. We know Z‘ = ϕ and so Z’ ⊂ Z. Hence Z must be a closed set.
Corollary 4. The set N of natural numbers is a closed set.
Proof. We know N' = ϕ and so N' ⊂ N. Hence N is a closed set.
Theorem. Prove that the supremum of a non-empty bounded set is either the greatest member of the set or is a limit point of the set.
Or
Prove that the supremum of a non-empty bounded set S of real numbers when not a member of S must be a limit point of S.
Proof. Since S is non-empty and bounded above, S must have the supremum, say s i.e., s = sup S (O-C Property).
⇒ x < s ∀ x ∈ S.
If s < S, then s is the greatest member of S. Let s ∉ S.
We shall show that s is a limit point of S.
For any ε > 0, we have s - ε < s. ...(1)
Since s is the l.u.b. of S, so s - ε cannot be an upper bound of S. Consequently, there exists some t ∈ S such that
t < s < s + ε ⇒ t < s + ε. ...(2)
From (1) and (2), it follows that any nbd. (s - ε, s + ε) of s contains a point t ∈ S, t ≠ s. Hence s is a limit point of S.
Theorem : Show that a non-empty, bounded and closed set S contains its supremum and infimum.
Proof : Since S is non-empty and bounded above S must have a supremum say s
i.e., s = sup S (O-C property). If we show that s ∈ S, then S has a maximum viz. s. We now proceed to show that s is a limit point of S.
Let ε > 0 be arbitrary. Since s = l.u.b.S. s - ε is not an upper bound of S and so there exists some t ∈ S such that
t > s - ε or s - ε < t ...(1)
Clearly, t < s < s + ε ...(2)
From (1) and (2), it follows that (s - ε, s + ε) contains a point t ∈ S, t ≠ s for all ε > 0.
Thus s is a limit point of S. Since S is a closed set, s ∈ S.
Hence s is a maximum of S. Similarly, s’ = inf S is a minimum of S.
Theorem : Prove that the derived set of any set is a closed set.
Proof : Let S’ be the derived set of a set S. We have to show that S’ is a closed set. Equivalently, we have to show that if p is limit point of S’, then p ⇐ S’.
Suppose p is a limit point of S’. The for any ε > 0, (p - ε, p + ε) contains a point q of S’, q ≠ p. Now q ∈ S’ ⇒ q is a limit point of S.
⇒ Every nbd. of q (in particular, (p - ε, p + ε) ) contains a point r of S, r ≠ q
(∵ r ≠ q and q ≠ p)
⇒ for each ε > 0, (p - ε, p + ε) contains a point r e S, where r ≠ p
⇒ p is a limit point os S p ∈ S’. Hence S’ is closed set.
Theorem : The derived set of a bounded set is bounded.
Proof : Let S be any bounded set whose bounds are
m = inf S and M = sup S
i.e., m < x < M ∀ x ∈ S ...(1)
We shall prove that
m < x < M ∀ x ∈ S’
Let, if possible, there exist some y ∈ S' such that y > M.
Now, y > M ⇒ y - M > 0.
We choose ε > 0 such that
ε < y - M ⇒ M < y - ε ...(2)
From (1) and (2),
x < x ∀ x ∈ S.
In follows that there exists a nbd. ( y - ε, y + ε ) of y which does not contain any point of S and so y in not a limit point of S i.e., y ∉ S’, which is contradiction.
Thus x < M ∀ x ∈ S’.
Similarly, we can show that
m < x ∀ x ∈ S’
Hence m < x < M ∀ x ∈ S' and so S’ is bounded.
Example 27: Show that S =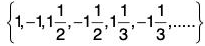 is closed but not open.
is closed but not open.
The only limit points of the set S are 1 and - 1 i.e., S’ = {1, - 1}.
Since S' ⊂ S, S is a closed set.Since S is not a nbd. of each of its points, S is not an open set.
Notice the for each ε > 0, 1 ∈ (1 - ε, 1 + ε) ⊄ S.
Example : Comment on the following statements :
(a) Can a singleton be closed?
Ans. Yes. Every singleton is closed
(b) Can a finite set be closed?
Ans. Yes. Every finite set is closed
(c) Is every finite set closed?
Ans. Yes.
(d) Is every infinite set closed?
Ans. No. S = 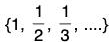 is an infinite set which is not closed, since its only limit point 0 does not belong to the set S.
is an infinite set which is not closed, since its only limit point 0 does not belong to the set S.
(e) Is the union of an arbitrary family of closed sets a closed set?
Ans. No. {Fn = [1/n, 2] : n ∈ N} is a family of infinite number of closed sets, and which is not a closed set.
(f) Is the intersection of an arbitrary family of closed sets a closed set?
Ans. Yes.
Example 28: Give Examples of sets S and S’ such that
(a) S ∩ S ’ = ϕ, ( b ) S ⊂ S ’ , (c) S ’ ⊂ S, (d) S = S ’ .
(a) Let S =
Then S’ = {0}. Clearly, S ∩ S’ = ϕ
(b) Let S = ( 0, 1 ) so that S’ = [0, 1]. Obviously, S ⊂ S’.
(c) Let S =Then S’ = {1, - 1} c S.
(d) Let S = [0,1] so that S’ = [0,1]. We have S = S’.
Example 29: Find the limit points of the following :
(i) N, (ii) (a, b], (iii) R ~ Q, (iv) finite set.
(i) N has no limit point.
(ii) {(a, b]}’ = [a,b]. Hence each point of [a, b] is a limit point of (a, b].
(iii) R ~ Q, consists of all irrational numbers. Every real number p ∈ R is a limit point of R ~ Q, since for each ε > 0, (p - ε, p + ε) contains an irrational number other than p.
(iv) A finite set has no limit point .
Example : State whether the following subsets of R are open, closed :
(i) the set Z of all integers.
Ans. Z is closed, (∵ Z = ϕ ⊂ Z.)
However, Z is not open as Z is not a nbd. of any of its points.
(ii)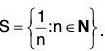
Ans. S is neither closed nor open.
We have S’ = {0} ⊄ S. Also S is not a nbd. of any of its points.
(iii) The segment (a, b).
Ans. It is an open set, since it is an open interval.
However, it is not a closed set, since { (a, b) }' = [a, b] ⊄ (a, b).
(iv) The set N of natural numbers.
Ans. N is closed. (v N’ = ϕ ⊂ N)
However, N is not open as N is not a nbd. of any of its points.
(v) S ={1, 2, 3, 4}.
Ans. S is a closed set, since it is a finite set and every finite set is closed. However, S is not open, since S is not a nbd. of any of its points.
(vi) The set R of real numbers.
Ans. R is both open and closed.
(vii) S = (0, 1) u (2, 3).
Ans. S being the union of two open sets is an open set.
Now S’ = { (0, 1) }’ u { (2, 3) }’ = [0, 1] u [2, 3] cz S.
Hence. S is not a closed set.
(viii)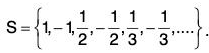
Ans. S’ = {0} ⊄ S and so S is not closed.
Since S is not a nbd. of each of its points, S is not open.
(ix) The set Q as rational numbers.
Ans. We know Q’ = R ⊄ Q and so Q is not closed.
Further Q is not a nbd. of any of its points and so Q is not open.
(x) S = [0, 1] ∪ [2, 3].
Ans. S being the union of two closed sets is a closed set (Every closed interval is a closed set). Since S is not a nbd. of the points 0, 1,2, and 3, S is not an open set.
Example 30: It F is a closed bounded set, then every infinite subset S of F has limit point in F.
Since S is a subset of the bounded set F, so S is a bounded and infinite set. By Bolzano Weierstrass Theorem, S has a limit point, say p. Since S ⊂ F, so p is also a limit point of F. Since F is a closed set and p is a limit point of F, so p ⇐ F. Hence S has its limit point in F.
Example 31: If A is open and B is closed, show that A ~ B is open and B ~ A is closed.
Since B is closed, R ∼ B is an open set. Now A ~ B = A ∩ (R ~ B), which being the intersection of two open sets is an open set. Thus A ~ B is an open set.
Again B ~ A = B ∩ (R~A), which being the intersection of two closed sets is a closed set. Thus B ~ A is a closed set.

|
Explore Courses for IIT JAM exam
|

|
 ....(1)
....(1)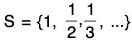 does not belong to the set i.e., 0 ∉ S’.
does not belong to the set i.e., 0 ∉ S’. is a nbd. of m which does not contain any point of N different from m.
is a nbd. of m which does not contain any point of N different from m.

 Then S’ = {0}. Clearly, S ∩ S’ = ϕ
Then S’ = {0}. Clearly, S ∩ S’ = ϕ Then S’ = {1, - 1} c S.
Then S’ = {1, - 1} c S.
















