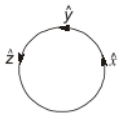
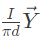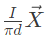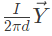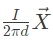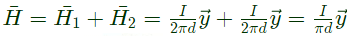Q1: The given equation represents a magnetic field strength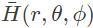 in the spherical coordinate system, in free space. Here,
in the spherical coordinate system, in free space. Here,  represent the unit vectors along r and θ, respectively. The value of P in the equation should be _____ (rounded off to the nearest integer). (2024)
represent the unit vectors along r and θ, respectively. The value of P in the equation should be _____ (rounded off to the nearest integer). (2024)
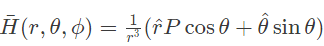 (a) 1
(a) 1
(b) 2
(c) 3
(d) 4
Ans: (b)
Sol:  Spherical coordinate system,
Spherical coordinate system,
On substituting P = 2.
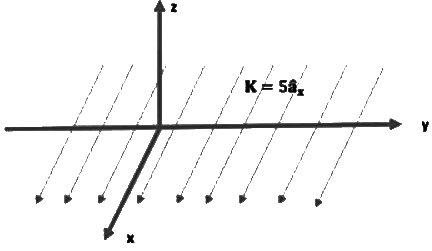
Q2: An infinite surface of linear current density 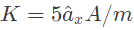 exists on the x - y plane, as shown in the figure. The magnitude of the magnetic field intensity (H) at a point (1, 1, 1) due to the surface current in Ampere/meter is ___ (Round off to 2 decimal places). (2023)
exists on the x - y plane, as shown in the figure. The magnitude of the magnetic field intensity (H) at a point (1, 1, 1) due to the surface current in Ampere/meter is ___ (Round off to 2 decimal places). (2023)
 (a) 1.45
(a) 1.45
(b) 6.36
(c) 2.5
(d) 3.54
Ans: (c)
Sol: 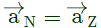
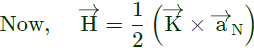
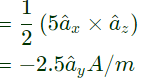 ∴ Magnitude,
∴ Magnitude, 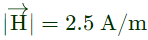
Q3: If the magnetic field intensity (H) in a conducting region is given by the expression, 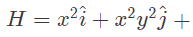
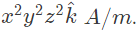 The magnitude of the current density, in A/m2 , at x = 1m, y = 2m and z = 1m, is (2022)
The magnitude of the current density, in A/m2 , at x = 1m, y = 2m and z = 1m, is (2022)
(a) 8
(b) 12
(c) 16
(d) 20
Ans: (b)
Sol: We have,
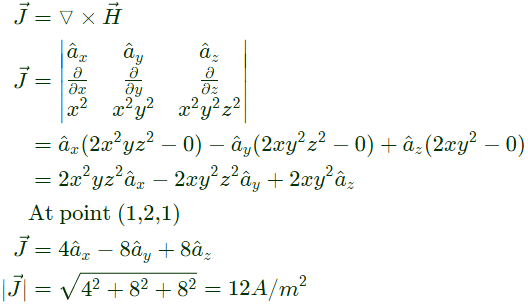
Q4: A long conducting cylinder having a radius 'b' is placed along the z axis. The current density is 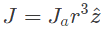 for the region r < b where r is the distance in the radial direction. The magnetic field intensity (H) for the region inside the conductor (i.e. for r < b) is (2022)
for the region r < b where r is the distance in the radial direction. The magnetic field intensity (H) for the region inside the conductor (i.e. for r < b) is (2022)
(a) 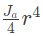
(b) 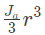
(c) 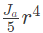
(d) 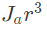
Ans: (c)
Sol: Using Ampere?s law,
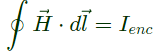 where,
where, 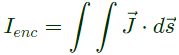
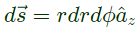
Q5: One coulomb of point charge moving with a uniform velocity 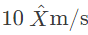 enters the region x ≥ 0 having a magnetic flux density
enters the region x ≥ 0 having a magnetic flux density 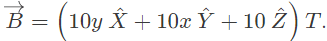 The magnitude of force on the charge at x = 0+ is ______ N.
The magnitude of force on the charge at x = 0+ is ______ N. 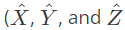 are unit vectors along x-axis, y-axis and z-axis, respectively.) (2021)
are unit vectors along x-axis, y-axis and z-axis, respectively.) (2021)
(a) 80
(b) 125
(c) 112
(d) 100
Ans: (d)
Sol: Force on a charge moving with 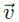 velocity due to magnetic field is
velocity due to magnetic field is
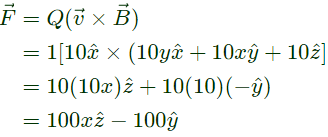
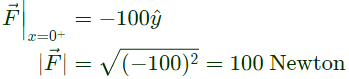
Q6: Which one of the following vector functions represents a magnetic field 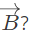
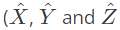 are unit vectors along x-axis, y-axis, and z-axis, respectively) (2021)
are unit vectors along x-axis, y-axis, and z-axis, respectively) (2021)
(a) 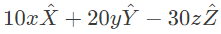
(b) 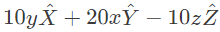
(c) 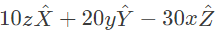
(d) 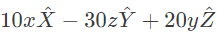
Ans: (a)
Sol: If 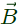 is magnetic flux density then
is magnetic flux density then 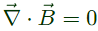
Q7: The magnitude of magnetic flux density (B) in micro Teslas (μT) at the center of a loop of wire wound as a regular hexagon of side length 1m carrying a current (I=1A), and placed in vacuum as shown in the figure is __________. (SET-1 (2017))
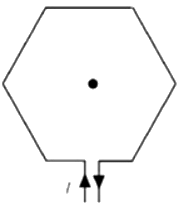 (a) 1.4
(a) 1.4
(b) 2.8
(c) 0.7
(d) 0.2
Ans: (c)
Sol: i = 1 A
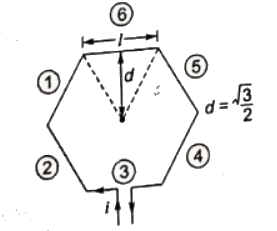 Here, B at point P is
Here, B at point P is
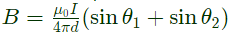 We know for each segment of hexagon
We know for each segment of hexagon
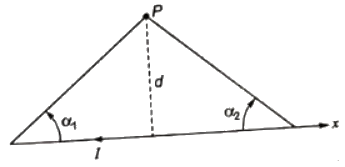 magnetic field intensity due to element length dx,
magnetic field intensity due to element length dx,

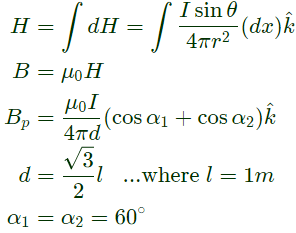
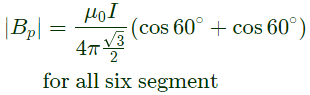
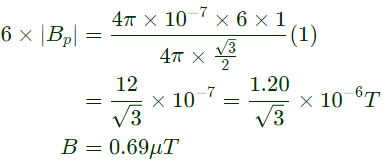
Q8: A solid iron cylinder is placed in a region containing a uniform magnetic field such that the cylinder axis is parallel to the magnetic field direction. The magnetic field lines inside the cylinder will (SET-1(2017))
(a) bend closer to the cylinder axis
(b) bend farther away from the axis
(c) remain uniform as before
(d) cease to exist inside the cylinder
Ans: (a)
Sol: Iron being a ferromagnetic material, magnetic lines of force bend closer to cylindrical axis.
Q9: A soft-iron toroid is concentric with a long straight conductor carrying a direct current I. If the relative permeability μr of soft-iron is 100, the ratio of the magnetic flux densities at two adjacent points located just inside and just outside the toroid, is _______. (SET-1 (2016))
(a) 100
(b) 200
(c) 300
(d) 350
Ans: (a)
Sol: Toroid has field,
B ∝ μ
As μ = 100 (inside field)
Magnetic field density B at any point at a distance at r is
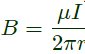
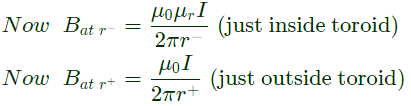
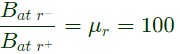
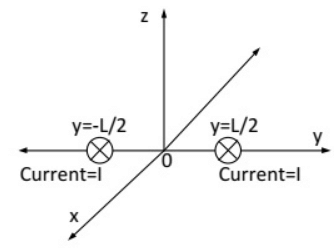
Q10: A steady current I is flowing in the -x direction through each of two infinitely long wires at 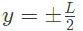 as shown in the figure. The permeability of the medium is μ0. The
as shown in the figure. The permeability of the medium is μ0. The 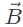 -field at (0, L, 0) is (SET-1 (2015))
-field at (0, L, 0) is (SET-1 (2015))
 (a)
(a) 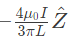
(b) 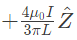
(c) 0
(d) 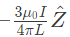
Ans: (a)
Sol: 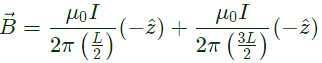
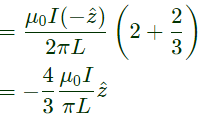
Q11: The magnitude of magnetic flux density 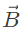 at a point having normal distance d meters from an infinitely extended wire carrying current of I A is
at a point having normal distance d meters from an infinitely extended wire carrying current of I A is 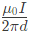 (in SI units). An infinitely extended wire is laid along the x -axis and is carrying current of 4 A in the +ve x direction. Another infinitely extended wire is laid along the y axis and is carrying 2 A current in the +ve y direction. μ0 is permeability of free space. Assume
(in SI units). An infinitely extended wire is laid along the x -axis and is carrying current of 4 A in the +ve x direction. Another infinitely extended wire is laid along the y axis and is carrying 2 A current in the +ve y direction. μ0 is permeability of free space. Assume 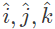 to be unit vectors along x,y and z axes respectively.
to be unit vectors along x,y and z axes respectively.
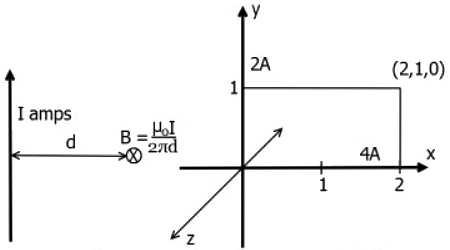 Assuming right handed coordinate system, magnetic field intensity,
Assuming right handed coordinate system, magnetic field intensity, 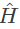 at coordinate (2,1,0) will be (SET-2 (2014))
at coordinate (2,1,0) will be (SET-2 (2014))
(a) 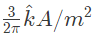
(b) 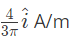
(c) 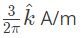
(d) 0 A/m
Ans: (c)
Q12: The following four vector fields are given in Cartesian co-ordinate system. The vector field which does not satisfy the property of magnetic flux density is (SET-1 (2014))
(a) 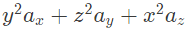
(b) 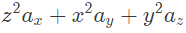
(c) 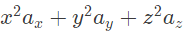
(d) 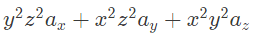
Ans: (c)
Sol: The property of a solid magnetic field is
 (i.e. solenoidal property)
(i.e. solenoidal property)
when,
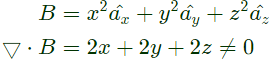
Q13: The flux density at a point in space is given by B = 4xax + 2kyay + 8az Wb/m2. The value of constant k must be equal to (2013)
(a) -2
(b) -0.5
(c) 0.5
(d) 2
Ans: (a)
Sol: 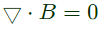
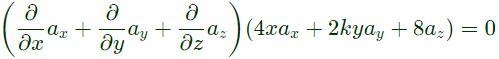
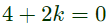 k = -2
k = -2
Q14: A coil of 300 turns is wound on a non-magnetic core having a mean circumference of 300 mm and a cross-sectional area of 300 mm2 . The inductance of the coil corresponding to a magnetizing current of 3 A will be (Given that μ0 = 4π × 10−7H/m) (2008)
(a) 37.68 μH
(b) 113.04 μH
(c) 37.68 μH
(d) 113.04 μH
Ans: (b)
Sol: n = 300
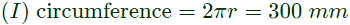
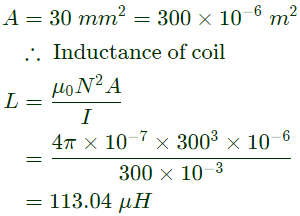
Q15: An inductor designed with 400 turns coil wound on an iron core of 16 cm2 cross sectional area and with a cut of an air gap length of 1 mm. The coil is connected to a 230 V, 50 Hz ac supply. Neglect coil resistance, core loss, iron reluctance and leakage inductance, μ0 = 4π×10−7 H/M
The average force on the core to reduce the air gap will be (2007)
(a) 832.29N
(b) 1666.22N
(c) 3332.47N
(d) 6664.84N
Ans: (a)
Sol: 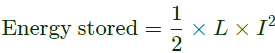
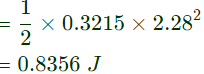 Force to reduce 1 mm air gap = E/d =
Force to reduce 1 mm air gap = E/d = 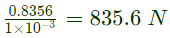
Q16: An inductor designed with 400 turns coil wound on an iron core of 16 cm2 cross sectional area and with a cut of an air gap length of 1 mm. The coil is connected to a 230 V, 50 Hz ac supply. Neglect coil resistance, core loss, iron reluctance and leakage inductance, μ0 = 4π × 10−7 H/M
The current in the inductor is (2007)
(a) 18.08A
(b) 9.04 A
(c) 4.56 A
(d) 2.28 A
Ans: (d)
Sol: 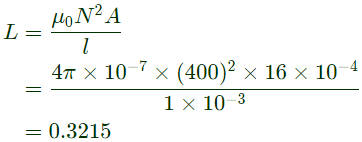
Q17: Which of the following statement holds for the divergence of electric and magnetic flux densities? (2006)
(a) Both are zero
(b) These are zero for static densities but non zero for time varying densities.
(c) It is zero for the electric flux density
(d) It is zero for the magnetic flux density
Ans: (d)
Sol: The divergence of magnetic field is always zzero because magnetic flux makes always a closed path.
So, ▽⋅B = 0 (Maxweel's equation)
while divergence of electric field,
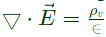
Q18: The inductance of a long solenoid of length 1000 mm wound uniformly with 3000 turns on a cylindrical paper tube of 60 mm diameter is (2004)
(a) 3.2 μH
(b) 3.2 mH
(c) 32.0 mH
(d) 3.2 H
Ans: (c)
Sol: 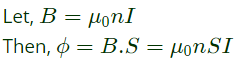 Where 'S' is the cross sectional area of solenoid flux linkage,
Where 'S' is the cross sectional area of solenoid flux linkage,
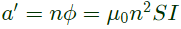 Hence, Inductance length = μ0n2S
Hence, Inductance length = μ0n2S
For,
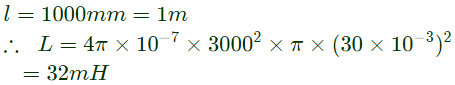
Q19: Two infinite strips of width w m in x -direction as shown in figure, are carrying forward and return currents of +I and -I in the z - direction. The strips are separated by distance of x m. The inductance per unit length of the configuration is measured to be L H/m. If the distance of separation between the strips in snow reduced to x/2 m, the inductance per unit length of the configuration is (2003)
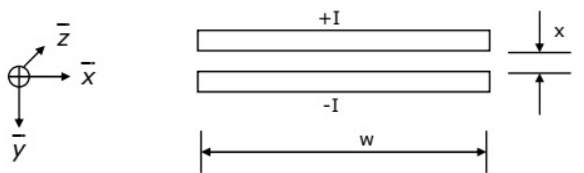 (a) 2L H/m
(a) 2L H/m
(b) L/4 H/m
(c) L/2 H/m
(d) 4L H/m
Ans: (c)
Sol: Inductance is proportional to separation between the strips so when separation is reduced to x/2 then inductance will become L/2 H/m.
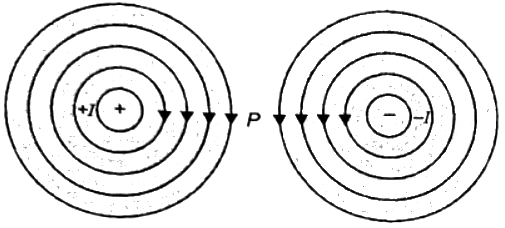
Q20: Two conductors are carrying forward and return current of +I and -I as shown in figure. The magnetic field intensity H at point P is (2003)
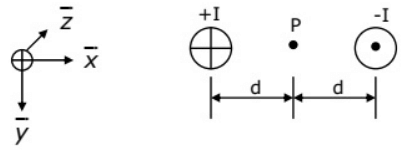 (a)
(a) 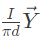
(b) 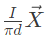
(c) 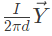
(d) 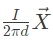
Ans: (a)
Sol: 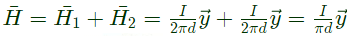
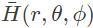 in the spherical coordinate system, in free space. Here,
in the spherical coordinate system, in free space. Here,  represent the unit vectors along r and θ, respectively. The value of P in the equation should be _____ (rounded off to the nearest integer). (2024)
represent the unit vectors along r and θ, respectively. The value of P in the equation should be _____ (rounded off to the nearest integer). (2024)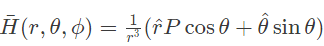 (a) 1
(a) 1 Spherical coordinate system,
Spherical coordinate system, exists on the x - y plane, as shown in the figure. The magnitude of the magnetic field intensity (H) at a point (1, 1, 1) due to the surface current in Ampere/meter is ___ (Round off to 2 decimal places). (2023)
exists on the x - y plane, as shown in the figure. The magnitude of the magnetic field intensity (H) at a point (1, 1, 1) due to the surface current in Ampere/meter is ___ (Round off to 2 decimal places). (2023) (a) 1.45
(a) 1.45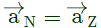
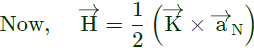
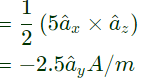 ∴ Magnitude,
∴ Magnitude, 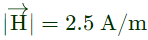
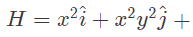
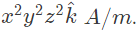 The magnitude of the current density, in A/m2 , at x = 1m, y = 2m and z = 1m, is (2022)
The magnitude of the current density, in A/m2 , at x = 1m, y = 2m and z = 1m, is (2022)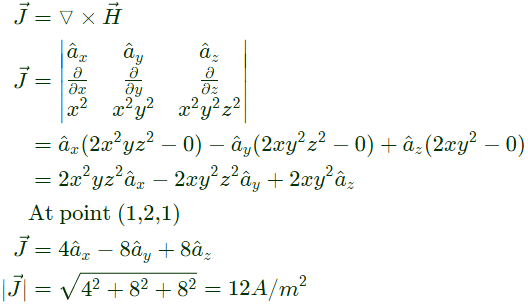
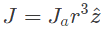 for the region r < b where r is the distance in the radial direction. The magnetic field intensity (H) for the region inside the conductor (i.e. for r < b) is (2022)
for the region r < b where r is the distance in the radial direction. The magnetic field intensity (H) for the region inside the conductor (i.e. for r < b) is (2022)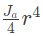
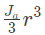
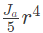
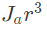
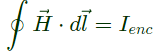 where,
where, 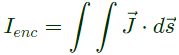
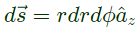
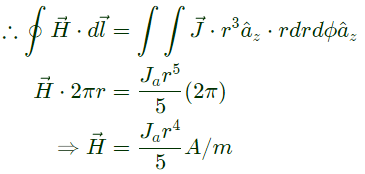
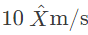 enters the region x ≥ 0 having a magnetic flux density
enters the region x ≥ 0 having a magnetic flux density 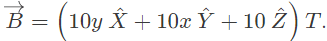 The magnitude of force on the charge at x = 0+ is ______ N.
The magnitude of force on the charge at x = 0+ is ______ N.  are unit vectors along x-axis, y-axis and z-axis, respectively.) (2021)
are unit vectors along x-axis, y-axis and z-axis, respectively.) (2021) velocity due to magnetic field is
velocity due to magnetic field is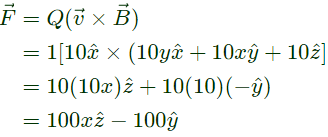
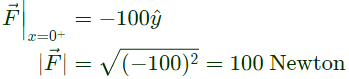

 are unit vectors along x-axis, y-axis, and z-axis, respectively) (2021)
are unit vectors along x-axis, y-axis, and z-axis, respectively) (2021)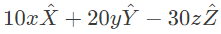
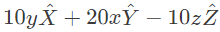
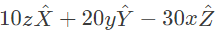
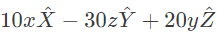
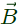 is magnetic flux density then
is magnetic flux density then 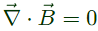
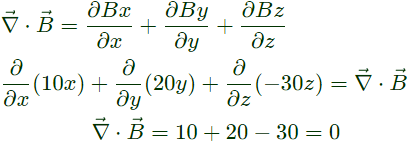
 (a) 1.4
(a) 1.4 Here, B at point P is
Here, B at point P is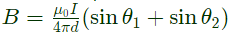 We know for each segment of hexagon
We know for each segment of hexagon magnetic field intensity due to element length dx,
magnetic field intensity due to element length dx,
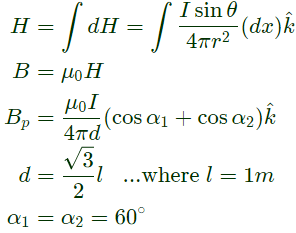
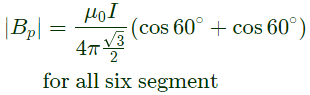
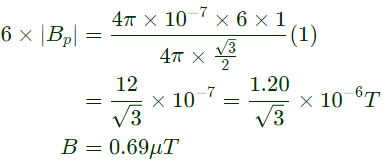
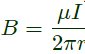
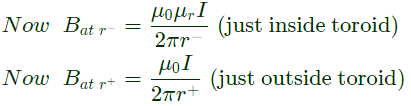
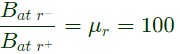
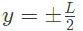 as shown in the figure. The permeability of the medium is μ0. The
as shown in the figure. The permeability of the medium is μ0. The 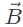 -field at (0, L, 0) is (SET-1 (2015))
-field at (0, L, 0) is (SET-1 (2015)) (a)
(a) 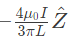
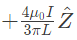
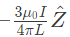
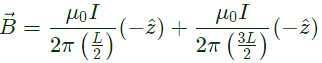
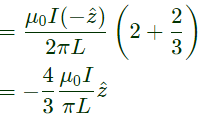
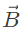 at a point having normal distance d meters from an infinitely extended wire carrying current of I A is
at a point having normal distance d meters from an infinitely extended wire carrying current of I A is 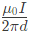 (in SI units). An infinitely extended wire is laid along the x -axis and is carrying current of 4 A in the +ve x direction. Another infinitely extended wire is laid along the y axis and is carrying 2 A current in the +ve y direction. μ0 is permeability of free space. Assume
(in SI units). An infinitely extended wire is laid along the x -axis and is carrying current of 4 A in the +ve x direction. Another infinitely extended wire is laid along the y axis and is carrying 2 A current in the +ve y direction. μ0 is permeability of free space. Assume 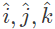 to be unit vectors along x,y and z axes respectively.
to be unit vectors along x,y and z axes respectively. Assuming right handed coordinate system, magnetic field intensity,
Assuming right handed coordinate system, magnetic field intensity, 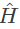 at coordinate (2,1,0) will be (SET-2 (2014))
at coordinate (2,1,0) will be (SET-2 (2014))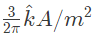
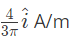
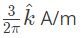
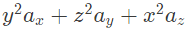
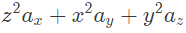
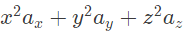
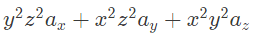
 (i.e. solenoidal property)
(i.e. solenoidal property)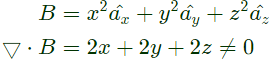
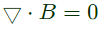
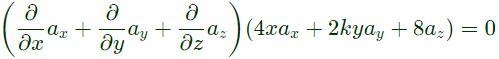
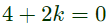 k = -2
k = -2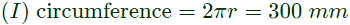
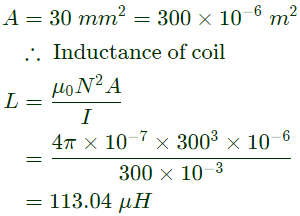
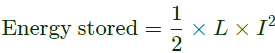
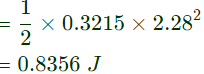 Force to reduce 1 mm air gap = E/d =
Force to reduce 1 mm air gap = E/d = 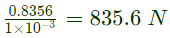
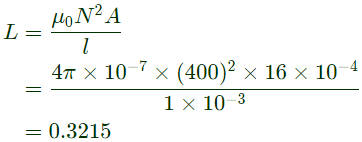
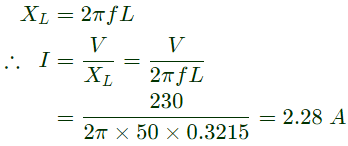
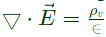
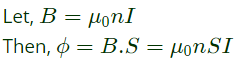 Where 'S' is the cross sectional area of solenoid flux linkage,
Where 'S' is the cross sectional area of solenoid flux linkage,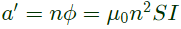 Hence, Inductance length = μ0n2S
Hence, Inductance length = μ0n2S
 (a) 2L H/m
(a) 2L H/m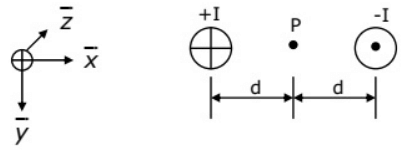 (a)
(a)