Quadratic Equations | Physics for JEE Main & Advanced PDF Download

What is a Quadratic  Equation?

A quadratic equation in the variable x is an equation of the form ax² + bx + c = 0, where a, b, c are real numbers and a ≠ 0 is called the standard form of a quadratic equation.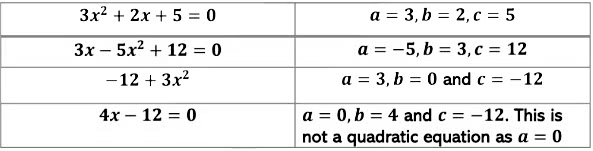

Example 1: Check whether the following are quadratic equations
(i) (x + 1)² = 2(x − 3)
⇒ (x + 1)² = x² + 2x + 1
∵ (a + b)² = a² + 2ab + b²
⇒ x² + 2x + 1 = 2(x − 3)
⇒ x² + 2x + 1 = 2x − 6
⇒ x² + 2x + 1 − 2x + 6 = 0
⇒ x² + 2x − 2x + 6 + 1 = 0
x² + 7 = 0
The above equation is a quadratic equation, where the coefficient of x is zero, i.e. b = 0

(ii) x(x + 1)(x + 8) = (x + 2)(x − 2)
LHS
⇒ x(x + 1)(x + 8)
⇒ x(x² + 8x + x + 8)
⇒ x(x² + 9x + 8)
⇒ x³ + 9x² + 8x
RHS
(x + 2)(x − 2)
⇒ x² − 4
∵ (a + b)(a − b) = a² − b²
Now, x³ + 9x² + 8x = x2 − 4
⇒ x³ + 9x² − x² + 8x + 4 = 0
x³ + 8x² + 8x + 4 = 0
It is not a quadratic equation as it is an equation of degree 3.

(iii) (x − 2)² + 1 = 2x − 3
LHS
(x − 2)² + 1 = x² − 2x + 4 + 1
∵ (a − b)² = a² − 2ab + b²
= x² − 2x + 5
RHS
2x − 3
⇒ x² − 2x + 5 = 2x − 3
⇒ x² − 2x − 2x + 5 + 3 = 0
⇒ x² − 4x + 8 = 0
The above equation is quadratic as it is of the form,
ax² + bx + c = 0

Example 2: The product of two consecutive positive integers is 420. Form the equation satisfying this scenario.
Let the two consecutive positive integers be x and x + 1 Product of the two consecutive integers= x(x + 1) = 420
⇒ x² + x = 420
⇒ x² + x − 420 = 0
x² + x − 420 = 0, is the required quadratic equation and the two integers satisfy this quadratic equation.

Solution of Quadratic Equation by Factorisation

A real number α is called a root of the quadratic equation x² +bx + c = 0 , a ≠ 0 if aα² + bα + c = 0
We say that x = α is a solution of the quadratic equation.
Example: x² − 2x − 3 = 0
If we put x = −1 in the LHS of the above equation we get,
(−1)² − 2(−1) − 3
1 + 2 − 3 = 0
Thus x = −1 is a solution of the equation x² − 2x − 3 = 0.
To find the roots of the quadratic equations we follow these steps.

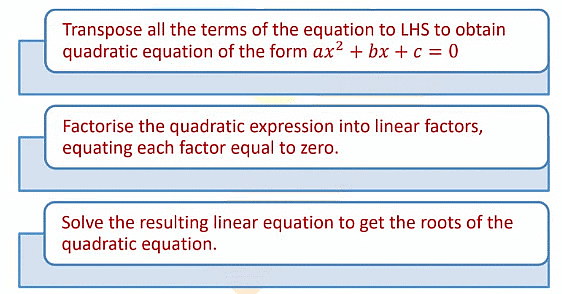
Example 3: Find the roots of the equation x² − 3x − 10 = 0
The given equation is x² − 3x − 10 = 0.
Here a = 1, b = −3 and c = −10
(1) Find the product of a and c.
Here, the product of a and c= -10 → (ac) is negative

(2) Write the factors of this product (ac) such that the sum of the two factors is equal to b.
∴ ac = m × n and m + n = b
Factors of 10 = 2×5
Let m = −5 and n = 2 → (ac=-10)
We write the given equation as,
x² − 5x + 2x − 10 = 0
x(x − 5) + 2(x − 5) = 0
x − 5 x + 2 = 0
Equate each factor to zero to get the roots of the equation.
x − 5 = 0 and x + 2 = 0
x = 5, −2
Therefore, 5 and -2 are the roots of the equation x² − 3x − 10 = 0

Example 4: Solve the following quadratic equation by factorisation method.
i) 4√3x²  + 5x − 2√3  = 0
The given equation is 4√3x²  + 5x − 2√3  = 0
Here, a = 4√3 , b = 5 and c = −2√3
The product of a and c
= 4√3  × (−2√3 )
= −8 × 3
= −24
Factors of 24 = 3×8 and 8 + (−3) = 5
The factors of the equation are 8, − 3
So, the given equation can be written as,
4√3x²  + (8 − 3)x − 2√3  = 0
⇒ 4 √3x² + 8x − 3x − 2√3  = 0
⇒ 4x( √3x + 2) −√3 (√3 x + 2) = 0
⇒ (4x − √3 )(√3x + 2) = 0
Equating each factor to zero we get,
(4x −√3 )=0 and (√3x + 2) = 0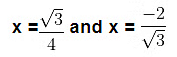

The roots of the equation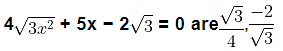


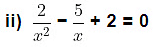
The given equation is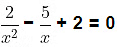
Multiplying the above equation by x² we get,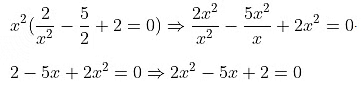

Here, a = 2, b = −5 and c = 2
The product of a and c = 2 × 2 = 4
The factors of 4 = 4 × 1 and 4 + 1 = 5
2x² − (4 + 1)x + 2 = 0
⇒ 2x² − 4x − 1x + 2 = 0
2x(x − 2) − (x − 2) = 0
(2x − 1)(x − 2) = 0
Equating each factor to zero we get,
(2x − 1) = 0 and (x − 2) = 0
x =  1/2 and x = 2
The roots of equation 2x² − 5x + 2 = 0 are  1/2  and 2

Example 5: The altitude of a right-angled triangle is 7 cm less than its base. If the hypotenuse is 13 cm long, then find the other two sides.
Let the length of the base be x cm, then altitude = x − 7 cm
Hypotenuse = 13 cm
We know, H² = P² + B²
132 = (x − 7)² + x² 
⇒ 169 = x² − 14x + 49 + x²
⇒ x² − 14x + 49 + x² = 169
⇒ 2x² − 14x + 49 − 169 = 0
⇒ 2x² − 14x − 120 = 0
Dividing the above equation by 2 we get,
x² − 7x − 60 = 0
Here, a = 1, b = −7 and c = −60
The product of a and c = 1 × (−60) = −60
The factors of 60 = 5 × 12 and −12 + 5 = 7
The given equation can be written as,
x² − 12x + 5x − 60 = 0
x(x − 12) + 5(x − 12) = 0
⇒ (x + 5)(x − 12) = 0
Equating each factor to zero we get,
(x + 5) = 0 and (x − 12) = 0
⇒ x = −5 and x = 12
The length of the base cannot be negative.
Therefore, Base = 12 cm
Altitude = x − 7 cm = 12 − 7 = 5 cm, Hypotenuse = 13 cm

Nature of Roots

The roots of the quadratic equation ax² + bx + c = 0 are given by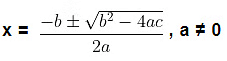

Where D = √b² − 4ac is called the discriminant.
This formula is known as the Quadratic Formula.
The nature of the roots depends upon the value of Discriminant, D.

Example 6: Find the roots of the equation,

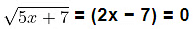
The given equation is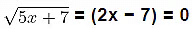
Squaring both sides of the equation we get,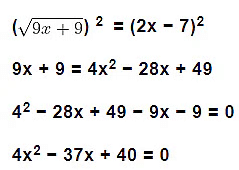

Here, a = 4, b = −37 and c = 40
Substituting the value of a, b and c in the quadratic formula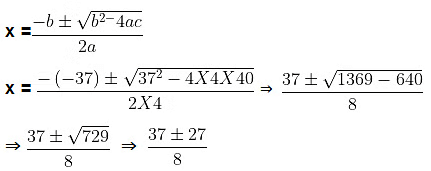

Taking +ve sign first,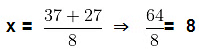

Taking -ve we get,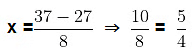

The roots of the given equation are 8 and 5/4.

Example 7: Find the numerical difference of the roots of the equation x² − 7x − 30 = 0
The given quadratic equation is x² − 7x − 30 = 0
Here a = 1, b = −7 and c = −30
Substituting the value of a, b and c in the quadratic formula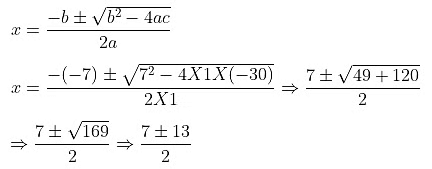

Taking +ve sign first,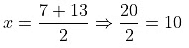

Taking -ve we get,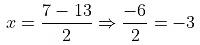

The two roots are 10 and -3
The difference of the roots= 10 − (−3) = 10 + 3 = 13

Example 8: Find the discriminant of the quadratic equation x² −4x − 5 = 0
The given quadratic equation is x² − 4x − 5 = 0.
On comparing with ax² + bx + c = 0 we get,
a = 1,b = −4, and c = −5

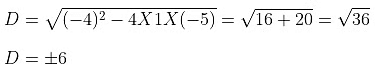
Example 9: Find the value of p, so that the quadratic equation px(x − 2) + 9 = 0 has equal roots.
The given quadratic equation is px(x − 2) + 9 = 0
px² − 2px + 9 = 0
Now comparing with ax² + bx + c = 0 we get,
a = p, b = −2p and c = 9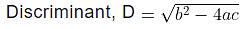

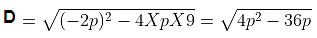
The given quadratic equation will have equal roots if D = 0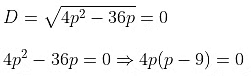

p = 0 and p − 9 = 0 ⇒ p = 9
p = 0 and p = 9
The value of p cannot be zero as the coefficient of x, (−2p) will become zero.
Therefore, we take the value of p = 9.

Example 10: If x = −1 is a root of the quadratic equations 2x² +px + 5 = 0 and the quadratic equation p(x² + x) + k = 0 has equal roots, then find the value of k.
The given quadratic equation is 2x² + px + 5 = 0. If x = −1 is the root of the equation then,
2(−1)2 + p(−1) + 5 = 0
2 − p + 5 = 0
⇒ −p = −7
p = 7
Putting the value of p in the equation p(x² + x) + k = 0,
7(x² + x) + k = 0
⇒ 7x² + 7x + k = 0
Now comparing with ax² + bx + c = 0 we get,
a = 7, b = 7 and c = k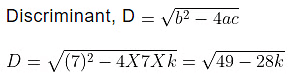

The given quadratic equation will have equal roots if D = 0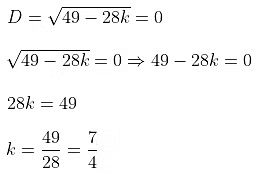

Therefore, the value of k is 7/4.

Solution of the Quadratic Equations by Completing the  Square (Deleted from NCERT Textbook)

If we have to find the solution of a quadratic equation by completing the square, we follow the steps given below.

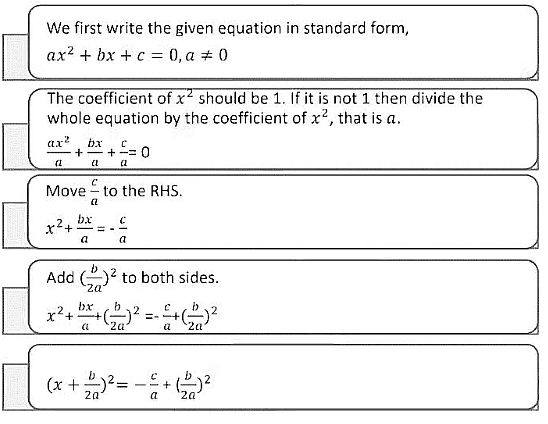
The complete square is,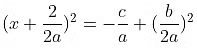

Example 11: Find the roots of the following quadratic equations by the method of completing the square:
(i) 2x² − 7x + 3 = 0
The given quadratic equation is 2x² − 7x + 3 = 0
The coefficient of x² is not 1, so we divide the whole equation by 2.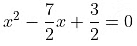

Now move 3/2 to RHS

Adding (7/4)² to both sides we get,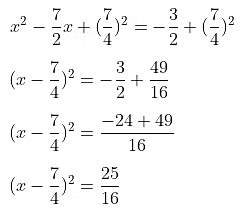

Taking square root of both sides we get,

The roots of the equation are 3 and 1/2

(ii) 4x² + 4√3x + 3 = 0
Dividing the whole equation by 4, so that the coefficient of x² is 1.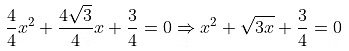

Shifting 3/4 to RHS

Adding 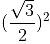 to both sides we get,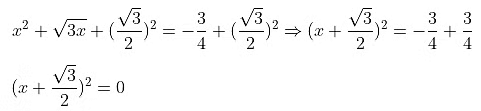

Taking the square root of both sides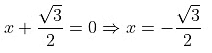

The roots of the given equation are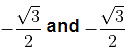

Example 12: Solve the quadratic equation

x² − (√5 + 1)x + √5 = 0 by completing the square method.
The given quadratic equation is, x² − (√5 + 1)x = −√5 = 0
Shifting √5 to RHS we get,
x² − (√5 + 1)x = −√5
Adding 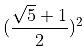 to both sides we get,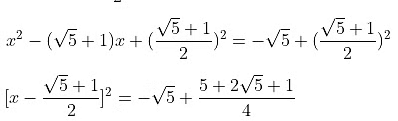

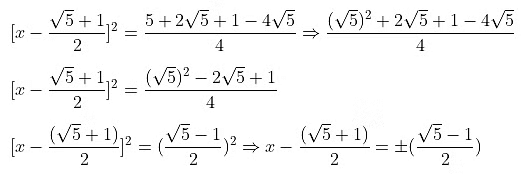
Taking +ve sign first

Taking –ve sign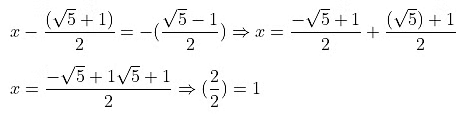

The roots of the given equation are √5 and 1