Fractions (Exercise 2.3) RD Sharma Solutions | Mathematics (Maths) Class 7 PDF Download
Q.1. Find the reciprocal of each of the following fractions and classify them as proper, improper and whole numbers:
(i) 3/7
(ii) 5/8
(iii) 9/7
(iv) 6/5
(v) 12/7
(vi) 1/8
Ans: Reciprocal of a non-zero fraction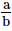 is
is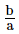
(i)
Reciprocal of 3/7 is 7 3
It's improper fraction because numerator is greater than denominator
(ii)
Reciprocal of 5/8 is 8/5
It's improper fraction because numerator is greater than denominator
(iii)
Reciprocal of 9/7 is 7/9
It's proper fraction because numerator is less than denominator
(iv)
Reciprocal of 6/5 is 5/6
It's proper fraction because numerator is less than denominator
(v)
Reciprocal of 12/7 is 7/12
It's proper fraction because numerator is less than denominator
(vi)
Reciprocal of 1/8 is 8/1 = 8
It is a whole number
Q.2. Divide:
(i)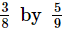
(ii)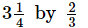
(iii)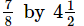
(iv)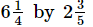
Ans:
(i)
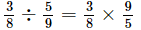
⇒ 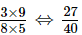
(ii)
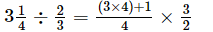
⇒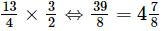
(iii)
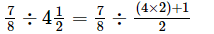
⇒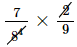
⇒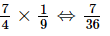
(iv)
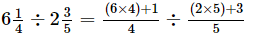
⇒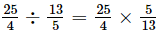
⇒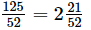
Q.3. Divide:
(i)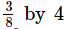
(ii)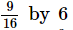
(iii)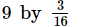
(iv)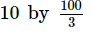
Ans:
(i)
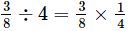
=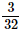
(ii)
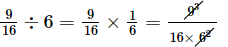
=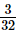
(iii)
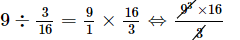
= 48
(iv)
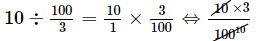
=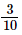
Q.4. Simplify:
(i)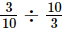
(ii)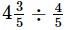
(iii)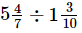
(iv)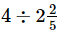
Ans:
(i)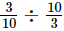 =
=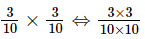
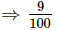
(ii)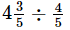 =
=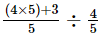
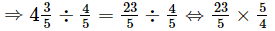
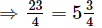
(iii)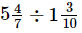 =
=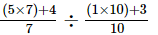
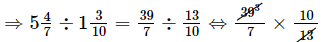
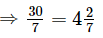
(iv)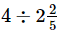 =
=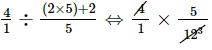
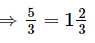
Q.5. A wire of length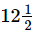 m is cut into 10 pieces of equal length. Find the length of each piece.
m is cut into 10 pieces of equal length. Find the length of each piece.
Ans:
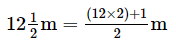
=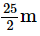
Length of one piece =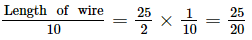
Length of one piece =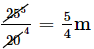
Q.6. The length of a rectangular plot of area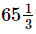 m2 is
m2 is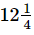 m. What is the width of the plot?
m. What is the width of the plot?
Ans: Area of rectangle = Length of rectangle × Width of rectangle
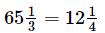 × Width of the rectangle
× Width of the rectangle
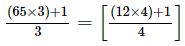 × Width of the rectangle
× Width of the rectangle
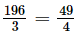 × Width of the rectangle
× Width of the rectangle
Width of the rectangle =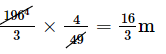
Q.7. By what number should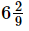 be multiplied to get
be multiplied to get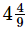 ?
?
Ans:
Let the required number be x.
According to the question:
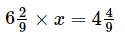
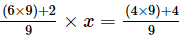
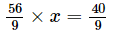
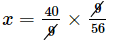
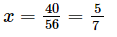
Q.8. The product of two numbers is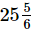 . If one of the numbers is
. If one of the numbers is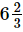 , find the other.
, find the other.
Ans:
Let the required number be x.
According to the question:
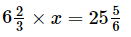
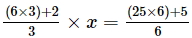
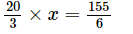
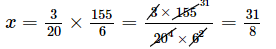
=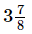
Q.9. The cost of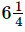 kg of apples is Rs 400. At what rate per kg are the apples being sold?
kg of apples is Rs 400. At what rate per kg are the apples being sold?
Ans:
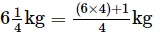
⇒ 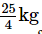
Cost of 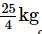 kg of apples = Rs. 400
kg of apples = Rs. 400
Cost of 1 kg of apples = 400÷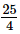 = 400 x
= 400 x 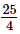 = Rs. 64
= Rs. 64
Q.10. By selling oranges at the rate of Rs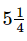 per orange, a fruit-seller gets Rs 630. How many dozens of oranges does he sell?
per orange, a fruit-seller gets Rs 630. How many dozens of oranges does he sell?
Ans:
Cost of 1 orange = Rs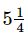 =
=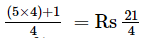
Number of oranges sold = 630÷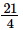
⇒ 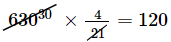
∵ 12 oranges = 1 dozen
∴ 120 oranges =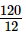 = 10 dozen
= 10 dozen
Q.11. In mid-day meal scheme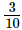 litre of milk is given to each student of a primary school. If 30 litres of milk is distributed every day in the school, how many students are there in the school?
litre of milk is given to each student of a primary school. If 30 litres of milk is distributed every day in the school, how many students are there in the school?
Ans:
Number of students in the school =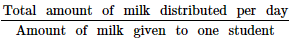
=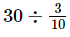
=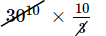
= 100
Q.12. In a charity show Rs 6496 were collected by selling some tickets. If the price of each ticket was Rs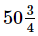 , how many tikets were sold?
, how many tikets were sold?
Ans:
Number of tickets sold =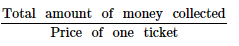
Price of one ticket:
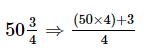
⇒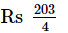
Number of tickets sold = 6496 ÷ 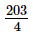
⇒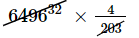
⇒128
Objective Type Questions
Q.1. Mark the correct alternative in each of the following:
If a fraction a/b is a lowest terms, then HCF of a and b is
(a) a
(b) b
(c) 1
(d) ab
Ans: We know that a fraction is in its lowest terms if its numerator and denominator have no common factor other than 1.
Thus, if the fraction a/b is in its lowest terms, then the HCF of a and b is 1.
Hence, the correct answer is option (c).
Q.2. Mark the correct alternative in each of the following:
The fraction 8498 in its lowest terms is
(a)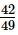
(b)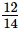
(c)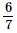
(d)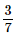
Ans: Factors of 84: 1, 2, 3, 4, 6, 7, 12, 14, 21, 28, 42, 84
Factors of 98: 1, 2, 7, 14, 49, 98
Common factors of 84 and 98: 1, 2, 14
∴ HCF of 84 and 98 = 14
Now,
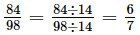 (Dividing numerator and denominator by the HCF of 84 and 98 i.e. 14)
(Dividing numerator and denominator by the HCF of 84 and 98 i.e. 14)
Hence, the correct answer is option (c).
Q.3. Mark the correct alternative in each of the following:
Which of the following is a vulgar fraction?
(a)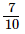
(b)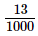
(c)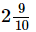
(d)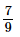
Ans: The fractions with denominator not equal to 10, 100, 1000 etc are called vulgar fractions.
Thus, the fraction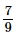 is a vulgar fraction.
is a vulgar fraction.
Hence, the correct answer is option (d).
Q.4. Mark the correct alternative in each of the following:
Which of the following fraction is an irreducible (or in its lowest terms)?
(a)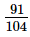
(b)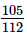
(c)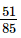
(d)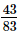
Ans: We know that a fraction is irreducible (or is in its lowest terms) if the HCF of its numerator and denominator is 1.
Consider the fraction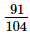 .
.
HCF of 91 and 104 = 13 ≠ 1
So, the fraction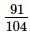 is reducible.
is reducible.
Consider the fraction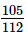 .
.
HCF of 105 and 112 = 7 ≠ 1
So, the fraction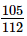 is reducible.
is reducible.
Consider the fraction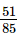 .
.
HCF of 51 and 85 = 17 ≠ 1
So, the fraction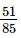 is reducible.
is reducible.
Now,
Consider the fraction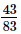 .
.
HCF of 43 and 83 = 1
So, the fraction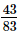 is irreducible (or is in its lowest terms).
is irreducible (or is in its lowest terms).
Hence, the correct answer is option (d).
Q.5. Mark the correct alternative in each of the following:
Which of the following is a proper fraction?
(a)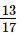
(b)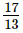
(c)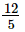
(d)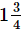
Ans: A fraction whose numerator is less than the denominator is called a proper fraction.
The numerator in each of the fractions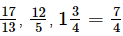 is more than the denominator, so these fractions are improper fractions.
is more than the denominator, so these fractions are improper fractions.
The numerator of the fraction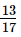 is less than the denominator, so this fraction is a proper fraction.
is less than the denominator, so this fraction is a proper fraction.
Hence, the correct answer is option (a).
Q.6. Mark the correct alternative in each of the following:
The reciprocal of the fraction 235 is
(a)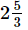
(b)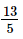
(c)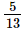
(d)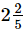
Ans: The reciprocal of a non-zero fraction a/b is the fraction b/a.
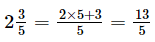
Now,
Reciprocal of the fraction 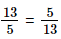
∴ Reciprocal of the fraction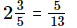
Hence, the correct answer is option (c).
Q.7. Mark the correct alternative in each of the following:
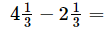
(a)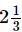
(b) 2
(c)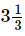
(d)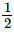
Ans:
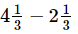
=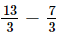
=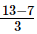
=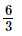
= 2
Hence, the correct answer is option (b).
Q.8. Mark the correct alternative in each of the following:
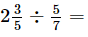
(a)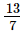
(b)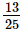
(c)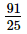
(d)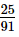
Ans:
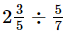
= 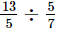
=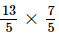
=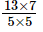
=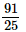
Hence, the correct answer is option (c).
Q.9. Mark the correct alternative in each of the following:
By what number should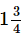 be divided to get
be divided to get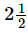 ?
?
(a)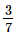
(b)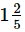
(c)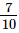
(d)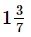
Ans: Let the required number be x.
Now,
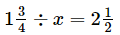
⇒ 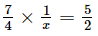
⇒ 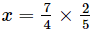
⇒ 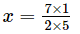
⇒ 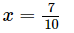
Thus, the required number is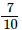
Hence, the correct answer is option (c).
Q.10. Mark the correct alternative in each of the following:
By what number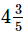 be multiplied to get
be multiplied to get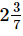 ?
?
(a)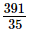
(b)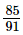
(c)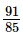
(d) None of these
Ans:
Product of two numbers =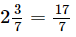
One of the numbers =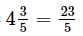
∴ Other number = Product of two numbers ÷ One of the numbers
= 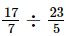
= 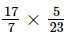
= 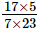
= 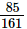
Hence, the correct answer is option (d).
Q.11. Mark the correct alternative in each of the following:
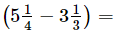
(a)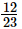
(b) 2
(c)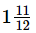
(d)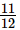
Ans:
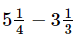
=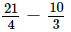
=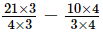 (LCM of 3 and 4 is 12)
(LCM of 3 and 4 is 12)
=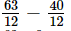
=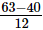
=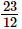
=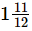
Hence, the correct answer is option (c).
Q.12. Mark the correct alternative in each of the following:
The fraction equivalent to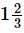 is
is
(a)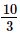
(b)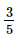
(c)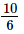
(d)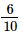
Ans:
The given fraction is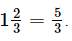
We know that if a/b and c/d are two equivalent fractions, then
a × d = b × c
Now,
5 × 6 = 3 × 10
So, the fractions 5/3 and 10/6 are equivalent fractions.
Thus, the fraction equivalent to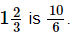
Hence, the correct answer is option (c).
Q.13. Mark the correct alternative in each of the following:
By what number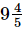 945 be multiplied to get 42?
945 be multiplied to get 42?
(a)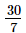
(b)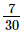
(c)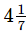
(d)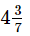
Ans:
Product of two numbers = 42
One of the numbers =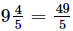
∴ Other number = Product of two numbers ÷ One of the numbers
=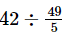
=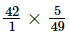
=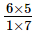
=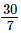
Hence, the correct answer is option (a).
Q.14. Mark the correct alternative in each of the following:
Which of the following statements is true?
(a)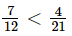
(b)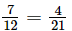
(c)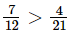
(d) None of these
Ans:
Consider the fractions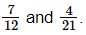
Prime factorisation of 12 = 2 × 2 × 3
Prime factorisation of 21 = 3 × 7
∴ LCM of 12 and 21 = 2 × 2 × 3 × 7 = 84
Firstly, convert the fractions to equivalent fractions with denominator 84.
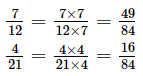
Now,
49 > 16
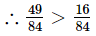
⇒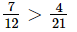
Hence, the correct answer is option (c).
Q.15. Mark the correct alternative in each of the following:
Which one of the following is the correct statement?
(a)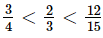
(b)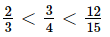
(c)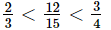
(d)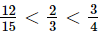
Ans:
Consider the fractions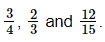
LCM of 4, 3 and 15 = 60
Firstly, convert the fractions into equivalent fractions with denominator 60.
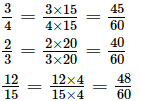
Now,
40 < 45 < 48
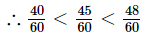
⇒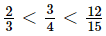
Hence, the correct answer is option (b).
Q.16. Mark the correct alternative in each of the following:
Which of the following fractions lies between 23 and 57?
(a) 3/4
(b) 4/5
(c) 5/6
(d) None of these
Ans:
Consider the fractions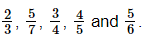
LCM of 3, 4, 5, 6 and 7 = 420
Firstly, convert the fractions into equivalent fractions with denominator 420.
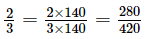
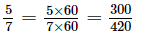
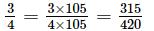
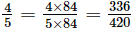
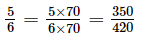
Now,
280 < 300 < 315 < 336 < 350
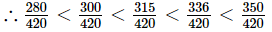
⇒ 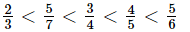
Thus, none of the fractions 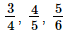 lies between the fractions
lies between the fractions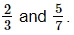
Hence, the correct answer is option (d).
Q.17. Mark the correct alternative in each of the following:
Which one of the following is true?
(a)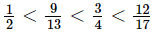
(b)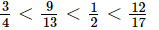
(c)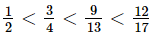
(d) 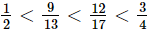
Ans:
Consider the fractions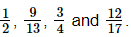
LCM of 2, 4, 13 and 17 = 884
Firstly, convert the fractions into equivalent fractions with denominator 884.
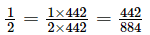
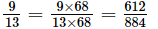
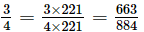
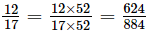
Now,
442 < 612 < 624 < 663
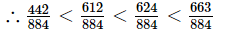
⇒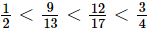
Hence, the correct answer is option (d).
Q.18. Mark the correct alternative in each of the following:
The smallest of the fractions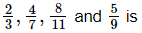
(a) 2/3
(b) 4/7
(c) 8/11
(d) 5/9
Ans:
The given fractions are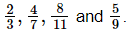
LCM of 3, 7, 9 and 11 = 693
Firstly, convert the fractions into equivalent fractions with denominator 693.
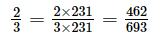
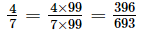
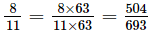
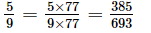
Now,
385 < 396 < 462 < 504
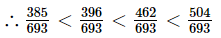
⇒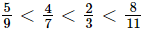
Thus, the smallest of the given fractions is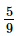 .
.
Hence, the correct answer is option (d).
Q.19. Mark the correct alternative in each of the following:
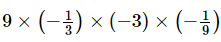 =
=
(a) 1
(b) −1
(c) −3
(d) 3
Ans: Since the number of negative terms in the product is odd. Therefore, their product is negative.
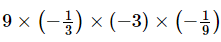
=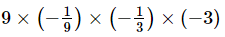
=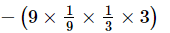
= −(1 × 1)
= −1
Hence, the correct answer is option (b).
Q.20. Mark the correct alternative in each of the following:
Which of the following is correct?
(a)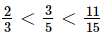
(b)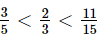
(c)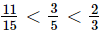
(d)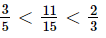
Ans:
Consider the fractions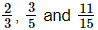 .
.
LCM of 3, 5 and 15 = 15
Firstly, convert the fractions into equivalent fractions with denominator 15.
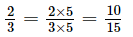
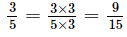
Now,
9 < 10 < 11
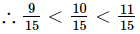
⇒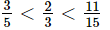
Hence, the correct answer is option (b).
Q.21. Mark the correct alternative in each of the following:
Which is the smallest of the following fractions?
(a)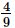
(b)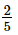
(c)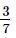
(d)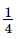
Ans:
Consider the fractions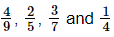 .
.
LCM of 4, 5, 7 and 9 = 1260
Firstly, convert the fractions into equivalent fractions with denominator 1260.
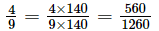
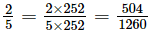
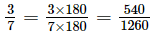
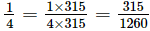
Now,
315 < 504 < 540 < 560
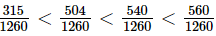
⇒ 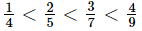
Thus, the smallest fraction is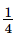 .
.
Hence, the correct answer is option (d).
Q.22. Mark the correct alternative in each of the following:
The difference between the greatest and the least fractions out of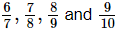 is
is
(a)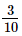
(b)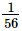
(c)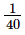
(d)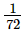
Ans:
Consider the fractions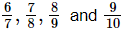 .
.
LCM of 7, 8, 9 and 10 = 2520
Firstly, convert the fractions into equivalent fractions with denominator 2520.
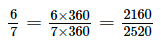
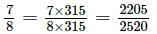
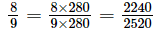
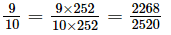
Now,
2160 < 2205 < 2240 < 2268
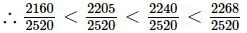
⇒ 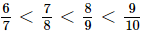
So,
Greatest fraction =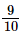
Least fraction =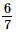
∴ Required difference
=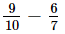
=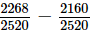
=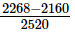
=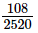
=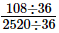 (HCF of 108 and 2520=36)
(HCF of 108 and 2520=36)
=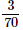
Disclaimer: None of the options given in the question matches with the answer.
Q.23. Mark the correct alternative in each of the following:
Which of the following fractions is greater than 3/4 and less than 5/6?
(a)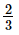
(b)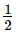
(c)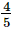
(d)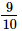
Ans:
Consider the fractions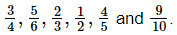
LCM of 2, 3, 4, 5, 6 and 10 = 60
Firstly, convert the fractions into equivalent fractions with denominator 60.
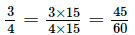
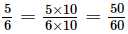
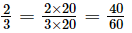
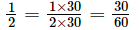
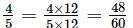
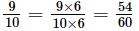
Now,
30 < 40 < 45 < 48 < 50 < 54
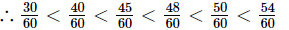
⇒ 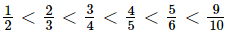
Thus, the fraction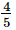 is greater than
is greater than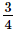 and less than
and less than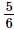 .
.
Hence, the correct answer is option (c).
Q.24. Mark the correct alternative in each of the following:
Which of the following fractions is more than one-third?
(a)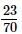
(b)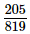
(c)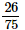
(d)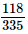
Ans:
Let a/b and c/d be two fractions. Then,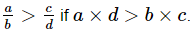
Consider the fractions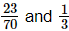 .
.
23 × 3 = 69
1 × 70 = 70
∴23 × 3 < 1 × 70
⇒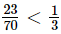
Consider the fractions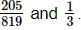
205 × 3 = 615
1 × 819 = 819
∴205 × 3 < 1 × 819
⇒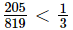
Consider the fractions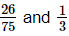 .
.
26 × 3 = 78
1 × 75 = 75
∴26 × 3 > 1 × 75
⇒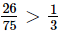
Consider the fractions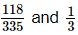 .
.
118 × 3 = 354
1 × 335 = 335
∴118 × 3 > 1 × 335
⇒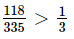
Thus, the fractions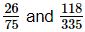 are more than the fraction
are more than the fraction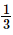 .
.
Hence, the correct answers are options (c) and (d).
Disclaimer: There are two correct options in the question. One of the two options among (c) and (d) must be changed accordingly to get only one correct answer.
|
76 videos|344 docs|39 tests
|
FAQs on Fractions (Exercise 2.3) RD Sharma Solutions - Mathematics (Maths) Class 7
| 1. What are fractions? |  |
| 2. How do you add fractions with different denominators? |  |
| 3. How do you subtract fractions? |  |
| 4. How do you simplify fractions? |  |
| 5. How do you convert fractions into decimals? |  |

|
Explore Courses for Class 7 exam
|

|

















