Revision Notes: Playing with Numbers | Mathematics & Pedagogy Paper 2 for CTET & TET Exams - CTET & State TET PDF Download
"Playing with numbers" refers to exploring the properties and patterns of numbers through various mathematical operations and activities. This topic is integral to the CTET (Central Teacher Eligibility Test) and other educational curricula as it helps students develop a deeper understanding of numbers and their relationships. Here’s an overview:
Factors:
- Rani has 6 marbles with her. She wants to arrange them in rows in such a way that each row has the same number of marbles.
She arranges them in the following ways and matches the total number of marbles:
(i) 1 marble in each row
Number of rows = 6

Total number of marbles = 1 × 6 = 6
(ii) 2 marbles in each row
Number of rows = 3

Total number of marbles = 2 × 3 = 6
(iii) 3 marbles in each row
Number of rows = 2

Total number of marbles = 3 × 2 = 6
(iv) She could not think of any arrangement in which each row had 4 marbles or 5 marbles. So, the only possible arrangement left was with all the 6 marbles in a row.
Number of rows = 1

Total number of marbles = 6 × 1 = 6
From these calculations, Rani observes that 6 can be written as a product of two numbers in different ways as:
6 = 1 × 6;
6 = 2 × 3;
6 = 3 × 2;
6 = 6 × 1
From 6 = 2 × 3; it can be said that 2 and 3 exactly divide 6. So, 2 and 3 are exact divisors of 6.
From the other product 6 = 1 × 6, the exact divisors of 6 are found to be 1 and 6.
Thus, 1, 2, 3 and 6 are exact divisors of 6. They are called the factors of 6.
Now, let's study more about the Factors:
What are Factors, Perfect Numbers & Multiples?
(i) Factors
A factor of a number is an exact divisor of that number.
Example: 1, 2, 3, and 6 are the factors of 6 as we have already seen in the above example.
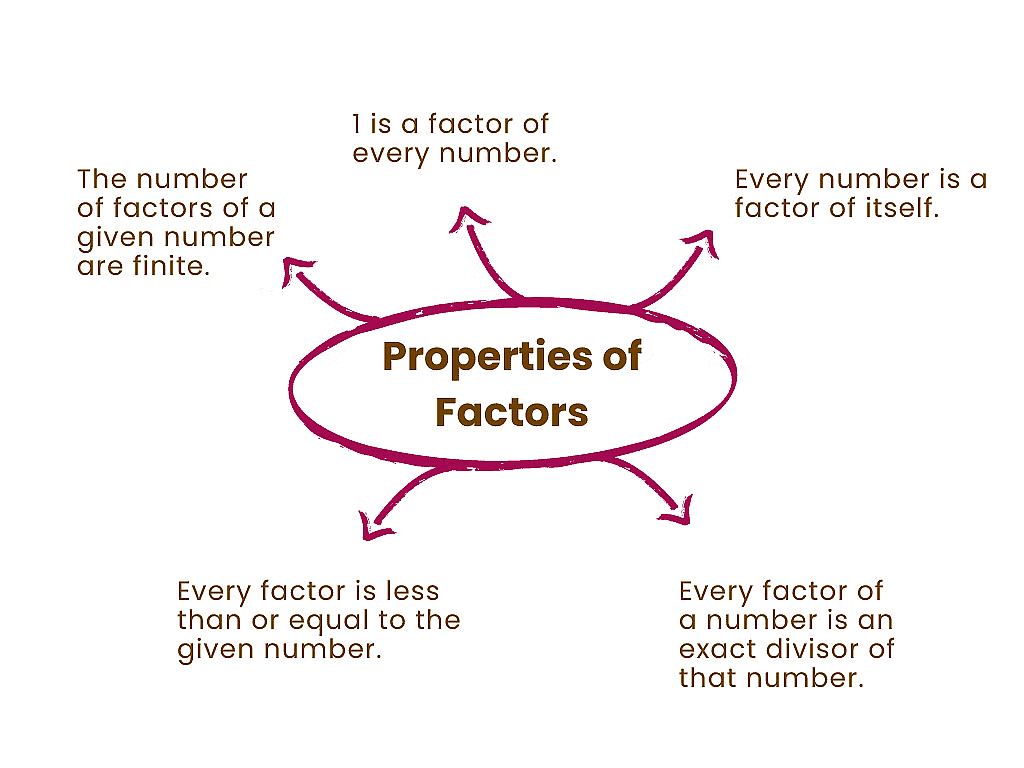
Properties of Factors
Understand more about the properties of Factors and Multiples through this video:
(ii) Perfect Numbers
A perfect number is a positive integer that is equal to the sum of its factors except for the number itself. In other words, perfect numbers are the positive integers that are the sum of its divisors.
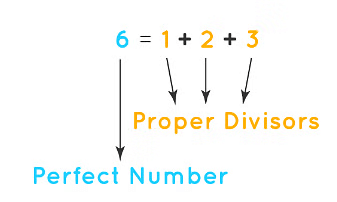
Example: The smallest perfect number is 6, which is the sum of its factors: 1, 2, and 3. It is to be noted that this sum does not include the number itself which is also a factor of itself.
Here are some more examples of Perfect Numbers:
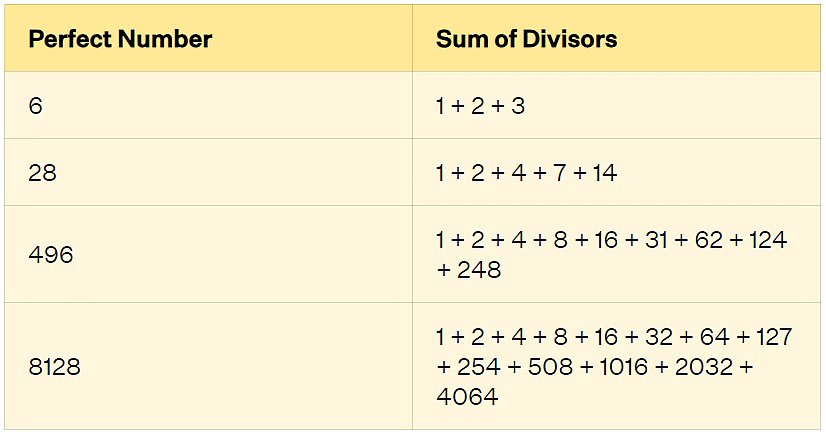 Examples of Perfect Numbers
Examples of Perfect Numbers
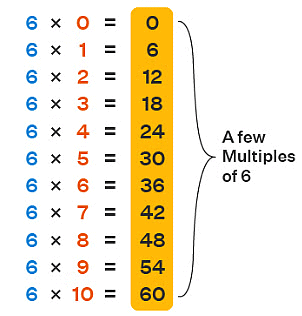
(iii) Multiples
Multiples of a number are those numbers that we get on multiplying the number by any integer.
Example:
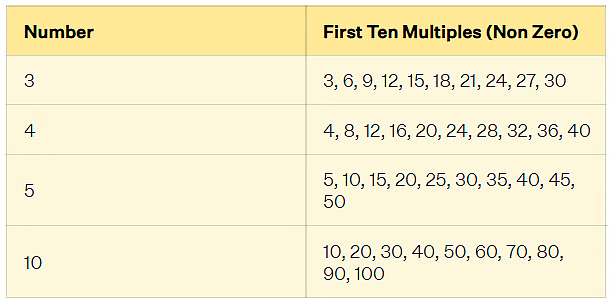
Properties of Multiples
- Every number is a multiple of 1.
As: 7 x 1 = 7,
9 x 1 = 9, - Every number is the multiple of itself.
As: 1 x 7 = 7
1 x 21 = 21 - Zero (0) is a multiple of every number.
As: 0 x 9 = 0,
0 x 11 = 0, - Every multiple except zero is either equal to or greater than any of its factors.
As, multiple of 7 = 7, 14, 28, 35, 77, …………., etc. - The product of two or more factors is the multiple of each factor.
As: 3 x 7 = 21,
So, 21 is the multiple of both 3 and 7.
30 = 2 x 3 x 5,
So, 30 is the multiple of 2, 3 and 5. - There is no end to multiples of a number.
5, 10, 15, 20, 25, …………….., 100, 105, 110, …………………., are the multiples of 5.
Lets us look into some solved example problems of Factors, Perfect Numbers and Multiples:
Q.1. List the first 5 multiples of 5, 8 and 9.
Ans: First 5 multiples of 5 are : 10, 15, 20, 25, 30
First 5 multiples of 8 are : 8, 16, 24, 32, 40
First 5 multiples of 9 are: 9, 18, 27, 36, 45
Q.2. Which number is a factor of 10 among the numbers 3,4,5 & 8?
Ans: 5 is a factor of 10 among the numbers 3,4,5 & 8. If you can multiply two whole numbers to create a third number, those two numbers are factors of the third. 2 x 5 = 10, so 2 and 5 are factors of 10
Q.3. Write all the factors of the following numbers: (a) 24 (b) 15 (c) 21
Ans: (a) Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
(b) Factors of 15: 1, 3, 5, 15
(c) Factors of 21: 1, 3, 7, 21
Q.4. Find all the multiples of 9 upto 100.
Certainly! Here are the multiples of 9 up to 100:
9, 18, 27, 36, 45, 54, 63, 72, 81, 90, 99
Q.5. : Write all the factors of 68.
We note that:
68 = 1 × 68
68 = 2 × 34
68 = 4 × 17
68 = 17 × 4
Stop here, because 4 and 17 have occurred earlier. Thus, all the factors of 68 are 1, 2, 4, 17, 34 and 68
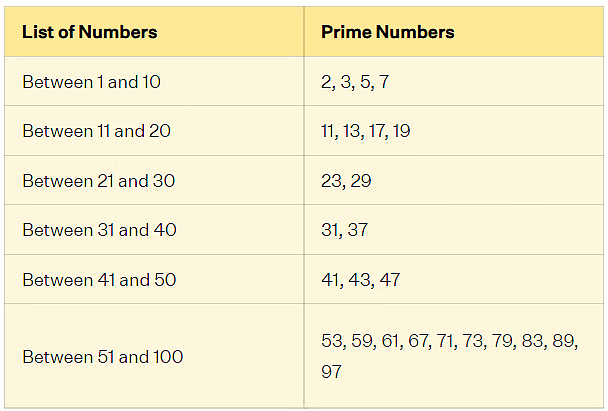
Prime and Composite Numbers
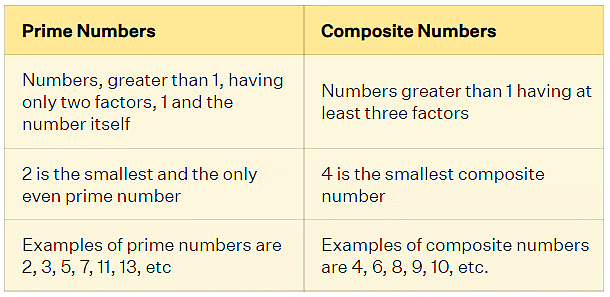
(i) Prime Numbers
Numbers other than 1 whose only factors are 1 and the number itself are called Prime numbers.
Example:
Properties of Prime Numbers
Some of the important properties of prime numbers are given below:
- A prime number is a whole number greater than 1.
- It has exactly two factors, that is, 1 and the number itself.
- There is only one even prime number, that is, 2.
- Any two prime numbers are always co-prime to each other.
- Every number can be expressed as the product of prime numbers.
(ii) Composite Numbers
Numbers having more than two factors are called Composite numbers.
Example: 4, 6, 8, etc.
Prime Numbers Vs Composite Numbers
Lets us look into some solved example problems of Prime and Composite Numbers:
Q.1. Write all the prime numbers less than 15
Ans: Prime numbers are numbers greater than 1 that have no positive divisors other than 1 and itself. Here are the prime numbers less than 15: 2, 3, 5, 7, 11, 13
Q.2. Express the following as the sum of two odd primes.
(a) 44 (b) 36 (c) 24 (d) 18
(a) 44: 19 + 25 = 44 (both 19 and 25 are odd primes)
(b) 36: 17 + 19 = 36 (both 17 and 19 are odd primes)
(c) 24: 5 + 19 = 24 (both 5 and 19 are odd primes)
(d) 18: There is no combination of two odd prime numbers that adds up to 18.
So, for (a), (b), and (c), we can express them as the sum of two odd primes, but for (d), it is not possible.
Q.3. Write down separately the prime and composite numbers less than 20
Here are the prime numbers and composite numbers less than 20:
Prime Numbers:
2, 3, 5, 7, 11, 13, 17, 19
Composite Numbers:
4, 6, 8, 9, 10, 12, 14, 15, 16, 18
Tests for Divisibility of Numbers
Divisibility Tests
A divisibility rule is a method of determining whether a given integer is divisible by a fixed divisor without performing division, usually by examining its digits.
We have divisibility rules for 2, 3, 4, 5, 6, 7, 8, 9, 10, and 11.
- Divisibility tests for 2
If one’s digit of a number is 0,2,4,6 or 8, then the number is divisible by 2.
Example: 12, 34, 56, and 78. - Divisibility tests for 3
A number is divisible by 3 if the sum of its digits is divisible by 3.
Example:
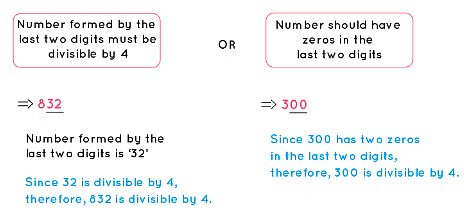
- Divisibility tests for 4
A number with 3 or more digits is divisible by 4 if the number formed by its last two digits (i.e. ones and tens) is divisible by 4.
Example:
- Divisibility tests for 5
If the one’s digit of a number is either 5 or 0, then it is divisible by 5.
Example:
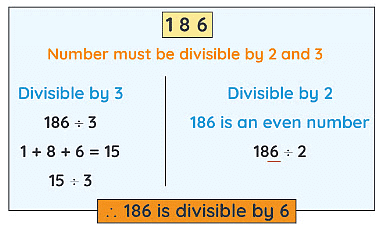
- Divisibility tests for 6
If a number is divisible by 2 and 3 both, then it is divisible by 6 also.
Example: Q. Use the divisibility test to determine whether the number 1258 is divisible by 6.
Q. Use the divisibility test to determine whether the number 1258 is divisible by 6.
Ans: Given number is 1258.
Its units digit is 8, which is divisible by 2. So, 1258 is divisible by 2.
Sum of its digits = 1 + 2 + 5 + 8 = 16, which is not divisible by 3. So, 1258 is not divisible by 3.
Since 1258 is divisible by 2 but not by 3, it is not divisible by 6. - Divisibility tests for 7
Double the last digit and subtract it from the remaining leading cut number. If the result is divisible by 7, then the original number is divisible by 7.
Example:
- Divisibility tests for 8
A number with 4 or more digits is divisible by 8, if the number formed by its last three digits is divisible by 8.
Example:
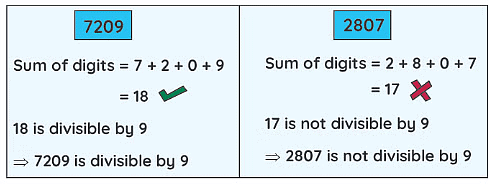
- Divisibility tests for 9
A number is divisible by 9 if the sum of its digits is divisible by 9.
Example:
- Divisibility tests for 10
If one’s digit of a number is 0, then the number is divisible by 10.
Example: 10, 20, 30, and 40. - Divisibility tests for 11
Find difference between sum of digits at odd places (from the right) and sum of digits at even places (from the right) of a number. If the difference is either 0 or divisible by 11, then the number is divisible by 11. Example: 1234321 is divisible by 11 since, (1+3+3+1) – (2+4+2) = 8 – 8 = 0, which is divisible by 11.
Example: 1234321 is divisible by 11 since, (1+3+3+1) – (2+4+2) = 8 – 8 = 0, which is divisible by 11.
Common Factors and Common Multiples
Common Factors
Factors that are common to two or more numbers are known as their common factors.
- The factors of 4 are 1, 2, and 4.
- The factors of 18 are 1, 2, 3, 6, 9, and 18.
- The numbers 1 and 2 are common factors of both 4 and 18.
Common Multiples
Multiples that are common to two numbers are known as common multiples of those numbers. Let us understand with the help of an example.
- Multiples of 3 are 3, 6, 9, 12, 15, 18,….
- Multiples of 5 are 5, 10, 15, 20, 25, 30,…
- Multiples of 6 are 6, 12, 18, 24, 30, 36,…
Therefore, common multiples of 3, 5, and 6 are 30, 60,….
Example 1: Find the common factors of 75, 60 and 210.
Solution: Factors of 75 are 1, 3, 5, 15, 25 and 75.
Factors of 60 are 1, 2, 3, 4, 5, 6, 10, 12, 15, 30 and 60.
Factors of 210 are 1, 2, 3, 5, 6, 7, 10, 14, 15, 21, 30, 35, 42, 70, 105 and 210.
Thus, common factors of 75, 60 and 210 are 1, 3, 5 and 15.
Example 2: Find the common multiples of 3, 4 and 9.
Solution: Multiples of 3 are 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, 48, ....
Multiples of 4 are 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, 44, 48,...
Multiples of 9 are 9, 18, 27, 36, 45, 54, 63, 72, 81, ...
Clearly, common multiples of 3, 4 and 9 are 36, 72, 108,...
Prime Factorization
When a number is expressed as a product of prime numbers, factorization is called prime factorization.
Example: Prime factorization of 36 is 2×2×3×3.

There are two methods to find the prime factors of a number:
1. Prime factorization using a factor tree
We can find the prime factors of 70 in two ways.
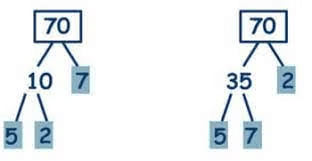
The prime factors of 70 are 2, 5 and 7 in both cases.
2. Repeated Division Method
Find the prime factorization of 64 and 80.
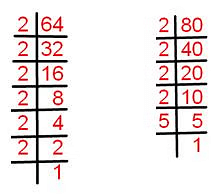
The prime factorization of 64 is 2 × 2 × 2 × 2 × 2 × 2.
The prime factorization of 80 is 2 × 2 × 2 × 2 × 5.
Q. Find the prime factorization of 2520.
Ans: 2520 = 2 × 1260
= 2 × 2 × 630
= 2 × 2 × 2 × 315
= 2 × 2 × 2 × 3 × 105
= 2 × 2 × 2 × 3 × 3 × 35
= 2 × 2 × 2 × 3 × 3 × 5 × 7
Highest Common Factor (HCF)
The Highest Common Factor (HCF) of two or more given numbers is the highest (or greatest) of their common factors. It is also known as the Greatest Common Divisor (GCD).
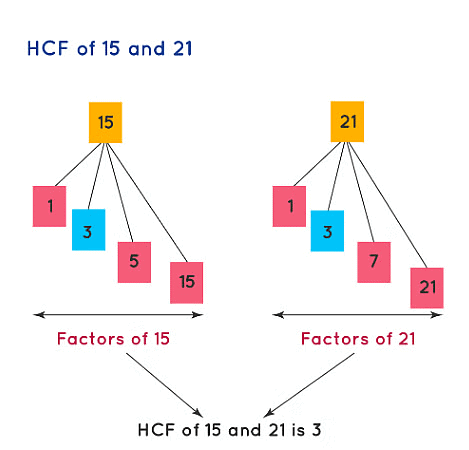
Example 1: Consider two numbers 15 and 21.
Factors of 15 are 1, 3, 5, 15
Factors of 21 are 1, 3, 7, 21
The common factors of 15 and 21 are 1 and 3.
The highest of these is 3. So, 3 is the HCF of 15 and 21.
Example 2: Consider two numbers 12 and 20.
Factors of 12 are 1, 2, 3, 4, 6, and 12.
Factors of 20 are 1, 2, 4, 5, 10, and 20.
The common factors of 12 and 20 are 1, 2, and 4.
The highest of these is 4. So, 4 is the HCF of 12 and 20.
Lowest Common Multiple (LCM)
The Lowest Common Multiple (LCM) of two or more given numbers is the lowest (or smallest or least) of their common multiples.

Example 1: Consider two numbers 8 and 10.
Multiples of 8: 8, 16, 24, 32, 40, 48, 56, 64, 72, 80, ...
Multiples of 10: 10, 20, 30, 40, 50, 60, 70, 80, 90, 100, ...
Common multiples: 40, 80, ...
So, the common multiples of 8 and 10 are 40 and 80. These are the numbers that are multiples of both 8 and 10.
The lowest of these is 40.
So, 40 is the LCM of 8 and 10.
Example 2: Consider two numbers 12 and 20.
Multiples of 12 are 12, 24, 36, 48, 60, 72, 84, 96, 108, 120, …
Multiples of 20 are 20, 40, 60, 80, 100, 120, ……..
The common multiples of 12 and 20 are 60, 120,…
The lowest of these is 60.
So, 60 is the LCM of 12 and 20.
How to Find HCF and LCM?
There are various methods that are used to find the Highest Common Factor (HCF) and Least Common Multiple (LCM) of numbers. The most common methods are:
- Prime factorization method
- Division method
Let us discuss these methods in detail.
1. Prime factorization method
By using the prime factorization method for finding LCM and HCF, we first need to find the prime factors of the given numbers. Then, we can calculate the values of HCF and LCM by following the process explained below.
HCF by Prime Factorization
In order to find the HCF of the given numbers by prime factorization, we find the prime factors of those numbers. After finding the factors, we find the product of the prime factors that are common to each of the given numbers. In some cases, we multiply the common prime factors that have the least or smallest power to obtain the HCF of those numbers. For example, let us find the HCF of 50 and 75 by the prime factorization method.
- The prime factors of 50 = 2 × 5 × 5 or 2 × 52
- The prime factors of 75 = 3 × 5 × 5 or 3 × 52
The common factors of 50 and 75 are 52 or 5 × 5. Thus, HCF of (50, 75) = 25.
LCM by Prime Factorization
To calculate the LCM of any given set of numbers using the prime factorization method, we follow the steps given below:
- Step 1: List the prime factors of the given numbers.
- Step 2: After finding the prime factors, we write them in their exponent form, and then find the product of only those prime factors that have the highest power. The product of these factors with the highest powers is the LCM of the given numbers.
Example: Let us find the LCM of 160 and 90 using prime factorization.
- Step 1: The prime factors of 160 = 2 × 2 × 2 × 2 × 2 × 5. After writing this in the exponent form, we get 25 × 5 and 90 = 2 × 3 × 3 × 5 or 2 × 32 × 5.
- Step 2: Now, if we pick the factors with the highest power, we get 25, 32, and 5. The product of all these prime factors = 25 × 32 × 5 = 1440.
Therefore, LCM of 160 and 90 = 1440.
2. Finding HCF and LCM by Division Method
There are two different ways to apply the division method to find LCM and HCF. Let us learn it one by one.
HCF by Division Method
To find the HCF by division method, follow the steps given below.
- Step 1: First, we need to divide the larger number by the smaller number and check the remainder.
- Step 2: Make the remainder of the above step as the divisor and the divisor of the above step as the dividend and perform the division again.
- Step 3: Continue the division process till the remainder is not equal to 0.
- Step 4: The last divisor will be the HCF of the given numbers.
Let us understand this method using an example.
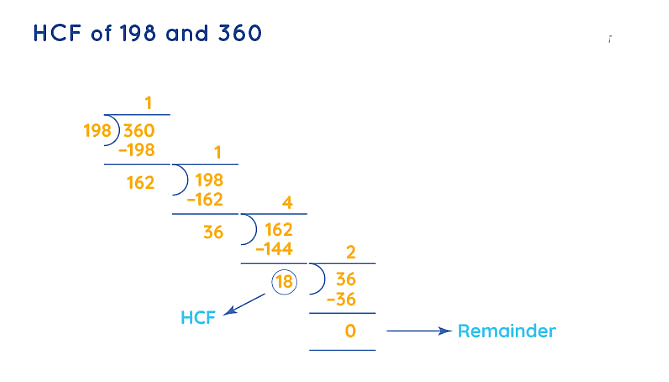
Example: Find the HCF of 198 and 360 using the division method.
Solution: Read out the following steps and relate them with the figure given below.
- Step 1: Divide 360 by 198. The obtained remainder is 162.
- Step 2: Make 162 as the divisor and 198 as the dividend and perform the division again. Here the obtained remainder is 36.
- Step 3: Make 36 as the divisor and 162 as the dividend and perform the division again. Here the obtained remainder is 18.
- Step 4: Make 18 as the divisor and 36 as the dividend and perform the division again. Here the obtained remainder is 0.
- Step 5: The last divisor, 18, is the HCF of 360 and 198.
LCM by Division Method
To find the LCM of numbers by the division method, we divide the numbers with prime numbers and stop the division process when we get only 1 in the final row. Observe the steps given below to find the LCM of the given numbers using the division method. Let us understand the method with the help of an example.
Example: Find the LCM of 7, 8, 14, and 21.
Solution: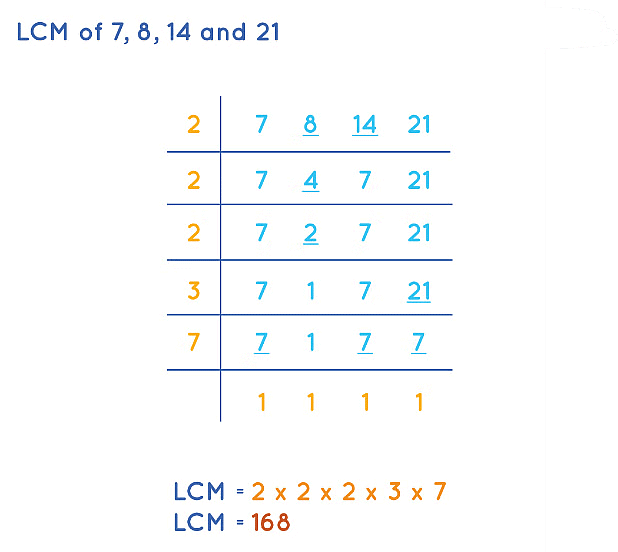
- Step 1: Divide the numbers by the smallest prime number such that the prime number should at least divide 1 of the given numbers. Here, we will divide the numbers 7, 8, 14, 21 by the smallest prime number, i.e., 2.
- Step 2: Write the quotients of the divisible numbers right below the numbers in the next row and copy the other numbers as it is. So, the next row will be written in this way: 7, 4, 7, and 21.
- Step 3: Now, for the next division step consider the above quotients as the new dividends. Repeat the process and write the quotient below the numbers. Here, on dividing 7, 4, 7, 21 by 2, we get the quotients as 7, 2, 7, 21. [Only 4 was divisible by 2 in this step, so we copy the other three numbers as it is in the next row]
- Step 4: Repeat the steps and divide the new dividends till we get 1.
- Step 5: Multiply all the prime numbers on the left-hand side of the bar to get the LCM of the given numbers. This will be 2 × 2 × 2 × 3 × 7 = 168. Therefore, the LCM of 7, 8, 14 and 21 is 168.
Note: Divide the numbers only by prime numbers.
Some Important Questions
Q.1. Two tankers contain 850 litres and 680 litres of kerosene oil respectively. Find the maximum capacity of a container which can measure the kerosene oil of both the tankers when used an exact number of times.
Given, Two tankers of kerosene oil is 850 litres & 680 litres
We need to find, Maximum measuring capacity of container to measure both tankers
Since measuring capacity = HCF of 850 & 680
We will find HCF
Maximum measuring capacity = HCF of 850 & 680
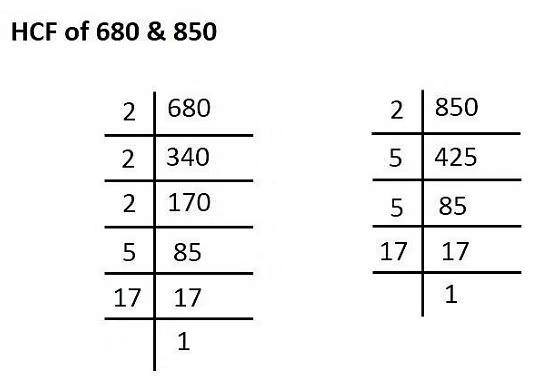
HCF of 680 & 850
Hence, 680 = 2 × 2 × 2 × 5 × 17
850 = 2 × 5 × 5 × 17
∴ HCF = 2 × 5 × 17 = 170
So, Maximum measuring capacity = 170 litres
To fill 850 litres, we measure 5 times (170 X 5 = 850)
To fill 680 litres, we measure 4 times (170 X 4 = 680)
Q.2. In a morning walk three persons step off together, their steps measure 80 cm, 85 cm and 90 cm respectively. What is the minimum distance each should walk so that he can cover the distance in complete steps?
Given: In a morning walk, three persons step off together. Their steps measure 80 cm, 85 cm and 90 cm.
To Find: minimum distance each should walk so that all can cover the same distance in complete steps.
The distance covered by each of them is required to be same as well as minimum. The required distance each should walk would be the L.C.M of the measures of their steps i.e. 80 cm, 85 cm, and 90 cm,
So we have to find the L.C.M of 80 cm, 85 cm, and 90 cm.
80 = 24 x 5
85 = 17 x 5
90 = 2 x 32x 5
L.C.M of 80, 85 and 90 = 24 x 32 x 5 x 17 = 12240 cm
Hence minimum 12240 cm distance each should walk so that all can cover the same distance in complete steps.
Q.3. The sum of the two numbers is 216 and HCF is 27. Find the numbers.
Ans: Let the numbers are 27a and 27b.
27a + 27 b = 216
a + b = 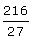 = 8
= 8
Co-primes with sum 8 are (1,7) and (3,5)
Required numbers are (27 x 1 ), (27 x 7) or (27 x 3 ), (27 x 5)
i.e. (27,189) or (81 x 135)
Q.4. Write all the numbers less than 100 which are common multiples of 3 and 4.
To find the common multiples of 3 and 4 less than 100, we need to find numbers that are divisible by both 3 and 4. These are numbers that are multiples of the least common multiple (LCM) of 3 and 4, which is 12. Here are the common multiples of 3 and 4 less than 100:
12, 24, 36, 48, 60, 72, 84, 96
|
75 videos|228 docs|70 tests
|
FAQs on Revision Notes: Playing with Numbers - Mathematics & Pedagogy Paper 2 for CTET & TET Exams - CTET & State TET
| 1. What are factors and how are they related to perfect numbers? |  |
| 2. How are prime and composite numbers different from each other? |  |
| 3. What is prime factorization and why is it important in finding common factors and multiples? |  |
| 4. How can tests for divisibility of numbers help in simplifying calculations involving factors and multiples? |  |
| 5. Why are common factors and common multiples important in mathematics and real-life applications? |  |
|
75 videos|228 docs|70 tests
|

|
Explore Courses for CTET & State TET exam
|

|


 Q. Use the divisibility test to determine whether the number 1258 is divisible by 6.
Q. Use the divisibility test to determine whether the number 1258 is divisible by 6.

 Example: 1234321 is divisible by 11 since, (1+3+3+1) – (2+4+2) = 8 – 8 = 0, which is divisible by 11.
Example: 1234321 is divisible by 11 since, (1+3+3+1) – (2+4+2) = 8 – 8 = 0, which is divisible by 11.
















