Thermodynamic Potentials | Physics for IIT JAM, UGC - NET, CSIR NET PDF Download
Introduction
- Thermodynamic potentials are important functions in statistical mechanics that connect the behavior of tiny particles to the overall properties of larger systems.
- These potentials help us find various thermodynamic quantities and allow us to predict how systems will act under different conditions.
- The main types of thermodynamic potentials include

- Each potential has its own natural variablesand uses, such as:
- Finding equilibrium conditions
- Predicting spontaneous processes
- Understanding phase transitions
Internal Energy
- The total energy in a thermodynamic system is represented as U.
- This energy includes the kinetic and potential energies of all the particles within the system.
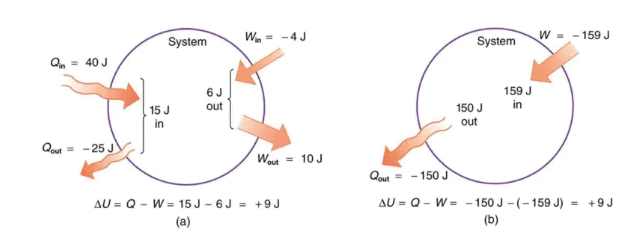
- U is essential for understanding the First Law of Thermodynamics, which is expressed as: dU = δQ - δW

- The energy Udepends on certain extensive properties, such as:
- Entropy
- Volume
- Number of particles
- The natural variablesthat relate to this energy are:
- Entropy (S)
- Volume (V)
Enthalpy
- H = U + PV, where P represents pressure and V stands for volume.
- It measures the total heat content of a system.
- This concept is especially useful for processes that happen at constant pressure, known as isobaric processes.
- Changes in enthalpy (ΔH) show the heat that is either absorbed or released during chemical reactions.
- Two important variables related to enthalpy are entropy (S) and pressure (P).
Helmholtz free energy
- A is expressed as A = U - TS, where T stands for temperature and S means entropy.
- This equation shows the useful work that can be obtained from a closed system when the temperature is constant.
- The value of A is at its lowest point when the system is in equilibrium, especially in situations with a fixed temperature and volume.
- The equation helps in figuring out the direction of spontaneous processes under conditions of constant temperature and constant volume.
- The natural variables in this context are temperature (T) and volume (V).
Gibbs free energy
- G is defined as G = H - TS or G = U + PV - TS
- G measures the maximum work that can be obtained from a system in a reversible way.
- G is at its lowest point when a system is in equilibrium at constant temperature and pressure.
- It is important for understanding chemical equilibrium and whether reactions will occur spontaneously.
- The key variables that affect G are temperature (T) and pressure (P).
Mathematical formulations
Legendre transformations
- Mathematical method used to change between different thermodynamic potentials.
- Facilitates the conversion between conjugate variables, which include both extensive and intensive properties.
- Maintains the information content while altering the independent variables.
- General formula:
- F(x,y) = f(x,y) - y ∂f/∂y
- This method allows for the derivation of new potentials from existing ones, such as transforming U (internal energy) to H (enthalpy) and A (Helmholtz free energy) to G (Gibbs free energy).
Partial derivatives relationships
Various thermodynamic properties can be linked through mathematical equations.
- Fundamental Equation:
- dU: This represents the change in internal energy.
- T: This is the temperature.
- dS: This indicates the change in entropy.
- P: This refers to pressure.
- dV: This signifies the change in volume.
- dN: This denotes the change in the number of particles.
- The fundamental equation can be expressed as: dU = TdS - PdV + μdN
- Derived Relationships:
- (∂U/∂S)V,N = T
- (∂U/∂V)S,N = -P
- (∂U/∂N)S,V = μ
These equations allow for the calculation of one thermodynamic quantity based on measurements of other quantities.
Maxwell Relations
F = F ( x , y ) and if it is perfect differential then d F = M dx + Ndy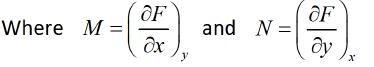 then, M and N will satisfy the condition
then, M and N will satisfy the condition 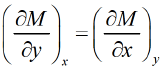 Maxwell relations are relationship between two derivatives of thermodynamic variables, and energy due to the equivalence of potential second derivative under a change of operation order
Maxwell relations are relationship between two derivatives of thermodynamic variables, and energy due to the equivalence of potential second derivative under a change of operation order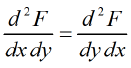 Where F is thermodynamic potentialand x and y are two of its natural independent variables.
Where F is thermodynamic potentialand x and y are two of its natural independent variables.Maxwell relations are extremely important for two reasons:
- First they show us that derivative of thermodynamic parameters are not all independent. This can serve as a consistency check in both experiments and in theoretical analysis.
- Maxwell relations provide a method for expressing some derivative in other ways. This enables as to connect difficult to measure quantities to those which are readily accessible experimentally.
The measurement of entropy and chemical potential can not be directly measurable in lab but with the help of Maxwell relation there thermodynamic property can be determine theoretically.
For Maxwell relation,
Let us Legendre the independent variable as x , and y such that
U = U(x,y), S = S(x, y) V = V(x, y)
So 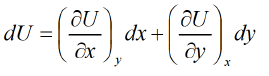
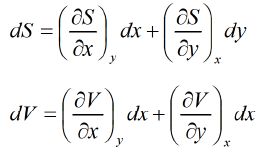 From first law of thermodynamic
From first law of thermodynamic
dU = TdS - PdV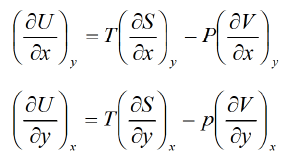 Hence U,V, and S are perfect differential.
Hence U,V, and S are perfect differential.
Then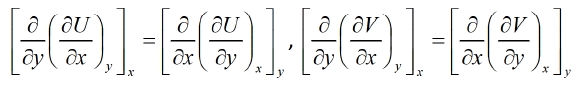
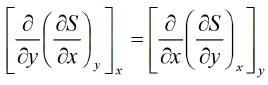
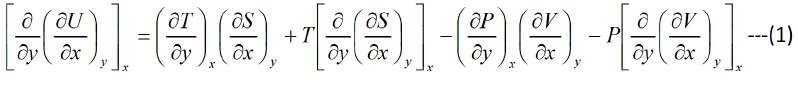
Similarly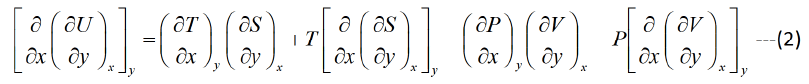
Equating equation (1) and (2),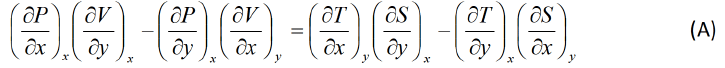
Maxwell first relation:- Put x = T , y = V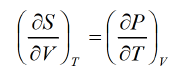
Maxwell Second Relation:- Put x = T ,y = P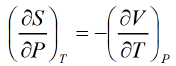 Maxwell Third Relation:- Put x = s , y = v
Maxwell Third Relation:- Put x = s , y = v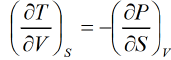
Maxwell Fourth Relation:- Put x = S, y = P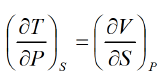
Thermodynamic potential is a scalar function used to represent the thermodynamic state of system. The concept of thermodynamic potentials was introduced by Pierre Duhem in 1886.
One main thermodynamic potential that has a physical interpretation is the internal energy.
It is energy of configuration of a given system of conservative forces. Expression for all other thermodynamic energy potentials are drivable via Legendre transformation.
Different Types of Thermodynamic Potential and Maxwell Relation
Thermodynamic potentials are different form of energy which can be used in different thermodynamic process. Thermodynamic potentials are path independent variables so they are perfect differential.
If F is unique thermodynamic potential defined by variables x and y as F = F ( x , y ) and if it is perfect differential then dF = Mdx + Ndy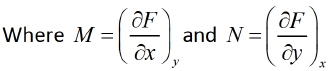 then M and N will satisfy the condition
then M and N will satisfy the condition
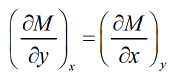
Internal Energy
U and second from the first laws of thermodynamics dU = TdS - PdVFrom Legendre transformationfrom given relation one can derive Maxwell relation
Enthalpy
(H) the enthalpy is defined as H = U + PV dH = dU + PdV + VdP from Laws of thermodynamics,TdS = dU + PdV & dH = TdS + VdP from Legendre transformation,
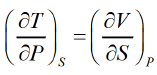
Helmholtz Free Energy
(F) the Helmholtz free energy is defined F = U - T S
dF = dU - TdS - SdT
From laws of thermodynamics, d U = T d S - P d V
dF = TdS - PdV - TdS - SdT
dF = - P d V - SdT
From Legendre transformation
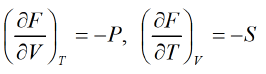
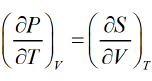
TdS - PdV + PdV + VdP - TdS - SdT
dG = VdP - SdT
from Legendre transformation
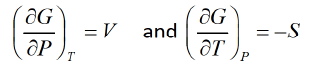
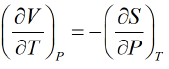
- The Gibbs free energy is Maximum amount of nonexpanding work that can be exacted from a closed system.
- The maximum will activated when the system is in reversible process.
- Gibbs free energy is also treated as chemical potential.
- In thermodynamics, chemical potential, as partial molar free energy, is a form of potential energy that can be absorbed or relived during a chemical reaction.
- The chemical potential of a species in the minute can be defined the slope of the energy at system with respect to a change in the no of moles.

where 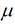 is chemical potential, G is Gibbs energy and N is no of molecules
is chemical potential, G is Gibbs energy and N is no of molecules
Example 1: Prove that internal energy U is given by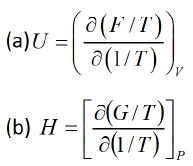 Solution: (a) F = U-TS =U = F + TS
Solution: (a) F = U-TS =U = F + TS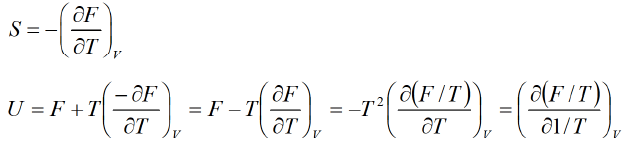
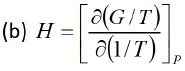 G = H-TS
G = H-TS
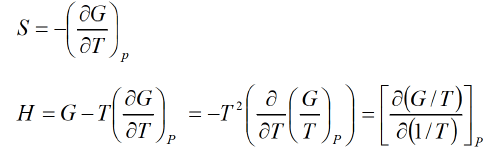 Application of Maxwell Relation
Application of Maxwell Relation
First T - dS equation
Let T, and \/are independent variable S = S (T, V)
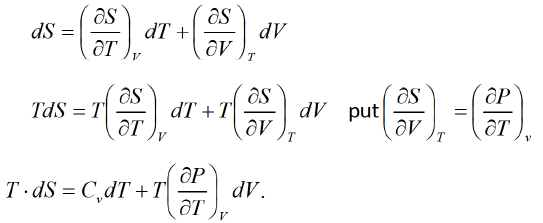
Second T - dS Equation
Let T and P are independent variable S = S(T, P).
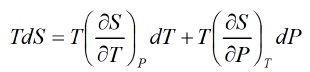 From Maxwell relation
From Maxwell relation
 Third T-dS Equation
Third T-dS Equation
Let P , V are independent variable S = S (P ,V )
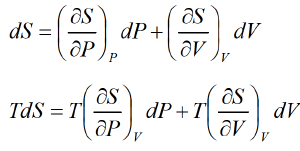
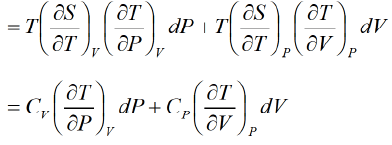
The First Energy Equation
Let T and V are independent variable U = U(T, V)
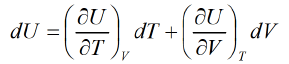 From first law of thermodynamics,
From first law of thermodynamics,
dU = TdS - PdV
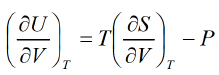 Using Maxwell relation
Using Maxwell relation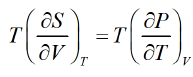
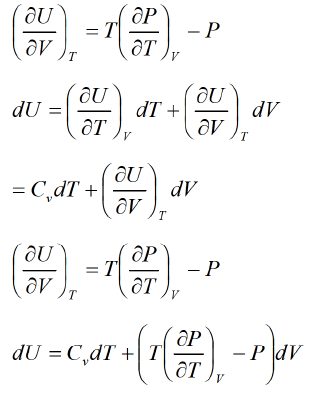
Second Energy Equation
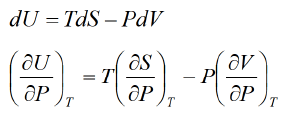
Using Maxwell relation,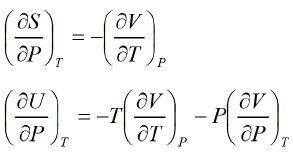 This is popularly known as second energy equation.
This is popularly known as second energy equation.
Application of second energy equation
If U is function of independent variable of T and P.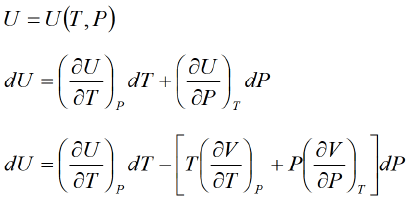
Example 2: From relation dU = TdS - P d V
Derive Maxwell relation 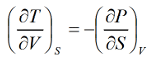
Solution:dU = TdS - PdV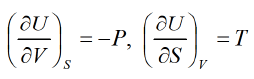 Hence U is exact differential
Hence U is exact differential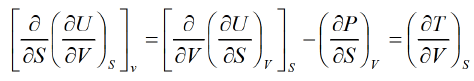
Example 3: A real gas which obey van der Waal's equation of state are kept in container which has temperature T0 and volume V0. if volume of container changes to V such that temperature of gas become T what is change in entropy?
Solution: Assume Cv is specific heat of constant volume
For van der Waal's gas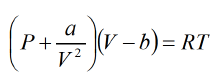 From first T -dS equation
From first T -dS equation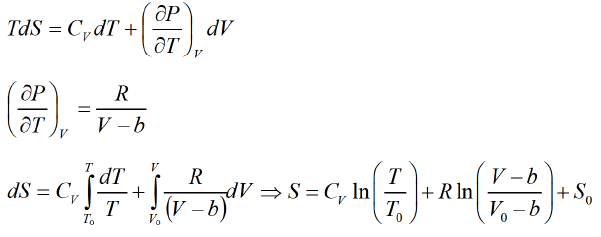 where S0 is integration constant.
where S0 is integration constant.
Some important FAQs are as follows:
Q1. Explain the significance of Legendre transformations in deriving Maxwell relations.
Sol.Legendre transformations allow the redefinition of thermodynamic potentials by replacing natural variables (like S and V) with their conjugates (like T and P). For instance:
dG = −S dT + V dP
This simplifies the derivation of Maxwell relations and enables the conversion of thermodynamic quantities into measurable forms.
Q2. What is the physical significance of chemical potential (μ) in thermodynamics?
Sol.Chemical potential (μ) represents the change in Gibbs free energy (G) with respect to the number of particles (N):
μ = (∂G / ∂N)T,P
It indicates the potential energy available for particle exchange during chemical reactions and phase transitions.
Q3. A system undergoes a reversible isothermal process. What happens to the Helmholtz free energy (A)?
Sol. During a reversible isothermal process (T = constant), the change in Helmholtz free energy (ΔA) represents the maximum work done by the system:
ΔA = −Wmax
At equilibrium, A is minimized.
Q4. Explain the relationship between enthalpy (H) and entropy (S) during an isobaric process.
Sol. During an isobaric process (P=constant), the change in enthalpy (ΔH) is equal to the heat absorbed or released:
ΔH = TΔS
Here, ΔS is the change in entropy. If ΔH > 0, the process is endothermic; if ΔH < 0, it is exothermic.
Q5. Why is Gibbs free energy important in chemical reactions?
Sol. Gibbs free energy G = H − T S indicates the maximum non-expanding work obtainable from a system at constant temperature and pressure. It predicts:
- Spontaneity: A reaction is spontaneous if ΔG < 0.
- Equilibrium: At equilibrium, ΔG = 0.
- Phase Transitions: Helps determine equilibrium conditions during phase changes.


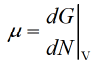
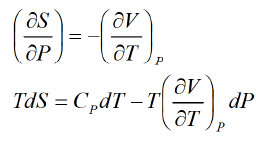 Third T-dS Equation
Third T-dS Equation















