Tips and Tricks for Number Series | Logical Reasoning (LR) and Data Interpretation (DI) - CAT PDF Download
Number Series Tips, Tricks and Shortcuts
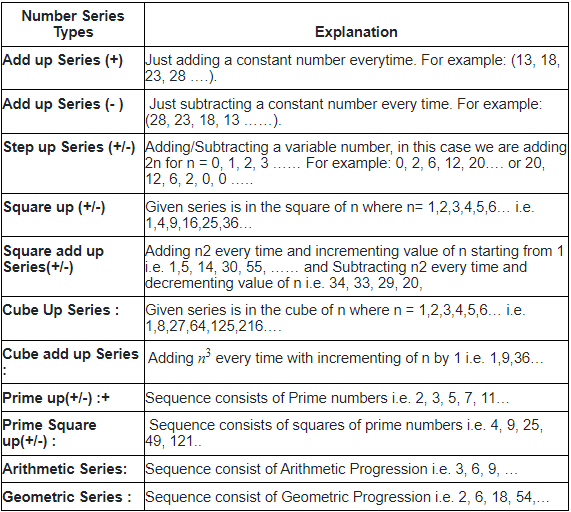
1. Tips and Trick– Perfect Square Series
Comprising a sequence of numbers that are perfect squares arranged in a specific order, the series has one number omitted. Our task is to identify the underlying pattern in the series and fill in the blank by determining that missing number.
Q1: 100,121,144,__, 196
Sol: This series consists of a perfect square of consecutive numbers 10, 11, 12, 13
Hence 169 will come in the blank.
2. Tips and Trick – Perfect Cube Series
It comprises a sequence of numbers arranged in a specific order, where each number is the cube of a given value, and the sequence involves continuously adding these cubic values.
Q2: 9,64, 125, __, 343
Sol: This series consists of a series of numbers with perfect cubes the is (3 x 3 x 3), (4 x 4 x 4), (5 x 5 x 5), (6 x 6 x 6), (7 x 7 x 7)
Hence 216 will be coming in the blank, as the series is following a trend of cubes of numbers in sequential order.
3. Tips and Tricks and Shortcuts- Ration Series
This series is composed of numbers organized in a sequential manner, adhering to a specific trend, whether it be an increase or decrease. Our objective is to identify this trend (which may involve *, /, +, or -) for each number in the series with respect to a constant value. This entails determining the proportional difference between consecutive numbers in the series.
Q3: 3, 6, 9, 12, __, 18, 21
Sol: Here the series is following an increasing trend in which three is added to each number of the series.
3
6 (3+3)
9 (6+3)
12 (9+3)
15 (12+3)
18 (15+3)
21 (18+3)
4. Tips and Tricks and Shortcuts – Arithmetic series
In sequences of this nature, each number is derived by either adding or subtracting a constant number from each term.
The formula for an arithmetic sequence (AS) is given by {a, a+d, a+2d, ...}, where:
- a represents the first term of the series,
- d is the common difference between consecutive terms.
Q4: 3, 6, 9 , 12
Sol: Here a = 3(first term of the series)
d = 3
Hence we get:
3 + 3 = 6
6 + 3 = 9
9 + 3 = 12
12 + 3 = 15
5. Tips and Tricks and Shortcuts – Geometric series
In sequences of this type, each number is obtained by multiplying or dividing each term by a constant number.
The formula for a geometric sequence (GS) is represented as {a, ar, ar2, ar3, ...}, where:
- a denotes the first term of the series,
- r is the factor or difference between terms, commonly known as the common ratio.
Q5: 1, 2, 4, 8, 16, 32
Sol: Here a = 1 (first term of the series)
r = 2 (a standard number that is multiplied with the consecutive number of the series)
Hence we get:
1
1 x 2
1 x 22
1 x 23, ….)
6. Tips and Tricks and Shortcuts – Mixed series
In these series, when computing the difference, two steps may be required to obtain the next consecutive number in the series. Therefore, we must adhere to the same pattern to determine subsequent numbers in the series.
Q6: 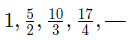
(a) 26/5
(b) 24/5
(c) 21/5
(d) 5
Ans: (a)
Sol: Here we’ve to observe the trend that this series is following, as each term is divided by a specific number, i.e., 1 then 2 then3 then 4 and so on. Hence we can make out that the denominator must be 5.
Now comes the numerator, where the 1’st number is 1.
Then comes 5, now how can we get 5 from the term number 2. It can come by 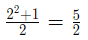
Next comes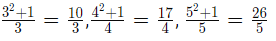
|
88 videos|119 docs|91 tests
|
FAQs on Tips and Tricks for Number Series - Logical Reasoning (LR) and Data Interpretation (DI) - CAT
| 1. What are some tips and tricks for solving number series involving perfect squares? |  |
| 2. How can I solve number series involving perfect cubes? |  |
| 3. What shortcuts can I use to solve ratio series in number sequences? |  |
| 4. How can I approach arithmetic series in number sequences? |  |
| 5. What tips and tricks can I use for solving geometric series in number sequences? |  |
|
88 videos|119 docs|91 tests
|

|
Explore Courses for CAT exam
|

|

















