Heron’s Formula Class 9 Worksheet Maths Chapter 10
Multiple Choice Questions
Q1: The difference between sides at right angles in a right-angled triangle is 14 cm. The area of the triangle is 120 cm2. The perimeter of the triangle is
(a) 80
(b) 45
(c) 60
(d) 64
Q2: ABCD is a trapezium with AB = 10cm, AD = 5 cm, BC = 4 cm and DC = 7 cm. Find the area of the ABCD?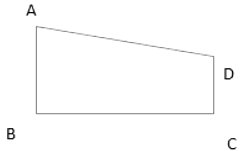 (a) 34 cm2
(a) 34 cm2
(b) 28cm2
(c) 20 cm2
(d) None of these
Q3: Find the area and perimeter of the right angle triangle whose hypotenuse is 5 cm and Base is 4 cm.
(a) 6 cm2 ,12 cm
(b) 12 cm2 ,14 cm
(c) 4 cm2, 6 cm
(d) 12 cm2 ,6 cm
Q4: In an isosceles triangle ABC with AB = AC = 13 cm. D is mid point on BC. Also BC=10 cm. Which of the following is true?
(a) Area of Triangle ABD and ADC are equal
(b) Area of triangle ABD is 30 cm2
(c) Area of triangle ABC is 60 cm2
(d) All the above
Q5: A triangle and a parallelogram have the same base and the same area. The sides of the triangle are 26 cm and 30 cm and parallelogram stands on the base 28 cm. Calculate the height of the parallelogram.
(a) 12 cm
(b) 14 cm
(c) 10cm
(d) 13 cm
True/False
Q1: Heron formula for area of triangle is not valid of all triangles.
Q2: If each side of the triangles is tripled, the area will becomes 9 times.
Q3: Base and corresponding altitude of the parallelogram are 8 and 5 cm respectively. Area of parallelogram is 40 cm2
Q4: If each side of triangle is doubled, the perimeter will become 4 times.
Q5: If p is the perimeter of the triangle of sides a,b,c ,the area of triangle is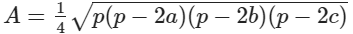
Q6: When two triangles are congruent, there areas are same.
Q7: Heron’s belongs to America.
Q8: If the side of the equilateral triangle is a rational number, the area would always be irrational number.
Concepts Questions
Q1: Find the area of a triangle having sides as a=5 cm ,b=4 cm,c=3 cm.
Q2: Find the area of an equilateral triangle having side a=2 cm
Q3: Find the area of a right angle triangle have base=4 cm and Height =3 cm
Q4: Find the area of a Parallelogram whose two sides are 10 cm and 16 cm and diagonal is 14 cm.
Q5: Rhombus of diagonals to 10 and 24 cm. Find its area.
The solutions of the worksheet "Worksheet Solutions: Heron’s Formula"
|
44 videos|412 docs|54 tests
|
FAQs on Heron’s Formula Class 9 Worksheet Maths Chapter 10
| 1. What is Heron's Formula and how is it used to find the area of a triangle? |  |
| 2. Can Heron's Formula be used for all types of triangles? |  |
| 3. How can Heron's Formula be derived or proved? |  |
| 4. Can Heron's Formula be used to find the area of a triangle if only the lengths of two sides and the included angle are given? |  |
| 5. Are there any limitations or restrictions to using Heron's Formula? |  |

|
Explore Courses for Class 9 exam
|

|


















