Linear Equations in Two Variables Class 9 Worksheet Maths Chapter 4

Clear all your Class 9 doubts with EduRev Join for Free

Join for Free

Q1. Write each of the following is an equation in two variables:
(i) x = –3
(ii)y = 2
(iii) 2x = 3
(iv) 2y = 5

Sol. 

(i)Given equation, x = -3

The above equation can be written in two variables as,

x + 0.y + 3 = 0

(ii) Given equation, y =2

The above equation can be written in two variables as,

0.x + y – 2 = 0

(iii) Given equation, 2x =3

The above equation can be written in two variables as,

2x + 0.y – 3 = 0

(iv) Given equation, 2y =5

The above equation can be written in two variables as,

2y-5= 0

(0)x + 2y- 5= 0

Q2. Write each of the following equations in the form ax + by + c = 0 and also write the values of a, b and c in each case:
(i) 2x + 3y = 3.47
(ii) x – 9 = √3 y
(iii) 4 = 5x – 8y
(iv) y = 2x

Sol. 

(i) 2x + 3y – 3.47 = 0; a = 2, b = 3 and c = –3.47
(ii) x – √3y – 9 = 0; a = 1, b = - √3 and c = –9
(iii) –5x + 8y + 4 = 0; a = –5, b = 8 and c = 4
(iv) –2x + y + 0 = 0; a = –2, b = 1 and c = 0

Q3. (a) Is (3, 2) a solution of 2x + 3y = 12?
(b) Is (1, 4) a solution of 2x + 3y = 12?
(c) Is 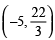 a solution of 2x + 3y = 12?
(d) Is 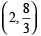 a solution of 2x + 3y = 12?

Sol.

(a) Yes, 

2(3)+ 3(2)= 6+6 =12

(b) No, 

2(1)+ 3(4)= 2+12 =14

(c) Yes, 

2(-5)+ 3(22/3)= -10+ 22 =12

(d) Yes, 

2(2)+ 3(8/3)= 4+8 =12

Q4. Find four different solutions of the equation x + 2y = 6.

Sol. To find four different solutions of the equation $x+2y=6x\; +\; 2y\; =\; 6$x+2y=6, we can choose different values for $yy$y and solve for $xx$x.

1. $y=0y\; =\; 0$ y=0

   $x+2\cdot 0=6x=6x\; +\; 2\; \backslash cdot\; 0\; =\; 6\; \backslash \backslash \; x\; =\; 6$x+2⋅0=6x=6

   So, one solution is $(6,0)$.

2. $y=\mathrm{1 }$y = 1

   $x+2\cdot 1=6x+2=6x=4x\; +\; 2\; \backslash cdot\; 1\; =\; 6\; \backslash \backslash \; x\; +\; 2\; =\; 6\; \backslash \backslash \; x\; =\; 4$x+2⋅1=6x+2=6x=4

   Another solution is $(4,1)(4,\; 1)$.

3. $y=2y\; =\; 2$ y=2

   $x+2\cdot 2=6x+4=6x=2x\; +\; 2\; \backslash cdot\; 2\; =\; 6\; \backslash \backslash \; x\; +\; 4\; =\; 6\; \backslash \backslash \; x\; =\; 2$x+2⋅2=6x+4=6x=2

   Another solution is $(2,2)(2,\; 2)$.

4. $y=3y\; =\; 3$ y=3

   $x+2\cdot 3=6x+6=6x=0x\; +\; 2\; \backslash cdot\; 3\; =\; 6\; \backslash \backslash \; x\; +\; 6\; =\; 6\; \backslash \backslash \; x\; =\; 0$x+2⋅3=6x+6=6x=0

   Another solution is $(0,3)(0,\; 3)$

Therefore, the four different solutions of the equation $x+2y=6x\; +\; 2y\; =\; 6$x+2y=6 are $(6,0),(4,1),(2,2),(0,3)(6,\; 0),\; (4,\; 1),\; (2,\; 2),\; (0,\; 3)$(6,0),(4,1),(2,2),(0,3).

Q5. Find two solutions for each of the following equations:
(i) 4x + 3y = 12
(ii) 2x + 5y = 0
(iii) 3y + 4 = 0

Sol. 

1) 4x+3y=12

for y=4

4x+12=12 x=0

for y=0

4x+0=12 x=3

(0,4) & (3,0) are 2 solution

2) 2x+5y=0

for y=−2

2x−10=0 x=5

for y=−4

2x−20=0 x=10

(5,−2) and (10,−4) are 2 solutions

3) 3y+4=0

y=−4/3 is only solution

Q6. Find the value of k such that x = 2 and y = 1 is a solution of the linear equation 2x – ky + 7 = 8

Sol. We can find the value of k by substituting the values of x and y in the given equation.

By substituting the values of x = 2 and y = 1 in the given equation

2x – ky + 7 = 8

⇒ 2(2) – k(1) + 7 = 8

⇒ 4- k+ 7=8

⇒ -k=8-11

k=3

Therefore, the value of k is 3.

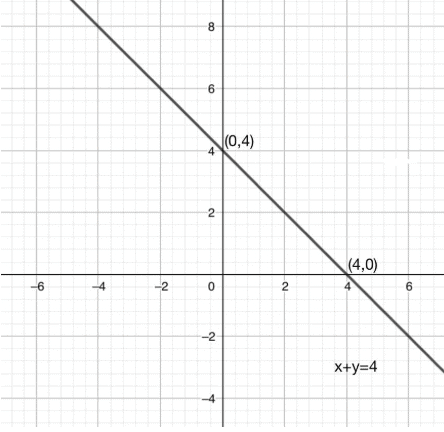
Q7. Draw the graph of  y+x = 4.

Sol. Let x be 0 = (0,4)

Let y be 0 = (4,0)

Q8. Force applied on a body is directly proportional to the acceleration produced in the body.
Write an equation to express this situation and plot the graph of the equation.

Sol. Given that, the force (F) is directly proportional to the acceleration (a).

i.e., F∝a

⇒F=ma [where, ,m=arbitrary constant and take value 6 kg of mass ]

∴                           F=6a

(i) If a=5m/s2, then from Eq. (i), we get

F=6×5=30N

(ii) If a=6m/s2, then from Eq. (i), we get

F=6×6=36N

Here, we find two points A (5, 30) and B (6, 36). So draw the graph by plotting the points and joining the line AB. 

Q9. For each of the graph given in the following figure select the equation whose graph it is from the choices given below:
(i) x + y = 0

(ii) x – y = 0

(iii) 2x = y
(iv) y = 2x + 1 

(i) x + y = 0

(ii) x – y = 0
(iii) y = 2x + 4
(iv) y = x – 4 

(i) x + y = 0

(ii) x – y = 0
(iii) y = 2x + 1
(iv) y = 2x – 4 

(i) x + y = 0
(ii) x – y = 0
(iii) 2x + y = –4
(iv) 2x + y = 4

Sol.
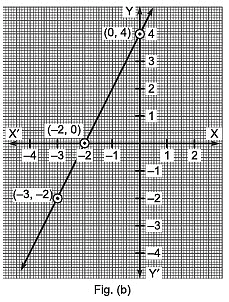
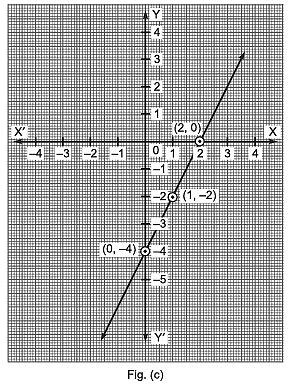
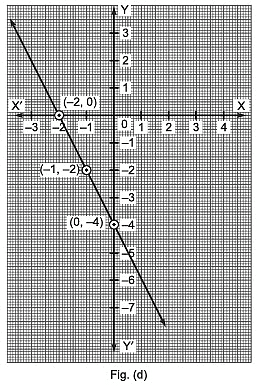
(a) x – y = 0
(b) y = 2x + 4
(c) y = 2x – 4
(d) 2x + y = –4

Q10. Which of the following is not a linear equation in two variables?
(i) px + qy + c = 0
(ii) ax² + bx + c = 0
(iii) 3x + 2y = 5

Sol. 

(ii) ax² + bx + c = 0 

(ii) is not a linear equation because it consists x² in it. Linear equation will not contain any exponent to variables

Q11. One of the solutions of the linear equation 4x – 3y + 6 = 0 is
(i) (3, 2)
(ii) (–3, 2)
(iii) (–3, –2)

Sol.  Option (iii) –3, –2 

Q12. lx + my + c = 0 is a linear equation in x and y. For which of the following, the ordered pair (p, q) satisfies it:
(i) lp + mq + c = 0
(ii) y = 0
(iii) x + y = 0
(iv) x = y

Sol. 

 $lp+mq+c=0lp\; +\; mq\; +\; c\; =\; 0$lp+mq+c=0

To check if $(p,q)(p,\; q)$(p,q) satisfies the equation: $l\cdot p+m\cdot q+c=0l\; \backslash cdot\; p\; +\; m\; \backslash cdot\; q\; +\; c\; =\; 0$l⋅p+m⋅q+c=0

This matches the form of the linear equation $lx+my+c=0lx\; +\; my\; +\; c\; =\; 0$lx+my+c=0, so statement (i) is correct.

Q13. What is the equation of the x-axis? 

Sol. The x-axis is the horizontal line where $y=0y\; =\; 0$y=0.

Equation of the x-axis: $\backslash boxed\{y\; =\; 0\}$y=0.

Q14. What is the equation of the y-axis? 

Sol. The y-axis is the vertical line where $x=0x\; =\; 0$x=0.

Equation of the y-axis: $\backslash boxed\{x$x=0.

Q15. How many solutions do a linear equation in two variables x and y have?

Sol. 

A linear equation in two variables will have infinite solutions