Fractions (Exercise 2.1) RD Sharma Solutions | Mathematics (Maths) Class 7 (Old NCERT) PDF Download
Q.1. Compare the following fractions by using the symbol > or < or =:
(i)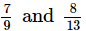
(ii)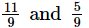
(iii)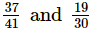
(iv)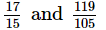
Ans: First, we need to find the LCM of denominators in each case. After that, we will equate the denominators in order to compare the two fractions.
(i) LCM of 9 and 13 is 117.
Now make both fraction equivalent with denominator as 117
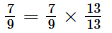
⇒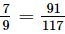
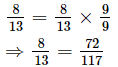
we know 91 > 72
⇒
⇒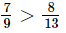
(ii) both fraction have same denominator as 9
we know 11 > 5
⇒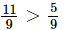
(iii) LCM of 41 and 30 is 1230
Now convert both fraction to their equivalent fractions with denominator as 1230
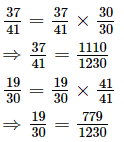
we know
1110 > 779
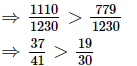
(iv) LCM of 15 and 105 is 105.
Now convert fraction to its equivalent fractions with denominator as 105
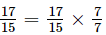
⇒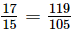
Q.2. Arrange the following fractions in ascending order:
(i)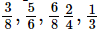
(ii)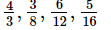
Ans:
(i)
LCM of the denominators 8, 6, 4 and 3 is 24.
Now, convert all fractions into their equivalent fractions with denominator 24.
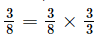
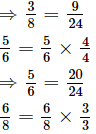
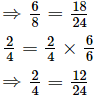
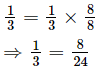
We know:
8 < 9 < 12 < 18 < 20
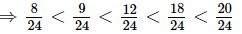
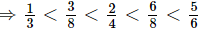
(ii)
LCM of the denominators 8, 12, 16 and 3 is 48.
Now, convert all fractions into their equivalent fractions with denominator 48.
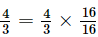
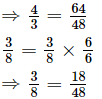
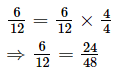
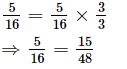
we know
15 < 18 < 24 < 64
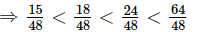
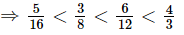
Q.3. Arrange the following fractions in descending order:
(i)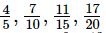
(ii)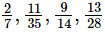
Ans:
(i)
LCM of the denominators 5, 10, 15 and 20 is 60.
Now, convert all fractions to their equivalent fractions with denominator 60.
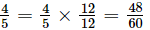
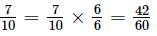
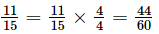
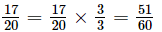
We know:
51 > 48 > 44 > 42
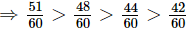
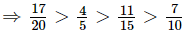
(ii)
LCM of the denominators 7, 35, 14 and 28 is 140.
Now, convert all fractions to their equivalent fractions with denominator 140.
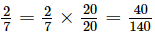
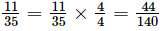

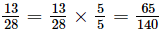
We know:
90 > 65 > 44 > 40
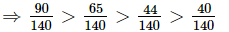
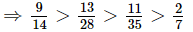
Q.4. Write five equivalent fractions of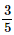 .
.
Ans: Five equivalent fractions of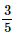 are:
are:
(i)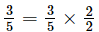
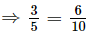
(ii)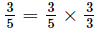
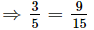
(iii)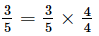
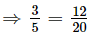
(iv)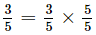
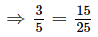
(v)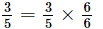
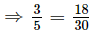
Q.5. Find the sum:
(i)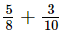
(ii)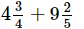
(iii)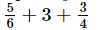
(iv)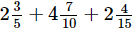
Ans:
(i)
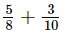
LCM of 8,10 is 40.
⇒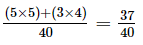
(ii)
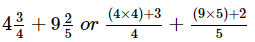
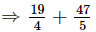
LCM of 5,4 is 20.
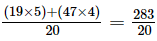
(iii)
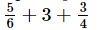
LCM of 6,4 is 24.
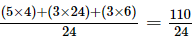
(iv)
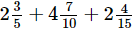 or
or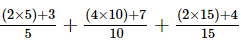
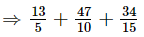
LCM of 15,10 and 5 is 30.
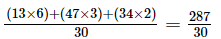
Q.6. Find the difference of
(i)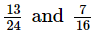
(ii)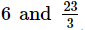
(iii)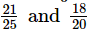
(iv)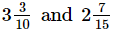
Ans:
(i)
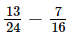
LCM of 24 and 16 is 48.
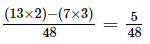
(ii)
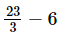
LCM of 3 and 1 is 3.
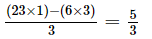
(iii)
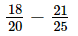
LCM of 20,25 is 100.
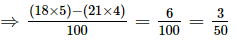
(iv)
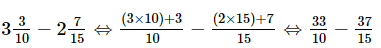
LCM of 10 and 15 is 30.
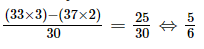
Q.7. Find the difference:
(i)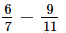
(ii)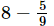
(iii)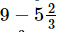
(iv)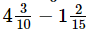
Ans:
(i)
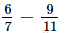
LCM of 7 and 11 is 77.
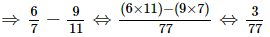
(ii)
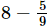
LCM of 1 and 9 is 9.
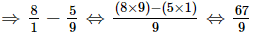
(iii)
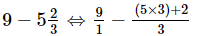
LCM of 1 and 3 is 3.
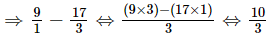
(iv)
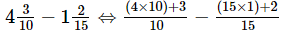
LCM of 10 and 15 is 30.
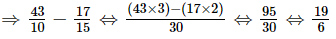
Q.8. Simplify:
(i)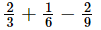
(ii)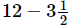
(iii)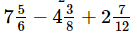
Ans:
(i)
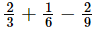
LCM of 3.6 and 9 is 18.
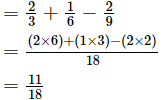
(ii)
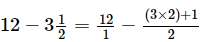
LCM of 2 and 1 is 2.
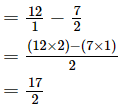
(iii)
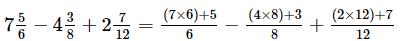
LCM of 6,8 and 12 is 24.
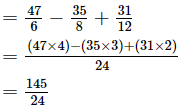
Q.9. What should be added to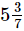 to get 12?
to get 12?
Ans:
Let x be the required fraction.
According to the question:
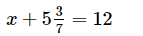
⇒ 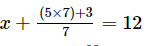
⇒ 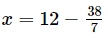
⇒ 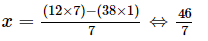
Q.10. What should be added to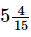 to get
to get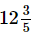 ?
?
Ans:
Let x be the required fraction.
According to the question:
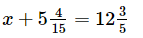
⇒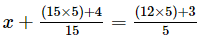
⇒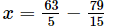
LCM of 5 and 15 is 15.
⇒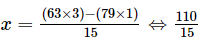
⇒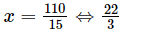
Q.11. Suman studies for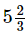 hours daily. She devotes
hours daily. She devotes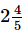 hours of her time for Science and Mathematics. How much time does she devote for other subjects?
hours of her time for Science and Mathematics. How much time does she devote for other subjects?
Ans: Suman studies for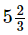 hours daily. Therefore, we have
hours daily. Therefore, we have
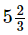 hours =
hours = 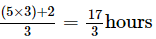
She studies science and mathematics for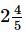 hours. Therefore, we have
hours. Therefore, we have
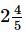 hours =
hours = 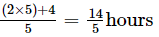
Time devoted to other subjects = Total study time − Time devoted to science and mathematics
=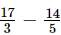 =
= 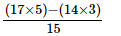
= 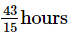
Q.12. A piece of wire is of length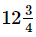 m. If it is cut into two pieces in such a way that the length of one piece is
m. If it is cut into two pieces in such a way that the length of one piece is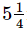 m, what is the length of the other piece?
m, what is the length of the other piece?
Ans: Let the length of second piece be x.
Total length of wire = Length of one piece + Length of second piece
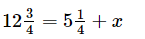
⇒ 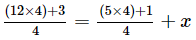
⇒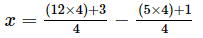
⇒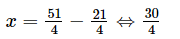
⇒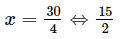
Q.13. A rectangular sheet of paper is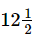 cm long and
cm long and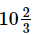 cm wide. Find its perimeter.
cm wide. Find its perimeter.
Ans:
Perimeter of rectangle = 2(length + width)
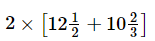
=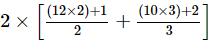
=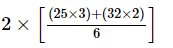
=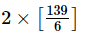
=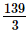 cm
cm
Q.14. In a "magic square", the sum of the numbers in each row, in each column and along the diagonal is the same. Is this a magic square?
| 4/11 | 9/11 | 2/11 |
| 3/11 | 5/11 | 7/11 |
| 8/11 | 1/11 | 6/11 |
Ans: Sum along columns and rows:
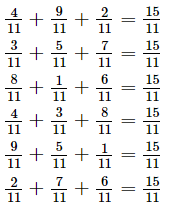
Sum along diagonals :

Since, all the sums in the square are equal along rows,columns and diagonals,it is a magic square.
Q.15. The cost of Mathematics book is Rs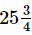 and that of Science book is Rs
and that of Science book is Rs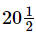 . Which costs more and by how much?
. Which costs more and by how much?
Ans: Cost of mathematics book =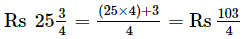
Cost of Science book =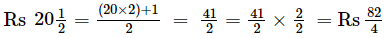
We know
82 < 103
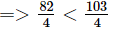
Thus, Mathematics book costs more.Difference in the cost of Mathematics and Science book = cost of Mathematics book−Cost of Science book
=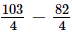
=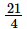 = = Rs
= = Rs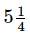
So, Mathematics book costs more by Rs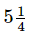
Q.16. (i) Provide the number in box and also give its simplest from in each of the following:
and also give its simplest from in each of the following:
(i)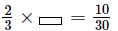
(ii)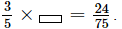
Ans:
(i)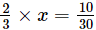
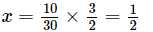
(ii)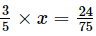
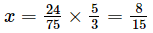
|
76 videos|452 docs|39 tests
|
FAQs on Fractions (Exercise 2.1) RD Sharma Solutions - Mathematics (Maths) Class 7 (Old NCERT)
| 1. What are fractions? |  |
| 2. How do you add fractions with different denominators? |  |
| 3. How do you simplify fractions? |  |
| 4. Can fractions be converted into decimals? |  |
| 5. How do you compare fractions? |  |
















