Short Question Answers: Number System

Q1. Find a rational number between 1 and 2.

Sol: Express 1 and 2 as rational numbers with the same denominator:
Now, the rational numbers between  are:Out of these, select any five:

Answer: The five rational numbers between 1 and 2 are:
$\backslash frac\{11\}\{10\},\; \backslash frac\{12\}\{10\},\; \backslash frac\{13\}\{10\},\; \backslash frac\{14\}\{10\},\; \backslash frac\{15\}\{10\}$

Q2. Find two rational numbers between 0.1 and 0.3.

Sol:  Express $0.10.1$ and $0.30.3$ as rational numbers with the same denominator:

$0.1=\frac{1}{10}, 0.3=\frac{3}{10}$

Now, the rational numbers between $\frac{1}{10}\backslash frac\{1\}\{10\}$ and $\frac{3}{10}\backslash frac\{3\}\{10\}$ can be written with a larger denominator to find numbers in between.
Let us express them with a denominator of $100100$100:

$0.1=\frac{10}{\mathrm{100 }},0.3=\frac{30}{\mathrm{100 }}\mathrm{ .}$

The rational numbers between $\frac{10}{100}\backslash frac\{10\}\{100\}$ and $\frac{30}{100}\backslash frac\{30\}\{100\}$ are:

$\frac{11}{100},\frac{12}{100},\frac{13}{100},...,\frac{29}{100}.\backslash frac\{11\}\{100\},\; \backslash frac\{12\}\{100\},\; \backslash frac\{13\}\{100\},\; \backslash ldots,\; \backslash frac\{29\}\{100\}$

any two:

$\frac{12}{100},\frac{25}{100}.\backslash frac\{12\}\{100\},\; \backslash frac\{25\}\{100\}.$$<br><br>$

Q3. Express   in the form of a decimal.

We have, Now, dividing 25 by 8,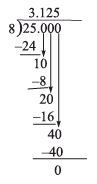
 

Since, the remainder is 0.
∴ The process of division terminates.

Q4. Express   as a rational number.

Multiplying (1) by 100, we have 100x = 100 x 0.3333...
⇒ 100x = 33.3333              ...(2)
Subtracting (2) from (1), we have
100x - x = 33.3333... - 0.3333...
⇒ 99x = 33

Q5. Simplify: (4+ √3) (4 -√3)

∵ (a + b)(a - b) = a² - b²
(4 +√3) (4 -√3) = (4)² - ( √3)² = 16 - 3 = 13
Thus, (4 +√3) (4 -√3) = 13

Q6. Simplify: (√3 +√2)²

∵ (a + b)² = a² + 2ab + b²
(√3 +√2)² = (√3)² + √2 ( √3 ×2) + (√2)² = 3 + 2 √6 + 2 = 5 + 2 √6
Thus, (√3 +√2)² = 5 + 2√6

Q7. Rationalise the denominator of 

Multiply and divide the given number by √6 + √5

Q8. Find (64)-1/3

Q9. Find a rational number lying between 1/5  and  1/2.

Rational numbers between   1/2 and  1/5 are infinite. Some of them are  3/10 ,   4/10 ,   45/100 ,  35/100 .
Step-by-step explanation:
As per the question, We need to find drational numbers lying between   1/5  and  1/2  As we know,

• Rational Numbers are numbers that can be expressed in the form of p/q where q is not equal to zero.
• Now, we know that  1/5 = 0.2 and 1/2  = 0.5
• So, numbers between 0.2 and 0.5 are infinite. Some of them are 0.3,0.4,0.45,0.35 etc.
• And these may be written as  310 ,   410 ,   45100 ,  35100  etc.

Hence, Rational numbers between 15  and  12 are infinite. Some of them are 3/10 ,   4/10 ,   45/100 ,  35/100

Q10. Express  0.245  as a fraction in the simplest form.

We know that

$0.245=\frac{245}{1000}0.245\; =\; \backslash frac\{245\}\{1000\}$

because 0.245 means 245 thousandths.

Now we simplify the fraction.

First, divide both numerator and denominator by 5:

Next, check if 49 and 200 have any common factor.
49 = 7 × 7
200 = 2 × 2 × 2 × 5 × 5

There is no common factor, so the fraction is already in simplest form.

∴ The simplest form of 0.245 is

$\frac{49}{200}\backslash boxed\{\backslash frac\{49\}\{200\}\}$