Shortcut Tricks for JEE Mains Maths | Mathematics (Maths) for JEE Main & Advanced PDF Download
Want to learn some cool SHORTCUT TRICKS to help you ACE your MATHS for JEE?
Students often label calculation errors as "silly mistakes" because they are simple slip-ups that could easily be avoided with greater care and attention. These errors commonly occur when students overlook details in the problems or when they rush through calculations without verifying their work and they can really make you lose some serious marks in JEE. Embracing math shortcut tricks not only accelerates calculation speed but also simplifies the breakdown of complex questions. While numerous tips and tricks for swift calculations abound on the internet, the challenge lies in managing and recalling these techniques. Nevertheless, integrating these strategies into your problem-solving approach can significantly optimize your time during your JEE examinations and help you devote that time to more challenging problems.
To help you improve your mathematical skills and problem-solving, here is a list of the best math shortcut tricks.
Addition Tricks
Aim for Ten
- When one of the numbers that you need to add is close to 10, round it off to 10 and therefore add or subtract whatever number you used to make the first number 10 from the other number.
Example: 7+9
Just borrow 1 from 7 so and give it to 9 to complete it 10
I.e 7 becomes 7-1=6 and 9 becomes 9+1=10
There you go, 7+9=6+10=16
Look for the Double
- Doubling numbers can be a quick trick to simplify certain additions.
For instance, when adding numbers like 17 and 19, identify the number between them (18), and then double it (18 x 2 = 36).
This method saves time and gives you the sum directly: 17 + 19 = 36
For 3-digit numbers
- In a left-to-right approach for adding two numbers, such as 953 and 867, follow these steps:
Add the hundreds place digit in the second number to the first number: 953+800=1753.
Add the tens place digit in the second number to the result obtained in the previous step:
1753+60=1813.
Add the units place digit in the second number to the result obtained in Step2: 1813+7=1820.
Divisibility Tricks
OK, so these are “rules” more so than math tricks, but learning them certainly helps students make quick work of division problems, so we’ve got to get started right here!
Divisibility by 2:
- A number is divisible by 2 if its last digit is even (0, 2, 4, 6, 8).
Example: 246 is divisible by 2 because the last digit, 6, is even.
Divisibility by 3:
- A number is divisible by 3 if the sum of its digits is divisible by 3.
Example: 513 is divisible by 3 because 5 + 1 + 3 = 9, and 9 is divisible by 3.
Divisibility by 4:
- A number is divisible by 4 if the last two digits form a number divisible by 4.
Example: 1,628 is divisible by 4 because 28 is divisible by 4.
Divisibility by 5:
- A number is divisible by 5 if its last digit is either 0 or 5.
Example: 450 is divisible by 5 because the last digit is 0.
Divisibility by 6:
- A number is divisible by 6 if it is divisible by both 2 and 3.
Example: 210 is divisible by 6 because it is divisible by 2 (ends in an even number) and by 3 (2 + 1 + 0 = 3 is divisible by 3).
Divisibility by 9:
- A number is divisible by 9 if the sum of its digits is divisible by 9.
Example: 891 is divisible by 9 because 8 + 9 + 1 = 18, and 18 is divisible by 9.
Multiplication Tricks
No Rules here, just some tricks to learn to help you speed up your multiplications!
Multiplication by 2:
- To double a number, simply add the number to itself.
Example: 5 × 2 = 5 + 5 = 10
Multiplication by 3:
- To find triple the value of a number, add the number to itself twice.
Example: 5 × 3 = 5 + 5 + 5 = 15
Multiplication by 4:
- To get four times a number, double the number and then double the result.
Example: 5 × 4 = (5 × 2) × 2 = 10 × 2 = 20
Multiplication by 5:
- Multiply the number by 10 by dividing it by 2.
Example: 8 × 5 = (8 ÷ 2) × 10 = 4 × 10 = 40
Multiplication by 8:
- Successively double the number.
Example: 5 × 8 = (5 × 4)×2 = (5 × 2)×2)×2=(10×2)×2= 20 + 20 = 40
Multiplication by 9:
- Add 1 to 9, then subtract the original number.
Example: 5 × 9 = (9 + 1) × 5 - 5 = 10 × 5 - 5 = 50 - 5 = 45
Multiplication by 11:
- The method for multiplying a two-digit number by 11 involves adding the digits of the number and placing the result between the original digits. Let me break it down:
Example 1: 32 x 11
Add the digits of 32: 3 + 2 = 5
Place the result (5) between the original digits: 352
So, 32 x 11 = 352.
Example 2: 82 x 11
Add the digits of 82: 8 + 2 = 10
Place the units digit of the result (0) between the original digits, and carry over the tens digit (1): 902
So, 82 x 11 = 902.
Another really cool trick you should use when multiplying BIG numbers:
Multiplication Expansion
Breaking down multiplications into simpler steps can make mental calculations easier. Let's use the example
23×67 to illustrate the process:
- Decompose the numbers into friendly components:
23=20+3,67=60+7 - Expand the multiplication using these components:
23×67=(20+3)×(60+7) - Apply the distributive property (FOIL or the distributive law):
=20×60+20×7+3×60+3×7 - Simplify each part separately:
=1200+140+180+21 - Combine the results:
=1541 - So,
23×67
23×67 can be broken down into
20×67+60×3+7×3
20×67+60×3+7×3, making it easier to mentally calculate each part and then sum them up. This method helps reduce the complexity of the multiplication by breaking it into simpler components.
Playing with Squares and Square Roots
Dealing with squares, especially with larger numbers, can be challenging and time-consuming. To streamline calculations and save time, it's beneficial to have some handy tips and tricks in mind for quickly calculating squares.
Steps to find the Square of a Number:
Example: 99
Choose a Base Value:
- Select a base value that is nearest to the given number. In this case, 99 is close to the base value of 100.
Calculate the Difference:
- Find the difference between the original number and the chosen base. For 99, the difference is 99−100=−1.
Adjust the Original Number:
- Add the difference to the original number.
99+(−1)=98.
Multiply by the Base:
- Multiply the adjusted result by the base value.
98×100=9800
Add the Square of the Difference:
- Add the square value of the difference to the result.
9800+1=9801
So, the square value of 99 is 9801.
Let's break down the process of squaring a number ending in 5, using the example of 55:
Squaring a Number Ending in 5:
Example 65
Step 1: Write down the end terms.
Square the last digit (5) to get the end terms. For 65, this gives us the end terms: _ _ 25.
Step 2: Multiply number on the left by the next consecutive number.
Multiply the number on the left (6) by the next consecutive number (6 + 1). In this case,
6×(6+1)=6×7=42
Combine the Results:
Combine the results from Step 1 and Step 2 to get the final answer:
4225.
So,
65×65=4225.
This method simplifies the calculation by recognizing a pattern when squaring numbers ending in 5. The end terms come from squaring the last digit, and the middle term comes from multiplying the original number by the next consecutive number.
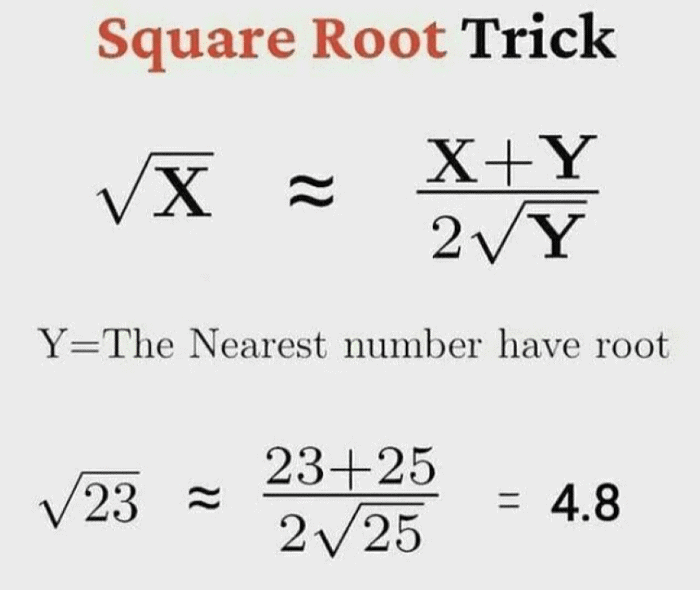
The Ultimate Square Root Trick
Having explored various methods for squaring numbers, let's now shift our focus to the realm of square roots:
- Find the number nearest that has a square root and to the number you have to find a root of
- Now simply use the formula given below:
NOW, you have your approximate answer to save your time with all the calculations
Since we are talking about Square Roots, an Important tip for you to REMEMBER:
The difference between the squares of two consecutive natural numbers is equal to the sum of the two numbers.
i.e (n+1)2 – n2 = n + (n+1).
Use this fact to find the square of a number if the square of its previous natural number is known. For e.g. 462 = 452 + 45 + 46.
Now, Use the squaring trick to find 452!
Percentages to Fractions
Make sure you have these on your mind, so as to save time of conversion during exams
- 20% is equivalent to 1/5.
- 33.33% is equivalent to 1/3.
- 25% is equivalent to 1/4.
- 11.11% is equivalent to 1/9.
- 12.5% is equivalent to 1/8.
- 9.09% is equivalent to 1/11.
- 6.66% is equivalent to 1/15.
- 6.25% is equivalent to 1/16.
Conclusion
Achieving proficiency in rapid calculations is a journey that demands commitment and perseverance. By engaging in daily and consistent practice, individuals can develop the essential skills within the span of a month. This skill set can prove invaluable, particularly in time-sensitive situation of JEE examinations.
|
209 videos|443 docs|143 tests
|
FAQs on Shortcut Tricks for JEE Mains Maths - Mathematics (Maths) for JEE Main & Advanced
| 1. What are some addition tricks to help ace math for JEE? |  |
| 2. What are some divisibility tricks to help ace math for JEE? |  |
| 3. What are some multiplication tricks to help ace math for JEE? |  |
| 4. How can playing with squares and square roots help in math for JEE? |  |
| 5. What is the ultimate square root trick for math in JEE? |  |
|
209 videos|443 docs|143 tests
|

|
Explore Courses for JEE exam
|

|