Seepage in Soils | Soil Mechanics - Civil Engineering (CE) PDF Download
Seepage Pressure and Seepage Force
Seepage pressure is exerted by the water on the soil due to friction drag. This drag force/seepage force always acts in the direction of flow.
The seepage pressure is given by
PS = hγω where, Ps = Seepage pressure
γω = 9.81 kN/m3
Here, h = head loss and z = length
(i) FS = hAγω where, Fs = Seepage force
(ii) 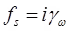 where, fs = Seepage force per unit volume.
where, fs = Seepage force per unit volume.
, i = h/z where, I = Hydraulic gradient.
Laplace Equation of Two Dimensional Flow and Flow Net

A rectangular soil element is shown with dimensions dx and dz in the plane, and thickness dy perpendicuar to this plane. Consider planar flow into the rectangular soil element.
In the x-direction, the net amount of the water entering and leaving the element is

Similarly in the z-direction, the difference between the water inflow and outflow is

For a two-dimensional steady flow of pore water, any imbalance in flows into and out of an element in the z-direction must be compensated by a corresponding opposite imbalance in the x-direction. Combining the above, and dividing by dx.dy.dz , the continuity equation is expressed as
From Darcy's law, , , where h is the head causing flow.
, where h is the head causing flow.
when the continuity equation is combined with Darcy's law, the equation for flow is expressed as:

For an isotropic material in which the permeability is the same in all directions (i.e. k x= k z), the flow equation is

This is the Laplace equation governing two-dimensional steady state flow. It can be solved graphically, analytically, numerically, or analogically.
For the more general situation involving three-dimensional steady flow, Laplace equation becomes:

One-dimensional Flow
For this, the Laplace Equation is 
Integrating twice, a general solution is obtained.

The values of constants can be determined from the specific boundary conditions.

As shown, at x = 0, h = H , and at x = L, h = 0
Substituting and solving,

The specific solution for flow in the above permeameter is

which states that head is dissipated in a linearly uniform manner over the entire length of the permeameter.
Flow Nets
Graphical form of solutions to Laplace equation for two-dimensional seepage can be presented as flow nets. Two orthogonal sets of curves form a flow net:
- Equipotential lines connecting points of equal total head h
- Flow lines indicating the direction of seepage down a hydraulic gradient
Two flow lines can never meet and similarly, two equipotential lines can never meet. The space between two adjacent flow lines is known as a flow channel, and the figure formed on the flownet between any two adjacent flow lines and two adjacent equipotential lines is referred to as a field. Seepage through an embankment dam is shown.

Calculation of flow in a channel
If standpipe piezometers were inserted into the ground with their tips on a single equipotential line, then the water would rise to the same level in each standpipe. The pore pressures would be different because of their different elevations.There can be no flow along an equipotential line as there is no hydraulic gradient.

Consider a field of length L within a flow channel. There is a fall of total head Dh. The average hydraulic gradient is

As the flow lines are b apart and considering unit length perpendicuar to field, the flow rate is

There is an advantage in sketching flow nets in the form of curvilinear 'squares' so that a circle can be insrcibed within each four-sided figure bounded by two equipotential lines and two flow lines.
In such a square, b = L , and the flow rate is obtained as Dq = k.Dh
Thus the flow rate through such a flow channel is the permeability k multiplied by the uniform interval Dh between adjacent equipotential lines.
Calculation of total flow
For a complete problem, the flow net can be drawn with the overall head drop h divided into Nd so that Dh = h / Nd.
If Nf is the no. of flow channels, then the total flow rate is

|
30 videos|76 docs|74 tests
|
FAQs on Seepage in Soils - Soil Mechanics - Civil Engineering (CE)
| 1. What is seepage in soils? |  |
| 2. How does seepage occur in soils? |  |
| 3. What are the effects of seepage in civil engineering projects? |  |
| 4. How can seepage be controlled or mitigated in civil engineering projects? |  |
| 5. What are the factors influencing seepage in soils? |  |

|
Explore Courses for Civil Engineering (CE) exam
|

|

















