Civil Engineering (CE) Exam > Civil Engineering (CE) Notes > Additional Documents & Tests for Civil Engineering (CE) > Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2)
Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE) PDF Download
Source or Sink
Source flow -
A flow with straight streamlines emerging from a point.
Velocity along each streamline varies inversely with distance from the point (shown in Fig. 20.3).
Only the radial component of velocity is non-trivial. (vθ=0, vz=0 ).
In a steady source flow the amount of fluid crossing any given cylindrical surface of radius r and unit length is constant 
(20.10a)
(which shows that velocity is inversely proportional to the distance )
where, K is the source strength 
is the volume flow rate
The definition of stream function in cylindrical polar coordinate states that
(20.11)
For the source flow,
(20.13)
Combining Eqs (20 .12) and (20.13) , we get
(20.14)
Thus
Because the flow is irrotational, we can write
(20.15)
The integration constants C1 and C2 in Eqs (20.14) and (20.15) have no effect on the basic structure of velocity and pressure in the flow.
The equations for streamlines and velocity potential lines for source flow become
(20.16)
K = source strength and is proportional to 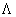  the rate of volume flow from the source per unit depth perpendicular to the page |
Sink flow
When
is negative , we get sink flow,here the flow is in the opposite direction of the source flow.
is negative , we get sink flow,
In Fig. 20.3, the point 0 is the origin of the radial streamlines. We visualize that point O is a point source or sink that induces radial flow in the neighbourhood .
The point source or sink is a point of singularity in the flow field (because vr becomes infinite).
The stream function and velocity potential function are
(20.17)
The document Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE) is a part of the Civil Engineering (CE) Course Additional Documents & Tests for Civil Engineering (CE).
All you need of Civil Engineering (CE) at this link: Civil Engineering (CE)
FAQs on Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) - Additional Documents & Tests for Civil Engineering (CE)
| 1. What are the assumptions made in analyzing inviscid, incompressible, irrotational flows? |  |
Ans. The assumptions made in analyzing inviscid, incompressible, irrotational flows are that the flow is frictionless (inviscid), the fluid has a constant density (incompressible), and the fluid particles do not rotate (irrotational). These assumptions simplify the mathematical analysis of the flow and allow for the use of potential flow theory.
| 2. What is the significance of inviscid flow in civil engineering? | |
Ans. Inviscid flow is significant in civil engineering as it allows engineers to analyze and predict the behavior of fluids in various structures and systems. By neglecting the effects of viscosity, engineers can simplify calculations and make design decisions based on the assumption of frictionless flow. This is particularly useful in designing fluid transport systems, such as pipelines and channels, where minimizing energy loss due to friction is important.
| 3. How does the assumption of incompressibility affect the analysis of fluid flow? | |
Ans. The assumption of incompressibility means that the fluid density remains constant throughout the flow. This assumption simplifies the mathematical equations used to analyze fluid flow by eliminating the need to consider changes in density. Incompressible flow is often used in civil engineering for practical purposes, as many fluids, such as water, can be approximated as incompressible under normal conditions.
| 4. What is the significance of irrotational flow in civil engineering applications? | |
Ans. Irrotational flow is significant in civil engineering as it allows for the analysis of fluid flow without considering the rotational motion of fluid particles. This simplifies the mathematical analysis and enables engineers to make design decisions based on potential flow theory. Irrotational flow is commonly used in the analysis of open channel flow, hydraulic structures, and aerodynamics.
| 5. What are the limitations of assuming inviscid, incompressible, irrotational flows? | |
Ans. The assumptions of inviscid, incompressible, irrotational flows have certain limitations. In reality, all fluids have some degree of viscosity, which affects the flow behavior. Additionally, compressibility effects become significant at high speeds or in the presence of large pressure gradients. Finally, the assumption of irrotational flow may not hold true in cases where vortices or rotational motion are important, such as in turbulent flow or near solid boundaries. Therefore, while these assumptions are useful for certain engineering analyses, they may not accurately represent real-world flow conditions in all cases.
Related Exams
About this Document
|
4.97/5 Rating |
|
Oct 31, 2024 Last updated |
Document Description: Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) for Civil Engineering (CE) 2024 is part of Additional Documents & Tests for Civil Engineering (CE) preparation.
The notes and questions for Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) have been prepared according to the Civil Engineering (CE) exam syllabus. Information about Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) covers topics
like and Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Example, for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2).
Introduction of Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) in English is available as part of our Additional Documents & Tests for Civil Engineering (CE)
for Civil Engineering (CE) & Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) in Hindi for Additional Documents & Tests for Civil Engineering (CE) course.
Download more important topics related with notes, lectures and mock test series for Civil Engineering (CE)
Exam by signing up for free. Civil Engineering (CE): Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE)
Description
Full syllabus notes, lecture & questions for Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE) - Civil Engineering (CE) | Plus excerises question with solution to help you revise complete syllabus for Additional Documents & Tests for Civil Engineering (CE) | Best notes, free PDF download
Information about Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2)
In this doc you can find the meaning of Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) defined & explained in the simplest way possible. Besides explaining types of
Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) theory, EduRev gives you an ample number of questions to practice Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) tests, examples and also practice Civil Engineering (CE)
tests
|
64 docs|6 tests
|
Download as PDF
|
Explore Courses for Civil Engineering (CE) exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
Previous Year Questions with Solutions
,Viva Questions
,Important questions
,Exam
,Semester Notes
,Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE)
,shortcuts and tricks
,mock tests for examination
,Analysis of Inviscid
,video lectures
,Analysis of Inviscid
,Summary
,practice quizzes
,ppt
,Free
,Objective type Questions
,Incompressible
,past year papers
,Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE)
,Extra Questions
,study material
,MCQs
,Analysis of Inviscid
,Irrotational Flows (Part - 2) | Additional Documents & Tests for Civil Engineering (CE)
,Incompressible
,Sample Paper
,Incompressible
;
Additional Information about Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) for Civil Engineering (CE) Preparation
Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Free PDF Download
The Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) is an invaluable resource that delves deep into the core of the Civil Engineering (CE) exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) now and kickstart your journey towards success in the Civil Engineering (CE) exam.
Importance of Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2)
The importance of Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) cannot be overstated, especially for Civil Engineering (CE) aspirants.
This document holds the key to success in the Civil Engineering (CE) exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Notes
Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2).
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Notes on EduRev are your ultimate resource for success.
Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Civil Engineering (CE) Questions
The "Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) Civil Engineering (CE) Questions" guide is a valuable resource for all aspiring students preparing for the
Civil Engineering (CE) exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) on the App
Students of Civil Engineering (CE) can study Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2),
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Analysis of Inviscid, Incompressible, Irrotational Flows (Part - 2) is prepared as per the latest Civil Engineering (CE) syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup