Definite integration, Business Mathematics & Statistics | Business Mathematics and Statistics - B Com PDF Download
| Table of contents |

|
| Definite Integrals |

|
| Notation |

|
| Definite Integral |

|
| Continuous |

|
| Properties |

|
| SummaryThe Definite Integral between a and b is the Indefinite Integral at b minus the Indefinite Integral ata. |

|
Definite Integrals
Integration can be used to find areas, volumes, central points and many useful things. But it is often used to find the area under the graph of a function like this:
The area can be found by adding slices that approach zero in width:
And there are Rules of Integration that help us get the answer.
Notation
he symbol for "Integral" is a stylish "S"
(for "Sum", the idea of summing slices):
After the Integral Symbol we put the function we want to find the integral of (called the Integrand),
and then finish with dx to mean the slices go in the x direction (and approach zero in width).
Definite Integral
A Definite Integral has start and end values: in other words there is an interval (a to b).
The values are put at the bottom and top of the "S", like this:
We can find the Definite Integral by calculating the Indefinite Integral at points a and b, then subtracting:
Example:
The Definite Integral, from 1 to 2, of 2x dx:
Subtract:
(22 + C) − (12 + C)
22 + C − 12 − C
4 − 1 + C − C = 3
And "C" gets cancelled out ... so with Definite Integrals we can ignore C.
In fact we can give the answer directly like this:
Check: with such a simple shape, let's also try calculating the area by geometry:
Yes, it does have an area of 3.
(Yay!)
Let's try another example:
Example:
The Definite Integral, from 0.5 to 1.0, of cos(x) dx:
(Note: x must be in radians)
The Indefinite Integral is:
We can ignore C when we do the subtraction (as we saw above):
And another example to make an important point:
Example:
The Definite Integral, from 1 to 3, of cos(x) dx
Notice that some of it is positive, and some negative.
The definite integral will work out the net area.
The Indefinite Integral is:∫cos(x) dx = sin(x) + C
So let us do the calculations
So there is more negative than positive parts, with the net result of −0.700....
Example: What is the area between y = cos(x) and the x-axis from x = 1 to x = 3?
This is like the example we just did, but all area is positive (imagine you had to paint it).
So now we have to do the parts separately:
- One for the area above the x-axis
- One for the area below the x-axis
The curve crosses the x-axis at x = π/2 so we have:
From 1 to π/2:
From π/2 to 3:
That last one comes out negative, but we want it to be positive, so:
Total area = 0.159... + 0.859... = 1.018...
This is very different from the answer in the previous example.
Continuous
Oh yes, the function we are integrating must be Continuous between a and b: no holes, jumps or vertical asymptotes (where the function heads up/down towards infinity).
Example:
A vertical asymptote between a and b affects the definite integral.
Properties
Reversing the interval
Reversing the direction of the interval gives the negative of the original direction.
Interval of zero length 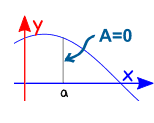
When the interval starts and ends at the same place, the result is zero:
Adding intervals 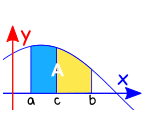
We can also add two adjacent intervals together:
Summary
The Definite Integral between a and b is the Indefinite Integral at b minus the Indefinite Integral ata.
|
124 videos|176 docs
|
FAQs on Definite integration, Business Mathematics & Statistics - Business Mathematics and Statistics - B Com
| 1. What is definite integration? |  |
| 2. How is definite integration used in business mathematics? |  |
| 3. What is the significance of definite integration in statistics? |  |
| 4. How can definite integration be applied in financial analysis? |  |
| 5. What are some real-life examples where definite integration is used? |  |




















