Theory Of Mixing, Crop Process Engineering | Crop Process Engineering Notes - Agricultural Engg - Agricultural Engineering PDF Download
Introduction
Mixing is a unit operation in which two or more materials are interspersed in space with one another. In agriculture and food processing, mixing operations are often used to blend ingredients. Particularly, mixing is used in the food industry with the main objective of reducing non-uniformities and gradients in properties such as concentration, colour, texture, or taste between different parts of a system (Uhl and Gray, 1986). The degree of uniformity required may vary somewhat, but most of the time it is important to provide a nutritionally balanced and palatable feed mixture.
The mixing and/or agitation of liquids, solids and (to a lesser extent) gases is one of the commonest of all operations in the food processing industries. Of the possible combinations of these states, those of principal interest are liquid–liquid mixtures, solid–solid mixtures and liquid–solid mixtures or pastes. However, it is important at this early stage to define exactly what is meant by the terms ‘agitation’ and/or ‘mixing’ and it is perhaps easiest to do this by considering liquid–liquid systems.
The agitation of a liquid may be defined as the establishment of a particular flow pattern within the liquid, usually a circulatory motion within a container. On the other hand mixing implies the random distribution, throughout a system, of two or more initially separate ingredients. Mixing is frequently employed to develop the desired product characteristics such as texture rather than simply ensure product homogeneity. If mixing fails to achieve the required product yield, quality, and organoleptic or functional attributes, production costs may increase significantly.
There are a number of reasons for agitating liquids amongst which may be listed: the suspension of solids within the liquid; the dispersion of a gas within the liquid; the dispersion of a second liquid as droplets (i.e. the formation of an emulsion); the promotion of heat transfer from a heat transfer surface to the bulk liquid; and the mixing of two or more liquids. Now the reasons for mixing (and this applies to all possible combinations of the three states of matter) are: to bring about intimate contact between different states in order for a chemical reaction to occur (this can include the dissolution of solids in a liquid and the extraction of a solute from either liquid or solid phases); and to provide a new property of the mixture which was not present in the original separate components. An example of the latter might be the inclusion of a specific proportion in a food mixture of a given component for nutritional purposes.
It should be clear from the foregoing that mixing is brought about by agitation. However, it would be tedious to continue to use both words according to their precise meaning and therefore throughout this chapter the term ‘mixing’ will be used to mean both the random distribution of components and the means of bringing about that randomness, that is, the mechanisms of agitation.
There are perhaps three criteria by which the performance of a mixer should be assessed. These are
(i) the degree of mixedness achieved;
(ii) the time required to bring about mixing; and
(iii) the power consumption required.
A wide range of dry food materials are mixed, including combinations of flour, sugar, salt, flavouring materials, dried milk, and dried vegetables and fruits.
17.1 Theory of solid mixing
As opposed to mixing of miscible liquids, the mixing of particles is often a readily reversible process. A mixture of miscible liquids leaving a mixing unit retains or even improves its mixedness during the transport process, while a well-mixed batch of particles can be separated almost completely at a subsequent process stage. Particles change their relative positions in response to movement and the subsequent rearrangement maybe more random. Powder mixing occurs when any particle changes its path of circulation. Three mechanisms have been recognized in solids mixing: convection, diffusion, and shear. In any particular process one or more of these three basic mechanisms may be responsible for the course of the operation. Other mechanisms such as segregation can also be involved during particle motions. Depending on the equipment used, mixing mechanisms can receive other classifications that will be mentioned further in this section.
17.1.1 Convective, Diffusive and Shear Mixing
During convective mixing, masses or groups of particles transfer together from one location to another, while in diffusion mixing, individual particles are randomly distributed over a surface developed within the mixture. In shear mixing, groups of particles are mixed through the formation of slipping planes developed within the mass of the mixture. Shear mixing is sometimes considered as part of the convective mechanism.
In convective mixing, a circulating flow of powders is usually caused by the rotational motion of a mixer vessel, an agitating impeller (such as a ribbon or a paddle), or gas flow. This circulating flow contributes mainly to a macroscopic mixing of bulk powder mixtures. Large portions of the total mix are moved at relatively high rates, and changes at a microscopic scale are not expected. Therefore, pure convection tends to be less effective, leading to a final mixture, which may still exhibit poor mixing characteristics on a fine scale. Convective mixing is beneficial for batch mode operations but gives unfavourable effects for continuous mode mixing.
Diffusive mixing (or random wall phenomenon) is caused by the random motion of powder particles. The rate of mixing by this mechanism is low compared with convective mixing, but diffusive mixing is essential for microscopic homogenization. It has been concluded that diffusion is the best mechanism for axial mixing, similar to diffusion of particles in gas and liquid phases. Pure diffusion, when feasible, is highly effective, producing very intimate mixtures at the level of individual particles but at an exceedingly slow rate. A trough mixer with a ribbon spiral can give almost pure convective mixing, while a simple barrel mixer gives mainly a form of diffusion mixing. These features of diffusion and convective mixing mechanisms suggest that an effective operation may be achieved by combining both, in order to take advantage of the speed of convection and the effectiveness of diffusion.
Shear mixing is induced by the momentum exchange of powder particles having different velocities (differential velocity distribution). Shear mixing is developed by the formation of slipping planes in the bulk material; the originally coherent particle groups are gradually broken along these planes. The velocity distribution develops around the agitating impeller and the vessel walls due to compression and extension of bulk powders. It is also developed in the powder layer in rotary vessel mixers and at blowing ports in gas-flow mixers. Shear mixing can enhance semi-microscopic mixing and be beneficial in both batch and continuous operations. In free-flowing powders, both diffusive mixing and shear mixing give rise to size segregation (or de-mixing), therefore, for such powders, convective mixing is the major mechanism promoting mixing.
Powder mixing proceeds in a mixer where the three mechanisms described above take place simultaneously. The characteristic curve of mixing is the plot of the degree of mixedness M (on a logarithmic scale) against the mixing time t (on a linear scale). The mixing time is the time measured from the start of mixing in a batch mode operation, whereas it corresponds to the mean residence time (the powder volume in a mixer divided by its volumetric flow rate) in a continuous operation. The characteristic curve of mixing is useful for the performance evaluation of mixers. Fig. 5.1 shows a schematic example of the curve, where the standard deviation is plotted on a logarithmic scale.
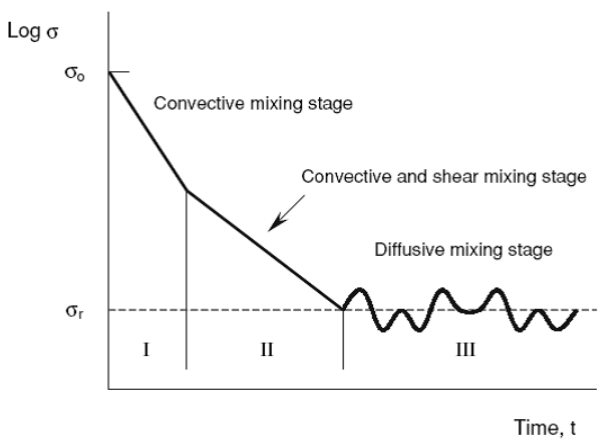
Convective mixing is dominant in the initial stage (I) and the mixing proceeds steadily by both convective and shear mechanisms in the intermediate stage (II). In the final stage (III), the effect of diffusive mixing appears and the dynamic equilibrium between mixing and segregation is reached. The degree of mixedness at this state is called the final degree of mixedness, M∞. Various powder mixers exhibit a variety of patterns in the characteristic curve of mixing. Operating conditions and powder properties significantly influence the value of M∞. In comparison with fluid mixing, in which diffusion can be normally regarded as spontaneous, particulate systems will only diffuse as a result of mechanical movement provided by gravity, shaking, tumbling, vibration, or any other mechanical mean. Lacey (1954) tried to adjust Fick’s equation, the simplest model for molecular diffusion in liquids, to the mixing of solids. Fick’s equation has the following form:
\[{{\partial C}\over{\partial t}}=D{{\partial ^2 C} \over {\partial x^2 }}\]...............................................(16.1)
where, C is the concentration of solids, D is the diffusivity, and x is the distance in the direction of dispersion. It is clear that the diffusivity D in solids does not have the same physical meaning as in liquids, given that D varies with the magnitude and direction of the force impelled to the powder bulk to generate movement. However, the model could describe a binary mixture of particles with the same mean diameter fed into rotating horizontal drum in such a way that a thin layer is perpendicular to the axis of rotation. The equation can be solved as a function of the number of revolutions of the mixer and the distance from one of the sidewalls. The movement of particles during a mixing operation, however, can also result in another mechanism that may retard, or even reverse, the mixing process, known as segregation.
Degree of Mixedness
Based on a diffusive mixing mechanism and employing a modified Fick’s diffusion equation, Rose (1970) proposed the following mixing rate equation to describe the process in which both mixing and de-mixing (i.e., segregation) occur simultaneously in a mixer.
\[{{dM} \over {dt}} = A\left( {1 - M} \right) - B\lambda \] .........................................................................(16.2)
Where, A and B are mixing and de-mixing rate constants respectively
M is the degree of mixedness and defined as;
\[M =1- {\sigma\over{\sigma o}}\]........................................................................................................(16.2.1)
Where, σ is the standard deviation
σ0 is the standard deviation at t=0 and
\[\lambda = \pm \sqrt {1 - M}\]...............................................................................................................(16.2.2)















