Subjective Type Questions: Sequences and Series | JEE Advanced | 35 Years Chapter wise Previous Year Solved Papers for JEE PDF Download

Q.1. The harmonic mean of two numbers is 4. Their arithmetic mean A and the geometric mean G satisfy the relation. 2A + G² = 27
 Find the two numbers. (1979) 

Ans. 3 and 6 or 6 and 3 

Sol. Let the two numbers be a and b, then

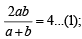
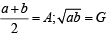
Also 2A + G² = 27 ⇒ a + b + ab = 27 ....(2)

Putting ab = 27 – (a+b) in eqn. (1), we get

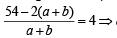a + b = 9 then ab = 27 –  9 = 18

Solving the two we get a = 6, b = 3 or a = 3, b = 6, which are the required numbers.

Q.2. Th e in ter ior an gles of a polygon ar e in ar ith metic progression. The smallest angle is 120°, and the common difference is 5°, Find the number of sides of the polygon. (1980)

Ans. 9

 Sol. Let there be n sides in the polygon.
Then by geometry, sum of all n interior angles of polygon = (n – 2) × 180° Also the angles are in A.P. with the smallest angle = 120° , common difference = 5° ∴ Sum of all interior angles of polygon

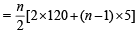
Thus we should have

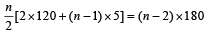
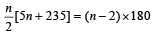
⇒ 5n² + 235n= 360n- 720
⇒ 5n² - 125n + 720= 0
⇒ n² - 25n + 144= 0
⇒ (n -16) (n - 9)= 0
⇒ n = 16,  9

Also if n = 16 then 16th angle = 120 + 15 × 5 = 195° > 180°

∴ not possible. Hence n = 9.

Q.3. Does there exist a geometric progression containing 27, 8 and 12 as three of its terms  ? If it exits, how many such progressions are possible ? (1982 - 3 Marks)

Ans. yes, infinite

Sol. If possible let for a G.P.
T_p= 27 = ARp^–1 ....(1)
T_q= 8 = AR^q–1 ....(2)
T_r= 12 = AR^r–1 ....(3)
From (1) and (2)

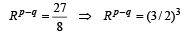....(4)

From (2) and  (3):

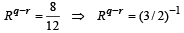....(5)

From (4) and (5):

R = 3/2; p – q = 3 ;   q – r = – 1 p – 2q + r = 4;     p, q, r ∈ N ....(6)

As there can be infinite natural numbers for p, q and r to satisfy equation (6)

∴ There can be infinite G.P’s.

Q.4. Find three numbers a, b, c, between 2 and 18 such that (i) their sum is 25 (ii) the numbers 2, a, b sare consecutive terms of an A.P. and (iii) the numbers b, c, 18 are consecutive terms of a G.P. (1983 - 2 Marks)

Ans.  a = 5,  b = 8,  c = 12

Sol. 2 < a, b, c  <  18         a + b + c = 25 ....(1)

2, a, b are in AP ⇒ 2a = b + 2 ⇒ 2a – b = 2 ....(2)

b, c, 18 are in GP ⇒ c² = 18b ....(3)

From  (2)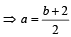  

+  

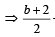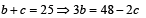
(3) ⇒ c² = 6 (48 - 2c) ⇒ c² + 12c - 288= 0

⇒ c = 12, – 24 (rejected)   ⇒ a = 5,  b = 8,  c = 12

Q. 5. If a > 0, b > 0 and c > 0, prove that

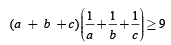         (1984 - 2 Marks)

Ans. Sol. Given that a, b, c > 0

We know for +ve numbers A.M. ≥ G.M.

∴ For +ve numbers a, b, c we get

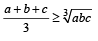             ....(1)

Also for +ve numbers 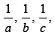 we get

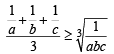....(2)

Multiplying in eqs (1) and (2) we get

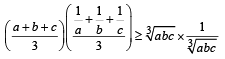
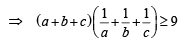Proved.

Q.6. If n is a natural number such that

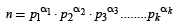an d p₁, p₂, ....., p_k are distinct primes, then show that ln n ≥ k ln2 (1984 - 2 Marks)  

Ans. Sol. Given that 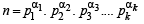         ....(1)

Where n∈N and  p₁,  p₂, p₃, ......p_k are distinct prime numbers.
Taking log on both sides of eq. (1),

we get log n = α₁ log p₁+ α₂ log p₂ + ....+ a_k  log p_k ....(2)

Since every prime number is such that

p_i ≥ 2

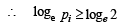....(3)

∀ i= 1 (1) k Using (2) and (3)

we get log n ≥ α₁ log 2 + α₂ log 2 + α₃ log 2 + ....+ α_k log 2
⇒ log n ≥ (α₁+α₂+ ....+ α_k) log 2
⇒ log n ≥ k log 2 Proved.

Q.7. Find the sum of the series :

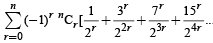 ... up to  terms]

Ans. Sol. The given series is

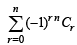
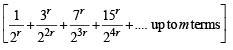
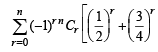
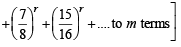
Now,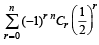  

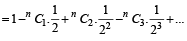
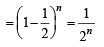
Similarly,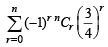  

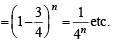
Hence the given series is,

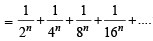.... to terms

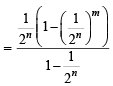[ Summing the G.P.]

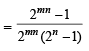
Q.8. Solve for x the following equation : (1987 - 3 Marks)  
 log_{(2 x +3)} (6 x² + 23x + 21) = 4 - log _(3x+7) (4 x² + 12x+ 9)

Ans.  -1/4

Sol. The given equation is log _{(2x + 3)} (6x² + 23 + 21)
= 4 – log_3x+7 (4x² + 12x + 9)

⇒ log_(2x+3) (6x² + 23x + 21) + log_(3x+7) (4x² + 12x + 9) = 4

⇒ log_(2x+3) (2x + 3) (3x + 7) + log_(3x+7) (2x + 3)²  x =4

⇒ 1+log_(2x+3) (3x + 7) + 2log_(3x+7) (2x + 3) =4

⇒ log_(2x+3)(3x + 7) +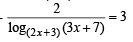

Let log_(2x+3)(3x + 7) = y ....(1)

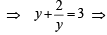y² -3y+2=0

⇒ (y – 1)  (y – 2) = 0 ⇒ y = 1, 2

Substituting the values of y in (1), we get

⇒ log_(2x+3)(3x + 7) = 1  and     log_(2x+3)(3x + 7) = 2

⇒ 3x + 7 = 2x + 3 an d3x + 7 = (2x + 3)² 

⇒ x  = – 4 an d4x² + 9x + 2 = 0

⇒ x = – 4 an d(x + 2) (4x + 1) = 0

⇒ x = – 4 and x = – 2, x = –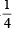

As log a x is defined for x > 0 and a > 0 (a ≠ 1), the possible value of x should satisfy all of the following inequalities :

⇒ 2x + 3 > 0 and 3x + 7 > 0

Also 2x + 3 ≠ 1 and 3x + 7 ≠ 1

Out of x  =  – 4, x = – 2 an d x  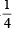= – only x  = –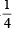

satisfies the above inequalities.

So only solution is x = –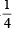

Q. 9. If log 3 ² , log₃ (2 ^x , 5) , and 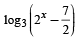are in arithmetic progression, determine the value of x. (1990 -  4 Marks)

Ans.  3

Sol. Given that log₃ 2, log3 (2^x – 5), log3 (2^x –7/2) are in A.P.
⇒ 2 log₃ (2^x – 5) = log₃ ² + log₃ (2x – 7/2)

⇒ (2 ^x - 5) ²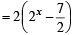 

⇒ (2^x)² – 10.2^x + 25 – 2.2^x + 7 = 0 ⇒ (2^x)² – 12.2^x + 32 = 0

Let 2^x  = y, then we get, y² – 12y + 32 = 0
⇒ (y – 4) (y – 8) = 0
⇒ y = 4  or  8
⇒ 2x = 22  or 23 ⇒ x = 2  or 3

But for log₃ (2^x – 5) and log₃ (2^x – 7/2) to be defined
2^x –  5 > 0 and 2^x  –  7/2 > 0 ⇒ 2^x  >  5 and 2^x  > 7/2
⇒ 2^x > 5 ⇒ x ≠ 2  and therefore x = 3.

Q.10. Let p be the first of the n arithmetic means between two numbers and q the first of n harmonic means between the same numbers. Show that q does not lie between p and

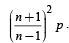(1991 -  4 Marks)

Ans. Sol. Let a and b be two numbers and A₁, A₂, A₃, .... A_n be n A.M’s between a and b.
Then a, A₁, A₂, ..... A_n, b are in A.P.
There are (n + 2) terms in the series, so that

a + (n + 1) d = b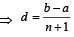

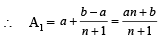
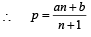....(1)

The first H.M. between a and b, when nHM’s are inserted between a and b can be obtained by  replacing a by 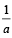  and b by  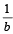 in eq. (1) and then taking its reciptocal.

Therefore,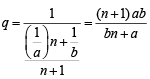

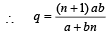...(2)

We have to prove that q cannot lie between p

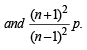
Now, n + 1>n –1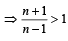

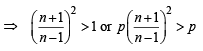
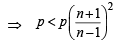....(3)
Now to prove the given, we have to show that q is less than p.

For this, let,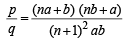  

⇒

⇒

⇒

⇒

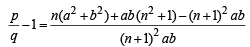
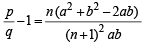
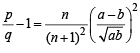
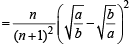
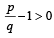
⇒ (provided a and b and hence p and q are +ve) p > q            ....(4)

From 3 and (4), we get,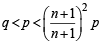  

∴ q can not lie between p and 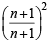 p, if a and b are +ve numbers.

Q.11. If S₁, S₂ ,S₃, ............... , S_n are the sums of infinite geometric series whose first terms are 1, 2, 3, ..............., n and whose common ratios are

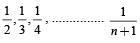 respectively,,then find the values of  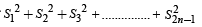(1991 -  4 Marks)

Ans. Sol.  We have,

....................................................
....................................................

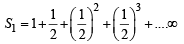
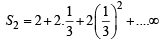
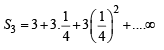
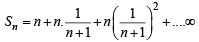
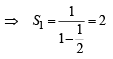
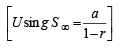
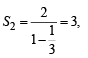
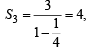
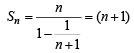
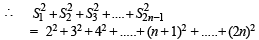
NOTE THIS STEP :

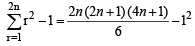
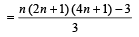
Q.12. The real numbers x₁, x₂, x ₃ s atis fying the equation x³ , x² + βx + γ < 0 are in AP. Find the intervals in which β and γ lie. (1996 - 3 Marks)

Ans. Sol. Since x₁,  x₂,  x₃ are in A.P.
Therefore, let  x₁ = a – d, x₂ = a and x₃ = a + d and x₁,  x₂,  x₃ are the roots of  x₃ – x₂ + βx  + γ =0
We have ∑α = a – d + a + a + d = 1 ....(1)
∑αβ = (a – d) a + a ( a + d) + (a – d) (a + d) = b ....(2)
αβγ = (a – d) a (a + d) = – γ ....(3)

From (1), we get, 3a = 1 ⇒ a = 1/3

From (2), we get, 3a² – d² = β

⇒ 3(1/3)² – d² = β ⇒ 1/3 – β = d² 

We know that d² > 0 ∀ d ∈ R

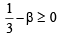            ∵ d 2 ≥ 0

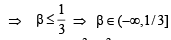
From (3), a (a² – d²)  = – γ

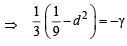
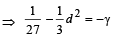
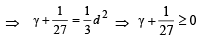
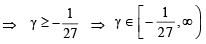
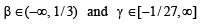
Q.13. Let a, b, c, d be real numbers in G.P. If u, v, w, satisfy the system of equations (1999 - 10 Marks)
   
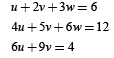
 then show that the roots of the equation

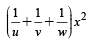
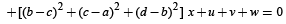
 and 20x² + 10 (a - d)² x - 9 = 0 are reciprocals of each other.

Ans. 

Sol. Solving the system of equations, u + 2v + 3w = 6, 

4u + 5v + 6w = 12 and 6u + 9v = 4

we get u = – 1/3, v = 2/3, w = 5/3

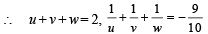
Let r be the common ratio of the G.P., a, b, c, d. Then b = ar, c = ar²,  d = ar³ .
Then the first equation

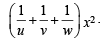+ [(b – c)² + (c – a)² + (d – b)²]x + (u + v + w) = 0 becomes

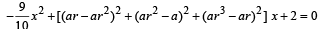
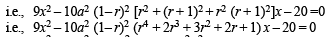
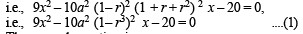
The second equation is, 20 x² + 10 (a – ar³)²  x – 9 = 0

i.e., 20 x² + 10 a²  (1 – r³)² x – 9 = 0 ....(2)

Since (2) can be obtained by the substitution x → 1/x , equations (1) and (2) have reciprocal roots.

Q.14. The fourth power of the common difference of an arithmatic progression with integer entries is added to the product of any four consecutive terms of it. Prove that the resulting sum is the square of an integer.           (2000 - 4 Marks)

Ans. 

Sol.  Let a – 3d, a – d, a + d and a + 3d  be any  four consecutive terms of an A.P. with common difference 2d.

∵ Terms of A.P. are integers, 2d is also an integer.
Hence  P = (2d)⁴ + (a – 3d) (a – d) (a + d) (a + 3d)

=16 d ⁴ + (a ² – 9 d ²) (a ² – d ²) = (a ² – 5 d ²)²

Now, a ² – 5 d ² = a ² – 9 d ² + 4 d ² = (a – 3 d) (a + 3 d) + (2 d)² =  some integer

Thus, P = square of an integer.

Q.15. Let a₁, a₂, …, a_n be positive real numbers in geometric progression. For each n, let A_n, G_n, H_n be respectively, the arithmetic mean, geometric mean, and harmonic mean of a₁, a₂, …, a_n. Find an expression for the geometric mean of G₁, G₂, …, G_n in terms of A₁, A₂ , …, A_n, H₁, H₂, …, H_n. (2001 - 5 Marks)

Ans.

 Sol. Given that a₁, a₂, ....a_n are +ve real no’s in G.P.

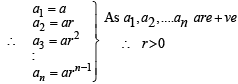
A_n is A.M. of a₁, a₂, ....., a_n

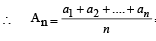
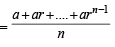
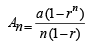.... (1)  (For r ≠ 1)

G_n is G.M. of a₁, a₂, ...., a_n

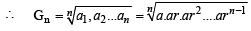
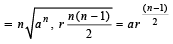
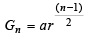          .... (2)   (r ≠ 1)

H_n is H.M. of a₁,  a₂, ....., a_n

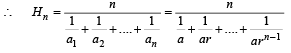
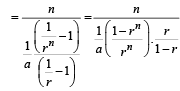
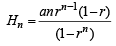(r ≠ 1) ...(3)
We also observe that

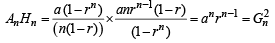
∴ A_n H_n =G_n²....(4)

∴ Now, G.M. of   G₁, G₂, .... G_n is

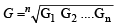
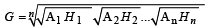  [Using equation (4)

G = (A₁A₂....A_nH₁H₂....H_n)1/2n   ...(5)

If  r = 1 then A_n = G_n = H_n = a

Also A_n H_n = G_n²

∴ For r = 1 also, equation (5) holds.

Hence we get, G = (A₁A₂....A_nH₁H₂....H_n)^1/2n

Q.16. Let a, b be positive real numbers. If a, A₁, A₂, b are in arithmetic progression, a, G₁, G₂, b are in geometric progression and a, H₁, H₂, b are in harmonic progression,

show that 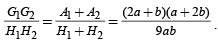     (2002 - 5 Marks)

Ans. 

Sol. Clearly A₁ + A₂ = a + b

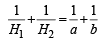
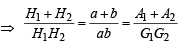
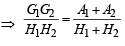
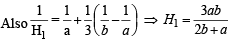
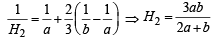
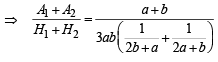
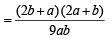
Q.17. If a, b, c are in A.P., a², b², c² are in H.P., then prove that either a = b = c or a, b, 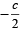 form a G.P.. (2003 - 4 Marks)

Ans. 

Sol. Given that a, b, c are in A.P.
⇒ 2b = a + c ....(1)
and a², b², c² are in H.P.

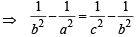
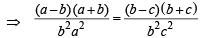
⇒ ac² + bc² = a²b + a²c     [∵ a – b = b – c]
⇒ ac (c – a) + b (c – a) (c + a) = 0
⇒ (c – a) (ab + bc + ca) = 0
⇒ either c – a = 0 or ab + bc + ca = 0

⇒ either c = a or (a + c) b + ca = 0 and then from (i)
2b² + ca = 0

Either a = b = c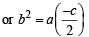    

i.e. a, b, – c/2 are in G.P. Hence Proved.

Q.18. If a_n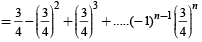and

b_n = 1 – a_n, then find the least natural number n₀ such that

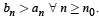 (2006 - 6M)

Ans. 

Sol.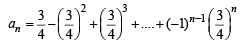

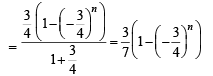
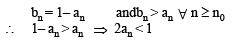
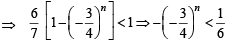
⇒ (– 3)^{n +1} < 2^2n–1 For n to be even, inequality always holds. For n to be odd, it holds for n ≥ 7.
∴ The least natural no., for which it holds is 6
(∵ it holds for every even natural no.)