JEE Advanced (Subjective Type Questions): Some Basic Concepts of Chemistry- 2 | Chapter-wise Tests for JEE Main & Advanced PDF Download
Q. 15. Balance the following equations.
(i) Cu2O + H+ + NO3- → Cu2+ + NO + H2O (1981 - 1 Mark)
(ii)K4[Fe(CN)6] + H2SO4 + H2O → K2SO4 + FeSO4 + (NH4)2SO4 + CO (1981 - 1 Mark)
(iii) C2H5OH + I2 + OH– → CHI3 + HCO 3- + I– + H2O (1981 - 1 Mark)
Ans. Sol. Balance the reactions by ion electron method.
(i) Cu2O + 2H+ → 2Cu2+ + H2O + 2e–] × 3 ......(i)
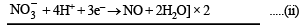
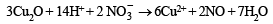
(ii)K4[Fe(CN)6] + 6H2SO4 + 6H2O
→ 2K2SO4 + FeSO4 + 3(NH4)2SO4 + 6CO
(iii) C2H5OH + 4I2 + 8OH–
→CHI3 + HCO3- + 5I– + 6H2O
Q. 16. Hydroxylamine reduces iron (III) according to the equation:
2NH2OH + 4Fe3+ → N2O(g) ↑ + H2O + 4 Fe2+ + 4H+
Iron (II) thus produced is estimated by titration with a standard permanganate solution. The reaction is :
MnO4- + 5 Fe2+ + 8H+ → Mn2+ + 5 Fe3+ + 4H2O
A 10 ml. sample of hydroxylamine solution was diluted to 1 litre. 50 ml. of this diluted solution was boiled with an excess of iron (III) solution. The resulting solution required 12 ml. of 0.02 M KMnO4 solution for complete oxidation of iron (II). Calculate the weight of hydroxylamine in one litre of the original solution. (H = 1, N = 14, O = 16, K = 39, Mn = 55, Fe = 56) (1982 - 4 Marks)
Ans. Sol. Given 2NH2 OH + 4Fe3+ → N2O + H2O + 4Fe2++ 4H+ .....(i)
and MnO4- + 5Fe 2+ + 8H+ → Mn2+ + 5Fe3++ 4H2O ..(ii)
∴ 10 NH2OH + 4MnO4- + 12H+ → 5N2O + 21H2O+ 4Mn2+ [On multiplying (i) by 5 and (ii) by 4 and then adding the resulting equations]
Molecular weight of NH2OH = 33
Thus 4000 ml of 1M MnO4– would react with NH2OH = 330g
∴ 12 ml of 0.02 M KMnO4 would react with NH2OH
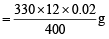
∴ Amount of NH2OH present in 1000 ml of diluted solution
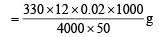
Since 10 ml of sample of hydroxylamine is diluted to one litre
∴ Amount of hydroxyl amine in one litre of original solution
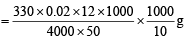 = 39.6 g
= 39.6 g
Q. 17. The density of a 3 M sodium thiosulphate solution (Na2S2O3) is 1.25 g per ml. Calculate (i) the percentage by weight of sodium thiosulphate, (ii) the mole fraction of sodium thiosulphate and (iii) the molalities of Na+ and S2O32– ions. (1983 - 5 Marks)
Ans. Sol. TIPS/Formulae :
(i) Mole fraction =
(ii) 1 mole of Na2S2O3 gives 2 moles of Na+ and 1 mole of S2O32– Molecular wt. of sodium thiosulphate solution (Na2S2O3)
= 23 × 2 + 32 × 2 + 16 × 3= 158
(i) The percentage by weight of Na2S2O3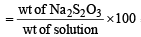
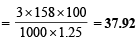
[Wt. of Na2S2O3 = Molarity × Mol wt] (ii) Mass of 1 litre solution = 1.25 × 1000 g = 1250 g
[∵density = 1.25g/l]
Mole fraction of Na2S2O3
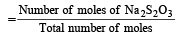
Moles of water 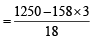 = 43.1
= 43.1
Mole fraction of Na2S2O3 = 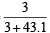 = 0.065
= 0.065
(iii) 1 mole of sodium thiosulphate (Na2S2O3) yields 2 moles of Na+ and 1 mole of S2 O32- .
Molality of Na2S2O3 = 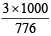 = 3.87
= 3.87
Molality of Na+ = 3.87 × 2 = 7.74m
Molality of S2 O32- = 3.87m
Q. 18. 4.08 g of a mixture of BaO and an unknown carbonate MCO3 was heated strongly. The residue weighed 3.64 g. This was dissolved in 100 ml of 1 N HCl. The excess acid required 16 ml of 2.5 N NaOH solution for complete neutralization.
Identify the metal M. (1983 - 4 Marks) (At. wt. H = 1, C = 12, O = 16, Cl = 35.5, Ba = 138)
Ans. Sol. Weight of MCO3 and BaO = 4.08 g (given)
Weight of residue = 3.64 g (given)
∴ Weight of CO2 evolved on heating = (4.08 – 3.64) g = 0.44 g
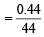 = 0.01 mole
= 0.01 mole
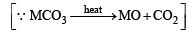
Volume of 1N HCl in which residue is dissolved = 100 ml
Volume of 1N HCl used for dissolution = (100 – 2.5 × 16) ml = 60 ml
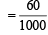 = 0.06 equivalents
= 0.06 equivalents
The chemical equation for dissolution can be written as
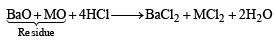
[Number of moles of BaO and MO = 1 + 1 = 2]
Number of moles of BaO + Number of moles of MO = 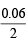
= 0.03
Number of moles of BaO = (0.03 – 0.01) = 0.02 moles
Molecular weight of BaO = 138 + 16 = 154
∴ Weight of BaO = (0.02 × 154) g = 3.08 g
Weight of MCO3 = (4.08 – 3.08) = 1.0 g
Since weight of 0.01 mole of MCO3 = 1.0 g
∴ Mol. wt. of MCO3 = 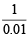 = 100
= 100
Hence atomic weight of unknown M = (100 – 60) = 40
The atomic weight of metal is 40 so the metal M is Ca.
Q. 19. Complete and balance the following reactions :
(i) Zn + NO3- → Zn2+ + NH +4 (1983 - 1 Mark)
(ii) Cr 2 O72- + C2H4O → C2H4O2 + Cr3+ (1983 - 1 Mark)
(iii) HNO3 + HCl → NO + Cl2 (1983 - 1 Mark)
(iv) Ce3+ + S2O82- → SO24- + Ce4+ (1983 - 1 Mark)
(v) Cl2 + OH– → Cl– + ClO– (1983 - 1 Mark)
(vi) Mn2+ + PbO2 → MnO -4 + H2O (1986 - 1 Mark)
(vii) S + OH– → S2– + S2O32- (1986 - 1 Mark)
(viii) ClO 3- + I– + H2SO4 → Cl– + HSO-4 (1986 - 1 Mark)
(ix) Ag++ AsH3 → H3AsO3 + H+ (1986 - 1 Mark)
Ans. Sol. TIPS/Formulae : Balance the atoms as well as charges by ion electron/ oxidation number method.
While balancing the equations, both the charges and atoms must balance.
(i) 4Zn + NO 3- + 10H+ —→ 4Zn2+ + NH +4 + 3H2O
(ii) Cr2O2-7 + 3C2H4O + 8H+ —→ 3C2H4O2 + 2Cr3+ + 4H2O
(iii) 2HNO3 + 6HCl —→ 2NO + 3Cl2 + 4H2O
(iv) 2Ce3+ + S2O82- —→ 2 SO42-+ 2Ce4+
(v) Cl2 + 2OH– —→ Cl– + ClO– + H2O
(vi) 2Mn2+ + 5PbO2 + 4H+ → 2 MnO -4 + 2H2O + 5Pb2+
(vii) 4S + 6OH– → 2S2– + S2 O32- + 3H2O
(viii) ClO 3- + 6I– + 6H2SO4 → Cl– + 6 HSO -4 + 3I2 + 3H2O
(ix) 6Ag++ AsH3 + 3H2O → 6Ag + H3AsO3 + 6H+
Q. 20. 2.68 × 10–3 moles of a solution containing an ion An+ require 1.61 × 10–3 moles of MnO-4 for the oxidation of An+ to AO3- in acid medium. What is the value of n? (1984 - 2 Marks)
Ans. Sol. TIPS/Formulae : Equivalents of A oxidised = Equivalents of A reduced.
Since in acidic medium, An+ is oxidised to AO3–, the change in oxidation state from
(+5) to (+n) = 5 – n [∵ O.S. of A in AO3- =+5]
∴ Total number of electrons that have been given out during oxidation of 2.68 × 10–3 moles of An+
= 2.68 × 10–3 × (5 – n)
Thus the number of electrons added to reduce 1.61 × 10–3 moles of MnO-4 to Mn2+, i.e. (+7) to (+2) =1.61 × 10–3 × 5
[Number of electrons involved = + 7 – (+2) = 5]
∴ 1.61 × 10–3 × 5 = 2.68 × 10–3 × (5 – n)
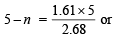
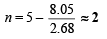
Q. 21. Five ml of 8N nitric acid, 4.8 ml of 5N hydrochloric acid and a certain volume of 17M sulphuric acid are mixed together and made upto 2litre. Thirty ml. of this acid mixture exactly neutralise 42.9 ml of sodium carbonate solution containing one gram of Na2CO3.10H2O in 100 ml. of water. Calculate the amount in gram of the sulphate ions in solution. (1985 - 4 Marks)
Ans. Sol. TIPS/Formulae : (i) Find normality of acid mixture and Na2CO3 . 10H2O.
Equate them to find volume of H2SO4.
(ii) Meq. of H2SO4 = V × N =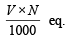
(iii) Equivalent of SO42– = equivalents of H2SO4 × Eq. wt. of SO4– –
N × V (ml.) = meq.
Acid mixture contains 5 ml of 8N, HNO3, 4.8 ml of 5N, HCl and say, ‘V’ ml of 17 M ≡ 34 N, H2SO4. [1MH2SO4 = 2N.H2SO4]
N of the acid mixture =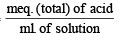
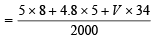 [Total volume = 2 L = 2000 ml]
[Total volume = 2 L = 2000 ml]
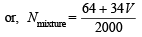
∵ Eq. of wt. of Na2CO3.10H2O =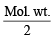
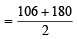 = 143
= 143
N of Na2CO3= 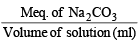
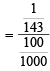
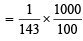 = 0.069N
= 0.069N
N1V1 = N2V2
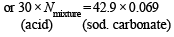
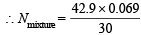
Hence 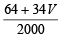 = 0.0986
= 0.0986
64 + 34 V = 0.0986 × 2000, 64 + 34 V = 197.2
34 V = 197.2 – 64.0 = 133.2 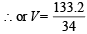 = 3.9 ml.
= 3.9 ml.
Hence meq. of H2SO4 = V × N of H2SO4
= 3.9 × 34 = 132.6 meq.
= 0.1326 eq. of H2SO4
= 0.1326 eq. of SO42-
= 0.1326 × 48 g of SO42-
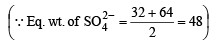
= 6.3648 g of SO42- are in 3.9 ml of 17M H2SO4
Q. 22. Arrange the following in increasing oxidation number of iodine. (1986 - 1 Mark)
I2, HI, HIO4, ICl
Ans. Sol. HI < I2 < ICl < HIO4; O.N. of I in I2 = 0, HI = –1, ICl = +1, HIO4 = +7.
Q. 23. (i) What is the weight of sodium bromate and molarity of solution necessary to prepare 85.5 ml of 0.672 N solution when the half-cell reaction is -
BrO3- + 6H+ + 6e– → Br– + 3H2O
(ii) What would be the weight as well as molarity if the half-cell reaction is : -
2 BrO3- + 12H+ + 10e– → Br2 + 6H2O (1987 - 5 Marks)
Ans. Sol. (i) From the given half-cell reaction, Here Eq. wt. of NaBrO3 = 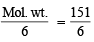 = 25.17
= 25.17
[∵ number of electron involved = 6]
Now we know that Meq. = Normality × Vol. in ml. = 85.5 × 0.672 = 57.456
Also Meq. = 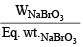 × 1000
× 1000
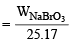 × 1000
× 1000
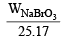 × 1000 = 57.456 g
× 1000 = 57.456 g
∴ WNaBrO3 = 1.446 g
Molarity of NaBrO3 =
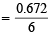 = 0.112 M
= 0.112 M
(ii) From the given half-cell reaction, Eq. wt. of NaBrO3 =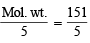 = 30.2
= 30.2
[Number of electron involved per BrO3– = 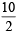 = 5]
= 5]
Thus, the amount of NaBrO3 required for preparing 1000 ml. of 1 N NaBrO3 = 30.2 g
∴ The amount of NaBrO3 required for preparing 85.5 ml of 0.672 N NaBrO3.
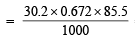 = 1.7532 g
= 1.7532 g
Hence, Molarity =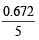 = 0.1344 M
= 0.1344 M
Q. 24. A sugar syrup of weight 214.2 g contains 34.2 g of sugar (C12H22O11). Calculate : (i) molal concentration and (ii) mole fraction of sugar in the syrup. (1988 - 2 Marks)
Ans. Sol. (i) Weight of sugar syrup = 214.2 g Weight of sugar in the syrup = 34.2 g
∴ Weight of water in the syrup = 214.2 – 34.2 = 180.0 g Mol. wt. of sugar, C12H22O11 = 342
∴ Molal concentration = 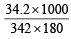 = 0.56
= 0.56
(ii) Mol. wt. of water, H2O = 18
∴ Mole fraction of sugar = 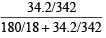
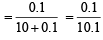 = 0.0099
= 0.0099
Q. 25. A sample of hydrazine sulphate (N2H6SO4) was dissolved in 100 ml. of water, 10 ml of this solution was reacted with excess of ferric chloride solution and warmed to complete the reaction. Ferrous ion formed was estimated and it required 20 ml. of M/50 potassium permanganate solution.
Estimate the amount of hydrazine sulphate in one litre of the solution. (1988 - 3 Marks)
Reaction : 4Fe3+ + N2H4 → N2 + 4Fe2+ + 4H+
MnO4- + 5Fe2+ + 8H+ → Mn2+ + 5Fe3+ + 4H2O.
Ans. Sol. TIPS/Formulae : No. of equivalents of KMnO4 = No. of equivatents of hydrazine sulphate.
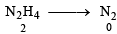
Change in oxidation state for each N2H4 = 2 × 2 = 4
Equivalent weight of N2H6SO4 =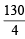 = 32.5
= 32.5
Normality of KMnO4 = 5 × 450 (∵ valence factor = 5)
Number of equivalents of KMnO4 = 20 ×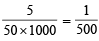 and if weight of hydrazin sulphate be x gm then equivalents of hydrazine sulphate =
and if weight of hydrazin sulphate be x gm then equivalents of hydrazine sulphate = 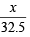
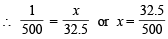 = 0.065 g
= 0.065 g
Hence wt. of N2H6SO4 in 10 ml solution = 0.065 g
∴ Wt. of N2H6SO4 in 1000 ml solution = 6.5 g
Q. 26. An equal volume of a reducing agent is titrated separately with 1M KMnO4 in acid neutral and alkaline media. The volumes of KMnO4 required are 20 ml. in acid, 33.4 ml. neutral and 100 ml. in alkaline media. Find out the oxidation state of manganese in each reduction product. Give the balanced equations for all the three half reactions. Find out the volume of 1M K2Cr2O7 consumed; if the same volume of the reducing agent is titrated in acid medium.(1989 - 5 Marks)
Ans. Sol. TIPS/Formulae : No. of equivalents of KMnO4 in neutral medium = No. of equivalents of reducing agent.
Assuming that KMnO4 shows the following changes during its oxidising nature.
Acidic medium Mn7+ + n1e– → Mna+ ∴ n1 = 7 – a
Neutral medium Mn7+ + n2e– → Mnb+ ∴ n2 = 7 – b
Alkaline medium Mn7+ + n3e– → Mnc+ ∴ n3 = 7 – c
Let V ml. of reducing agent be used for KMnO4 in different medium.
∴ Meq. of reducing agent
= Meq. of KMnO4 in acid mediumMeq. of KMnO4 in neutral medium
= Meq. of KMnO4 in alkaline medium= 1 × n1 × 20 = 1 × n2 × 33.4 = 1 × n3 × 100 = n1 = 1.667 n2 = 5 n3
Since n1, n2 and n3 are integers and n1 is not greater than 7
∴ n3 = 1 Hence n1 = 5 and n2 = 3
∴ Different oxidation states of Mn in Acidic medium Mn7+ + 5e– → Mna+ or a = + 2
Neutral medium Mn7+ + 3e– → Mnb+ or b = + 4
Alkaline medium Mn7+ + 1e– → Mnc+ or c = + 6
Further, same volume of reducing agent is treated with K2Cr2O7, and therefore Meq. of reducing agent = Meq. of K2Cr2O7
1 × 5 × 20= 1 × 6 × V [Q Cr+6 + 6e– → 2Cr+3]
V = 16.66 mL ∴ 1M = 6 × 1N
Q. 27. A mixture of H2C2O4 (oxalic acid) and NaHC2O4 weighing 2.02 g was dissolved in water and solution made upto one litre. Ten millilitres of the solution required 3.0 ml. of 0.1 N sodium hydroxide solution for complete neutralization. In another experiment, 10.0 ml. of the same solution, in hot dilute sulphuric acid medium. require 4.0 ml. of 0.1 N potassium permanganate solution for complete reaction.
Calculate the amount of H2C2O4 and NaHC2O4 in the mixture. (1990 - 5 Marks)
Ans. Sol. TIPS/Formulae : No. of equivalents of KMnO4
= No. of equivatents of reducing agents.
Case I. Reaction of NaOH with H2C2O4 and NaHC2O4.
(i) H2C2O4 + 2NaOH → Na2C2O4 + 2H2O
(ii) NaHC2O4 + NaOH → Na2C2O4 + H2O
Number of milliequivalents of NaOH = N × V = 3.0 × 0.1 = 0.3
∴ Combined normality of the mixture titrated with NaOH
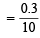 = 0.03
= 0.03
Case II. Reaction of C2O4– ion and KMnO4
(iii) 5C2O4– + MnO4– + 16H+ → 2Mn2+ + 10CO2 + 8H2O KMnO4 will react in same manner with both NaHC2O4 and H2C2O4 as it can be seen from the above reaction.
Number of milliequivalents of KMnO4 = 4.0 × 0.1 = 0.4
∴ Combined normality of the mixture titrated with KMnO4
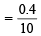 = 0.04
= 0.04
The difference (0.04 N – 0.03 N = 0.01 N) is due to NaHC2O4 The total normality of NaHC2O4 will be = 0.01 + 0.01 = 0.02 N From equation (ii) in case I.
Eq. wt. of NaHC2O4 = 112 Amount of NaHC2O4 in one litre of solution formed = 0.01 × 112 = 1.12 g and amount of H2C2O4 = 2.02 – Wt. of NaHC2O4 = 2.02 – 1.12 = 0.90 g
Q. 28. A solid mixture (5.0 g) consisting of lead nitrate and sodium nitrate was heated below 600ºC until the weight of the residue was constant. If the loss in weight is 28.0 per cent, find the amount of lead nitrate and sodium nitrate in the mixture. (1990 - 4 Marks)
Ans. Sol. TIPS/Formulae : Let the amount of NaNO3 in the mixture = x g
∴ The amount of Pb(NO3)2 in the mixture = (5 – x) g
Heating effect of sodium nitrate and lead nitrate
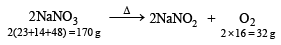
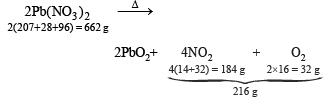
Now since, 170 g of NaNO3 gives = 32 g of O2
∴ xg of NaNO3 gives = 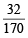 × x g of O2
× x g of O2
Similarly, 662 g of Pb(NO3)2 gives = 216 g of gases (NO2 + O2)
(5 – x) g of Pb(NO3)2 gives =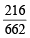 × (5 – x) g of gases(NO2 + O2)
× (5 – x) g of gases(NO2 + O2)
Actual loss, on heating, is 28% of 5 g of mixture
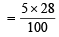 = 1.4 g
= 1.4 g
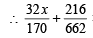 × (5 – x) = 1.4
× (5 – x) = 1.4
32 x × 662 + 216(5 – x) × 170 = 1.4 × 170 × 662
21184 x + 183600 – 36720 x = 157556 – 15536 x = – 26044, x = 1.676 g
Wt. of NaNO3 = 1.676 g and
Wt. of Pb(NO3)2 = 5 – 1.676 g = 3.324 g
|
446 docs|929 tests
|
















