JEE Advanced (Subjective Type Questions): Solutions- 2 | Chapter-wise Tests for JEE Main & Advanced PDF Download
7. The vapour pressure of ethanol and methanol are 44.5 mm and 88.7 Hg respectively. An ideal solution is formed at the same temperature by mixing 60 g of ethanol with 40 g of methanol. Calculate the total vapour pressure of the solution and the mole fraction of methanol in the vapour. (1986 - 4 Marks)
Ans : 66.17 mm, 0.65
Solution :
TIPS/Formulae :
Ptotal = pA + pB
Molecular weight of CH3OH = 12 + 3 + 16 + 1 = 32
Molecular weight of C2H5OH= 24 + 5 + 16 + 1 = 46
According to Raoult’s law
Ptotal = p1 + p2
where Ptotal = Total vapour pressure of the solution
p1 = Partial vapour pressure of one component
p2 = Partial vapour pressure of other component
Again, p1 = Vapour pressure  × mole fraction
× mole fraction
Similarly, p2 = Vapour pressure 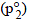 x mole fraction
x mole fraction
Mole fraction of CH3OH = 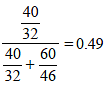
Mole fraction of ethanol = 
NOTE THIS STEP : Thus now let us first calculate the partial vapour pressures, i.e., p1 and p2 of the two component.
Partial vapour pressure of CH3OH(p1)= 88.7 × 0.49 = 43.48 mm
Partial vapour pressure of C2H5OH(p2) = 44.5 × 0.51 = 22.69 mm
∴ Total vapour pressure of the solution = 43.48 + 22.69 mm = 66.17 mm
Mole fraction of CH3OH in vapour = 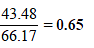
8. The vapour pressure of a dilute aqueous solution of glucose (C6H12O6) is 750 mm of mercury at 373 K. Calculate (i) molality and (ii) mole fraction of the solution. (1989 - 3 Marks)
Ans : 0.7503 mol/kg, 0.9868
Solution :
TIPS/Formulae :
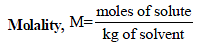
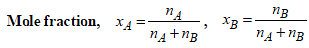
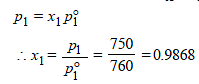
x2 (solute) = 1 – 0.9868 = 0.0132
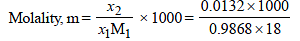
= 0.7503 mol kg–1
9. The vapour pressure of pure benzene at a certain temperature is 640 mm Hg. A non-volatile non-electrolyte solid weighing 2.175 g is added to 39.0 g of benzene. The vapour pressure of the solution is 600 mm Hg. What is the molecular weight of the solid substance? (1990 - 3 Marks)
Ans : 65.25
Solution :
TIPS/Formulae :
According to Raoult’s law,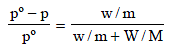
Here, pº = 640 mm
p = 600 mm
w= 2.175 g
= 39.0
m = ?
M = 78
Substituting the various values in the above equation for Roult's law :
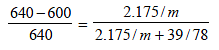

10. The degree of dissociation of calcium nitrate in a dilute aqueous solution, containing 7.0 g. of the salt per 100 gm of water at 100ºC is 70%. If the vapour pressure of water at 100ºC is 760 mm, calculate the vapour pressure of the solution. (1991 - 4 Marks)
Ans : 746.3 mm Hg
Solution :
TIPS/Formulae : First find moles of Ca(NO3)2 and water.
Then use the expression 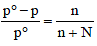 to find vapour pressure of solution.
to find vapour pressure of solution.
Let initially 1 mole of Ca(NO3)2 is taken
Degree of dissociation of Ca(NO3)2 = 70/100 = 0.7
Ionisation of Ca(NO3)2 can be represented as
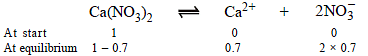
∴ Total number of moles in the solution at equilibrium = (1 – 0.7) + 0.7 + 2 × 0.7 = 2.4
No. of moles when the solution contains 1 gm of calcium nitrate instead of 1 mole of the salt
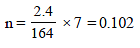
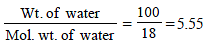

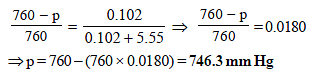
11. Addition of 0.643 g of a compound to 50 ml. of benzene (density : 0.879 g/ml.) lowers the freezing point from 5.51ºC to 5.03ºC. If Kf for benzene is 5.12 K kg mol–1, calculate the molecular weight of the compound. (1992 - 2 Marks)
Ans : 156.056
Solution :
TIPS/Formulae :
Given Wt. of benzene (solvent),
W = Volume × density = 50 × 0.879 = 43.95 g
Wt. of compound (solute), w = 0.643 g
Mol. wt. of benzene, M = 78; Mol. wt. of solute, m = ?
Depression in freezing point, DTf = 5.51 – 5.03 = 0.48
Molal freezing constant, Kf = 5.12
Now we know that,
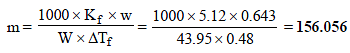
12. What weight of the non-volatile solute, urea (NH2 – CO – NH2) needs to be dissolved in 100g of water, in order to decrease the vapour pressure of water by 25%? What will be the molality of the solution? (1993 - 3 Marks)
Ans : 18.52 m
Solution :
TIPS/Formulae :
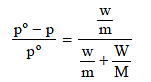
Here, w and m are wt. and molecular wt. of solute, W and M are wt. and molecular weight of solvent
p = Pressure of solution; pº = Normal vapour pressure
Let the initial (normal) pressure (pº) = p
∴ Pressure of solution = 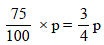
m = 60, M = 18, W = 100 gm

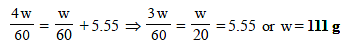

13. The molar volume of liquid benzene (density=0.877 g mL–1) increases by a factor of 2750 as it vaporises at 20°C and that of liquid toluene (density=0.867 g mL–1) increases by a factor of 7720 at 20°C. A solution of benzene and toluene at 20°C has a vapour pressure of 46.0 Torr. Find the mole fraction of benzene in the vapour above the solution. (1996 - 3 Marks)
Ans : 0.73
Solution :
TIPS/Formulae :
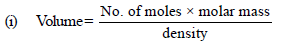
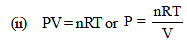
Volume of 1 mole of liq. benzene =78/0.877
Volume of 1 mole of toluene = 92/0.867
In vapour phase,
At 20°C, for 1 mole of benzene,
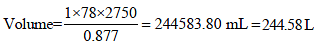
Similarly for 1mole of toluene,
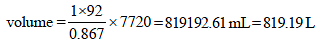
As we know that, PV = nRT
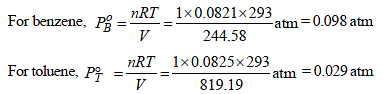
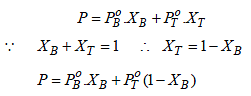
Total vapour-pressure = 46 torr = 46/760 = 0.060 atm
Thus, 0.060 = 0.098 XB + 0.029 (1 – XB)
⇒ 0.060 = 0.098 XB + 0.029 – 0.029 XB
⇒ 0.031 = 0.069 XB
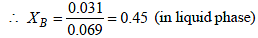
XB + XT = 1
XT = 1– 0.45 = 0.55 (in liquid phase)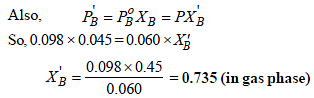
|
446 docs|930 tests
|

|
Explore Courses for JEE exam
|

|

















