Important Trigonometric Functions - 2 Formulas for JEE and NEET
Trigonometric Ratios :
A trigonometric ratio is a ratio of the lengths of two sides of a right triangle. The word trigonometry is derived from the ancient Greek language and means measurement of triangles. The three basic trigonometric ratios are sine, cosine and tangent, which are abbreviated as sin, cos and tan, respectively.
Trigonometric Ratios
Let us consider the right ΔABC shown below
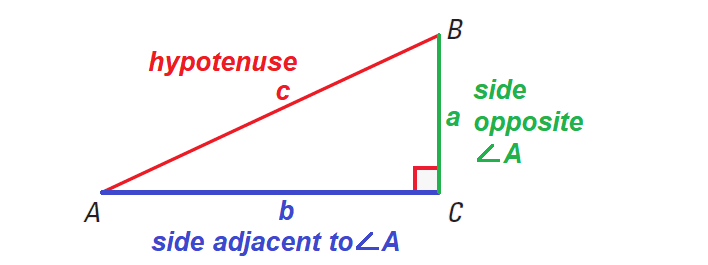
The sine, the cosine, and the tangent of the acute angle ∠A are defined as follows.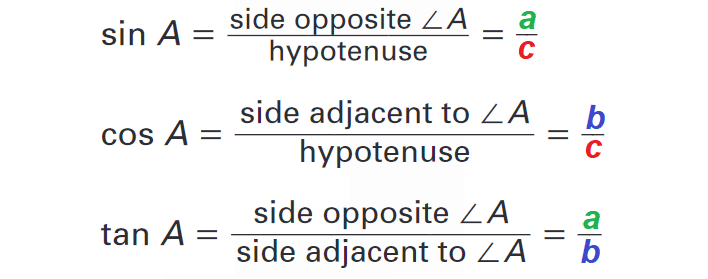
Note :
The value of a trigonometric ratio depends only on the measure of the acute angle, not on the particular right triangle that is used to compute the value.
Finding Trigonometric Ratios
Example 1 :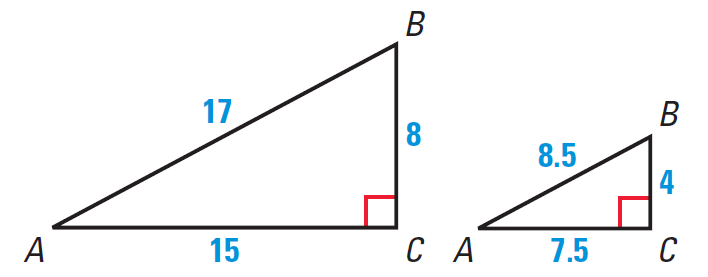
Compare the sine, the cosine, and the tangent ratios for ∠A in each triangle below.
Solution :
By the SSS Similarity Theorem, the triangles are similar. Their corresponding sides are in proportion, which implies that the trigonometric ratios for ∠A in each triangle are the same.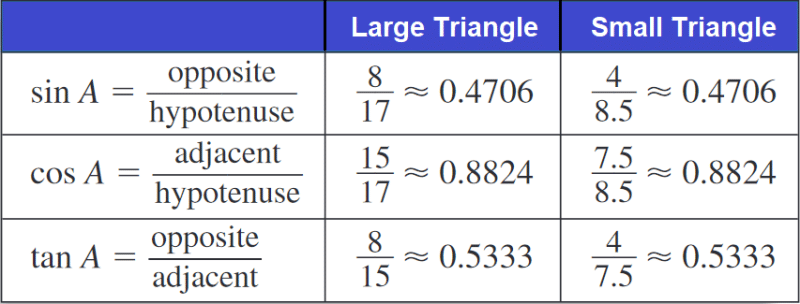
Trigonometric ratios are frequently expressed as decimal approximations.
Example 2 :
Find the sine, the cosine, and the tangent of the indicated angle.
a. ∠S
b. ∠R
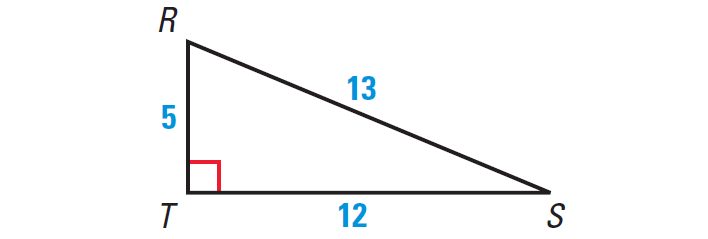
Solution (a) :
The length of the hypotenuse is 13. For ∠S, the length of the opposite side is 5, and the length of the adjacent side is 12.
Sin S = opp. / hyp. = 5 / 13 ≈ 0.3846
Cos S = adj. / hyp. = 12 / 13 ≈ 0.9231
Tan S = opp. / adj. = 5 / 12 ≈ 0.4167
Solution (b) :
The length of the hypotenuse is 13. For ∠R, the length of the opposite side is 12, and the length of the adjacent side is 5.
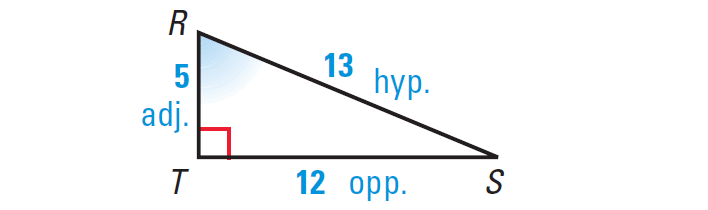
Sin R = opp. / hyp. = 12 / 13 ≈ 0.9231
Cos R = adj. / hyp. = 5 / 13 ≈ 0.3846
Tan R = opp. / adj. = 12 / 5 ≈ 2.4
Trigonometric Ratios for 45°
Example :
Find the sine, the cosine, and the tangent of 45°.
Solution :
Begin by sketching a 45°-45°-90° triangle. Because all such triangles are similar, we can make calculations simple by choosing 1 as the length of each leg. From the Pythagorean Theorem, it follows that the length of the hypotenuse is √2.
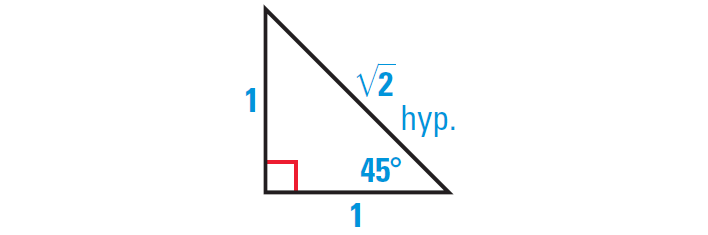
Sin 45° = opp. / hyp. = 1 / √2 = √2 / 2 ≈ 0.7071
Cos 45° = adj. / hyp. = 1 / √2 = √2 / 2 ≈ 0.7071
Tan 45° = opp. / adj. = 1 / 1 = 1
Trigonometric Ratios for 30°
Example:
Find the sine, the cosine, and the tangent of 30°.
Solution :
Begin by sketching a 30°-60°-90° triangle. To make the calculations simple, we can choose 1 as the length of the shorter leg. From 30°-60°-90° Triangle theorem, it follows that the length of the longer leg is √3 and the hypotenuse is 2.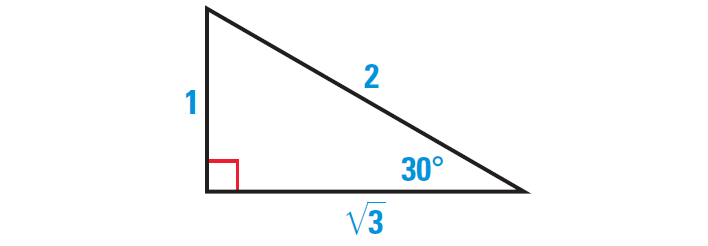
Sin 30° = opp. / hyp. = 1 / 2 = 0.5
Cos 30° = adj. / hyp. = √3 / 2 ≈ 0.8660
Tan 30° = opp. / adj. = 1 / √3 = √3 / 3 ≈ 0.5774
Trigonometric Identities
A trigonometric identity is an equation involving trigonometric ratios that is true for all acute angles.
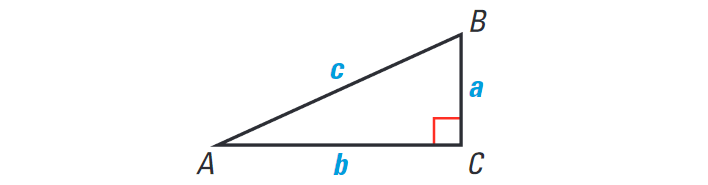
In the triangle shown above, we can have the following important trigonometric identities.
sin2A + cos2A = 1
tan A = sin A / cos A
Using Trigonometric Ratios in Real Life
Suppose you stand and look up at a point in the distance, such as the top of the tree in the example given below. The angle that your line of sight makes with a line drawn horizontally is called the angle of elevation.
Example :
Jacob is measuring the height of a Sitka spruce tree in North Carolina. He stands 45 feet from the base of the tree. He measures the angle of elevation from a point on the ground to the top of the tree to be 59°. How can he estimate the height of the tree ?
Solution : 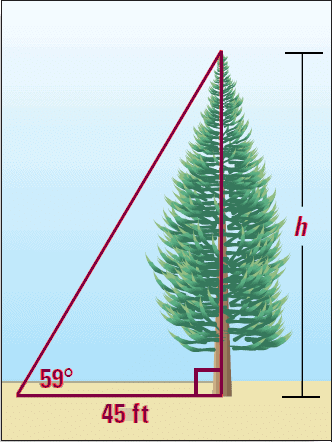
To estimate the height of the tree, Jacob can write a trigonometric ratio that involves the height h and the known length of 45 feet.
In the above right triangle, for the angle 59°, h is opposite side and the side has length 45 ft is adjacent side.
The trigonometric ratio that involves opposite side and adjacent side is tangent.
Write ratio :
tan 59° = opp. / adj.
Substitute.
tan 59° = h / 45
Multiply each side by 45.
45 ⋅ tan 59° = h
Use calculator or table to find the value of tan 59° and substitute.
45 ⋅ 1.6643 = h
Simplify.
74.9 ≈ h
Hence, the tree is about 75 feet tall.
Estimating a Distance
Example :
The escalator at the University Metro Rail Station near University of Miami in Coral Gables, Florida rises 76 feet at a 30° angle as shown in the diagram below. Find the distance d a person travels on the escalator stairs. 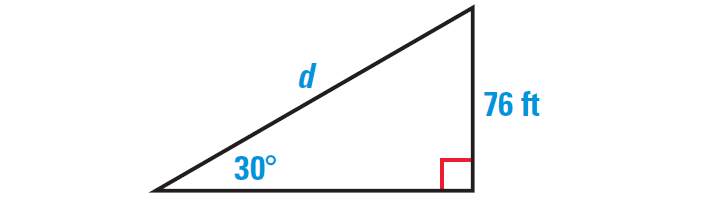
Solution :
To find the distance d a person travels on the escalator stairs, we can write a trigonometric ratio that involves the side has length d and the known length of 76 feet.
In the above right triangle, for the angle 30°, d is hypotenuse and the side has length 76 feet is opposite side.
The trigonometric ratio that involves opposite side and hypotenuse is sin.
Write ratio :
sin 30° = opp. / hyp.
Substitute.
sin 30° = 76 / d
Multiply each side by d.
d ⋅ sin 30° = 76
Substitute 0.5 for sin 30°.
d ⋅ 0.5 = 76
Divide each side by 0.5
d = 76 / 0.5
d = 152
Hence, a person travels 152 feet on the escalator stairs.
|
209 videos|443 docs|143 tests
|
FAQs on Important Trigonometric Functions - 2 Formulas for JEE and NEET
| 1. What are the basic trigonometric functions? |  |
| 2. How do I calculate the sine, cosine, and tangent of an angle? |  |
| 3. What is the difference between cosecant, secant, and cotangent? |  |
| 4. How can I use trigonometric functions to solve real-life problems? |  |
| 5. Are there any trigonometric identities that can simplify calculations? |  |
|
209 videos|443 docs|143 tests
|

|
Explore Courses for JEE exam
|

|


















