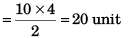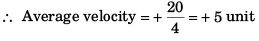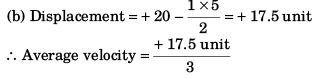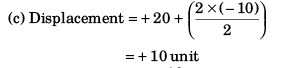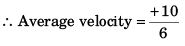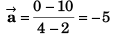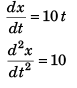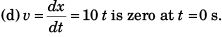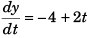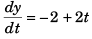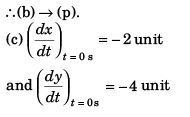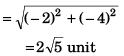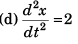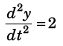Match the Columns
Ques 1: Match the following two columns.
Column I
| Column II
|
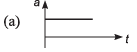 | (p) speed must be increasing
|
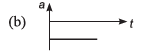 | (q) speed must be decreasing
|
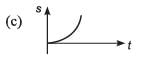 | (r) speed may be increasing
|
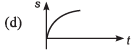 | (s) speed may be decreasing
|
Ans: (a) → r,s (b) → r,s (c) → p (d) → q
Sol:
(a)
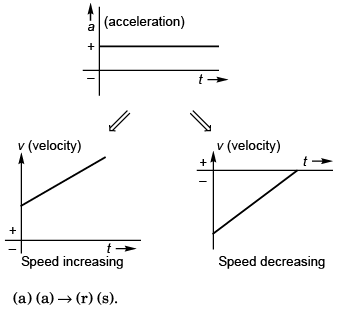
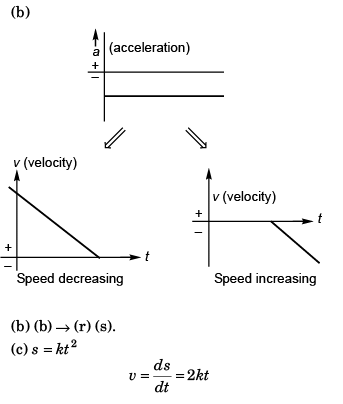
∴ v must be increasing with time.
⇒ speed must also be increasing with time.
(c) → (p).
(d) Slope is + ive and decreasing.
∴ Velocity must be decreasing with time.
⇒ speed must also be decreasing with time. (d) → (q).
Ques 2: Match the following two columns.
Column I
| Column II
|
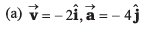 | (p) speed increasing
|
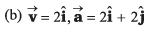 | (q) speed decreasing
|
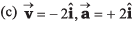 | (r) speed constant
|
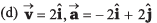 | (s) Nothing can be said
|
Ans: (a) → P (b) → p (c) → q (d) → q
Sol:
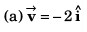
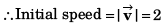
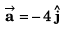
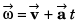
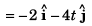
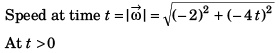
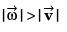
Speed increasing.
∴ (a) → (p).
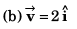
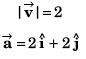
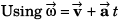
∴ 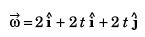
∴ 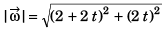
As t > 0, 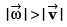
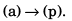
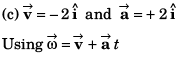
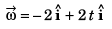
or 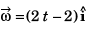
∴ 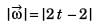
At t = 0 s 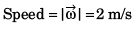
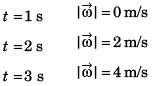
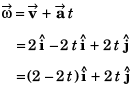
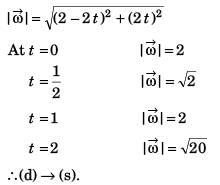
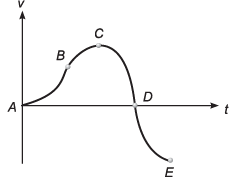
Ques 3: The velocity-time graph of a particle moving along X-axis is shown in figure. Match the entries of Column I with entries of Column II.
Column I
| Column II
|
(a) For AB, particle is
| (p) Moving in +ve X-direction with increasing speed
|
(b) For BC, particle is
| (q) Moving in +ve X-direction with decreasing speed
|
(c) For CD, particle is
| (r) Moving in -ve X-direction with increasing speed
|
(d) For ZXE, particle is
| (s) Moving in +ve X-direction with decreasing speed
|
Ans: (a) → P (b) → p (c) → q (d) → r
Sol: (a) From A to B, v is increasing and area is + ive.
∴ (a) → (p).
(b) From B to C, v is increasing and area is + ive.
∴ (b) → (p).
(c) From C to D, v is decreasing while area is + ive.
∴ (c) → (q).
(d) From D to E, v is - ive and  is
is
increasing, area is -ive.
∴ (d) → (r).
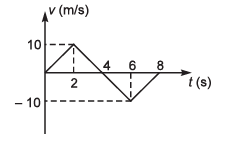
Ques 4: Corresponding to velocity-time graph in one dimensional motion of a particle is shown in figure, match the following two columns.
Column I
| Column II
|
(a) Average velocity between zero sec and 4 s
| (p) 10 SI units
|
(b) Average acceleration between 1 s and 4 s
| (q) 2.5 SI units
|
(c) Average speed between zero
| (r) 5 SI units
|
(d) Rate of change of speed at 4s
| (s) None
|
Ans: (a) → (r) (b) → (s) (c) → (r) (d) → (r)
Sol: (a) Displacement = Area 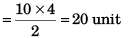
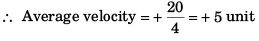
i.e., (a) → (r).
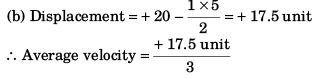
= 5.83 unit
∴(b) → (s)
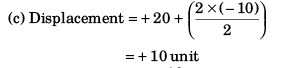
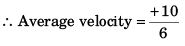
∴ (c) → (s)
(d) Rate of change of velocity at t = 4 s
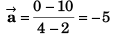
∴ Rate of change of speed at
t = 4 s would be 5.
i.e., (d) ∴ (r).
Ques 5: A particle is moving along x-axis. Its x-coordinate varies with time as: x = -20 + 5t2
For the given equation match the following two columns
Column I
| Column II
|
(a) Particle will cross the origin at
| (p) zero sec
|
(b) At what time velocity and acceleration are equal
| (q) 1 s
|
(c) At what time particle changes its direction of motion
| (r) 2 s
|
(d) At what time velocity is zero
| (s) None
|
Ans: (a) → (r) (b) → (q) (c) → (s) (d) → (p)
Sol: (a) x = - 20 + 5t2
0 = - 20 + 5t2
⇒ t = 2 s
i.e., (a) → (r).
(b) x = - 20 + 5t2
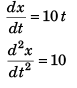
Velocity will be numerically equal to acceleration at t = 1 s.
Thus (b) → (q).
(c) At t = 0 s x = - 20 m
t = 1 s x = - 15 m
t = 2 s x = 0 m
t = 3 s x = + 25 m
Particle is always moving along + ive x-axis.
∴ (c) → (s).
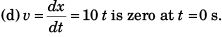
∴ (d) → (p).
Ques 6: x and y-coordinates of particle movingin x - y plane are,
x = 1 - 2t + t2 and y = 4 - 4t + t2
For the given situation match the following two columns.
Column I
| Column II
|
(a) y - component of velocity when it crosses the y-axis
| (p) + 2 SI unit
|
(h) x - component of velocity when it crosses the x-axis
| (q) - 2 SI units
|
(c) Initial velocity of particle
| (r) + 4 SI units
|
(d) Initial acceleration of particle
| (s) None
|
Ans: (a) → (q) (b) → (p) (c) → (s) (d) → (s)
Sol: (a) x = 1 - 2t + t2
0 = 1 - 2t + t2 [for particle to cross y-axis]
i.e., t = 1 s
Y = 4 - 4t + t2
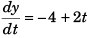
= - 4 + (2 x 1) [at t = 1 s]
= - 2 unit
∴ (a) → (q).
(b) Y = 4 - 4t + t2
0 = 4 - 4t + t2 [For particle to cross x-axis]
i.e., t = 2 s
x = 1 - 2t + t2
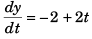
= - 2 + (2 x 2) [at t = 2 s]
= + 2 unit
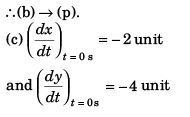
∴ Initial velocity of particle
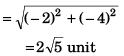
i.e., (c) → (s).
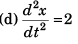
and 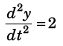
∴ Initial acceleration of particle = 2√2 unit
i.e., (d) → (s).






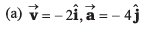
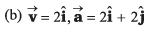
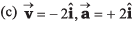
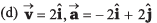
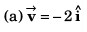
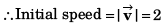
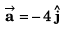
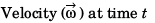
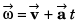
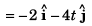
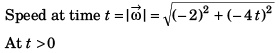
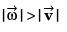
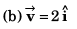
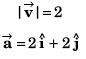
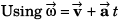
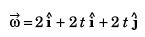
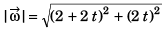
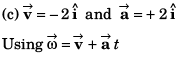
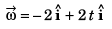
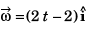
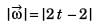
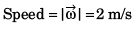
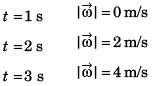
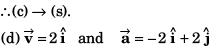
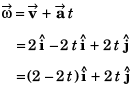
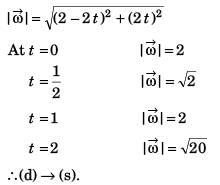

 is
is