Definition of the Definite Integral | Calculus - Mathematics PDF Download
Definition Of The Definite Integral
In this section we will formally define the definite integral and give many of the properties of definite integrals. Let’s start off with the definition of a definite integral.
Definite Integral
Given a function f(x) that is continuous on the interval [a,b] we divide the interval into nn subintervals of equal width, Δx, and from each interval choose a point,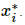 Then the definite integral of f(x) from a to b is
Then the definite integral of f(x) from a to b is
The definite integral is defined to be exactly the limit and summation that we looked at in the last section to find the net area between a function and the x-axis. Also note that the notation for the definite integral is very similar to the notation for an indefinite integral. The reason for this will be apparent eventually.
There is also a little bit of terminology that we should get out of the way here. The number “a” that is at the bottom of the integral sign is called the lower limit of the integral and the number “b” at the top of the integral sign is called the upper limit of the integral. Also, despite the fact that a and b were given as an interval the lower limit does not necessarily need to be smaller than the upper limit. Collectively we’ll often call aa and bb the interval of integration.
Example 1: Using the definition of the definite integral compute the following.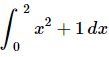
Ans: First, we can’t actually use the definition unless we determine which points in each interval that well use for 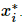 In order to make our life easier we’ll use the right endpoints of each interval.
In order to make our life easier we’ll use the right endpoints of each interval.
From the previous section we know that for a general n the width of each subinterval is,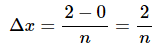
The subintervals are then,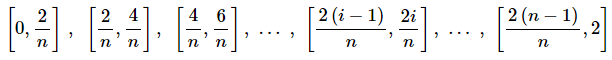
As we can see the right endpoint of the ith subinterval is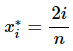
The summation in the definition of the definite integral is then,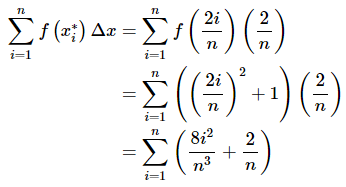
Now, we are going to have to take a limit of this. That means that we are going to need to “evaluate” this summation. In other words, we are going to have to use the formulas given in the summation notation review to eliminate the actual summation and get a formula for this for a general n .
To do this we will need to recognize that n is a constant as far as the summation notation is concerned. As we cycle through the integers from 1 to n in the summation only i changes and so anything that isn’t an ii will be a constant and can be factored out of the summation. In particular any n that is in the summation can be factored out if we need to.
Here is the summation “evaluation”.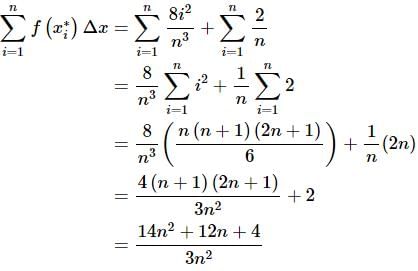
We can now compute the definite integral.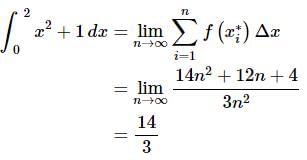
We’ve seen several methods for dealing with the limit in this problem so we’ll leave it to you to verify the results.
Wow, that was a lot of work for a fairly simple function. There is a much simpler way of evaluating these and we will get to it eventually. The main purpose to this section is to get the main properties and facts about the definite integral out of the way. We’ll discuss how we compute these in practice starting with the next section.
So, let’s start taking a look at some of the properties of the definite integral.
Properties
1. We can interchange the limits on any definite integral, all that we need to do is tack a minus sign onto the integral when we do.
2. If the upper and lower limits are the same then there is no work to do, the integral is zero.
3. where c is any number. So, as with limits, derivatives, and indefinite integrals we can factor out a constant.
4. We can break up definite integrals across a sum or difference.
5. where c is any number. This property is more important than we might realize at first. One of the main uses of this property is to tell us how we can integrate a function over the adjacent intervals, [a,c] and [c,b]. Note however that c doesn’t need to be between a and b.
6. The point of this property is to notice that as long as the function and limits are the same the variable of integration that we use in the definite integral won’t affect the answer.
See the Proof of Various Integral Properties section of the Extras chapter for the proof of properties 1 – 4. Property 5 is not easy to prove and so is not shown there. Property 6 is not really a property in the full sense of the word. It is only here to acknowledge that as long as the function and limits are the same it doesn’t matter what letter we use for the variable. The answer will be the same. Let’s do a couple of examples dealing with these properties.
Example 2: Use the results from the first example to evaluate each of the following.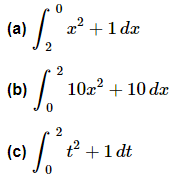
Ans: All of the solutions to these problems will rely on the fact we proved in the first example. Namely that,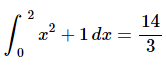
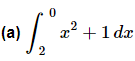
Ans: (a) In this case the only difference between the two is that the limits have interchanged. So, using the first property gives,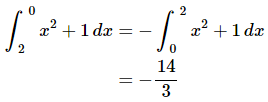
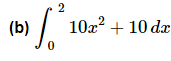
Ans: (b) For this part notice that we can factor a 10 out of both terms and then out of the integral using the third property.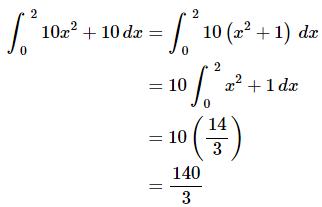
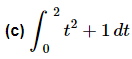
Ans: In this case the only difference is the letter used and so this is just going to use property 6.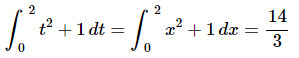
Example 3: Evaluate the following definite integral.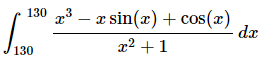
Ans: There really isn’t anything to do with this integral once we notice that the limits are the same. Using the second property this is,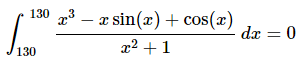
Example 4: Given that 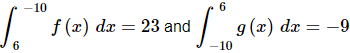 determine the value of
determine the value of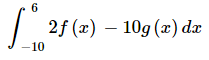
Ans: We will first need to use the fourth property to break up the integral and the third property to factor out the constants.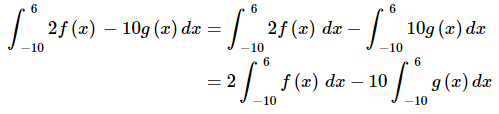
Now notice that the limits on the first integral are interchanged with the limits on the given integral so switch them using the first property above (and adding a minus sign of course). Once this is done we can plug in the known values of the integrals.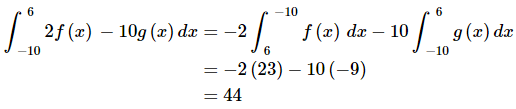
Example 5: Given that 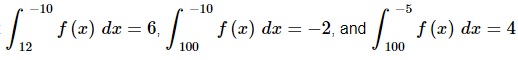 determine the value of
determine the value of 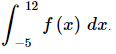
Ans: This example is mostly an example of property 5 although there are a couple of uses of property 1 in the solution as well.
We need to figure out how to correctly break up the integral using property 5 to allow us to use the given pieces of information. First, we’ll note that there is an integral that has a “-5” in one of the limits. It’s not the lower limit, but we can use property 1 to correct that eventually. The other limit is 100 so this is the number c that we’ll use in property 5.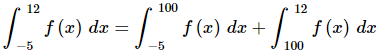
We’ll be able to get the value of the first integral, but the second still isn’t in the list of know integrals. However, we do have second integral that has a limit of 100 in it. The other limit for this second integral is -10 and this will be c in this application of property 5.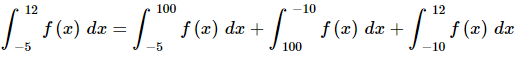
At this point all that we need to do is use the property 1 on the first and third integral to get the limits to match up with the known integrals. After that we can plug in for the known integrals.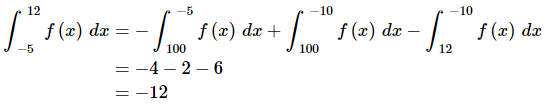
More Properties
7. is any number.
8. If
9. If
10. If
11.
Interpretations of Definite Integral
There are a couple of quick interpretations of the definite integral that we can give here.
First, as we alluded to in the previous section one possible interpretation of the definite integral is to give the net area between the graph of f (x) and the x- axis on the interval [a,b]. So, the net area between the graph of 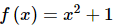 and the x- axis on [0,2] is,
and the x- axis on [0,2] is,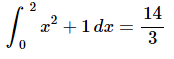
If you look back in the last section this was the exact area that was given for the initial set of problems that we looked at in this area.
Another interpretation is sometimes called the Net Change Theorem. This interpretation says that if f(x) is some quantity (so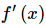 is the rate of change of f (x) then,
is the rate of change of f (x) then,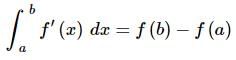
is the net change in f(x) on the interval [a,b]. In other words, compute the definite integral of a rate of change and you’ll get the net change in the quantity. We can see that the value of the definite integral, f (b) - f (a) does in fact give us the net change in f (x) and so there really isn’t anything to prove with this statement. This is really just an acknowledgment of what the definite integral of a rate of change tells us.
So as a quick example, if V (t) is the volume of water in a tank then,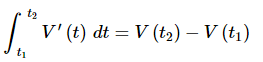
is the net change in the volume as we go from time t1 to time t2.
Likewise, if s (t) is the function giving the position of some object at time t we know that the velocity of the object at any time 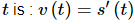 Therefore, the displacement of the object time t1 to time t2 is,
Therefore, the displacement of the object time t1 to time t2 is,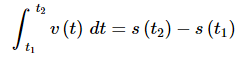
Note that in this case if v(t) is both positive and negative (i.e. the object moves to both the right and left) in the time frame this will NOT give the total distance traveled. It will only give the displacement, i.e. the difference between where the object started and where it ended up. To get the total distance traveled by an object we’d have to compute,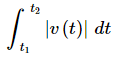
It is important to note here that the Net Change Theorem only really makes sense if we’re integrating a derivative of a function.
Fundamental Theorem of Calculus, Part I
As noted by the title above this is only the first part to the Fundamental Theorem of Calculus. We will give the second part in the next section as it is the key to easily computing definite integrals and that is the subject of the next section.
The first part of the Fundamental Theorem of Calculus tells us how to differentiate certain types of definite integrals and it also tells us about the very close relationship between integrals and derivatives.
Fundamental Theorem of Calculus, Part I
If f(x) is continuous on [a,b] then,
is continuous on [a,b] and it is differentiable on (a,b) and that,
An alternate notation for the derivative portion of this is,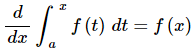
Example 6: Differentiate each of the following.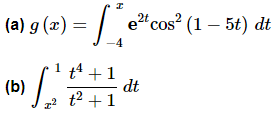
Ans: 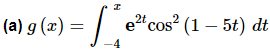
This one is nothing more than a quick application of the Fundamental Theorem of Calculus.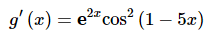
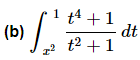
Ans: This one needs a little work before we can use the Fundamental Theorem of Calculus. The first thing to notice is that the Fundamental Theorem of Calculus requires the lower limit to be a constant and the upper limit to be the variable. So, using a property of definite integrals we can interchange the limits of the integral we just need to remember to add in a minus sign after we do that. Doing this gives,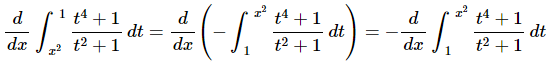
The next thing to notice is that the Fundamental Theorem of Calculus also requires an x in the upper limit of integration and we’ve got x2. To do this derivative we’re going to need the following version of the chain rule.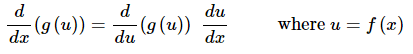
So, if we let u= x2 we use the chain rule to get,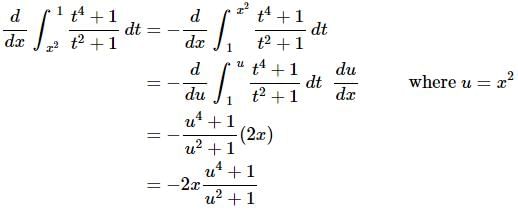
The final step is to get everything back in terms of x.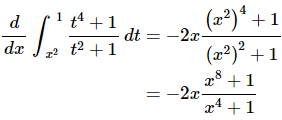
Using the chain rule as we did in the last part of this example we can derive some general formulas for some more complicated problems.
First,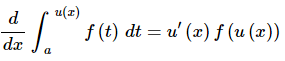
This is simply the chain rule for these kinds of problems.
Next, we can get a formula for integrals in which the upper limit is a constant and the lower limit is a function of x. All we need to do here is interchange the limits on the integral (adding in a minus sign of course) and then using the formula above to get,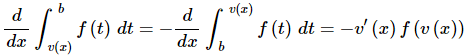
Finally, we can also get a version for both limits being functions of x. In this case we’ll need to use Property 5 above to break up the integral as follows,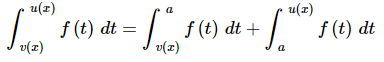
We can use pretty much any value of a when we break up the integral. The only thing that we need to avoid is to make sure that f(a) exists. So, assuming that f(a) exists after we break up the integral we can then differentiate and use the two formulas above to get,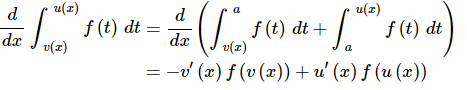
Example 7: Differentiate the following integral.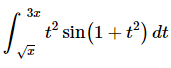
Ans: This will use the final formula that we derived above.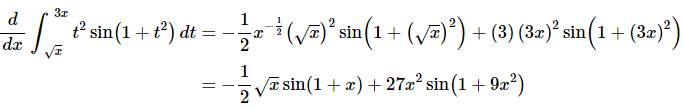
Practice problems: Definition Of The Definite Integral
For problems 1 & 2 use the definition of the definite integral to evaluate the integral. Use the right end point of each interval for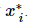
Q.1. Use the definition of the definite integral to evaluate the integral. Use the right end point of each interval for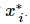
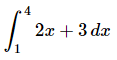
Ans: The width of each subinterval will be,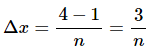
The subintervals for the interval [1,4] are then, From this it looks like the right end point, and hence
From this it looks like the right end point, and hence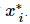 , of the general subinterval is,
, of the general subinterval is,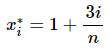
The summation in the definition of the definite integral is then,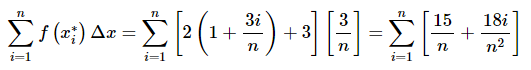
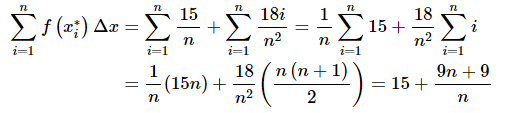
Finally, we can use the definition of the definite integral to determine the value of the integral.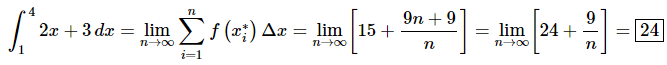
Q.2. Use the definition of the definite integral to evaluate the integral. Use the right end point of each interval for 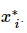
Ans: The width of each subinterval will be,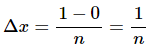
The subintervals for the interval [ 0,1] are then,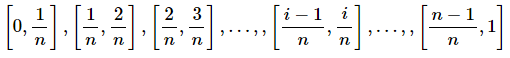
From this it looks like the right end point, and hence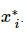 of the general subinterval is,
of the general subinterval is,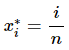
The summation in the definition of the definite integral is then,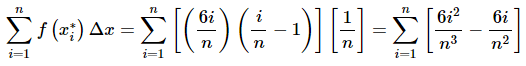
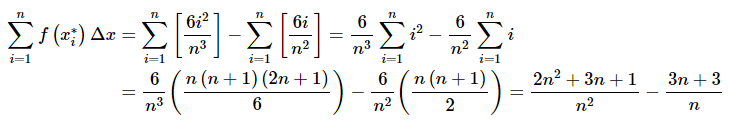
Finally, we can use the definition of the definite integral to determine the value of the integral.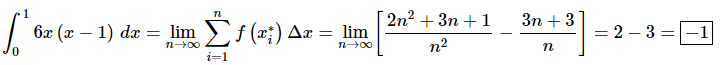
Q.3. Evaluate : 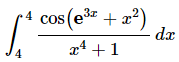
Ans: There really isn’t much to this problem other than use Property 2 from the notes on this section.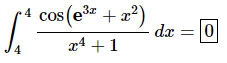
For problems 4 & 5 determine the value of the given integral given that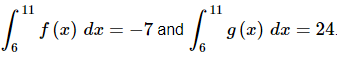
Q.4. Determine the value of 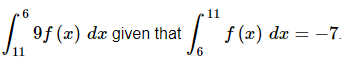
Ans: There really isn’t much to this problem other than use the properties from the notes of this section until we get the given interval at which point we use the given value.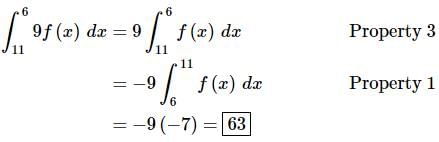
Q.5. Determine the value of 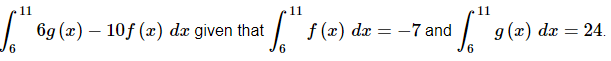
Ans: There really isn’t much to this problem other than use the properties from the notes of this section until we get the given intervals at which point we use the given values.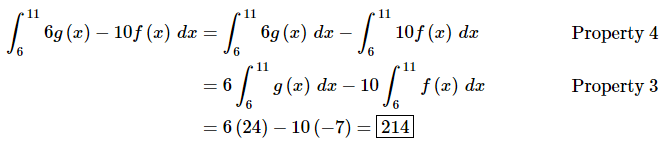
Q.6. Determine the value of 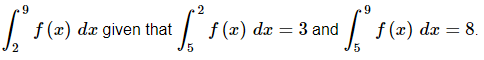
Ans: First we need to use Property 5 from the notes of this section to break up the integral into two integrals that use the same limits as the integrals given in the problem statement.
Note that we won’t worry about whether the limits are in correct place at this point.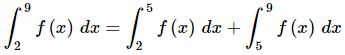
Finally, all we need to do is use Property 1 from the notes of this section to interchange the limits on the first integral so they match up with the limits on the given integral. We can then use the given values to determine the value of the integral.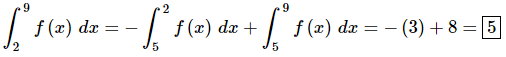
Q.7. Determine the value of 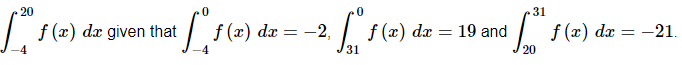
Ans: First we need to use Property 5 from the notes of this section to break up the integral into three integrals that use the same limits as the integrals given in the problem statement.
Note that we won’t worry about whether the limits are in correct place at this point.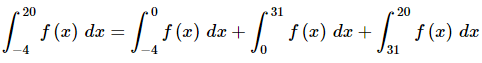
Finally, all we need to do is use Property 1 from the notes of this section to interchange the limits on the second and third integrals so they match up with the limits on the given integral. We can then use the given values to determine the value of the integral.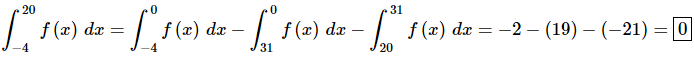
For problems 8 & 9 sketch the graph of the integrand and use the area interpretation of the definite integral to determine the value of the integral.
Q.8. For 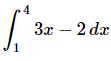 sketch the graph of the integrand and use the area interpretation of the definite integral to determine the value of the integral.
sketch the graph of the integrand and use the area interpretation of the definite integral to determine the value of the integral.
Ans: Here is the graph of the integrand, f(x)=3x−2, on the interval [1,4].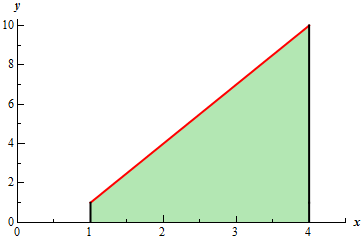
Now, we know that the integral is simply the area between the line and the x -axis and so we should be able to use basic area formulas to help us determine the value of the integral. Here is a “modified” graph that will help with this.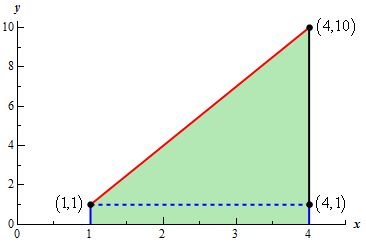
From this sketch we can see that we can think of this area as a rectangle with width 3 and height 1 and a triangle with base 3 and height 9. The value of the integral will then be the sum of the areas of the rectangle and the triangle.
Here is the value of the integral,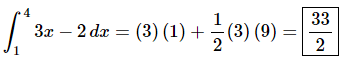
Q.9. For 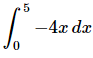 sketch the graph of the integrand and use the area interpretation of the definite integral to determine the value of the integral.
sketch the graph of the integrand and use the area interpretation of the definite integral to determine the value of the integral.
Ans: Here is the graph of the integrand, f(x)=−4x on the interval [0,5].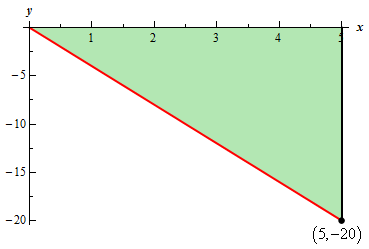
Now, we know that the integral is simply the area between the line and the x-axis and so we should be able to use basic area formulas to help us determine the value of the integral.
In this case we can see the area is clearly a triangle with base 5 and height 20. However, we need to be a little careful here and recall that area that is below the x-axis is considered to be negative area and so we’ll need to keep that in mind when we do the area computation.
Here is the value of the integral,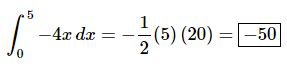
For problems 10 – 12 differentiate each of the following integrals with respect to x.
Q.10. Differentiate the following integral with respect to x.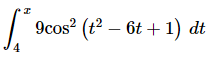
Ans: This is nothing more than a quick application of the Fundamental Theorem of Calculus, Part I.
The derivative is,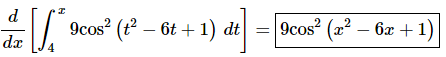
Q.11. Differentiate the following integral with respect to x.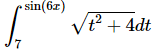
Ans: This is nothing more than a quick application of the Fundamental Theorem of Calculus, Part I.
Note however, that because the upper limit is not just xx we’ll need to use the Chain Rule, with the “inner function” as sin(6x).
The derivative is,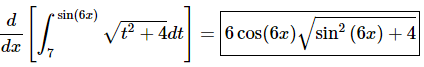
Q.12. Differentiate the following integral with respect to x.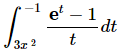
Ans: This is nothing more than a quick application of the Fundamental Theorem of Calculus, Part I.Note however, that we’ll need to interchange the limits to get the lower limit to a number and the x’s in the upper limit as required by the theorem. Also, note that because the upper limit is not just xx we’ll need to use the Chain Rule, with the “inner function” as 3x2.The derivative is,
The derivative is,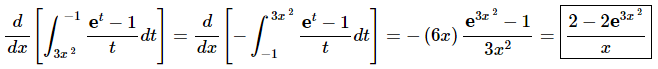
|
112 videos|65 docs|3 tests
|
FAQs on Definition of the Definite Integral - Calculus - Mathematics
| 1. What is the definition of the definite integral in mathematics? |  |
| 2. How is the definite integral denoted in mathematical notation? |  |
| 3. What does the definite integral measure or represent in mathematics? |  |
| 4. How can the definite integral be interpreted geometrically? |  |
| 5. What is the relationship between the definite integral and antiderivatives? |  |