Time Series
How do people get to know that the price of a commodity has increased over a period of time? They can do so by comparing the prices of the commodity for a set of a time period. A set of observations ordered with respect to the successive time periods is a time series.
In other words, the arrangement of data in accordance with their time of occurrence is a time series. It is the chronological arrangement of data. Here, time is just a way in which one can relate the entire phenomenon to suitable reference points. Time can be hours, days, months or years.
A time series depicts the relationship between two variables. Time is one of those variables and the second is any quantitative variable. It is not necessary that the relationship always shows increment in the change of the variable with reference to time. The relation is not always decreasing too.
It may be increasing for some and decreasing for some points in time. Can you think of any such example? The temperature of a particular city in a particular week or a month is one of those examples.
Uses of Time Series
- The most important use of studying time series is that it helps us to predict the future behaviour of the variable based on past experience
- It is helpful for business planning as it helps in comparing the actual current performance with the expected one
- From time series, we get to study the past behaviour of the phenomenon or the variable under consideration
- We can compare the changes in the values of different variables at different times or places, etc.
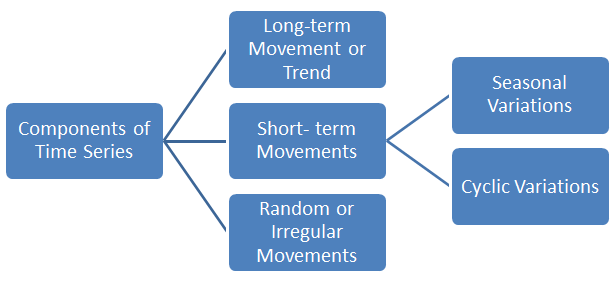
Components for Time Series Analysis
The various reasons or the forces which affect the values of an observation in a time series are the components of a time series. The four categories of the components of time series are
- Trend
- Seasonal Variations
- Cyclic Variations
- Random or Irregular movements
Seasonal and Cyclic Variations are the periodic changes or short-term fluctuations.
Trend
The trend shows the general tendency of the data to increase or decrease during a long period of time. A trend is a smooth, general, long-term, average tendency. It is not always necessary that the increase or decrease is in the same direction throughout the given period of time.
It is observable that the tendencies may increase, decrease or are stable in different sections of time. But the overall trend must be upward, downward or stable. The population, agricultural production, items manufactured, number of births and deaths, number of industry or any factory, number of schools or colleges are some of its example showing some kind of tendencies of movement.
Linear and Non-Linear Trend
If we plot the time series values on a graph in accordance with time t. The pattern of the data clustering shows the type of trend. If the set of data cluster more or less round a straight line, then the trend is linear otherwise it is non-linear (Curvilinear).
Periodic Fluctuations
There are some components in a time series which tend to repeat themselves over a certain period of time. They act in a regular spasmodic manner.
Seasonal Variations
These are the rhythmic forces which operate in a regular and periodic manner over a span of less than a year. They have the same or almost the same pattern during a period of 12 months. This variation will be present in a time series if the data are recorded hourly, daily, weekly, quarterly, or monthly.
These variations come into play either because of the natural forces or man-made conventions. The various seasons or climatic conditions play an important role in seasonal variations. Such as production of crops depends on seasons, the sale of umbrella and raincoats in the rainy season, and the sale of electric fans and A.C. shoots up in summer seasons.
The effect of man-made conventions such as some festivals, customs, habits, fashions, and some occasions like marriage is easily noticeable. They recur themselves year after year. An upswing in a season should not be taken as an indicator of better business conditions.
Cyclic Variations
The variations in a time series which operate themselves over a span of more than one year are the cyclic variations. This oscillatory movement has a period of oscillation of more than a year. One complete period is a cycle. This cyclic movement is sometimes called the ‘Business Cycle’.
It is a four-phase cycle comprising of the phases of prosperity, recession, depression, and recovery. The cyclic variation may be regular are not periodic. The upswings and the downswings in business depend upon the joint nature of the economic forces and the interaction between them.
Random or Irregular Movements
There is another factor which causes the variation in the variable under study. They are not regular variations and are purely random or irregular. These fluctuations are unforeseen, uncontrollable, unpredictable, and are erratic. These forces are earthquakes, wars, flood, famines, and any other disasters.
Mathematical Model for Time Series Analysis
Mathematically, a time series is given as
yt = f (t)
Here, yt is the value of the variable under study at time t. If the population is the variable under study at the various time period t1, t2, t3, … , tn. Then the time series is
t: t1, t2, t3, … , tn
yt: yt1, yt2, yt3, …, ytn
or, t: t1, t2, t3, … , tn
yt: y1, y2, y3, … , yn
Additive Model for Time Series Analysis
If yt is the time series value at time t. Tt, St, Ct, and Rt are the trend value, seasonal, cyclic and random fluctuations at time t respectively. According to the Additive Model, a time series can be expressed as
yt = Tt + St + Ct + Rt.
This model assumes that all four components of the time series act independently of each other.
Multiplicative Model for Time Series Analysis
The multiplicative model assumes that the various components in a time series operate proportionately to each other. According to this model
yt = Tt × St × Ct × Rt
Mixed models
Different assumptions lead to different combinations of additive and multiplicative models as
yt = Tt + St + Ct Rt.
The time series analysis can also be done using the model yt = Tt + St × Ct × Rt or yt = Tt × Ct + St × Rt etc.
Solved Example on Time Series Analysis
Question: For which type of data the seasonal fluctuations do not appear in a time series?
Solution: The seasonal variations do not appear in a series of annual data.
Question: What does the term ‘long period of time’ in a trend depict?
Solution: The term ‘long period of time’ is a relative term. It cannot be defined exactly. For some cases, a period as long as a few years may not be enough while in some cases a period as small as a week is considered a long period of time. It is totally dependent on the variable under study.