Class 10 Maths Chapter 3 Previous Year Questions - Pair of Linear Equations in Two Variables
| Table of contents |

|
| Previous Year Questions 2024 |

|
| Previous Year Questions 2023 |

|
| Previous Year Questions 2022 |

|
| Previous Year Questions 2021 |

|
| Previous Year Questions 2020 |

|
| Previous Year Questions 2019 |

|
Previous Year Questions 2024
Q1: The pair of linear equations x + 2y + 5 = 0 and – 3x = 6y – 1 has. (CBSE 2024)(a) unique solution
(b) exactly two solutions
(c) infinitely many solutions
(d) no solutions
 View Answer
View Answer 
Ans: (d)
x + 2y + 5 = 0
On comparing with
a1x + b1y + c1 = 0, we get a1 = 1, b1 = 2, c1 = 5 – 3x = 6y – 1
3x + 6y – 1 = 0
On comparing with a2x + b2y + c2 = 0, we get a2 = 3, b2 = 6, c2 = – 1
a1a2 = 13 , b1b2 = 26 = 13 ,
c1c2 = 5-1 = -5
Here, we get ( a1a2 = b1b2 ≠ c1c2 )
Q2: If 2x + y = 13 and 4x – y = 17, find the value of (x – y). (CBSE 2024)
 View Answer
View Answer 
Ans:
2x + y = 13 ...(i)
4x – y = 17 ...(ii)
On adding eqn.(i) and eqn.(ii)
6x = 30
x = 5
Put the value of x in eqn.(i)
2 × 5 + y = 13
⇒10 + y = 13
∴ y = 3
So, x – y = 5 – 3 = 2
Q3: The value of k for which the pair of linear equations 5x + 2y − 7 = 0 and 2x + ky + 1 = 0 do not have a solution is ______. (CBSE 2024)
(a) 5
(b) 4/5
(c) 5/4
(d) 5/2
 View Answer
View Answer 
Ans: (b) 4/5
Given that,
Pair of linear equations as 5x + 2y − 7 = 0 and 2x + ky + 1 = 0
For no solution,
→ 52 = 2k ≠ -71
→ 52 = 2k
→ k5 = 45
Q4: Solve the following pair of linear equations for x and y algebraically:
x + 2y = 9 and y − 2x = 2 (CBSE 2024)
 View Answer
View Answer 
Ans: Given the pair of linear equations as,
⇒ x + 2y = 9 ...(i)
⇒ y − 2x = 2
⇒ −2x + y = 2 ...(ii)
Multiplying eqn (i) by 2 and adding to eqn (ii), we get
⇒ (−2x + y) + ( 2x + 4y) = 2 + 18
⇒ 5y = 20
⇒ y = 4
Putting in eqn (i),
⇒ x + 2(4) = 9
⇒ x = 9 − 8
⇒ x = 1
So, the required solution is x = 1 and y = 4
Q5: Check whether the point (−4, 3) lies on both the lines represented by the linear equations x + y + 1 = 0 and x − y = 1. (CBSE 2024)
 View Answer
View Answer 
Ans: Given the equations of line are
⇒ x + y = −1 ...(i)
⇒ x − y = 1 ...(ii)
The intersection point of both the lines will be the point that lies on both the lines,
So, adding eqn (i) and (ii),
⇒ (x + y) + (x − y) = −1 + 1
⇒ 2x = 0
⇒ x = 0
Putting in eqn (i),
⇒ 0 + y = −1
⇒ y = −1
So, the point will be (x, y) = (0, −1)
Hence, the point (−4, 3) does not lie on both the lines.
Q6: In the given figure, graphs of two linear equations are shown. The pair of these linear equations is: (CBSE 2024)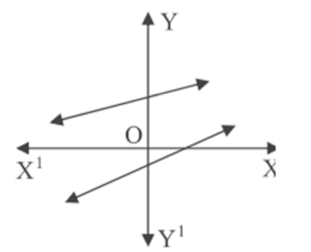
(a) consistent with unique solutions.
(b) consistent with infinitely many solutions.
(c) inconsistent.
(d) inconsistent but can be made consistent by extending these lines.
 View Answer
View Answer 
Ans: (a) consistent with unique solution.
Q7: Solve the following system of linear equations 7x - 2y= 5 and 8x + 7y = 15 and verify your answer. (CBSE 2024)
 View Answer
View Answer 
Ans: Given system of linear equations are
7x − 2y = 5 ...(i)
8x + 7y = 15 ...(ii)
By multiplying eq. (i) by 7 and eq. (ii) by 2, we get
49x − 14y = 35
16x + 14y = 30
65x = 65
∴ x = 1
Substituting the value of x is eq (i), we get
7(1) − 2y = 5
or, 7 − 2y = 5
or −2y = −2, or y = 1
Therefore, x = 1 and y = 1
Q8: Three years ago, Rashmi was thrice as old as Nazma. Ten years later, Rashmi will be twice as old as Nazma. How old are Rashmi and Nazma now? (CBSE 2024)
 View Answer
View Answer 
Ans: Let the age of Rashmi = x years
and the age of Nazma = y years
Three years ago,
Rashmi's age = (x − 3) years
Nazma's age = (y − 3) years
According to the question,
(x − 3) = 3(y − 3)
⇒ x − 3 = 3y − 9
⇒ x = 3y − 6 ...(i)
Ten years later,
Rashmi's age = x + 10
Nazma's age= y + 10
According to the question,
(x + 10) = 2(y + 10)
x + 10 = 2y + 20
x = 2y + 10 ...(ii)
From eq. (i) and (ii), we get
3y − 6 = 2y + 10
y = 16
Substituting the value of y in eq. (i), we get
x = 3 × 16 − 6 = 48 − 6 = 42
Thus, Rashmi is 42 years old, and Nazma is 16 years old.
Previous Year Questions 2023
Q9: The pair of linear equations 2x = 5y + 6 and 15y = 6x - 18 represents two lines which are (2023)(a) intersecting
(b) parallel
(c) coincident
(d) either intersecting or parallel
 View Answer
View Answer 
Ans: (c)
Sol: The given pair of linear equations is 2x = 5y+ 6 and 15y = 6x - 18
i.e ., 2x - 5y - 6 = 0 and 6x- 15y- 18 = 0
As, 2/6 = -5/-15 = -6/-18
i.e.. 1 /3 = 1 / 3 = 1/3
Since, a1/a2=b1/b2=c1/c2
Therefore, the lines are coincident.
Q10: If the pair of linear equations x - y = 1, x + ky = 5 has a unique solution x = 2, y = 1. then the value of k (2023)
(a) -2
(b) -3
(c) 3
(d) 4
 View Answer
View Answer 
Ans: (c)
Sol: x + ky = 5
At x = 2, y = 1
2 + k(1) = 5
∴ k = 3
Q11: The pair of linear equations x + 2y + 5 = 0 and -3x - 6y + 1 = 0 has (2023)
(a) A unique solution
(b) Exactly two solutions
(c) Infinitely many solutions
(d) No solution
 View Answer
View Answer 
Ans: (d)
x + 2y + 5 = 0
On comparing with
a1x + b1y + c1 = 0, In x + 2y + 5 = 0, we get a1 = 1, b1 = 2, c1 = 5
Rearranging – 3x = 6y – 1 , We get 3x + 6y – 1 = 0
On comparing with a2x + b2y + c2 = 0, we get a2 = 3, b2 = 6, c2 = – 1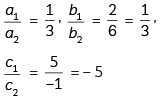
Q12: Solve the pair of equations x = 5 and y = 7 graphically. (2023)
 View Answer
View Answer 
Ans: Given equations are
x = 5 ---------------(i)
y = 7 ---------------(ii)
Draw the line x = 5 parallel to the y-axis and y= 7 parallel to the x-axis.
∴ The graph of equation (i) and (ii) is as follows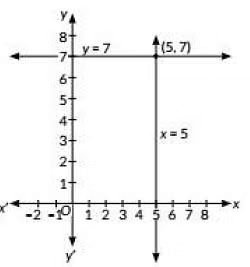
The lines x = 5 and y = 7 intersect each other at (5, 7).
Q13: Using the graphical method, find whether a pair of equations x = 0 and y = -3 is consistent or not. (2023)
 View Answer
View Answer 
Ans: Given pair of equations are
x = 0 ------(i)
and y = -3 ------(ii)
x = 0 means y-axis and draw a line y = -3 parallel to x-axis. The graph of given equations (i) and (ii) is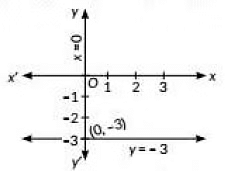
The lines intersect each other at (0, -3). Therefore, the given pair of equations Is consistent.
Q14: Half of the difference between two numbers is 2. The sum of the greater number and twice the smaller number is 13. Find the numbers. (2023)
 View Answer
View Answer 
Ans: Let x and y be two numbers such that x> y
According to the question,
and x + 2y = 13 ---- (ii)
Subtracting (i) from (ii), we get
3y = 9
⇒ y = 3
Substitute y = 3 in (i) we get
x - 3 = 4
⇒ x = 7
Q15: (A) If we add 1 to the numerator and subtract 1 from the denominator, a fraction reduces to 1 It becomes 1/2 if we only add 1 to the denominator. What is the fraction?
OR
(B) For which value of 'k' will the following pair of linear equations have no solution? (2023)
3x + y = 1
(2k - 1)x + (k - 1)y = 2k + 1
 View Answer
View Answer 
Ans: (A) Let required fraction be x/y
According to question,
x + 1y - 1 = 1
⇒ x + 1 = y - 1
⇒ x = y - 2 ... (i)
Also, xy + 1 = 12
⇒ 2x = y + 1 ...(ii)
From equations (i) and (ii), we get
2y — 4 = y + 1
y = 5
∴ x = 3
Required fraction x/y is 3/5
OR
(B) 3x + y = 1
(2k - 1 )x + (k - 1 )y = 2k + 1
For no solution;
⇒ 32k - 1 = 1k - 1 ≠ 12k + 1
2k - 1 = 3k - 3
⇒ k = 2
Also, 1k - 1 ≠ 12k + 1
2k + 1 ≠ k - 1
⇒ k ≠ -2
Q16: Two schools 'P' and 'Q' decided to award prizes to their students for two games of Hockey Rs. x per student and Cricket Rs. y per student. School 'P' decided to award a total of Rs. 9,500 for the two games to 5 and 4 students respectively, while school 'Q' decided to award Rs. 7,370 for the two games to 4 and 3 students respectively.

Based on the given information, answer the following questions.
(i) Represent the following information algebraically (in terms of x and y).
(ii) (a) What is the prize amount for hockey?
(b) Prize amount on which game is more and by how much?
(iii) What will be the total prize amount if there are 2 students each from two games? (CBSE 2023)
 View Answer
View Answer 
Ans: (i) For Hockey, the amount given to per student = x
For cricket, the amount given to per student = y
From the question,
5x + 4y =9500 (i)
4x + 3y = 7370 (ii)
(ii) (a) Multiply (1) by 3 and (2) by 4 and then subtracting, we get
15x + 12y- (16x + 12y) = 28500 - 29480
⇒ - x = - 980
⇒ x = ₹980
The prize amount given for hockey is Rs. 980 per student
(b) Multiply (1) by 4 and (2) by 5 and then subtracting, we get
20x + 16y- 20x - 15y = 38000 - 36850
⇒ y = 1150
The prize amount given for cricket is more than hockey by (1150 - 980) = 170.
(iii) Total prize amount = 2 x 980 + 2 x 1150
= Rs. (1960 + 2300) = Rs. 4260
Previous Year Questions 2022
Q17: The pair of lines represented by the linear equations 3x + 2y = 7 and 4x + 8y -11 = 0 are (2022)(a) perpendicular
(b) parallel
(c) intersecting
(d) coincident
 View Answer
View Answer 
Ans: (c)
Sol:
- Calculate a1 / a2 = 3 / 4
- Calculate b1 / b2 = 2 / 8 = 1 / 4
- Calculate c1 / c2 = -7 / 11
Comparing the ratios:
a1 / a2 = 3 / 4
b1 / b2 = 1 / 4
c1 / c2 = 7 / 11
Since a1 / a2 ≠ b1 / b2, the lines are not parallel. Also, since none of the ratios are equal, the lines are not coincident.
Clearly, from the graph, we can see that both lines intersect each other.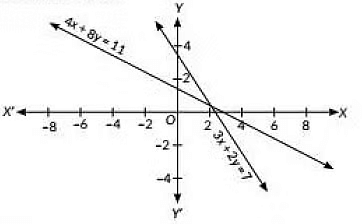
Q18: The pair of equations y = 2 and y = - 3 has (2022)
(a) one solution
(b) two solutions
(c) infinitely many solutions
(d) no solution
 View Answer
View Answer 
Ans: (d)
Sol: Given equations are, y = 2 and y = - 3.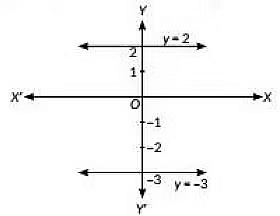
Clearly, from the graph, we can see that both equations are parallel to each other.
So, there will be no solution.
Q19: A father is three times as old as his son. In 12 years time, he will be twice as old as his son. The sum of the present ages of the father and the son is (2022)
(a) 36 years
(b) 48 years
(c) 60 years
(d) 42 years
 View Answer
View Answer 
Ans: (b)
Sol: Let age of father be 'x' years and age of son be 'y' years.
According to the question, x = 3y ..(i)
and x + 12 = 2 (y + 12)
⇒ x - 2y = 12 ..(ii)
From (i) and (ii), we get x = 36, y = 12
∴ x + y = 48 years
Q20: If 17x - 19y = 53 and 19x - 17y = 55, then the value of (x + y) is (2022)
(a) 1
(b) -1
(c) 3
(d) -3
 View Answer
View Answer 
Ans: (a)
Sol: Given,17x - 19y = 53 ...(i)
and 19x - 17y = 55 _(ii)
Multiplying (i) by 19 and (ii) by 17, and by subtracting we get,
323x - 361y -(323x - 289y) = 1007 - 935
⇒ - 72y = 72
⇒ y = - 1
Putting y = - 1 in (i), we get,
17x - 19 (-1) = 53
⇒ 17x = 53 - 19
⇒ 17x = 34
x = 2
∴ x + y = 2 - 1
= 1
Previous Year Questions 2021
Q21: The value of k. for which the pair of linear equations x + y - 4 = 0, 2x + ky - 3 = 0 have no solution, is (2021)(a) 0
(b) 2
(c) 6
(d) 8
 View Answer
View Answer 
Ans: (b)
Given equations:
x + y − 4 = 0
2x + ky − 3 = 0
The general form of a linear equation is ax + by + c = 0. So, comparing terms:
For the first equation, a1 = 1, b1 = 1, c1 = −4.For the second equation, a2 = 2, b2 = k, c2= −3.
For the lines to be parallel (and hence have no solution), we need:
a1a2 = b1b2 ≠ c1c2
So, 1/2 = 1/k
Cross-multiplying gives:
k = 2
Now, let’s check the condition for the
c1c2 = -4-3 = 43
Since 1/2 = 1/k when k = 2 but 1/2 ≠ 4/3, the condition for no solution is satisfied.
Thus, the value of k for which the equations have no solution is: 2
So, the correct answer is (b) 2.
Q22: The solution of the pair of linear equations x = -5 and y = 6 is (2021)
(a) (-5, 6)
(b) (-5, 0)
(c) (0, 6)
(d) (0, 0)
 View Answer
View Answer 
Ans: (a)
Sol: (-5, 6) is the solution of x = -5 and y = 6.
Q23: The value of k for which the pair of linear equations 3x + 5y = 8 and kx + 15y = 24 has infinitely many solutions, is (2021)
(a) 3
(b) 9
(c) 5
(d) 15
 View Answer
View Answer 
Ans: (b)
Sol: For. infinitely many solutions
a1a2 = b1b2 = c1c2
⇒ 3k = 515 = 824
k = 9
Q24: The values of x and y satisfying the two equations 32x + 33y = 34, 33x + 32y = 31 respectively are: (2021)
(a) -1, 2
(b) -1, 4
(c) 1, -2
(d) -1, -4
 View Answer
View Answer 
Ans: (a)
Sol: 32x + 33y = 34 ...(i)
33x + 32y = 31 ...(ii)
Adding equation (i) and (ii) and subtracting equation (ii) from (i),
we get 65x + 65y = 65 or x + y = 1 ...(iii)
and - x + y = 3 ...(iv)
Adding equation (iii) and (iv),
we get y = 2
Substituting the value of y in equation (iii),
x = -1
Q25: Two lines are given to be parallel. The equation of one of the lines is 3x - 2y = 5. The equation of the second line can be (2021)
(a) 9x + 8 y = 7
(b) - 12 x - 8 y = 7
(c) - 12 x + 8y = 7
(d) 12x + 8y = 7
 View Answer
View Answer 
Ans: (c)
Sol: If two lines a1x + b1y + c1 = 0 and a2x + b2y + c2 = 0 are parallel, then
a1a2 = b1b2 ≠ c1c2
It can only possible between 3x - 2y = 5 and -12x + 8y = 7.
Q26: The sum of the numerator and the denominator of a fraction is 18. If the denominator is increased by 2, the fraction reduces to 1/3. Find the fraction. (2021)
 View Answer
View Answer 
Ans: 5/13
Let the numerator be x and the denominator be y of the fractions. Then, the fraction = x /y.
Given , x + y = 18 - (i)
and 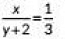
⇒ 3x - y = 2 . . (ii)
Adding (i) and (ii), we get
4x = 20 ⇒ x = 5
Put the value of x in (i), we get
5+ y= 18
⇒ y = 13
∴ The required fraction is 5/13
Q27: Find the value of K for which the system of equations x + 2y = 5 and 3x + ky + 15 = 0 has no solution. (2021)
 View Answer
View Answer 
Ans: Given, the system of equations
x + 2y = 5
3k + ky = - 15 has no solution.
∴ 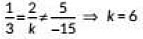
For K = 6 the given system of equations has no solution.
Q28: Case study-based questions are compulsory.
A bookstore shopkeeper gives books on rent for reading. He has a variety of books in his store related to fiction, stories, quizzes etc. He takes a fixed charge for the first two days and an additional charge for subsequent days Amruta paid ₹22 for a book and kept it for 6 days: while Radhika paid ₹16 for keeping the book for 4 days.
Assume that the fixed charge is ₹x and the additional charge (per day) is ₹y.
Based on the above information, answer any four of the following questions.
(i) The situation of the amount paid by Radhika. is algebraically represented by (2021)
(a) x - 4 y = 16
(b) x + 4 y = 16
(c) x - 2 y = 16
(d) x + 2 y = 16
 View Answer
View Answer 
Ans: (d)
Sol: For Amruta, x + (6 - 2)y = 22
i. e., x + 4y = 22 ...(i)
For Radhika, x + (4 - 2)y = 16 i.e.,x + 2y = 16 ...(ii)
Solving equation (i) and (ii). we get
x = 10 and y = 3
i.e., Fixed charges (x) = 10 ...(iii)
and additional charges per subsequent day
(y) = ₹ 3 ...(iv)
x + 2 y = 16 [From equation (ii)]
(ii) The situation of the amount paid by Amruta. is algebraically represented by (2021)
(a) x - 2y = 11
(b) x - 2y = 22
(c) x + 4 y = 22
(d) x - 4 y = 11
 View Answer
View Answer 
Ans: (c)
Sol: For Amruta, x + (6 - 2)y = 22
i. e., x + 4y = 22 ...(i)
For Radhika, x + (4 - 2)y = 16 i.e.,x + 2y = 16 ...(ii)
Solving equation (i) and (ii). we get
x = 10 and y = 3
i.e., Fixed charges (x) = 10 ...(iii)
and additional charges per subsequent day
(y) = ₹ 3 ...(iv)
x + 4 y = 22 [From equation (i)]
(iii) What are the fixed charges for a book? (2021)
(a) ₹ 9
(b) ₹ 10
(c) ₹ 13
(d) ₹ 15
 View Answer
View Answer 
Ans: (b)
Sol: For Amruta, x + (6 - 2)y = 22
i. e., x + 4y = 22 ...(i)
For Radhika, x + (4 - 2)y = 16 i.e.,x + 2y = 16 ...(ii)
Solving equation (i) and (ii). we get
x = 10 and y = 3
i.e., Fixed charges (x) = 10 ...(iii)
and additional charges per subsequent day
y = ₹ 3 ...(iv)
x = ₹ 10 [From equation (iii)]
(iv) What are the additional charges for each subsequent day for a book? (2021)
(a) ₹ 6
(b) ₹ 5
(c) ₹ 4
(d) ₹ 3
 View Answer
View Answer 
Ans: (d)
Sol: For Amruta, x + (6 - 2)y = 22
i. e., x + 4y = 22 ...(i)
For Radhika, x + (4 - 2)y = 16 i.e.,x + 2y = 16 ...(ii)
Solving equation (i) and (ii). we get
x = 10 and y = 3
i.e., Fixed charges (x) = 10 ...(iii)
and additional charges per subsequent day
y = ₹ 3 ...(iv)
y = ₹ 3 [From equation (iv)]
(v) What is the total amount paid by both, if both of them have kept the book for 2 more days? (2021)
(a) ₹ 35
(b) ₹ 52
(c) ₹ 50
(d) ₹ 58
 View Answer
View Answer 
Ans: (c)
For Amruta, x + (6 - 2)y = 22
i. e., x + 4y = 22 ...(i)
For Radhika, x + (4 - 2)y = 16 i.e.,x + 2y = 16 ...(ii)
Solving equation (i) and (ii). we get
x = 10 and y = 3
i.e., Fixed charges (x) = 10 ...(iii)
and additional charges per subsequent day
y = ₹ 3 ...(iv)
Total amount paid for 2 more days by both
= (x + 4 y) + 2 y + (x + 2y ) + 2 y
= 2 x + 10y
= 2 x 10 + 10 x 3
= ₹ 50
Previous Year Questions 2020
Q29: The pair of equations x = a and y = b graphically represent lines which are (2020)
(a) Intersecting at (a, b)
(b) Intersecting at (b, a)
(c) Coincident
(d) Parallel
 View Answer
View Answer 
Ans: (a)
Sol: The pair of equations x = a and y = b graphically represent lines which are parallel to the y-axis and x-axis respectively.
The lines will intersect each other at (a, b).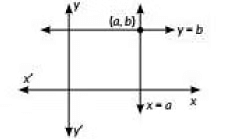
Q30: If the equations kx - 2y = 3 and 3x + y = 5 represent two intersecting lines at unique points, then the value of k is _________. (2020)
 View Answer
View Answer 
Ans: For any real number except k = -6
kx - 2y = 3 and 3x + y = 5 represent lines intersecting at a unique point.
⇒ k3 ≠ -21
⇒ k ≠ -6
For any real number except k ≠ -6
The given equation represent two intersecting lines at unique point.
Q31: The value of k for which the system of equations x + y - 4 = 0 and 2x + ky = 3 has no solution. is (2020)
(a) -2
(b) ≠2
(c) 3
(d) 2
 View Answer
View Answer 
Ans: (d)
Sol: For no solution; a1a2 = b1b2 ≠ c1c2
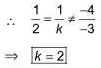
Q32: Determine graphically the coordinates of the vertices of a triangle, the equations of whose sides are given by 2y - x = 8, 5y - x = 14, and y - 2x = 1. (2020)
 View Answer
View Answer 
Ans: Solutions of linear equations
2y - x = 8 ..(i)
5y - x = 14 ...(ii)
and y - 2x = 1 ...(iii)
are given below: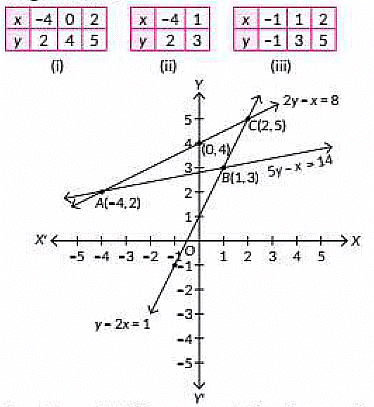
From the graph of lines represented by given equations, we observe that
Lines (i) and (iii) intersect each other at C(2, 5),
Lines (ii) and {iii) intersect each other at B(1, 3) and Lines (i) and (ii) intersect each other at 4(-4, 2).
Coordinates of the vertices of the triangle are A(-4, 2), B(1, 3) and C(2, 5).
Q33: Solve the equations x + 2y = 6 and 2x - 5y = 12 graphically. (2020)
 View Answer
View Answer 
Ans: Solution of linear equations
x + 2y = 6 and 2x - 5y = 12
are given below
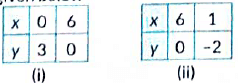
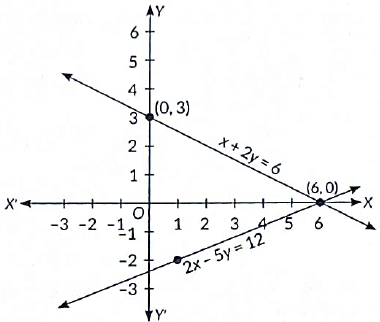
From the graph, the two lines intersect each other at point (6, 0)
∴ x = 6 and y = 0
Q34: A fraction becomes 1/3 when 1 is subtracted from the numerator, and it becomes 1/4 when 8 is added to its denominator. Find the fraction. (CBSE 2020)
 View Answer
View Answer 
Ans: Let the required fraction be x/y.
According to question, we have
x - 1y = 13 ... (i)
and
xy + 8 = 14 ... (ii)
From (i), 3x - 3 = y
⇒ 3x - y - 3 = 0 ... (iii)
From (ii), 4x = y +8
so, 4x - y - 8 = 0 ... (iv)
Subtracting (iii) from (iv),
we get x = 5
Substituting the value of x in (iii),
we get y = 12
Thus, the required fraction is 5/12
Q35: The present age of a father is three years more than three times the age of his son. Three years hence, the father's age will be 10 years more than twice the age of the son. Determine their present ages. (2020)
 View Answer
View Answer 
Ans: Let the present age of son be x years and that of father be y years.
According to question, we have
y = 3x+ 3 ⇒ 3x - y + 3 = 0 (i)
And y + 3 = 2(x + 3) + 10
⇒ y + 3 = 2x + 6 +10
⇒ 2x - y + 13 = 0 (ii)
Subtracting (ii) from (i), we get x = 10
Substituting the value of x in (ii). we get y = 33
So. the present age of the son is 10 years and that of the father is 33 years.
Q36: Solve graphically : 2x + 3y = 2, x - 2y = 8 (2020)
 View Answer
View Answer 
Ans: Given lines are 2x + 3y = 2 and x - 2y = 8
2x + 3y = 2
and x - 2y = 8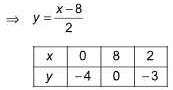
∴ We will plot the points (1, 0), (-2, 2) and (4, - 2 ) and join them to get the graph of 2x + 3y = 2 and we will plot the points (0, -4), (8, 0) and (2, -3) and join them to get the graph of x - 2y = 8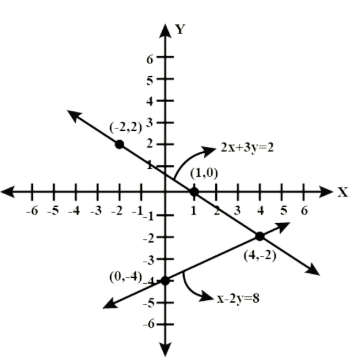
Q37: A train covered a certain distance at a uniform speed. If the train had been 6 km/hr faster, it would have taken 4 hours less than the scheduled time and if the train were slower by 6 km/hr, it would have taken 6 hours more than the scheduled time. Find the length of the journey. (CBSE 2020)
 View Answer
View Answer 
Ans: Let the original uniform speed of the train be x km/hr and the total length of journey be l km. Then, scheduled time taken by the train to cover a distance of l km = l/x hours
Now,
lx + 6 = lx - 4
⇒ lx - lx + 6 = 4
⇒ x + 6 - xx(x + 6) = 4
⇒ 6lx(x + 6) = 4
⇒ l = 2x(x + 6)3 ... (i)
Also,
lx - 6 = lx + 6
⇒ lx - 6 - lx = 6
⇒ x - x + 6(x - 6)x = 6
⇒ 6l(x - 6)x = 6
⇒ l = x(x - 6) ... (ii)
From equations (i) and (ii), we have
2x(x + 6)3 = x(x - 6)
⇒ 2x + 12 = 3x - 18
⇒ x = 30
Putting the value of x in eq. (ii), we get
l = 30(30 – 6)
= 30 × 24
= 720
Hence, the length of the journey is 720 km.
Previous Year Questions 2019
Q38: Draw the graph of the equations x - y + 1 = 0 and 3x + 2y - 12 = 0. Using this graph, find the values of x and y which satisfy both the equations. (2019) View Answer
View Answer 
Ans: Solutions of linear equation
x - y + 1 = 0 ...(i)
and 3x + 2y - 12 = 0 ...(ii)
are given below:
From the graph, the two lines intersect each other at the point (2, 3)
∴ x = 2, y = 3.
Q39: The larger of two supplementary angles exceeds the smaller by 18°. Find the angles. (2019)
 View Answer
View Answer 
Ans: Let the larger angle be x° and the smaller angle be y°. We know that the sum of two supplementary pairs of angles is always 180°.
We have x° + y° = 180° (i)
and x° - y° = 18° (ii) [Given]
By (1), we have x° = 180° - y° _(iii)
Put the value of x° in (ii), we get
180° - y° - y° = 18°
⇒ 162° = 2y°
⇒ y = 81
From (3), we have x° = 180° - 81° = 99°
The angles are 99° and 81°
Q40: Solve the following pair of linear equations: 3x - 5y =4, 2y+ 7 = 9x. (2019)
 View Answer
View Answer 
Ans: Given, pair of linear equations:
3x - 5y = 4, ....... (i)
2y+ 7 = 9x
9x - 2y = 7 ........ (ii)
Multiply (i) by 3 and subtract from (ii), as
⇒ 9x - 2y - 9x + 15y = -5 → 13y = -5
⇒ y = -513
Put y = -513 in (i), we get
3x - 5 -513 = 4
⇒ 3x + 2513 = 4
⇒ 3x = 4 - 2513
⇒ x = 2713 × 3 = 913
Hence, x = 9/13 and y = -5/13
Q41: A father's age is three times the sum of the ages of his two children. After 5 years his age will be two times the sum of their ages. Find the present age of the father. (2019)
 View Answer
View Answer 
Ans: Let the ages of two children be x and y respectively.
Father's present age = 3(x +y)
After 5 years, sum of ages of children = x + 5 + y + 5
= x + y + 10
and age of father = 3(x + y) + 5
According to the question,
3(x + y) + 5 = 2(x + y+ 10)
3x + 3y + 5 = 2x + 2y + 20
⇒ x + y = 15
Hence, present age of father = 3(x + y)
= 3 x 15 = 45 years
Q42: A fraction becomes 1/3 when 2 is subtracted from the numerator and will becomes 1/2 when 1 is subtracted from the denominator. Find the fraction. (2019)
 View Answer
View Answer 
Ans: Let the fraction be x/y
Then, according to question.
x - 2y = 13 and xy - 1 = 12
⇒ 3x - 6 = y and 2x = y - 1
⇒ 3x - y - 6 = 0 ... (i) and 2x - y + 1 = 0 ... (ii)
Subtracting (ii) from (i), we get x - 7 = 0
So, x = 7
From (i) ,3(7) - y - 6 = 0
⇒ 21 - 6 = y
⇒ y = 15
Therefore required fraction is 7/15
Q43: Find the value(s) of k so that the pair of equations x + 2y = 5 and 3x + ky + 15 = 0 has a unique solution. (2019)
 View Answer
View Answer 
Ans: The given pair of linear equations is
x + 2y = 5
3x + ky= -15
Since the system of equations has a unique solution
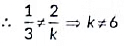
∴ For all values of k except k = 6, the given pair of linear equations will have a unique solution.
Q44: Find the relation between p and q if x = 3 and y = 1 is the solution of the pair of equations x - 4y + p = 0 and 2x + y - q -2 = 0. (2019)
 View Answer
View Answer 
Ans: Given pair of equations are
x - 4y + p = 0 (i)
and 2x + y - q - 2 = 0 (ii)
It is given that x = 3 and y = 1 is the solution of (i) and (ii)
∴ 3 - 4 x 1+ p = 0
⇒ p = 1
and 2 x 3 + 1 - q - 2 = 0
⇒ q = 5
∴ q = 5p
Q45: For what value of k, does the system of linear equations 2x + 3y=7 and (k – 1)x + (k + 2) y = 3k have an infinite number of solutions? (CBSE 2019)
 View Answer
View Answer 
Ans: The given system of linear equations are:
2x + 3y = 7
(k – 1)x + (k + 2)y = 3k
For infinitely many solutions:
a1a2 = b1b2 = c1c2
Here,
a1 = 2, b1 = 3, c1 = -7
a2 = (k - 1), b2 = (k + 2), c2 = -3k
⇒ 2k - 1 = 3k + 2 = -7-3k
⇒ 2(k + 2) = 3(k - 1); 3(3k) = 7(k + 2)
⇒ 2k – 3k = –3 – 4; 9k – 7k = 14
⇒ –k = –7; 2k = 14
⇒ k = 7; k = 7 Hence, the value of k is 7
|
127 videos|584 docs|79 tests
|
FAQs on Class 10 Maths Chapter 3 Previous Year Questions - Pair of Linear Equations in Two Variables
| 1. What are the key concepts covered in the Pair of Linear Equations in Two Variables chapter? |  |
| 2. How can I prepare effectively for the Pair of Linear Equations in Two Variables section in the CBSE exam? |  |
| 3. What types of questions are typically asked in the CBSE exams regarding Pair of Linear Equations in Two Variables? |  |
| 4. Are there any important formulas or theorems related to Pair of Linear Equations in Two Variables that I should remember? |  |
| 5. How do I analyze the solutions of a system of linear equations graphically? |  |
















