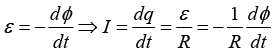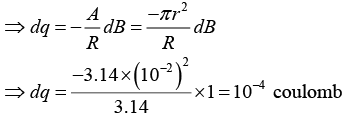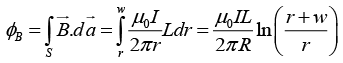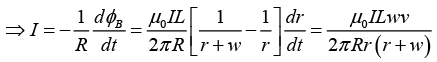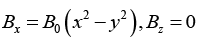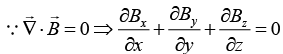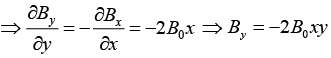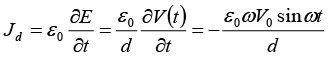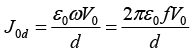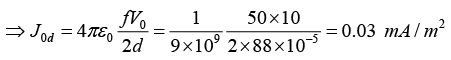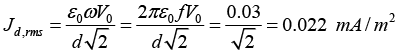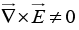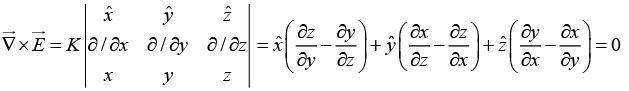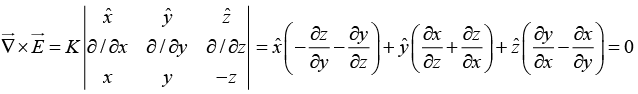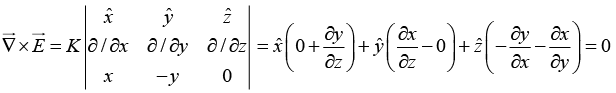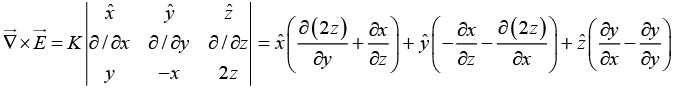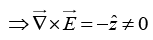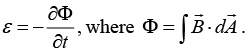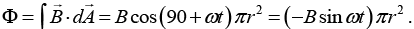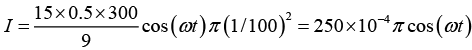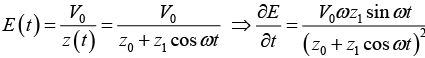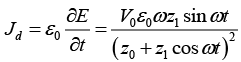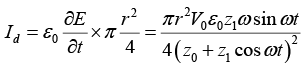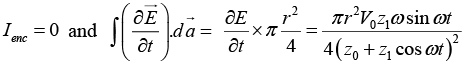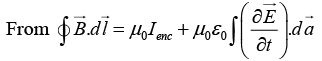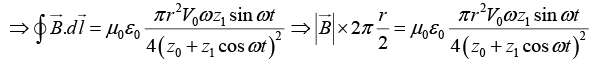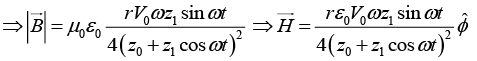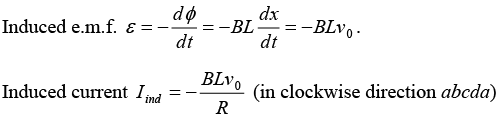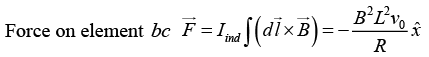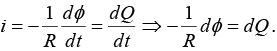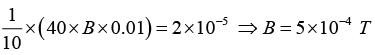Maxwell's Equations: Assignment | Electricity & Magnetism - Physics PDF Download
Q.1. A uniform magnetic field in the positive z -direction passes through a circular wire loop of radius 1 cm and resistance 3.14Ω lying in the xy-plane. The field strength is reduced from 10 tesla to 9 tesla in 1s . Find the charge transferred across any point in the wire.
Q.2. The circuit shown below is in a uniform magnetic field that is into the page and is decreasing in magnitude at the rate of 150 Tesla/sec. Then find the ammeter reading.
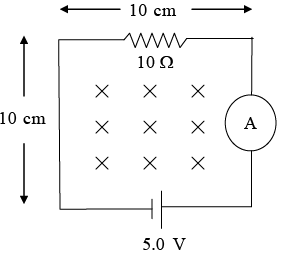
From Ohm’s Law V - ε = IR , one can obtain the current. (Note that V = 5.0 V is the voltage of the battery. The voltage induced acts to oppose this emf from the battery).
The problem gives dB/dt = 150T/s. The area is just 0.01 m2.
Thus, the induced emf is ε = dB/dt A = 150 x 0.01 = 1.5
Thus, V - e = 3.5 = IR ⇒ I = 0.35 A, since R = 10Ω.
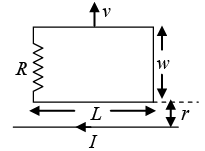
Q.3. A rectangular loop of dimension L and width w moves with a constant velocity v away from an infinitely long straight wire carrying a current I in the plane of the loop as shown in the figure below. Let R be the resistance of the loop. Show that the current in the loop at the instant the near side is at a distance r from the wire is 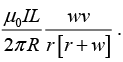
Q.4. The x and z -components of a static magnetic field in a region are Bx = B0 (x2- y2) and Bz = 0, respectively. Find one of the possible solution for its y -component which is consistent with the Maxwell equations?
Q.5. A parallel plate air-gap capacitor is made up of two plates of area 10cm2 each kept at a distance of 0.88 mm. A sine wave of amplitude 10V and frequency 50Hz is applied across the capacitor as shown in the figure.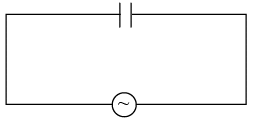 (a) Find the amplitude of the displacement current density between the plates.
(a) Find the amplitude of the displacement current density between the plates.
(b) Find the r.m.s value of the displacement current density between the plates.
(c) Find the average value of the displacement current density (in mA/m2) between the plates.
Displacement current density
(a) Amplitude of the displacement current density
(b) The r.m.s value of the displacement current density is
(c) The average value of the displacement current density is zero.
Q.6. Which of the following expressions represent an electric field due to a time varying magnetic field?
(a) 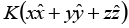
(b) 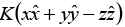
(c) 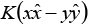
(d) 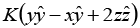
For time varying fields
(a)
(b)
(c)
(d)
Q.7. A coil of 15 turns, each of radius 1 centimeter, is rotating at a constant angular velocity ω=300 radians per second in a uniform magnetic field of 0.5 tesla, as shown in the figure. Assume at time t = 0 that the normal 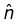 to the coil plane is along the y -direction and that the self-inductance of the coil can be neglected. If the coil resistance is 9 ohms, what will be the magnitude of the induced current in milliamperes?
to the coil plane is along the y -direction and that the self-inductance of the coil can be neglected. If the coil resistance is 9 ohms, what will be the magnitude of the induced current in milliamperes?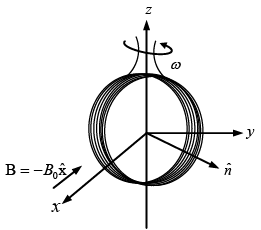
The voltage induced is equal to the change in magnetic flux
Noting the initial condition (ϕ(t = 0) = 0) , since the field and area normal are perpendicular). One finds that
Thus,
Now, to find the current, one uses Ohm’s Law in Faraday’s Law to getwhere N is the number of turns.
Thus,
Ampere
I = 25π cos (ωt) mA
Q.8. Consider a capacitor placed in free space, consisting of two concentric circular parallel plates of radii r. The separation z between the plates oscillates with a constant frequency ω, i.e. z(t )= z0 +z1 cosωt. Here z0 and z1 (< z0) are constants. The separation z(t )(<< r ) is varied in such a way that the voltage V0 across the capacitor remains constant.
(a) Calculate the displacement current density and the displacement current between the plates through a concentric circle of radius r/2.
(b) Calculate the magnetic field vector 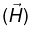 between the plates at a distance r/2
between the plates at a distance r/2
from the axis of the capacitor.
(a) If the capacitor plates are very close together, then the electric field between them is:
Displacement current density
Displacement current
(b) Consider an amperian loop of radius r /2,
where,
Q.9. A square loop of side L and mass M is made of a wire of cross-sectional area A and resistance R. The loop, moving with a constant velocity 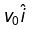 in the horizontal xy-plane, enters a region 0 ≤ x ≤ 2L having constant magnetic field
in the horizontal xy-plane, enters a region 0 ≤ x ≤ 2L having constant magnetic field 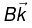 .
.
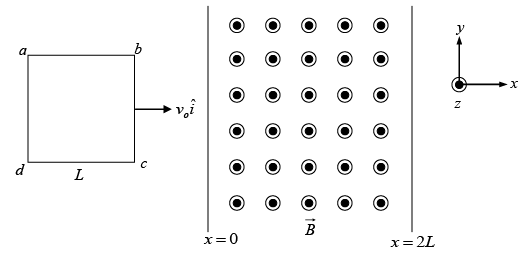
Find an expression for the x-component of the force  acting on the loop in terms of its velocity
acting on the loop in terms of its velocity  B,L and R.
B,L and R.
Initial flux ϕ0= BLx
Flux after time dt, ϕ = BL(x + dx)
Change in flux dϕ = ϕ - ϕ0 = BLdx.
Q.10. A small loop of wire of area A = 0.01m2, N = 40 turns and resistance R = 10Ω is initially kept in a uniform magnetic field B in such a way that the field is normal to the loop. When it is pulled out of the magnetic field, a total charge of Q = 2x10-5C flows through the coil. Find the magnitude of the field B.
Magnetic flux through the loop ϕ = NBA
Induced e.m.f ε = - dϕ/dt and induced current
Thus
|
82 videos|32 docs|22 tests
|
FAQs on Maxwell's Equations: Assignment - Electricity & Magnetism - Physics
| 1. What are Maxwell's equations? |  |
| 2. What is the importance of Maxwell's equations? |  |
| 3. How do Maxwell's equations relate to the IIT JAM exam? |  |
| 4. Can you briefly explain each of Maxwell's equations? |  |
| 5. How can one apply Maxwell's equations to solve practical problems? |  |