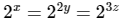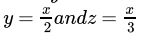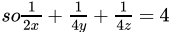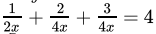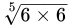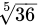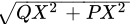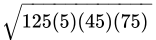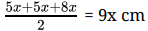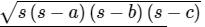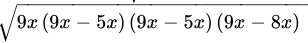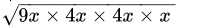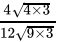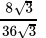Class 9 Math: Sample Question Paper Term I- 7 (With Solutions) | Mathematics (Maths) Class 9 PDF Download
| Table of contents |

|
| Class 09 Mathematics |

|
| Time: 90 Minutes |

|
| Max. Marks: 40 |

|
| Section - A |

|
| Section - B |

|
| Section - C |

|
Class 09 Mathematics
Time: 90 Minutes
Max. Marks: 40
General Instructions :
- The question paper contains three parts A, B and C
- Section A consists of 20 questions of 1 mark each. Attempt any 16 questions
- Section B consists of 20 questions of 1 mark each. Attempt any 16 questions.
- Section C consists of 10 questions based on two Case Studies. Attempt any 8 questions.
- There is no negative marking.
Section - A
Q.1: Every natural number is
(a) not a fraction
(b) an irrational number
(c) always a whole number
(d) not an integer
Correct Answer is Option (c)
All natural number together with zero form the collection of all whole number.
So,
(1) every natural number is a whole number.
(2) zero is a whole number which is not a natural number.
Q.2: The graph of the linear equation x + y = 0 passes through the point
(a) (1, -1)
(b) (1, 1)
(c) (1, 0)
(d) (0, 1)
Correct Answer is Option (a)
The graph of the linear equation x + y = 0 passes through the point (1, -1) because the co-ordinate of x and y axis satisfy the given equation.
x + y = 0
1 - 1 = 0
So we can say (1, -1) is a solution of above equation.
Q.3: In figure, AB and CD are parallel to each other. The value of x is: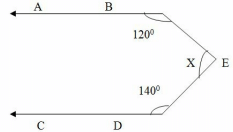
(a) 120°
(b) 100°
(c) 140°
(d) 90°
Correct Answer is Option (b)
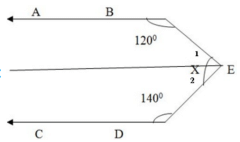
let us draw a line from point E parallel to line AB , CD
X = ∠1 + ∠2
AB EF
∠1 + 120° = 180° (co - interior angle)
∠1 = 180° - 120°
∠1 = 60°
CD EF
∠2 + 140° = 180° (co - interior angle)
∠2 = 180° - 140°
∠1 = 40°
X = ∠1 + ∠2
X = 60° + 40°
Q.4: Each side of an equilateral triangle is 10 cm long. The height of the triangle is
(a) 10√3cm
(b) 10√2cm
(c) 5√3cm
(d) 5cm
Correct Answer is Option (c)
Height of equilateral triangle = √3/2 x Side
= √3/2 x 10
= 5√3cm
Q.5: Decimal representation of a rational number cannot be
(a) non-terminating non-repeating
(b) non-terminating
(c) non-terminating repeating
(d) terminating
Correct Answer is Option (a)
Decimal representation of a rational number cannot be non-terminating non-repeating. It is always be terminating or non terminating repeating.
Q.6: If the point (3,4) lies on the graph of 3y = ax + 7 then the value of a is
(a) 2/5
(b) 2/7
(c) 3/5
(d) 5/3
Correct Answer is Option (d)
Given equation: 3y = ax + 7
Also, (3, 4) lies on the graph of the equation.
Putting x = 3, y = 4 in the equation, we get:
⇒ 3 x 4 = 3a + 7
⇒ 12 = 3a + 7⇒ 3a = 12 − 7 = 5
⇒ a = 5/3
Q.7: Each angle of an equilateral triangle is
(a) 30°
(b) 90°
(c) 45°
(d) 60°
Correct Answer is Option (d)
Let the angle of an equilateral triangle be xo
x + x + x = 180o (Angle sum property)
3x = 180o
x = 60o
Q.8: In a triangle, an exterior angle at a vertex is 95° and its one of the interior opposite angle is 55°, then the measure of the other interior angle is
(a) 85°
(b) 55°
(c) 90°
(d) 40°
Correct Answer is Option (d)
Let the other interior opposite angle be x°.
Then, we have x° + 55° = 95°
⇒ x°= 95°- 55° = 40°
Q.9: The simplest rationalisation factor of (2√2 - √3) is
(a) √2 + √3
(b) 2√2 + √3
(c) 2√2 + 3
(d) √2 - √3
Correct Answer is Option (b)
2√2 + √3
Q.10: In an examination, ten students scored the following marks: 60, 58, 90, 51, 47, 81, 70, 95, 87, 99. The range of this data is
(a) 81
(b) 52
(c) 60
(d) 51
Correct Answer is Option (b)
Difference between the maximum and minimum value of the observation is called as range.
So, 99 - 47 = 52
Q.11: An angle which is greater than 180o & less than 360o is-
(a) Straight Angle
(b) Complete Angle
(c) Obtuse Angle
(d) Reflex Angle
Correct Answer is Option (d)
Reflex angles are angles measuring greater than 180 degrees and less than 360 degrees.
Q.12: The product of two irrational numbers is
(a) always irrational
(b) always an integer
(c) always rational
(d) either irrational or rational
Correct Answer is Option (d)
√5 x √2 = √10 is a irrational number
√5 x √5 = √5 is a rational number
Q.13: If 2x = 4y = 8z and 1/2x + 1/4y + 1/4z = 4 then the value of x is
(a) 16/7
(b) 3/4
(c) 4/3
(d) 7/16
Correct Answer is Option (d)
2x = 4y = 8z
⇒
⇒ x = 2y = 3x
⇒
⇒
⇒ 7/4x = 4
⇒ x = 7/16
Q.14: If the difference between two supplementary angles is 40 then the angles are-
(a) 65° 115°
(b) 110°, 70°
(c) 80°, 100°
(d) 40°,140°
Correct Answer is Option (b)
110°, 70°
Let the supplementary angles be x and y, then according to question we have
x - y = 40° ...(i)
Also, being supplementary, we have
x + y = 180° ...(ii)
Adding (i) and (ii), we get
2x = 220°
⇒ x = 110° and hence y = 180° - 110° = 70°
Therefore, the angles are 110° and 70°.
Q.15: The equation of a line parallel to y-axis and 7 units to the left of origin is
(a) y = -7
(b) y = 7
(c) x = -7
(d) x = 7
Correct Answer is Option (c)
The equation of a line parallel to y-axis and 7 units to the left of the origin is
x = -7
because when a line parallel to y axis in that case equation of line is x = a
where a is the co-ordinate of x-axis and 7 units to the left of the origin value x -co-ordinate is -7
so required equation is x = -7
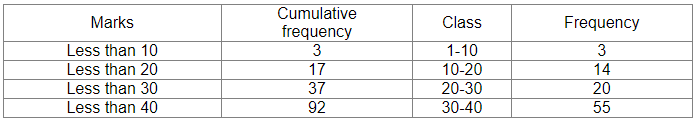
Q.16: ‘Less than’ cumulative frequency table for a given data is as follows. Then, the frequency of class interval 20-30 is
(a) 34
(b) 55
(c) 14
(d) 20
Correct Answer is Option (d)
A cumulative frequency distribution is the sum of the class and all classes below it in a frequency distribution.
Less than 30 has the class interval 20-30. Frequency of this class interval will be corresponding to
37 - 17 = 20
Q.17: If the area of an isosceles right triangle is 8 cm2, what is the perimeter of the triangle?
(a) 8 + 4√2 cm2
(b) 8 + √2 cm2
(c) 12√2 cm2
(d) 4 + 8√2 cm2
Correct Answer is Option (a)
Let each of the two equal sides of an isosceles right triangle be a cm
Then, third side = a√2m
Area of Δ = 1/2 x 2 x 2
⇒ 8 = a2/2
⇒ a2 = 16
⇒ a = 4
⇒ Perimeter
⇒ a + a + a√2 = 4 + 4 + 4√2 = 8 + 4√2cm2
Q.18: If 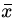 represents the mean of observations
represents the mean of observations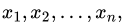 then value of
then value of 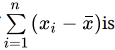 is
is
(a) - 1
(b) 1
(c) n - 1
(d) 0
Correct Answer is Option (d)
Since mean is equal to the sum of all the values in the data set divided by the number of values in the data set also called as average.
Hence, sum of difference of all the numbers & mean value will be zero.
Q.19: 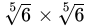 is equal to
is equal to
(a) 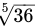
(b) 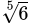
(c) 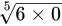
(d) 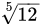
Correct Answer is Option (a)
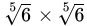
=
=
Q.20: Two straight lines AB and CD intersect one another at the point O. If ∠AOC + ∠COB + ∠BOD = 274°, then ∠AOD =
(a) 86°
(b) 137°
(c) 94°
(d) 90°
Correct Answer is Option (a)
Given,
∠AOC + ∠COB + ∠BOD = 274° ......(i)
∠AOD + ∠AOC + ∠COB + ∠BOD = 360° (Angles at a point)
∠AOD + 274° = 360°
∠AOD = 86°
Section - B
Q.21: The choices given below, choose the equation whose graphs are given in Fig.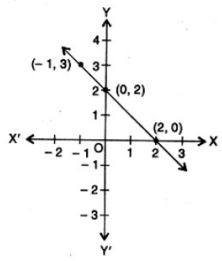
(a) x + 2y = 6
(b) y = x + 2
(c) y = -x + 2
(d) y = x - 2
Correct Answer is Option (c)
The correct equation is y = –x + 2 for: x=2,y=0=(2,0) for y=2,x=0(0,2) these points are given in the graph.
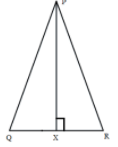
Q.22: The base of an isosceles triangle is 16 cm and its area is 48 cm2. The perimeter of the triangle is
(a) 48 cm
(b) 324 cm
(c) 41 cm
(d) 36 cm
Correct Answer is Option (d)
Let ΔPQR be an isosceles triangle and PX ⊥ QR
Now,
Area of triangle = 48 cm2
⇒ 1/2 x QR x PX
⇒ h = 96/16 = 6cm
Also,
QX = 1/2 x 24 = 12cm and PX = 12 cm
PQ =
a == 10cm
∴ Perimeter = (10 + 10 + 16) cm = 36 cm
Q.23: The equation of a line parallel to y-axis and 4 units to the right of origin is
(a) x = 4
(b) x = -4
(c) y = -4
(d) y = 4
Correct Answer is Option (a)
The equation of a line parallel to y-axis at a distance of 4 units from it, to its right from the origin.
x = 4
Because when a line parallel to y-axis in that case equation of line is x = 4.
So required equation is x = 4.
Q.24: The signs of abscissa and ordinate of a point in quadrant IV are
(a) (-, -)
(b) (+, +)
(c) (-, +)
(d) (+, -)
Correct Answer is Option (d)
In the Cartesian plane, if co-ordinate of any point P is P(x, y), then x-coordinate is called the abscissa of the point and, y-coordinate is called ordinate of the point.
The signs of abscissa and ordinate of a point in quadrant IV is (+, -)
(c) are many rational numbers
Q.25: Between any two rational numbers there
(a) is no irrational number
(b) is no rational number
(c) are many rational numbers
(d) are exactly two rational numbers
Correct Answer is Option (c)
Between any two rational number there are many rational number,
Example -4 and 8
We have 5,6,7,7.5, and many more
Q.26: A gardener want to plant grass inside the given piece of land. How much area does he need to plant.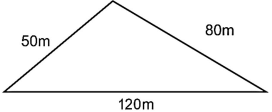
(a) 97√15 rn2
(b) 85√15 rn2
(c) 110√15 rn2
(d) 375√15 rn2
Correct Answer is Option (d)
Perimeter: 50 + 80 + 120 = 250 m
Then,
Semi-perimeter = 250/2 = 125m
s - a = (125 - 120) = 5m
s - b = (125 - 80) = 45m
s - c = (125 - 50) = 75m
Area =
Q.27: If figure, AB ⊥ BE and FE ⊥ BE. If BC = DE and AB = EF, then ΔABD is congruent to
(a) ΔCEF
(b) ΔEFC
(c) ΔECF
(d) ΔFEC
Correct Answer is Option (d)
In ΔABD and ΔFEC,
AB= FE (Given)
∠B = ∠E (Each 90°)
BC = DE (Given)
Add CD both sides, we get
BD = EC
Therefore, by S.A.S. theorem,
ΔABD ≌ ΔFEC
Q.28: The value of [3 - 4(3 - 4)4]3, is
(a) 7
(b) 0
(c) -1
(d) 1
Correct Answer is Option (c)
[3 - 4(3 - 4)4]
=[3-4]3= -1
Q.29: The point which lies on y-axis at a distance of 6 units in the positive direction of y-axis is
(a) (-6, 0)
(b) (0, -6)
(c) (6, 0)
(d) (0, 6)
Correct Answer is Option (d)
Since it lies on the y-axis so it's abscissa x will be zero.
Thus, the point will be (0, 6).
Q.30: The difference between the upper and the lower class limits is called
(a) mean
(b) class size
(c) frequency
(d) mid-points
Correct Answer is Option (b)
The difference between the upper class limit and the lower class limit is called class size.
Q.31: The length of the sides of a triangle are 5x, 5x and 8x. The area of the triangle is :
(a) 100 x2 sq. units
(b) 24 x2 sq. units
(c) 12 x2 sq. units
(d) 144 x2 sq. units
Correct Answer is Option (c)
s =
Area of triangle =
=
=
= 12x2 sq. cm
Q.32: The value of 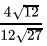 is
is
(a) 1/9
(b) 2/9
(c) 4/9
(d) 8/9
Correct Answer is Option (b)
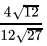
=
=
= 2/9
Q.33: In Figure, if BP||CQ and AC = BC, then the measure of x is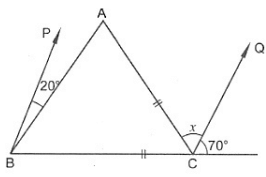
(a) 30°
(b) 20°
(c) 25°
(d) 35°
Correct Answer is Option (a)
∠PBC = ∠QCD (Corresponding angles, OP || CQ and BC is transverse)
⇒ ∠PBC = 70°
Now, ∠PBA + ∠ABC = ∠PBC
⇒ 20° + ∠ABC = 70°
⇒ ∠ABC = 50°
In ΔABC,∠ABC + ∠BAC + ∠ACB = 180° ...(i)
Now, ∠ABC + ∠BAC = 50° (isosceles )
And, ∠ACB = 180° - (70° + x)
From (i),
50° + 50° + 180° - (70° + x) = 180°
Hence x = 30°
Q.34: In a grouped frequency distribution, the class intervals are 1-20, 21-40, 41-60, ..., then the class width is
(a) 10
(b) 30
(c) 19
(d) 20
Correct Answer is Option (d)
The class width is the difference between the upper or lower class limits of consecutive classes.
In this case, class width equals to the difference between the lower limits of the first two classes.
Let, W be the class width
W = 21 - 1 = 20
So class width is 20
Q.35: In the figure, measure of x is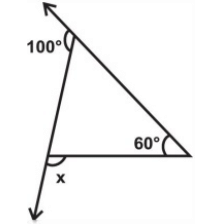
(a) 20°
(b) 60°
(c) 140°
(d) 100°
Correct Answer is Option (c)
Clearly, we can see that the internal angles are:
180 - 100, 60 and 180 - x
Or 80, 60 and 180 - x
By angle sun property of triangles, we have
80 + 60 + 180 - x = 180
⇒ x = 60 + 80
⇒ x = 140
Q.36: If (4, 19) is a solution of the equation y = ax + 3, then a =
(a) 4
(b) 6
(c) 3
(d) 5
Correct Answer is Option (a)
Given, (4, 19) is a solution of the equation y=ax+3
=19 = 4a + 3
= a = 4
Q.37: In right Δ ABC, AB = BC. Then ∠A is equal to
(a) None of these
(b) 90°
(c) 60°
(d) 45°
Correct Answer is Option (d)
AB = BC [Given]⇒∠A = ∠C (angle opposite to equal sides are equal)
Let ∠A = ∠C = xNow, 2x + 90o = 180o
x = 45o
⇒∠A =45o
Q.38: If √2 = 1.41 then 1/√2 = ?
(a) 0.705
(b) 7.05
(c) 0.75
(d) 0.075
Correct Answer is Option (a)
0.705
Q.39: If all the three angles of a triangle are equal, then each one of them is equal to
(a) 60°
(b) 30°
(c) 45°
(d) 90°
Correct Answer is Option (a)
Let the measure of each angle be x°.
Now, the sum of all angles of any triangle is 180°.
Thus, x° + x° + x° = 180°
i.e. 3x° = 180°
i.e. x° = 60°
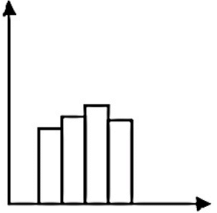
Q.40: Which of the figures represent a histogram correctly-
(a) 
(b) 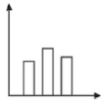
(c) 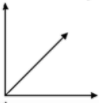
(d) 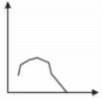
Correct Answer is Option (a)
As there are no gaps between the bars. So, this is the right way to draw a histogram.
Section - C
Question No. 41 to 45 are based on the given text. Read the text carefully and answer the questions:
Once the Maths teacher of class IX D told students that today we will prove that the sum of all three angles is 180°. As shown in the figure, he told to draw any triangle ABC in the notebook.
Further side BC was extended to D.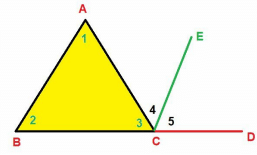
Now the teacher said to draw CE||BA.
Further angles were named 1 to 5 as shown in the figure.
Q.41: ∠2 is equal to which angle?
(a) ∠2
(b) ∠4
(c) ∠5
(d) ∠3
Correct Answer is Option (c)
∠5
Q.42: BA || CE and AC is the transverse line, So ∠1 is equal to which angle?
(a) ∠2
(b) ∠3
(c) ∠5
(d) ∠4
Correct Answer is Option (c)
∠5
Q.43: What is value of ∠3 + ∠4 + ∠5?
(a) 120°
(b) 360°
(c) 180°
(d) 200
Correct Answer is Option (c)
180°
Q.44: What is value of ∠ECD = ∠4 + ∠5?
(a) ∠1 + ∠2
(b) ∠3 + ∠4
(c) ∠2 + ∠3
(d) ∠3 + ∠5
Correct Answer is Option (a)
∠1 + ∠2
Q.45: What is value of ∠1 + ∠2 + ∠3?
(a) 360°
(b) ∠3 + ∠4 + ∠5 = 180°
(c) 280°
(d) ∠3 + ∠4 = 100°
Correct Answer is Option (b)
∠3 + ∠4 + ∠5 = 180°
Question No. 46 to 50 are based on the given text. Read the text carefully and answer the questions:
The following data given the weight (in grams) of 30 oranges picked from a basket:
106, 107, 76, 109, 187, 95, 125, 92, 70, 139, 128, 100, 88, 84, 99, 113, 204, 141, 136, 123, 90, 115, 110, 97, 90, 107, 75, 80, 118, 82.
Frequency distribution table: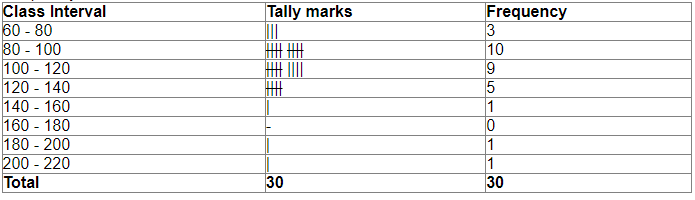
Q.46: Class Size of given class data
(a) 10
(b) 20
(c) 15
(d) 30
Correct Answer is Option (b)
20
Q.47: Classmark of forth class
(a) 20
(b) 130
(c) 70
(d) 15
Correct Answer is Option (b)
130
Q.48: The number of oranges, whose weight is more than 180 g.
(a) 3
(b) 1
(c) 4
(d) 2
Correct Answer is Option (d)
2
Q.49: The number of oranges, whose weight is less than 100 g.
(a) 3
(b) 13
(c) 5
(d) 10
Correct Answer is Option (b)
13
Q.50: The range of data is
(a) 204
(b) 274
(c) 134
(d) 70
Correct Answer is Option (c)
134
|
40 videos|471 docs|57 tests
|