JEE Advanced (Single Correct Type): Complex Numbers | Chapter-wise Tests for JEE Main & Advanced PDF Download
Q.1. The value of 1 + i2 + i4 + i6 + … + i2n is
(a) positive
(b) negative
(c) 0
(d) cannot be evaluated
Correct Answer is option (d)
1 + i2 + i4 + i6 + … + i2n = 1 – 1 + 1 – 1 + … (–1)n
This cannot be evaluated unless the value of n is known.
Q.2. If a complex number z lies in the interior or on the boundary of a circle of radius 3 units and centre (– 4, 0), the greatest value of |z +1| is
(a) 4
(b) 6
(c) 3
(d) 10
Correct Answer is option (b)
The distance of the point representing z from the centre of the circle is |z – (-4 + i0)| = |z + 4|
According to the given,
|z + 4| ≤ 3
Now,
|z + 1| = |z + 4 – 3| ≤ |z + 4| + |-3| ≤ 3 + 3 ≤ 6
Hence, the greatest value of |z + 1| is 6.
Q.3. If 1 – i, is a root of the equation x2 + ax + b = 0, where a, b ∈ R, then the value of a – b is
(a) -4
(b) 0
(c) 2
(d) 1
Correct Answer is option (a)
Given that 1 – i is the root of x2 + ax + b = 0.
Thus, 1 + i is also the root of the given equation since non-real complex roots occur in conjugate pairs.
Sum of roots = −a/1 = (1 – i) + (1 + i)
⇒ a = – 2
Product of roots, b/1 = (1 – i)(1 + i)
b = 1 – i2
b = 1 + 1 {since i2 = -1}
⇒ b = 2
Now, a – b = -2 – 2 = -4
Q.4. If [(1 + i)/(1 – i)]x = 1, then
(a) x = 2n + 1, where n ∈ N
(b) x = 4n, where n ∈ N
(c) x = 2n, where n ∈ N
(d) x = 4n + 1, where n ∈ N
Correct Answer is option (b)
Given,
[(1 + i)/(1 – i)]x = 1
By rationalising the denominator,
[(1 + i)(1 + i)/ (1 – i)(1 + i)]x = 1
[(1 + i)2/ (1 – i + i – i2)]x = 1
[(1 + i2 + 2i)/(1 + 1)]x = 1
[(1 – 1 + 2i)/ 2]x = 1
ix = 1
Thus, ix = i4n, where n is any positive integer.
Q.5. The simplified value of (1 – i)3/(1 – i3) is
(a) 1
(b) -2
(c) -i
(d) 2i
Correct Answer is option (b)
(1 – i)3/(1 – i3)
= (1 – i)3/(13 – i3)
= (1 – i)3/ [(1 – i)(1 + i + i2)]
= (1 – i)2/(1 + i – 1)
= (1 – i)2/i
= (1 + i2 – 2i)/i
= (1 – 1 – 2i)/i
= -2i/i
= -2
Q.6. sin x + i cos 2x and cos x – i sin 2x are conjugate to each other for:
(a) x = nπ
(b) x = [n + (1/2)] (π/2)
(c) x = 0
(d) No value of x
Correct Answer is option (d)
Consider sin x + i cos 2x and cos x – i sin 2x are conjugate to each other.
So, sin x – i cos 2x = cos x – i sin 2x
On comparing real and imaginary parts of both sides, we get
⇒ sin x = cos x and cos 2x = sin 2x
⇒ sin x/cos x = 1 and (cos 2x/sin 2x) = 1
⇒ tan x = 1 and tan 2x = 1
Now, consider tan 2x = 1
Using the formula tan 2A = 2 tan A/(1 – tan2A),
(2 tan x)/(1 – tan2x) = 1
However, this is not possible for tan x = 1.
Therefore, for no value of x, sinx + i cos 2x and cos x – i sin 2x are conjugate to each other
Q.7. If a + ib = c + id, then
(a) a2 + c2 = 0
(b) b2 + c2 = 0
(c) b2 + d2 = 0
(d) a2 + b2 = c2 + d2
Correct Answer is option (d)
Given,
a + ib = c + id
⇒ |a + ib| = |c + id|
⇒ √(a2 + b2) = √(c2 + d2)
Squaring on both sides, we get;
a2 + b2 = c2 + d2
Q.8. The value of arg (x) when x < 0 is
(a) 0
(b) π/2
(c) π
(d) none of these
Correct Answer is option (c)
Let z = x + 0i and x < 0
Since the point (-x, 0) lies on the negative side of the real axis,
|z| = |x + oi| = √[(-1)2 + 0)] = 1
∴ Principal argument (z) = π
Alternative method:
Let x = cos θ + i sin θ
For θ = π, x should be negative.
Thus, x < 0 for θ = π.
Q.9. Number of solutions of the equation z2 + |z|2 = 0 is
(a) 1
(b) 2
(c) 3
(d) infinitely many
Correct Answer is option (d)
Given,
z2 + |z|2 = 0, z ≠ 0
⇒ (x + iy)2 + [√(x2 + y2)]2 = 0
⇒ x2 – y2 + i2xy + x2 + y2 = 0
⇒ 2x2 + i2xy = 0
⇒2x (x + iy) = 0
⇒ x = 0 or x + iy = 0 (not possible)
Therefore, x = 0 and z ≠ 0.
Thus, y can have any real value.
Hence, there exist infinitely many solutions.
Q.10. If the complex number z = x + iy satisfies the condition |z + 1| = 1, then z lies on
(a) x-axis
(b) circle with centre (1, 0) and radius 1
(c) circle with centre (–1, 0) and radius 1
(d) y-axis
Correct Answer is option (c)
Given,
z = x + iy
and
|z + 1| = 1
|x + iy + 1| = 1
⇒ |(x + 1) + iy| = 1
⇒ √[(x +1)2 + y2] = 1
Squaring on both sides,
(x + 1)2 + y2 = 1
This is the equation of a circle with centre (–1, 0) and radius 1.
Q.11. If Z1, Z2 are two complex numbers such that |Z1|= 1, |Z2| = 1 then the maximum value of |Z1 + Z2| + |Z1 - Z2| is
(a) 2
(b) 2√2
(c) 4
(d) none of these
Correct Answer is option (b)
Let α - β = θ
Q.12. For complex numbers z1, z2 satisfy |z1| = 12 and |z2 – 3 – 4i| = 5, the minimum value of |z1 – z2| is
(a) 0
(b) 2
(c) 7
(d) 17
Correct Answer is option (b)
We know,
| z1 - z2 |=| z1 - (z2 -3- 4i) - 3+ 4i | ≥ | z1 |- | z2 - 3- 4i |-| 3+ 4i | ≥ 12 - 5 - 5
(using | z1 - z2 | ≥ | z1 | - | z2 | )
∴ | z1 - z2 | ≥ 2
Q.13. If P(z) and A(z1) two be variable points such that zz1 = |z|2 and 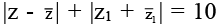 then area enclosed by the curve formed by them
then area enclosed by the curve formed by them
(a) 25p
(b) 20 p
(c) 50
(d) 100
Correct Answer is option (c)
Now let z = x + iy
⇒ z1 = x - iy
So,
Which represent a square of area 25.
Q.14. If Z = 1 and Z ≠ 1 , then all the values of 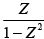 lie on
lie on
(a) a line not passing through the origin
(b) Z = √2
(c)The x - axis
(d) The y – axis
Correct Answer is option (d)
Let z = cosθ + i sinθ
⇒
=
Hencelies on the imaginary axis i.e. y-axis.
Q.15. If |z1| and |z2| are two distinct non-zero complex numbers such that |z1| = |z2|, then
(a) purely real
(b) purely imaginary
(c) equal to zero
(d) none of these
Correct Answer is option (b)
=
=
⇒is purely imaginary.
Q.16. If |z1 + z2|2 = |z1 – z2|2 where z1 and z2 are non-zero complex numbers, then
(a) Re(z1/z2) = 0
(b) Im(z1/z2) = 0
(c) Re(z1 + z2) = 0
(d) none of these
Correct Answer is option (a)
⇒
⇒
Q.17. If z = x + iy, z1/3 = a – ib and 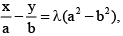 then λ is equal to
then λ is equal to
(a) 2
(b) 3
(c) 4
(d) 1
Correct Answer is option (c)
z1/3 = a – ib
⇒ x + iy = (a + i(B)3 = a3 + (-i(B)3 - 3aib(a - i(B)
⇒ x + iy = a3 + ib3 – 3a2bi – 3ab2
⇒ x = a3 – 3ab2, y = b3 – 3a2b
⇒
∴
Q.18. The circles x2 + y2 – 10x + 9 = 0 and x2 + y2 = r2 intersect each other in two distinct points if
(a) r > 8
(b) r < 2
(c) 7 < r < 11
(d) 1 < r < 9
Correct Answer is option (d)
C1 ≡ (5, 0), r1 = 4
C2 ≡ (0, 0), r2 = r
(C1 C2) = 5
So, r - 4 < 5 < r + 4
⇒ r < q & r > 1
⇒ 1 < r < 9.
Q.19. If P and Q are represented by the numbers z1 and z2 such that 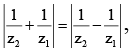
then the circumcentre of DOPQ, (where O is the origin) is
(a) 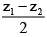
(b) 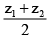
(c) 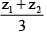
(d) z1 + z2
Correct Answer is option (b)
⇒ |z1 + z2| = |z1 – z2|
⇒
z1/z2 is purely imaginary
⇒
⇒
⇒ Circumcentre of DPOQ is the mid point of PQ i.e.
Q.20. Let z1 and z2 be two complex numbers with α and β as their principal arguments, such that α + β > π , then principal arg( z1z2 )is given by
(a) α + β + π
(b) α + β - π
(c) α + β - 2π
(d) α + β
Correct Answer is option (c)
arg (z1z2) = arg (z1) + arg(z2) + 2mπ, m ∈ I = α + β - 2π
which should be equivalent to negative angle 7π / 6 - 2π
|
446 docs|930 tests
|

|
Explore Courses for JEE exam
|

|






 lies on the imaginary axis i.e. y-axis.
lies on the imaginary axis i.e. y-axis.


 is purely imaginary.
is purely imaginary.


























