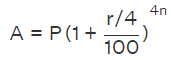Compound Interest | Mathematics for JAMB PDF Download
Compound interest is an interest accumulated on the principal and interest together over a given time period. The interest accumulated on a principal over a period of time is also accounted under the principal. Further, the interest calculation for the next time period is on the accumulated principal value. Compound interest is the new method of calculation of interest used for all financial and business transactions across the world. The power of compounding can easily be understood, when we observe the compound interest values accumulated across successive time periods.
A sum of money of $100 invested over a period of time for a 10% rate would give a simple interest of $10, $10, $10... over successive time periods of 1 year, but would give a compound interest of $10, $11, $12.1, $13.31...
What is meant by Compound Interest?
Compound interest is the interest paid on both principal and interest, compounded at regular intervals. At regular intervals, the interest so far accumulated is clubbed with the existing principal amount and then the interest is calculated for the new principal. The new principal is equal to the sum of the Initial principal, and the interest accumulated so far.
Compound Interest = Interest on Principal + Compounded Interest at Regular Intervals The compound interest is calculated at regular intervals like annually(yearly), semi-annually, quarterly, monthly, etc; It is like, re-investing the interest income from an investment makes the money grow faster over time! It is exactly what the compound interest does to the money. Banks or any financial organization calculate the amount based on compound interest only.
Compound Interest Formula
The compound interest is calculated, after calculating the total amount over a period of time, based on the rate of interest, and the initial principal. For an initial principal of P, rate of interest per annum of r, time period t in years, frequency of the number of times the interest is compounded annually n, the formula for calculation of amount is as follows.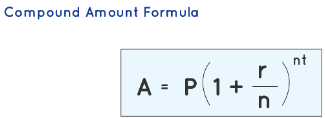
The above formula represents the total amount at the end of the time period and includes the compounded interest and the principal. Further, we can calculate the compound interest by subtracting the principal from this amount. The formula for calculating the compound interest is as follows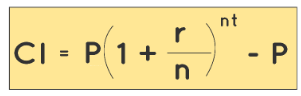
In the above expression,
- P is the principal amount
- r is the rate of interest(decimal)
- n is frequency or no. of times the interest is compounded annually
- t is the overall tenure.
It is to be noted that the above-given formula is the general formula when the principal is compounded n number of times in a year. If the given principal is compounded annually, the amount after the time period at percent rate of interest, r, is given as:
A = P(1 + r/100)t, and C.I. would be: P(1 + r/100)t - P .
 |
Test: Simple & Compound Interest
|
Start Test |
Derivation of Compound Interest Formula
The formula for compound interest can be derived from the formula for simple interest. The formula for simple interest is the product of the principal, time period, and rate of interest (SI = ptr/100). Before looking into to derivation of the formula for compound interest, let us understand the basic difference between simple interest, compound interest computation. The principal remains constant over a period of time, for simple internet computation, but for compound interest computation the interest is added to the principal, for compound interest computation.
Derivation:
The derivation of the compound interest formula is given in the following steps:
- Let the principal be P and the rate of interest be R% per annum. Since the interest is compounded annually, so the compounding period is 1 year. It is to be noted that the principal (P) will change after every 1 year. So, let us calculate the interest for the first year. The interest for the first year will be as follows:
- Let the interest for the first year be I1
- I1 = R% of P = R/100 × P
- Now, the amount at the end of the first year will be:
- A1 = P + I1 = P + (R/100 × P). This will give A1 = P (1 + R/100)
- Now, let us calculate the interest for the second year. It is to be noted that the amount of the first year will become the Principal of the second year. Let this Principal be P2
- Now, P2 = A1 = P (1 + R/100)
- Now, let us take the interest for the second year to be I2 = R% of P2, which is R/100 × P2 = R/100 × P(1 + R/100)
- Now, the amount at the end of the second year will be A2 = P2 + I2 which is P (1 + R/100) + R/100 × P(1 + R/100)
- This expression can be written as A2 = P (1 + R/100) (1 + R/100) = P (1 + R/100)2
- Continuing in this manner for n compounding periods, the amount at the end of the nth compounding period can be expressed as A = P(1 + R/100)n.
- From the above formulas and computations, you can observe that the compound interest is the same as simple interest for the first interval. But, over a period of time, there is a remarkable difference in returns.
The simple interest value for each of the years is the same, as the principal on which it is calculated is constant. But the compound interest is varying and increasing across the years. Because the principal on which the compound interest is calculated is increasing. The principal for a particular year is equal to the sum of the initial principal value, and the accumulated interest of the past years.
For example, a sum of $10,000 is deposited at a rate of 10%. The below table explains the difference between simple interest and compound interest computation on this principal: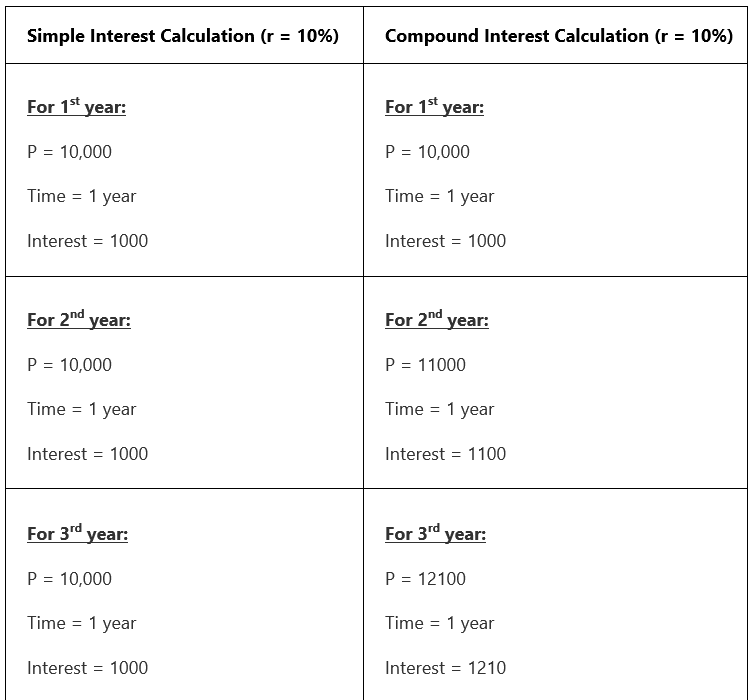
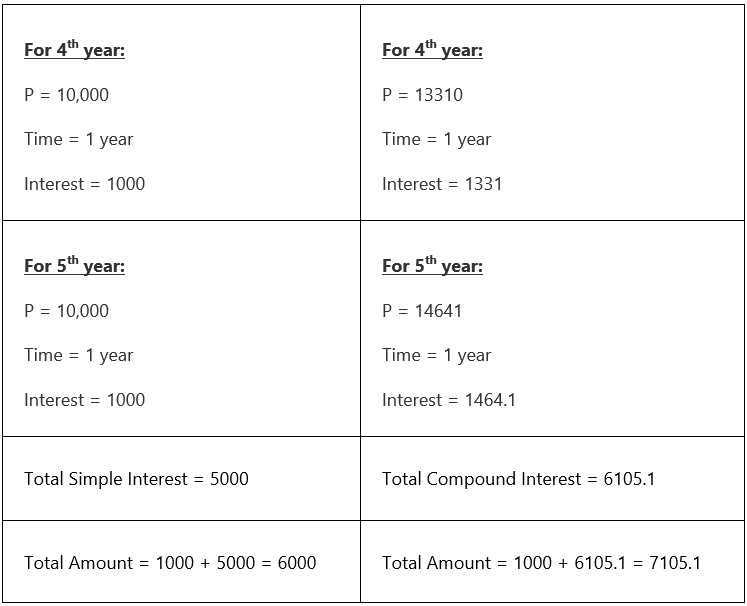
Compound Interest Formula for Different Time Periods
Compound interest for a given principal can be calculated for different time periods using different formulas.
Compound Interest Formula - Half Yearly
The interest in the case of compound interest varies based on the period of computation. If the time period for the calculation of interest is half-yearly, the interest is calculated every six months, and the amount is compounded twice a year. The formula to calculate the compound interest when the principal is compounded semi-annually or half-yearly is given as: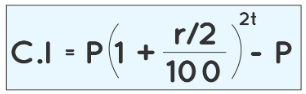
Here the compound interest is calculated for the half-yearly period, and hence the rate of interest r, is divided by 2 and the time period is doubled. The formula to calculate the amount when the principal is compounded semi-annually or half-yearly is given by: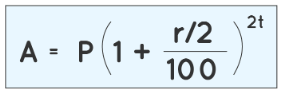
In the above expression,
- A is the amount at the end of the time period
- P is the initial principal value, r is the rate of interest per annum
- t is the time period
- C.I. is the compound interest.
Compound Interest Formula - Quarterly
If the time period for the calculation of interest is quarterly, the interest is calculated for every three months, and the amount is compounded 4 times a year. The formula to calculate the compound interest when the principal is compounded quarterly is given as: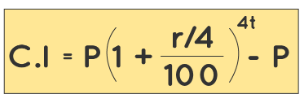
Here the compound interest is calculated for the quarterly time period, and hence the rate of interest r, is divided by 4 and the time period is quadrupled. The formula to calculate the amount when the principal is compounded quarterly is given by: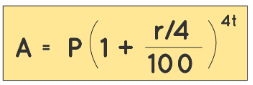
In the above expression,
- A is the amount at the end of the time period
- P is the initial principal value, r is the rate of interest per annum
- t is the time period
- C.I. is the compound interest.
Monthly Compound Interest Formula
The monthly compound interest formula is also known as the interest calculated per month i.e., n = 12.
Total compound interest is the final amount excluding the principal amount.
The monthly compound interest formula is expressed as:
CI = P (1 + r/12)12t - P
Daily Compound Interest Formula
When the amount compounds daily, it means that the amount compounds 365 times in a year. i.e., n = 365. The daily compound interest formula is expressed as:
CI = P (1 + r/365)365t - P
Important Notes
- Compound interest depends on the amount accumulated at the end of the previous tenure but not on the original principal.
- Banks, insurance companies, etc. generally levy compound interest.
- If the interest is compounded quarterly, the formula of amount is given by:

- While calculating the compound interest, the rate of interest, and each time period must be of the same duration.
Tips & Tricks
- The rule of 72: It is a quick method to know how long it will take for your money to double. Doubling Time = 72/Interest Rate
- Using the rule of 72, we can find the number of years to double your money by simply dividing 72 by the rate of interest. For example, at an 8% compounded interest rate your money will double in 72 ÷ 8 = 9
- The time duration over which an interest rate is applicable is referred to in many different terms. Sometimes it is called “per annum” or “annual” or “per year”. All of these mean you’ll get the given rate of interest over a period of 1 year. Semi-annual is 6 months. While quarterly is 3 months duration.
|
139 videos|82 docs|101 tests
|