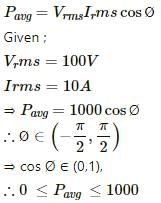HC Verma Questions and Solutions: Chapter 39: Alternating Current- 1 | HC Verma Solutions - JEE PDF Download
Short Answers
The reactance of a capacitor is given by,
For a DC source, ω = 0
So, for a constant DC source, reactance of a capacitor is infinite.
Q.2. The voltage and current in a series AC circuit are given by V = V0cos ωt and i = i0 sin ωt. What is the power dissipated in the circuit?
Voltage, V = V0cos ωt
Current, i = i0 sin ωt or i = i0 cos
Power dissipated in an AC circuit is given by,
where Irms = rms value of current
Vrms= rms value of voltage
ϕ = phase difference between current and voltage
Here, ϕ = π/2
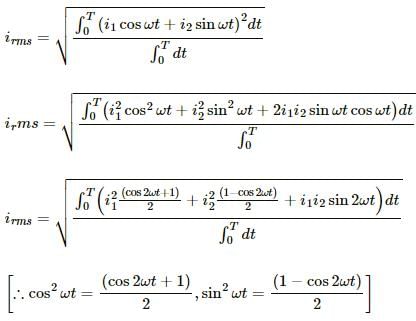
Q.3. Two alternating currents are given by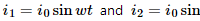
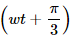 Will the rms values of the currents be equal or different?
Will the rms values of the currents be equal or different?
The rms value of current is given by,
Since peak value of current i0 is same for both currents, their rms values will be same.
Q.4. Can the peak voltage across the inductor be greater than the peak voltage of the source in an LCR circuit?
Let a LCR circuit is connected across an AC supply with the emf E = E0 sin ωt.
Let the inductance in the circuit be L
Let the net impedence of the circuit be Z =
Where,
R = resistance in the circuit
XL = reactance due to inductor
XC = reactance due to capacitor
The magnitude of the voltage across the inductor is given by
The current in the circuit can be written as
Where, ϕ is the phase difference between the current and the supply voltage
Thus, the voltage across the inductor can be written as
Therefore, the peak voltage across the inductor is given by
if (L/R) x L,
if L/R > 1
V > E0
Therefore if magnitude of (L/R) > 1 at resonance the value of the voltage across the inductor will bw greater than the peak value of the supply voltage.
Q.5. In a circuit, containing a capacitor and an AC source, the current is zero at the instant the source voltage is maximum. Is it consistent with Ohm's Law?
Ohm's Law is valid for resistive circuits only. It is not valid for capacitive or inductive circuits, or a combination of both.
Q.6. An AC source is connected to a capacitor. Will the rms current increase, decrease or remain constant if a dielectric slab is inserted into the capacitor?
The reactance of a capacitor is given by,
Also,
where C = capacitance
K = dielectric constant
A = area of plates
d = distance between the plates.
K > 1
∴ The capacitance C of the capacitor will increase on inserting the dielectric slab and, consequently, the reactance Xc will decrease.
Rms current,
Therefore, rms current will decrease.
Q.7. When the frequency of the AC source in an LCR circuit equals the resonant frequency, the reactance of the circuit is zero. Does it mean that there is no current through the inductor or the capacitor?
The condition for resonance is:
The peak current through the circuit is given by,
From the condition of resonance, we get:
i0 = V0/R
The current will flow through the all circuit elements. But since the reactance of the capacitor and inductor are equal, the potential difference across them will be equal and opposite and will cancel each other.
Q.8. When an AC source is connected to a capacitor, there is a steady-state current in the circuit. Does it mean that the charges jump from one plate to the other to complete the circuit?
No. When an AC source is connected to a capacitor, there is a steady in the circuit to transfer change to the plates of the capacitor. This produces a potential difference between the plates. The capacitance is alternatively charged and discharged as the current reverses after each half cycle.
Q.9. A current i1 = i0 sin ωt passes through a resistor of resistance R. How much thermal energy is produced in one time period? A current i2 = −i0 sin ωt passes through the resistor. How much thermal energy is produced in one time period? If i1 and i2 both pass through the resistor simultaneously, how much thermal energy is produced? Is the principle of superposition obeyed in this case?
The thermal energy produced for an AC circuit in one time period is given by,
For current, i1 = i0 sin ωt,
For current, i2 = −i0 sin ωt,
Hence, the same thermal energy will be produced due to this current.
Since, the direction of i1 and i2 are opposite and their magnitude is same, the net current through the resistor will become zero when both are passed together. Yes, the principle of superposition is obeyed in this case.
Q.10. Is energy produced when a transformer steps up the voltage?
When a transformer steps up the voltage, the voltage increases but current decreases. Neglecting any loss of energy, the power remains constant and, hence, energy is not produced. It remains constant.
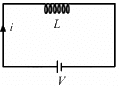
Q.11. A transformer is designed to convert an AC voltage of 220 V to an AC voltage of 12 V. If the input terminals are connected to a DC voltage of 220 V, the transformer usually burns. Explain.
A transformer is ideally an inductive coil. For an inductor connected across a DC voltage,
For a DC source, the current across the inductor will increase with time and can reach a very large value, which can burn the transformer.
Q.12. Can you have an AC series circuit in which there is a phase difference of (a) 180° (b) 120° between the emf and the current?
Let us consider an AC series LCR circuit of angular frequency ω. The impedance of the circuit is given by,
The phase difference between V and I is given by,
From the above formula, we can clearly see that
So, we cannot have a phase difference of 180° or 120°.
Q.13. A resistance is connected to an AC source. If a capacitor is included in the series circuit, will the average power absorbed by the resistance increase or decrease? If an inductor of small inductance is also included in the series circuit, will the average power absorbed increase or decrease further?
When a capacitor is included in a series circuit, the impedance of the circuit,
The power absorbed by a resistor is given by,
Since impedance increases due to introduction of a capacitor, the rms value of current Irms will decrease and, hence, the power absorbed by the resistor will decrease.
When a small inductance is introduced in the circuit, the impedance of the circuit,
Since the impedance now decreases a little, the rms value of current will increase and, hence, the power absorbed by the resistor will increase.
Q.14. Can a hot-wire ammeter be used to measure a direct current of constant value? Do we have to change the graduations?
A hot-wire ammeter measures the rms value of current for an alternating current. So, it can be used to measure the direct current of constant value because that constant value will be equal to the rms value of current. As, the rms value of the current is same as the direct current thus we need not change the graduations.
Multiple Choice Questions
|
134 docs
|
FAQs on HC Verma Questions and Solutions: Chapter 39: Alternating Current- 1 - HC Verma Solutions - JEE
| 1. What is alternating current (AC)? |  |
| 2. What are the advantages of using alternating current over direct current? |  |
| 3. How is alternating current generated? |  |
| 4. What is the frequency of alternating current? |  |
| 5. How is alternating current measured? |  |

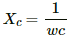
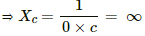
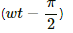
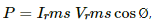
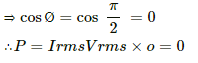
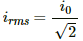
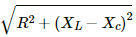
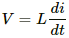
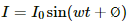
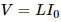
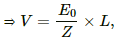
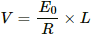
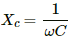
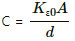
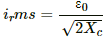
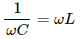
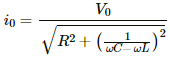
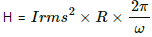
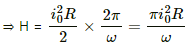
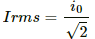
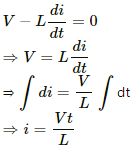
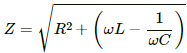
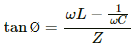
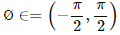
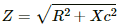
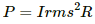
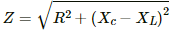
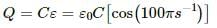
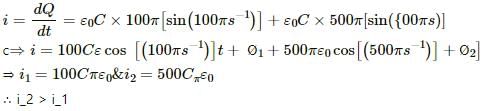
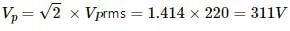
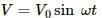
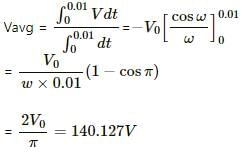
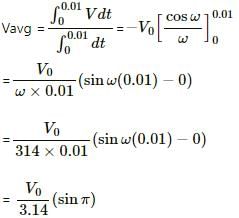
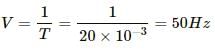
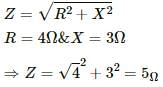
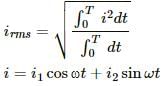
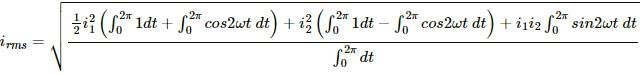
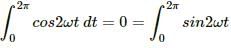
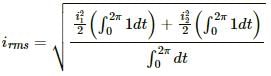
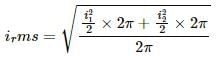
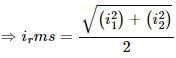
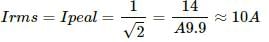
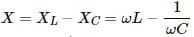
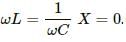 so, the circuit contains an inductor and a capacitor.
so, the circuit contains an inductor and a capacitor.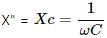
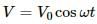
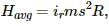 which is non zero
which is non zero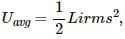 which is also non zero
which is also non zero