Class 7 Maths Chapter 6 Practice Question Answers - Lines and Angles

Q1. Two supplementary angles are in the ratio 4 : 5. The angles are
(a) 90^∘,90^∘
(b) 80^∘,100^∘
(c) 30^∘,150^∘
(d) 45^∘,45^∘
Ans: (b)
Sol: Let the angles be 4x and 5x
Angles are supplementary
∴ 4x + 5x = 180^∘
⇒9x = 180^∘
⇒ x = 20^∘
So the angles are
4 × 20^∘ = 80^∘
5 × 20^∘ = 100^∘

Q2. The complement of (90^∘ −a) is :
(a) −a
(b) 90^∘ +a
C90^∘ −a
(d) a
Ans: (d)
Sol: Let the complement be y.
We know, two angles are complementary if their sum is 90^∘ .
∴ 90^∘ −a + y = 90^∘
⇒ y = a.
Hence, option D is correct.

Q3. Find the angle which is 20^o  more than its supplement.
(a) 90
(b) 95
(c) 100
(d) 105
Ans: (c)
Sol: Let the required angle be x, then its supplement =(180 − x)
Given that x = (180 − x) + 20
⇒ 2x = 200
⇒ x = 100 

Q4. Find the angle which is 30^o  more than its complement.
(a) 50^o
(b) 55^o
(c) 60^o
(d) 65^o
Ans: (c)
Sol: Let the required angle be x.
Then, its complement =(90^o −x).
Given, the angle is 30^o  more than its complement.
Then, x=(90^o −x)+30^o
⇒ x + x=90^o +30^o
⇒ 2x=120^o
⇒ x = 120^o/2
⇒ x = 60^o

Q5. Find the angle which is equal to its supplement
(a) 80^o
(b) 90^o
(c) 110^o
(d) 154^o
Ans: (b)
Sol: Let the required angle be x, then its supplement = (180^o − x)
Given that x = (180^o − x)
⇒ 2x = 180^o
⇒ x = 90^o

Q6. If a transversal intersects a pair of lines in such a way that the sum of interior angles on the same side of transversal is 180^o , then the lines are
(a) parallel
(b) intersecting
(c) coincident
(d) None of these
Ans: (a)
Sol: If a transversal intersects a pair of lines in such a way that the sum of interior angles on the same side of transversal is 180^∘ then the lines are parallel.
Option A is correct.

 
Q7. If two angles are complementary of each other, then each angle is:
(a) an obtuse angle
(b) a right angle
(c) an acute angle
(d) a supplementary angle
Ans: (c)
Sol: We know, two angles whose sum is equal to 90^o  are known as complementary angles.
Also, acute angle is the angle which is greater than 0^o  and less than 90^o .
Hence, if two angles are complement of each other, then each of them should necessarily be acute angle.
Therefore, option C is correct.

Q8. If one angle of a linear pair is acute, then its other angle will be ______.
(a) Obtuse angle
(b) Acute angle
(c) Right angle
(d) None of these
Ans: (a)
Sol: Given: An angle of a linear pair is acute i.e., it is less than 90^∘ .
We know that linear pair of angles are supplementary i.e., they add up to form 180^∘
So the other angle must be greater than 90^∘
So the other angle should be obtuse.

Q9. Find the measure of the complementary angle of each of 20^∘.
(a) 50^∘
(b) 70^∘
(c) 160^∘
(d) 80^∘
Ans: (b)
Sol: We know, two angles are complementary when they add up to 90^∘ .
Given, measure of one complementary angle is =20^∘
⇒ Measure of other complementary angle = 90^∘ - 20^∘ = 70^∘
∴ Measure of a complementary angle of 20^∘ = 70^∘
Therefore, option B is correct.

Q10. A ray stands on a line, then the sum of the two adjacent angles so formed is ______.
(a) 180^∘
(b) 90^∘
(c) 360^∘
(d) 270^∘
Ans: (a)
Sol: If a ray stands on a line, then the sum of two adjacent angles so formed is equal to 180^∘ which is also known as a linear pair.

Q11. If two straight lines intersect, the measures of the vertically opposite angles are ________.
(a) equal
(b) not equal
(c) cannot be determined
(d) Nont of these
Ans: (a)
Sol: If two straight lines intersect, the measures of the vertically opposite angles are Equal.
To prove : ∠CBE=∠DBA
We know,
∠CBE+∠DBC=180^∘  ( Linear Pair)
∠CBE=180^∘  −∠DBC→ (I)
Again,
∠DBA+∠DBC=180^∘  ( Linear Pair)
∠DBA=180^∘ −∠DBC→  (II)
∴∠CBE=∠DB(a)

Q12. If an angle is 28^∘ less than its complement, find its measure.
(a) 31^∘
(b) 131^∘
(c) 28^∘
(d) 32^∘
Ans: (a)
Sol: Let the required angle be x.
Then, its complement = (90^∘ - x)
Given, the angle is 28^∘  less than its complement.
Then, x = (90^∘ - x) - 28^∘
⇒ x + x = 90^∘  - 28^∘
⇒ 2x = 62^∘
x = 62^∘ / 2
x = 31^∘

Q13. Find the measure of the supplementary angle of 138^∘ .
(a) 48^∘
(b) 42^∘
(c) 52^∘
(d) 38^∘
Ans: (b)
Sol: Two angles are supplementary when they add upto form 180^∘.
Given, one angle = 138^∘.
Let the supplement angle be x
Hence, x = 180^∘ - 138^∘ = 42^∘
Hence, supplementary angle of the following angle is 42^∘.

Q14. Supplementary angle of 102^∘  is:
(a) 180^∘
(b) 90^∘
(c) 78^∘
(d) 60^∘
Ans: (c)
Sol: As sum of supplementary angles if 180^∘
Supplementary angle of 102^∘ = 180^∘  −102^∘ = 78^∘

Q15. Find the measure of the supplementary angle of 132^∘ .
(a) 48^∘
(b) 32^∘
(c) 42^∘
(d) 38^∘
Ans: (a)
Sol: Two angles are Supplementary when their sum is equal to 180^∘ .
If one angle =132^∘ , let the other angle be x.
Hence, x + 132^∘ = 180^∘
x = 180^∘ − 132^∘
= 48^∘
Hence, supplementary angle of 132^∘  is 48^∘ .

Q16. Which angles in the given figure are complementary?

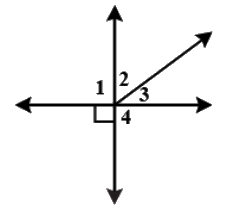(a) ∠1 and ∠2
(b) ∠2 and ∠3
(c) ∠3 and ∠4
(d) ∠4 and ∠1
Ans: (b)
Sol: We know, two angles whose sum is equal to 90^∘  are known as complementary angles.
Here, the sum, ∠2+∠3, is vertically opposite to a perpendicular.
⇒∠2 + ∠3=90^∘ .
Hence ∠2 and ∠3 are complementary.
Therefore, option B is correct. 

Q17. If two interior angles on the same side of a transversal intersecting two parallel lines are in the ratio 3:7, then the measure of the larger angle is
(a) 54^∘
(b) 120^∘
(c) 126^∘
(d) 108^∘
Ans: (c)
Sol: Let the angles be 3x and 7x.
We know that sum of two interior angles on the same side of transversal is 180^∘ .
3x + 7x = 180^∘
⇒ 10x = 180^∘
⇒ x = 18^∘
Therefore, the greater angle is 7x = 7 × 18^∘ = 126^∘

Q18. Find the measure of the complementary angle of 90^∘ .
(a) 0^∘
(b) 45^∘
(c) 90^∘
(d) 60^∘
Ans: (a)
Sol: The pair of angles is said to be complementary, when their sum is 90^∘ .
Let x, y be any two complementary angles and (∠x) = 90^∘
⇒ (∠x) + (∠y) = 90^∘   ........ (By definition of complementary angles)
90^∘ + (∠y) = 90^∘
(∠y) = 0^∘
Hence, measure of complementary angle of 90^∘ is 0^∘ .

Q19. Given : AB || C(d) If ∠4 is an obtuse angle, then ∠5 is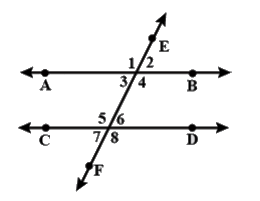

(a) Acute
(b) Right
(c) Obtuse
(d) Straight
Ans: (c)
Sol: As AB∣∣CD and EF is transversal.
∠5=∠4  (Alternate angles)
Now ∠4 is obtuse therefore ∠5 is also obtuse

Q20. If two lines intersect such that four vertical angles are equal, then each angle is:
(a) 45^∘
(b) 100^∘
(c) 180^∘
(d) 90^∘
Ans: (d)
Sol: Let each vertical angle be x
Now the sum of vertical angles is 360^∘
⇒ x + x + x + x = 360^∘
⇒ 4x = 360^∘
⇒ x = 360^∘ / 4 = 90^∘
So option D is correct.

Q21. Which two angles are supplementary?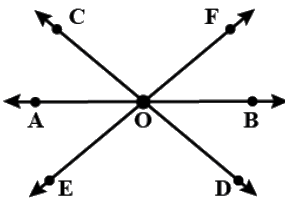

(a) ∠COF and ∠AOC
(b) ∠COF and ∠COE
(c) ∠COF and ∠EOD
(d) ∠COF and ∠AOE
Ans: (b)
Sol: ∠COF and ∠COE are supplementary because they formed on the line EOF
⇒ ∠COF + ∠COE = 180^∘
So option B is correct.

Q22. The complementary angle of 30^∘  is:
(a) 60^∘
(b) 90^∘
(c) 150^∘
(d) none of these
Ans: (a)
Sol: We know, two angles are complementary when they add up to 90^∘
Given, measure of one complementary angle is 30^∘
⇒ Measure of other complementary angle = 90^∘ - 30^∘ = 60^∘
∴ Measure of a complementary angle of 30^∘ =60^∘
Hence, option A is correct.

Q23. The measure of ∠POQ in the following figure is: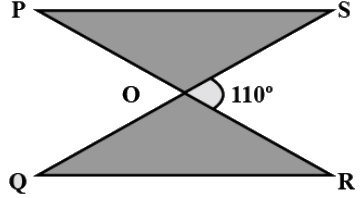

(a) 90^∘
(b) 70^∘
(c) 20^∘
(d) 110^∘
Ans: (d)
Sol: If two straight lines intersect the measures of vertically opposite angles are equal.
Given, ∠SOR=110^∘
Therefore, ∠SOR=∠POQ
⇒∠POQ=110^∘

Q24. If sum of two angles is 90^∘ . They will be:
(a) Linear pair
(b) Supplementary angles
(c) Complementary angles
(d) Corresponding angles
Ans: (c)
Sol: By definition we know that two angles, the sum of whose measure is 90^∘ are called complimentary angles.
Then, such angles are called complement of each other.
E.g.: Let the measure of one complementary angle is 58^∘ .
⇒ Measure of other complementary angle = 90^∘ - 58^∘ = 32^∘
∴ Measure of the complementary angle of 58^∘ = 32^∘
Hence, 58^∘ and 32^∘ are complements of each other.
That is, if the sum of two angles is 90^∘
Therefore, option C is correct.

Q25. Two adjacent angles whose sum is 180^∘  is called
(a) complementary angles
(b) linear pair
(c) vertically opposite angles
(d) none
Ans: (b)
Sol: Two adjacent angles whose sum is 180^∘ is called linear pair.
Ex: 70^∘ + 110^∘ = 180^∘ then these two angles is called a linear pair.

Q26. A line which intersects two or more lines at different points is:
(a) perpendicular
(b) transversal
(c) parallel
(d) none of these
Ans: (b)
Sol: 

(A) Perpendiculars are the lines which intersect each other.
(C) Parallel lines do not intersect each other at any point.
(B) In geometry, a line that passes through two or more lines in the same plane at two or more different points is called transversal.
Transversals play a role in establishing whether two other lines in the Euclidean plane are parallel.
Hence, option B is correct.

Q27. p∣∣q,r∣∣s then p is _____.
(a) p∣∣s
(b) p∣∣r
(c) not parallel to s
(d) none of these
Ans: (c)
Sol: Given p∣∣q  and   r∣∣s.
No relation is given between line p with r and s
So p is not parallel to s
Option C is correct.

Q28. A pair of angles with a common vertex and common arm are called
(a) Adjacent angles
(b) Complementary
(c) Supplementary
(d) None
Ans: (a)
Sol: A pair of angles with a common vertex and common arm are called adjacent angles.

Q29. Two angles the sum of whose measure is 90^∘  are called ______ angles.
(a) Supplementary
(b) Complementary
(c) Corresponding
(d) None of these
Ans: (b)
Sol: By definition we know that two angles, the sum of whose measure is 90^∘  are called complementary angles. Then, such angles are called complement of each other.
Therefore, option B is correct.

Q30. The supplement of an acute angle is a/an __________ angle.
(a) Acute
(b) Obtuse
(c) Right
(d) Straight
Ans: (b)
Sol: We know acute angle  <90^∘
Let us take an example.
∠x = 45^∘ .....x is an acute angle
Supplement of x is
180^∘ - 45^∘ = 135^∘ ....it is an Obtuse angle
Obtuse angle  >90^∘ .