Class 10 Maths Chapter 13 Practice Question Answers - Statistics
Q1. Value of the middle-most observation(s) is called:
(a) Mean
(b) Median
(c) Mode
(d) None of these
Ans: (b)
Sol:
To find the Median, place the numbers in value order and find the middle number.
If there are two middle numbers, take the mean of the two numbers and this will be the median of the data set.
The middle most observation of a data series is called the median of the series.
Q2. If the mode of the following data 10,11,12,10,15,14,15,13,12,x,9,7 is 15, then the value of x is:
(a) 10
(b) 15
(c) 12
(d) 21/2
Ans: (b)
Sol:
The mode is the value which occurs the most often in a given set of data.
In the data set provided here, the mode is 15, hence 15 should occur the most number of times.
10,11,12,10,15,14,15,13,12,x,9,7
Excluding x and arranging these numbers in ascending order,
7,9,10,10,11,12,12,13,14,15,15
Here 10, 12 and 15 are occurring twice.
Since 15 is the mode, it should occur more than twice.
Therefore, the value of x should be 15 if the value of mode is 15.
Q3. The mode of the following series is 36. Find the missing frequency in it (a) 10
(a) 10
(b) 15
(c) 16
(d) 12
Ans: (a)
Sol:
We know that, 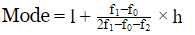
Where l is lower limit of the modal class, $$h$$ is size of the class interval, f1 = frequency of the modal class, f0 = frequency of the class preceding the modal class, f2 = frequency of the class succeeding the modal class
Since the class 30−40 has the highest frequency 16, it is the modal class.
Q4. Which of the following is a measure of central tendency?
(a) Mean
(b) Median
(c) Mode
(d) All of the above
Ans: (d)
Sol:
Step -1: Writing the measures of central tendency.
As central tendency is a tendency for a set of values to gather around the middle of the set.
And mean, median and mode are the measures of central tendency.
Step -2: Finding the correct option.
∴Option A, B, C all three are true.
Hence, the correct option is D.
Q5. The mean and median of same data are 24 and 26 respectively. The value of mode is :
(a) 23
(b) 26
(c) 25
(d) 30
Ans: (d)
Sol:
Given that mean =24 and median =26
We know that mode=3median−2mean
⟹mode=3(26)−2(24)=78−48=30
Q6. Find the mode of the following data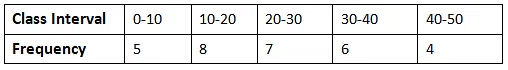
(a) 16.5
(b) 17.5
(c) 18
(d) 18.5
Ans: (b)
Sol:
As 8 is the highest frequency, modal class is 10−20 and the mode lies in this class
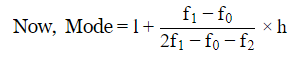
Where l1 lower limit of the modal class
f1 = frequency of the modal class
f0 = frequency of the class preceding the modal class
f2 = frequency of the class succeeding the modal class
h= size of the modal class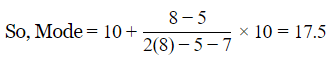
Q7. Median divides the total frequency into _____ equal parts.
(a) 2
(b) 3
(c) 4
(d) None of the above
Ans: (a)
Sol:
The median of the data series is the middle term or the mean of the two middle terms.
Hence, it divides the data series or the frequency of terms into two equal halves.
Q8. The lower limit of the modal class of the following data is :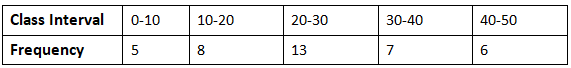
(a) 10
(b) 30
(c) 20
(d) 50
Ans: (c)
Sol:
Modal is the class which has the highest frequency.
Therefore, the highest frequency is 13 which occurs in the interval 20−30
Lower limit of the class is the smallest value of the class.
Therefore, the lower limit of the class 20−30 is 20
Q9. The distribution below gives the weights of 30 students of a class. Find the median weight of the students.
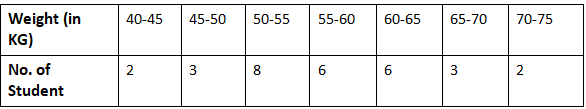
Sol: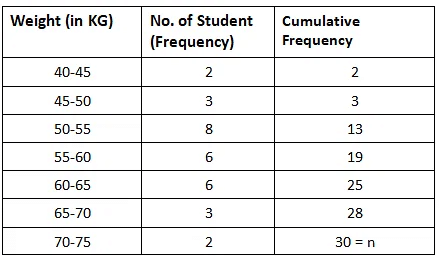 We have, n=30
We have, n=30
n/2=15
The cumulative frequency just greater than n/2 is 19 and the corresponding class is 55–60.
Thus, 55–60 is the median class such that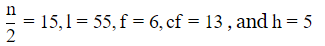
Substituting these values in the formula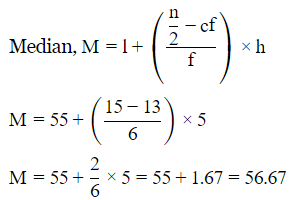
Hence, the median weight =56.67 kg .
Q10. 2(Median−Mean)=Mode−Mean
(a) True
(b) False
(c) Neither
(d) Either
Ans: (b)
Sol:
2(Median−Mean)=Mode−Mean
2(Median)=Mode + Mean
This essentially means that the statement is saying that the Median is the average of Mode and Mean in the given data, which is not correct.
Q11. If in a frequency distribution, the mean and median are 21 and 22 respectively, then its mode is approximately
(a) 22.0
(b) 20.5
(c) 25.5
(d)24.0
Ans: (d)
Sol:
We have,
Mode+2×Mean=3×Median
⇒Mode=3×Median−2×Mean
Given : Mean=21 and Median=22
Putting the given values, we get
Mode=3×22−2×21=66−42=24.
Hence, option D is correct.
Q12. The modal value is the value of the variate which divides the total frequency into two equal parts.
(a) True
(b) False
(c) Neither
(d) Either
Ans: (b)
Sol:
False. Modal value is the value which occurs maximum number of times in the data.
Q13. In a moderately skewed distribution the values of mean and median are 4 and 5 respectively. The value of mode is approx.
(a) 4
(b) 5
(c) 6
(d) 7
Ans: (d)
Sol:
Mode = 3× median −2× mean
=3×5−2×4
=15−8=7
Hence, option 'D' is correct
Q14. State true or false: The mode is the most frequently occurring observation.
(a) True
(b) False
(c) Can't determine
(d) None of these
Ans: (a)
Sol:
The observation occurring the most number of times or which has highest frequency is called the mode.
Thus, the given statement is true.
Q15. The abscissa of the point of intersection of the less than type and of the more than type cumulative frequency curves of a grouped data gives its:
(a) mean
(b) median
(c) mode
(d) all the three above
Ans: (b)
Sol:
The ogive is the free hand graph.
The abscissa (X-coordinate) of the intersection of the less than ogive and more than ogive gives the median of the data.
In the above graph, the intersection point is (15,40). The X-coordinate 15 is the median.
|
127 videos|584 docs|79 tests
|
















