Class 9 Maths Chapter 10 Practice Question Answers - Heron’s Formula
Q1: The sides of a triangle are 3 cm, 4 cm and 5 cm. Its area is .......
(a) 12cm2
(b) 15cm2
(c) 20cm2
(d) 6cm2
Ans: (d)
By Heron's formula,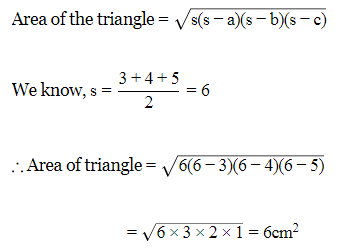
Q2: Area of an equilateral triangle of side a units can be calculated by using the formula :
(a)
(b)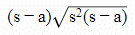
(c) 
(d) 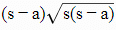
Ans: (d)
We know that for an equilateral triangle, a=b=c
By Heron's formula, we get
So, area of given equilateral triangle
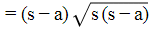
Q3: The area of an equilateral triangle with side 2√3cm is
(a) 5.196cm2
(b) 0.866cm2
(c) 3.496cm2
(d) 1.732cm2
Ans: (a)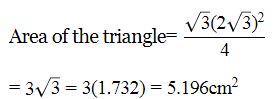
Q4: The sides of a triangle are 4,5 and 6 cm. The area of the triangle is equal to
(a) 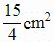
(b) 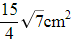
(c) 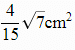
(d) None of these
Ans: (b)
Given that, a = 4 cm, b = 5 cm, and c = 6 cm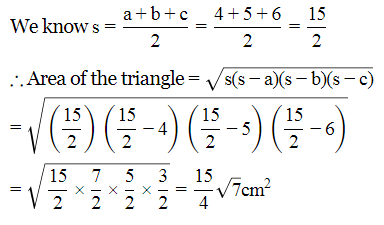
Q5: Heron's formula is :
(a) 
(b) 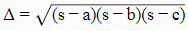
(c) 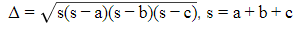
(d) 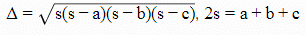
Ans: (d)
Step -1: A triangle having side lengths as a, b and c.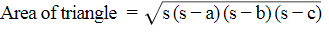
Where, s is the semi perimeter of the triangle; 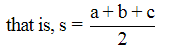
Thus, the Heron’s formula is 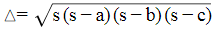 Where, 2s = a + b + c
Where, 2s = a + b + c
Hence, option D is correct.
Q6: Area of traingle ABC whose sides are 24m, 40m and 32m is-
(a) 96m2
(b) 384m2
(c) 43m2
(d) 192m2
Ans: (b)
Area of triangle with sides a, b and c and 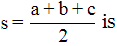
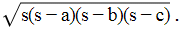
For triangle with sides 24 m, 40 m and 32 m, 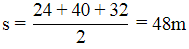
Area of the triangle with sides 24 m, 40 m and 32 m =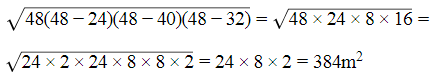
Hence, option 'B' is correct.
Q7: Find the area of a triangle whose two sides are 18 cm and 10 cm and the perimeter is 42 cm.
(a) 83.67 cm2
(b) 46.12 cm2
(c) 77.15 cm2
(d) 69.69 cm2
Ans: (d)
Let a, b and c be the sides of triangle.
Perimeter =a + b + c = 42 cm
a = 18, b = 10
As a + b + c = 42
∴ c = 42 − b − a = 42 − 18 − 10
∴c=14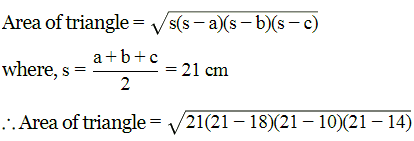
Q8: The sides of a triangle are 56 cm, 60 cm and 52 cm long. Then the area of the triangle is
(a) 1322 cm2
(b) 1311 cm2
(c) 1344 cm2
(d) 1392 cm2
Ans: (c)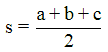
By Heron's formula,Area of the triangle 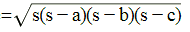
Where a,b,c are sides of the triangle.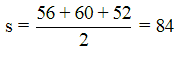
Area of the triangle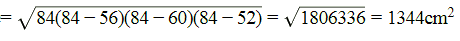
Q9: Find the cost of laying grass in a triangular field of sides 50m,65m and 65m at the rate of Rs 7 per m2.
(a) Rs 10500
(b) Rs 11375
(c) Rs 10000
(d) Rs 21000
Ans: (a)
Sides of the triangle are a =50m, b = 65m, c = 65m
Area of triangle, by Heron's formula 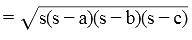
where,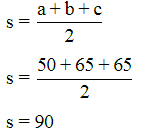
Area of triangle 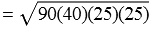
Area of triangle = 1500m2
Cost of laying grass = Area × 7
Cost of laying grass =1500 × 7
Cost of laying grass = Rs 10500
Q10: The area of an isosceles triangle having base 2 cm and the length of one of the equal sides 4 cm, is
(a) 
(b) 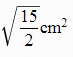
(c) 
(d) 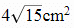
Ans: (a)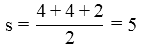
Area of the triangle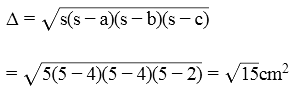
Q11: The triangular side - wall of a flyover have been used for advertisements, The sides of the walls are 122 m, 22m and 120 m. The advertisements requires rent of Rs 5000 per m2 per year. A company hired one of its walls for 3 months. How much rent did it pay ?
(a) 1750000
(b) 1600000
(c) 1650000
(d) None of these
Ans: (c)
Sides of a triangular wall is 122m,22m,120m.
Hence, area of triangle 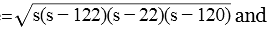
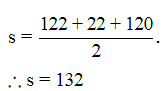
∴Area of triangular wall = 1320m2
Since, The advertisements yeild an earning of Rs 5000 per m2 per year.
Hence, Earning per 3 months = 5000/4 = 1250 per m2
∴ Rent paid = 1250(1320) = 1650000 Rs.
Q12: A traffic signal board, indicating 'SCHOOLAHEAD', is an equilateral triangle with side a. Find the area of the signal board, using Heron's formula. If its perimeter is 180cm, what will be the area of the signal board?
(a) 900√3 cm2
(b) 300√3 cm2
(c) 600√3 cm2
(d) 900 √2 cm2
Ans: (a)
Area of triangle 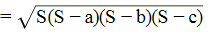
Perimeter =180cm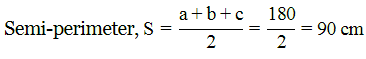
Given triangle is an equilateral triangle, therefore a = b = c
Perimeter =3a
180 = 3a ⇒ a = 60
a = b = c = 60 cm
Area of triangle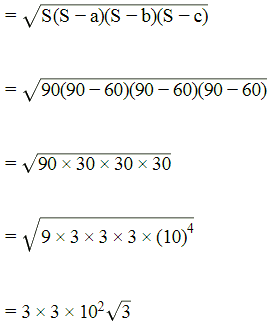
=900√3cm2
Q13: Two sides of a plot measure 32 m and 24 m and the angle between them is a perfect right angle The other two sides measure 25 m each and the other three angles are not right angles What is the area of the plot? (a) 768 m2
(a) 768 m2
(b) 534 m2
(c) 696.5 m2
(d) 684 m2
Ans: (d)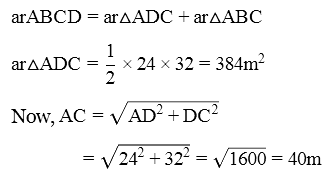
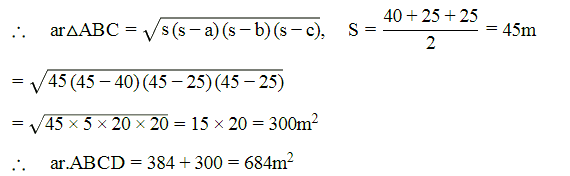
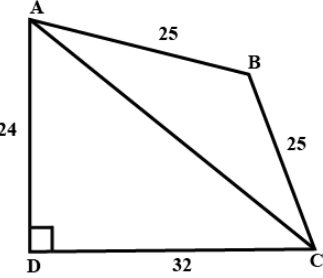
Q14: If each sides of a triangle is double, then find the ratio of area of the new triangle thus formed and the given triangle.
Ans: Let a triangle whose sides are e(a).
New triangle whose area is 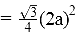
Let a triangle is equilateral
Then, its area 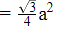
Ratio of area of new triangle to gine triangle is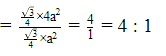
Q15: Find the area of a triangle whose sides. are 18 cm , 10 cm and 14 cm.
Ans: Given sides are 18cm,10cm and 14cm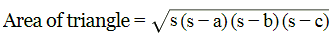
⇒ a = 18cm, b = 10 cm and c = 14cm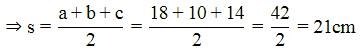
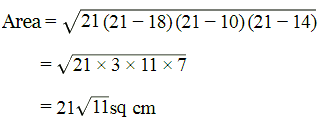
Hence, the answer is 21√11 sqcm.
|
40 videos|421 docs|51 tests
|





















